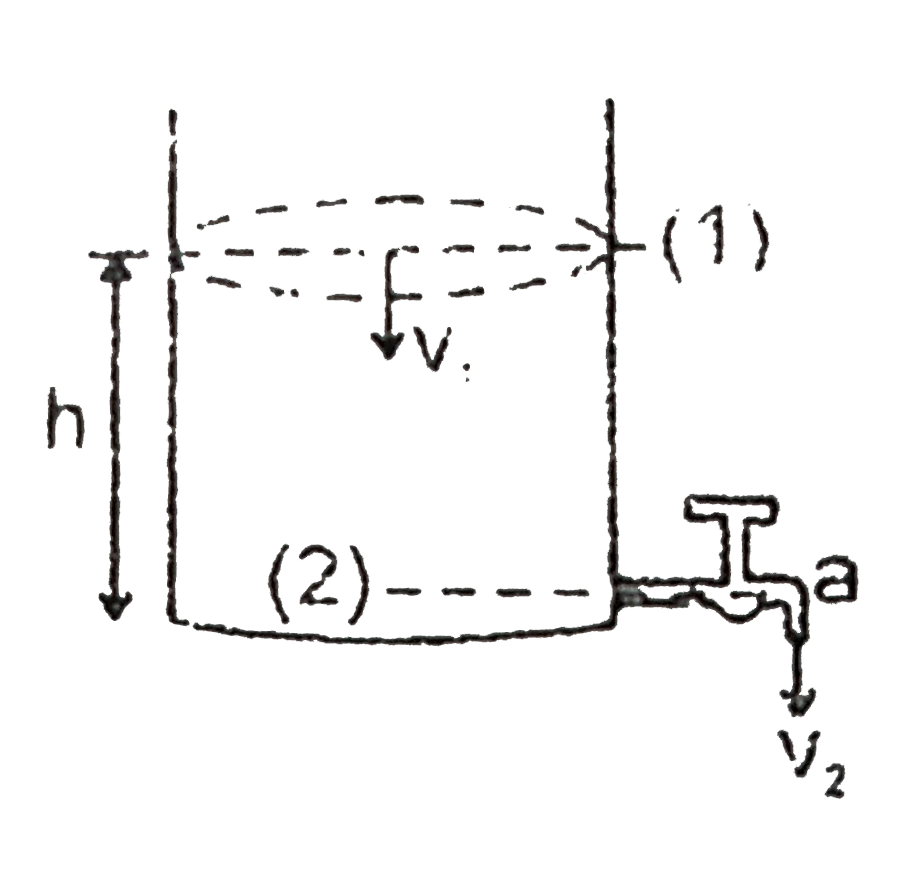
A cylindrical container of cross-section area, A is filled up with water upto height 'h' Water may exit through a tap of cross sectional area 'a' in the bottom of container. Find out

(a) Velocity of water just after opening of tap.
(b) The area of cross-section of water stream coming out of tape at depth h0 below tap in terms of 'a' just after opening of tap.
(c) Time in which conainer becomes empty.
(Given : (aA)1/2=0.22,h=20cm,h0=20cm
Applying Bernoulli's between (1) and (2).
Pa+ρgh+12ρv21=Pa+12ρv22
Through continuity equation :
Av1=av2,v1=av2aρgh+12ρv21=12ρv22
on solving - v2=
⎷2gh1−a2A2=2m/sec. .........(1)
(b) Appling Bernoulli's equation between (2) and (3)
12ρv22+ρgh0=12ρv23
Through continuity equation -
av2=a'v3⇒v3=av2a'
⇒12ρv22+ρgh0=12ρ(av2a')2
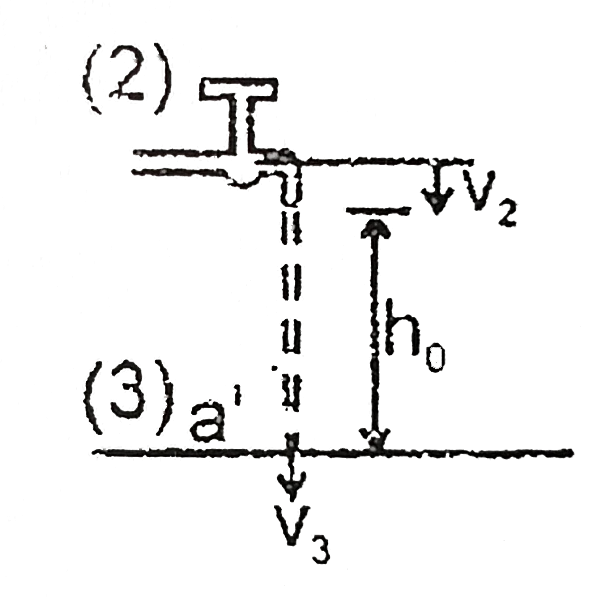
12×2×2+gh0=12(aa')2×2×2
(aa)2=1+9.8×.202⇒(aa')2=1.98⇒a'=a√1.98
(c) From (1) at any height 'h' of liquid level in container, the velocity through tap,
v=√2gh0.98=√20h
We know, volume of liquid coming out of tap = decrease in volume of liquid in container.
For any small ltime interval 'dt'
av2dt=−A⋅dx
a√20xdt=−Adx⇒t∫0dt=−Aa0∫hdx√20x
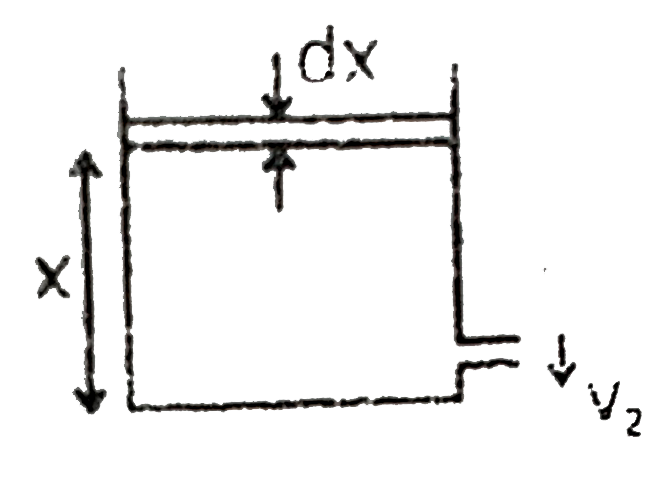
t=Aa√20[2√x]0h⇒t=Aa√202√h
=Aa×2×√h20=2Aa√0.2020=2Aa×0.1
Given (aA)1/2=0.2 or Aa=10.0004=2500
Thus t=2×2500×0.1=500 second.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - RESONANCE ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Board Level Exercise
18 Videos
- FLUID MECHANICSBOOK - RESONANCE ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Exercise- 1 PART - I Section (A)
3 Videos
- FLUID MECHANICSBOOK - RESONANCE ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Advanced Level Problems
8 Videos
- ELECTROSTATICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise
52 Videos
- FULL TEST 1BOOK - RESONANCE ENGLISHCHAPTER - FULL TEST 1EXERCISE - Exercise
30 Videos
Similar Questions
A cylindrical vessel of area of cross section A is filled with a liquid up to a height H. A very small hole of area of cross section a is made at the bottom of the vellel. Find the time taken by the vessel to become empty.
View SolutionIf water falls from a tap, then the volume rate of flow of water at depth of h, (A0 is the area of cross-section of the mouth and A02 is the corresponding area at depth h)
View SolutionA large cylindrical tank has a hole of area A at its bottom. Water is oured in the tank by a tube of equal cross sectional area A ejecting water at the speed v.
View SolutionA cylindrical vessel of area of cross-section A is filled with water to a height H. It has capillary tube of length l and radius r fitted horizontally at its bottom. If the coeffiecient of viscosity of water is η, then time required in which level will fall to a height H2 is (density of water is ρ)
View SolutionWill the height of the water column change if the cross section of the water column is made wider ?
View SolutionIf cross- sectional area of limb I is A1 and that of limb II is A2 then velocity of the liquid in the tube will be, (cross- sectional area of tube is very small)

View SolutionWater rises to a height h in a capillary tube of cross-sectional area A. the height to which water will rise in a capillary tube of cross-sectional area 4A will be
View SolutionWater rises to a height h in a capillary tube of cross-sectional area A. the height to which water will rise in a capillary tube of cross-sectional area 4A will be
View SolutionA cylinder containing water upto a height of 25cm has a hole of area of cross section 14cm2 in its bottom. It is counterpoised in a balance. The initial change in its weight, when water just starts flowing out is
View SolutionThe water flowing through the same tube having two different cross section areas has different flow rates .
View Solution
