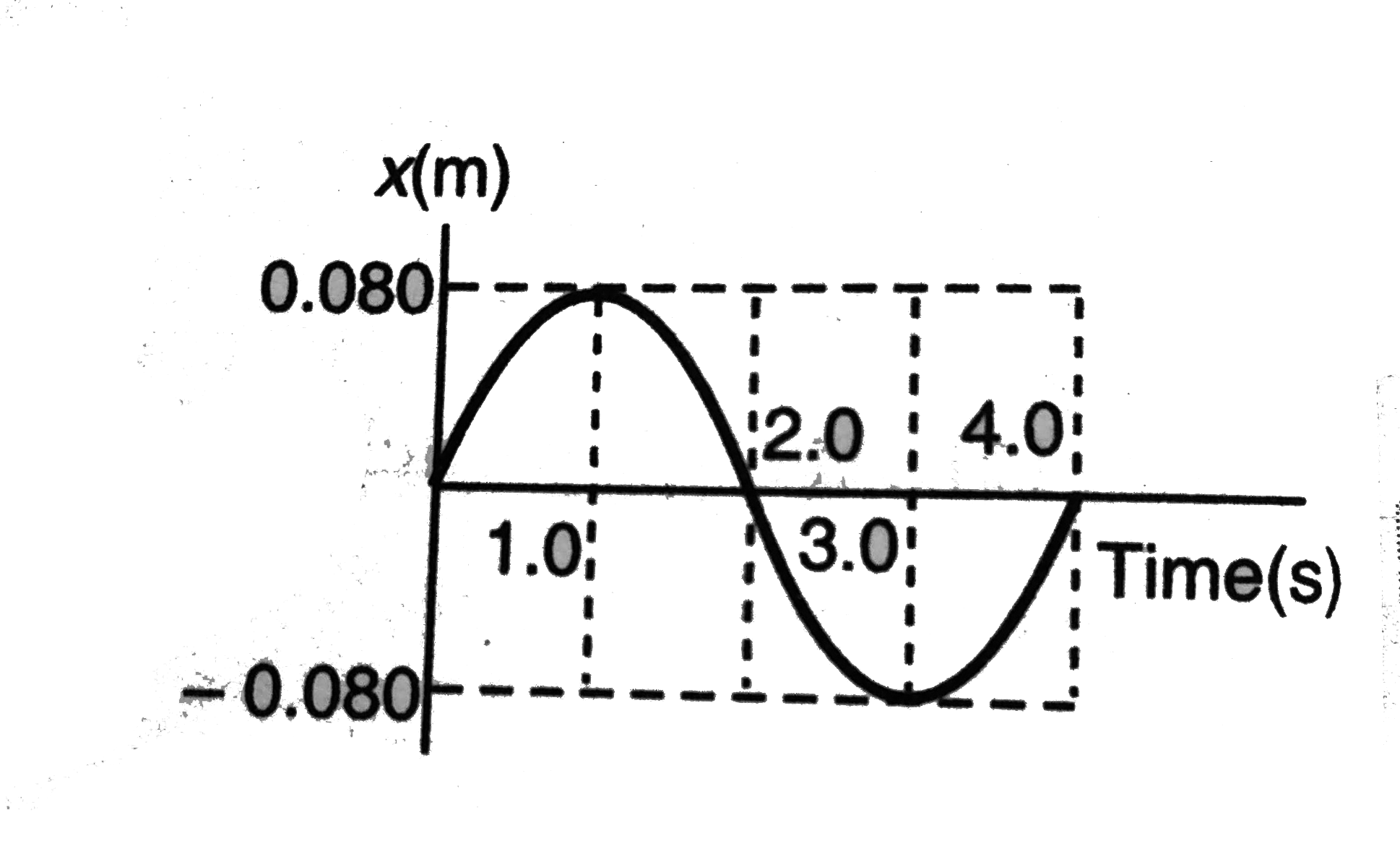
An object of mass 0.8kg is attached to one end of a spring and the system is set into simple harmonic motion. The displacement x of the object as a fuction of time is shown in the figure. With the help of the data, determine
(a) the amplitude A of the motion,
(b) the angular frequency ω,
( c) the spring constant K,
(d) the speed of the object at t=1.0s and
(e) the magnitude of the object's acceleration at t=1.0s.
zero.
1.57m/s2
0.197m/s2
0.157m/s2
From the figure
T=4sec
ω=2πT=π2=1.57rad/sec
ω=√km⇒k=(0.8)(π2)2=1.97N/m
|a|=ω2x=0.197m/s2
Topper's Solved these Questions
Similar Questions
An object of mass 0.8kg is attached to one end of a spring and the system is set into simple harmonic motion. The displacement x of the object as a fuction of time is shown in the figure. With the aid of the data, determine
(a) the amplitude A of the motion,
(b) the angular frequency ω,
( c) the spring constant K,
(d) the speed of the object at t=1.0s and
(e) the magnitude of the object's acceleration at t=1.0s.
 View Solution
View SolutionA body of mass m attached to one end of an ideal spring of force constant k is executing simple harmonic motion. Establish that the time - period of oscillation is T=2π√m/k.
View SolutionThe displacement of a particle in simple harmonic motion in one time period is
View SolutionA 5kg mass bounces in simple harmonic motion at the end of a spring. At which point the acceleration of the mass is the greatest?
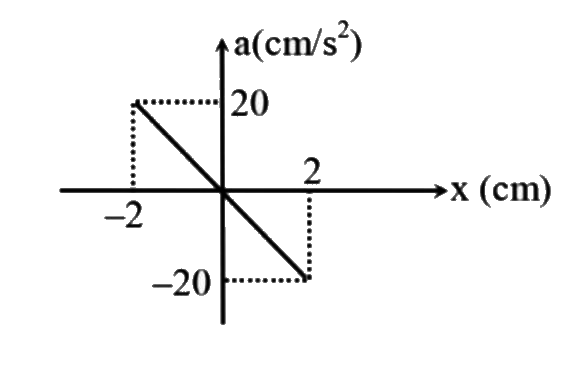
View SolutionThe acceleration-displacement graph of a particle executing simple harmonic motion is shown in the figure. The frequency of oscillation is
 View Solution
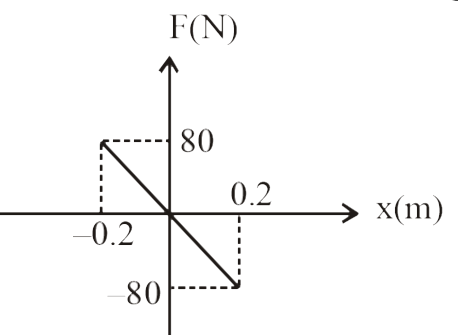
View SolutionA body of mass 0.01 kg executes simple harmonic motion (SHM) about x=0 under the influence of a force shown in the figure. The period of the SHM is
 View Solution
View SolutionA body of mass 0.01 kg executes simple harmonic motion about x = 0 under the influence of a force as shown in figure. The time period of SHM is
 View Solution
View SolutionAs shown in figure in a simple harmonic motion oscillator having identical four springs has time period
 View Solution
View SolutionThe acceleration-displacement (a−X) graph of a particle executing simple harmonic motion is shown in the figure. Find the frequency of oscillation.
 View Solution
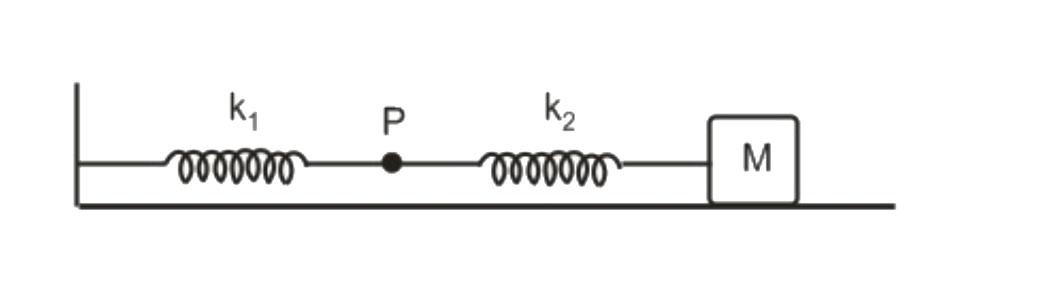
View SolutionThe mass M shown in figure ocillates in simple harmonic motion with amplitude A.
The amplitude of the point P is
 View Solution
View Solution

