Two identically charged particles are fastened to the two ends of a spring of spring constant 100Nm−8C, find the extension In the length of the apring. Assume that the extension is small as scompared to the natural length . Justify this assumption after you solve the problem.Solution in Gujrati
E≤ctricforce=spr∈gforce
14πε0q2(x+0.1)2=K(0.1+x)
or(0.1+x)3=9×109×(2.0×10−8)210−2
=36×109×10−1610−2
=36×10−5
orx=3.6×10−6m
Topper's Solved these Questions
- ELECTRIC FIELD AND POTENTIALBOOK - HC VERMACHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Objective 2
8 Videos
- ELECTRIC CURRENT THROUGH GASESBOOK - HC VERMACHAPTER - ELECTRIC CURRENT THROUGH GASESEXERCISE - Exercises
23 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE
9 Videos
Similar Questions
Mark out the correct potons.
View SolutionA discharge tube contains helium at a low pressure. A large potential difference is applied across the tube. Consider a helium atom that has just been ionized due to the detachment of an atomic electron. Find the ratio of the distance travelled by the free electron to that by the positive ion in a short time dt after the ionization.
View SolutionShown a metallic square frame of edga a in a vertical plane. A uniform magnetic field B exists in the space in a directon perpendicular to the plane of the figure. Two boys pull the opposite corners of the square ot deform it into a rhombus. They start pulling the corners t t=0 and sisplace the corners at a uniform speed u. (a) find the induced emf in the frame at the instant when the angles at these corners reduce to 60∘. (b) find the induced current in the frame at this instatnt if the total resistance of the frame is R. (c) Find the total charge which flows through a side of the frame by the time the square is deformed into a straight line.
View SolutionTwo identically charged particles are fastened to the two ends of a spring of spring constant 100Nm−8C, find the extension In the length of the apring. Assume that the extension is small as scompared to the natural length . Justify this assumption after you solve the problem.
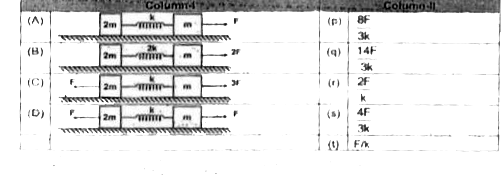
View SolutionA block of mass 'm' is attached to a spring in natural length of spring constant 'k'. The other end A of the spring is moved with a constat velocity v away from the block . Find the maximum extension in the spring.

View SolutionThe system is released from rest with spring intially in its natural length. If mass of the block m = 10 kg. and spring constant k = 100 N//m , then maximum extension in spring is :
View SolutionNatural length of a spring is 60 cm and its spring constant is 4000 N/m. A mass of 20 kg is hung from it. The extension produced in the spring is (Take, g = 9.8 m//s^(2) )
View SolutionA block ofmass m is attached with a spring in its natural length, of spring constant k. The other end A of spring is moved with a constant acceleration 'a' away from the block as shown in the figure -3.74 . Find the maximum extension in the spring. Assume that initially block and spring is at rest w.r.t ground frame:
View SolutionIn spring constant of the spring is 100 N//m Extension produced in the spring is: (g=10 m//s^(2))
View SolutionA mass m attached to spring of natural length l0 and spring constant k. One end of string is attached to centre of disc in horizontal plane which is being rotated by constant angular speed ω. Find extension per unit length in spring (given k>>mω2 ) :
View SolutionInitially spring is in natural length and both blocks are in rest condition. Then deter mine maximum extension in spring . K = 20 N/M
View SolutionForce constant of a spring is 100 N//m . If a 10kg block attached with the spring is at rest, then find extension in the spring. ( g= 10 m//s^(2) )
View SolutionIn the situations shown in the figure surfaces are frictionless. Find the maximum extension of the springs if blocks are initially at rest and springs are initially in natural lengths.
View SolutionTwo identically charged particles are fastened to the two ends of a spring of spring constant 100Nm−1, find the extension In the length of the spring. Assume that the extension is small as compared to the natural length . Justify this assumption after you solve the problem.
View SolutionA mass m attached to spring of natural length l0 and spring constant k. One end of string is attached to centre of disc in horizontal plane which is being rotated by constant angular speed ω. Find extension per unit length in spring (given k>>mω2 ) :
View SolutionNatural length of a spring is 60 cm and its spring constant is 4000 N/m. A mass of 20 kg is hung from it. The extension produced in the spring is (Take, g=9.8m/s2)
View Solution
