Question

A rectangular tank having base $15cm\times 20cm$ is filled with water (density $\rho =1000kg/m^3)$ up to $20cm$ and force constant $K=280N/m$ is fixed to the bottom of the tank so that the spring remains vertical.
This system is in an elevator moving downwards with acceleration $a=2m/s^2$. A cubical block of side $l=10cm$ and mass $m=2kg$ is gently placed over the spring and released gradually, as shown in the figure.

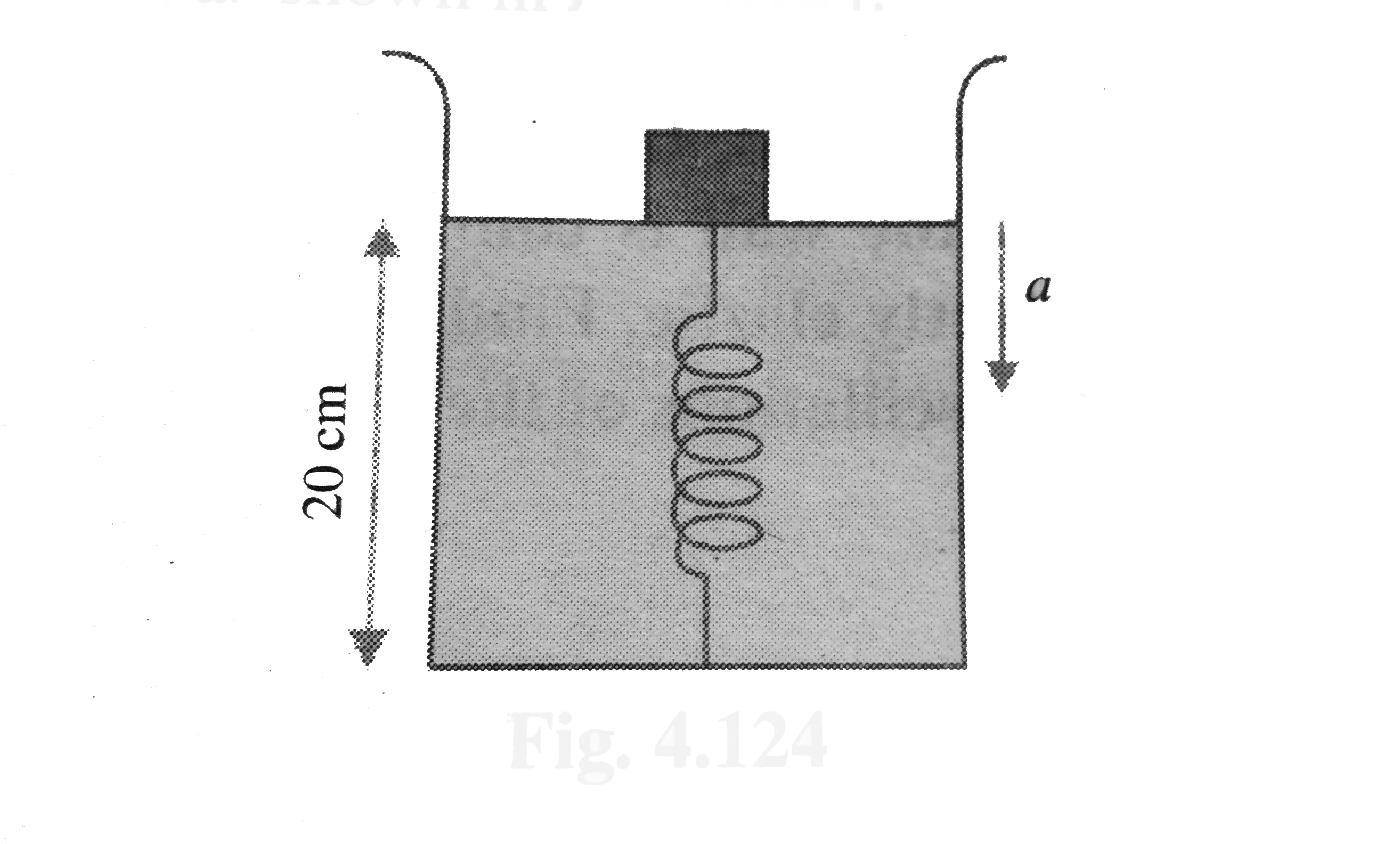
a. Calculate compression of the spring in equilibrium position.
b. If the block is slightly pushed down from equilibrium position and released, calculate frequency of its vertical oscillations.

Answer

`2 pi sqrt(m {((l)/(b))^(2) (l)/(k_(1)) + (l)/(k_(2))}`

Explanation

Let, in equlibrium position, compression of spring be x Liquid of valum `l^(2)x` is displaced from its original position and level of liquid in tank rises as shown in figure

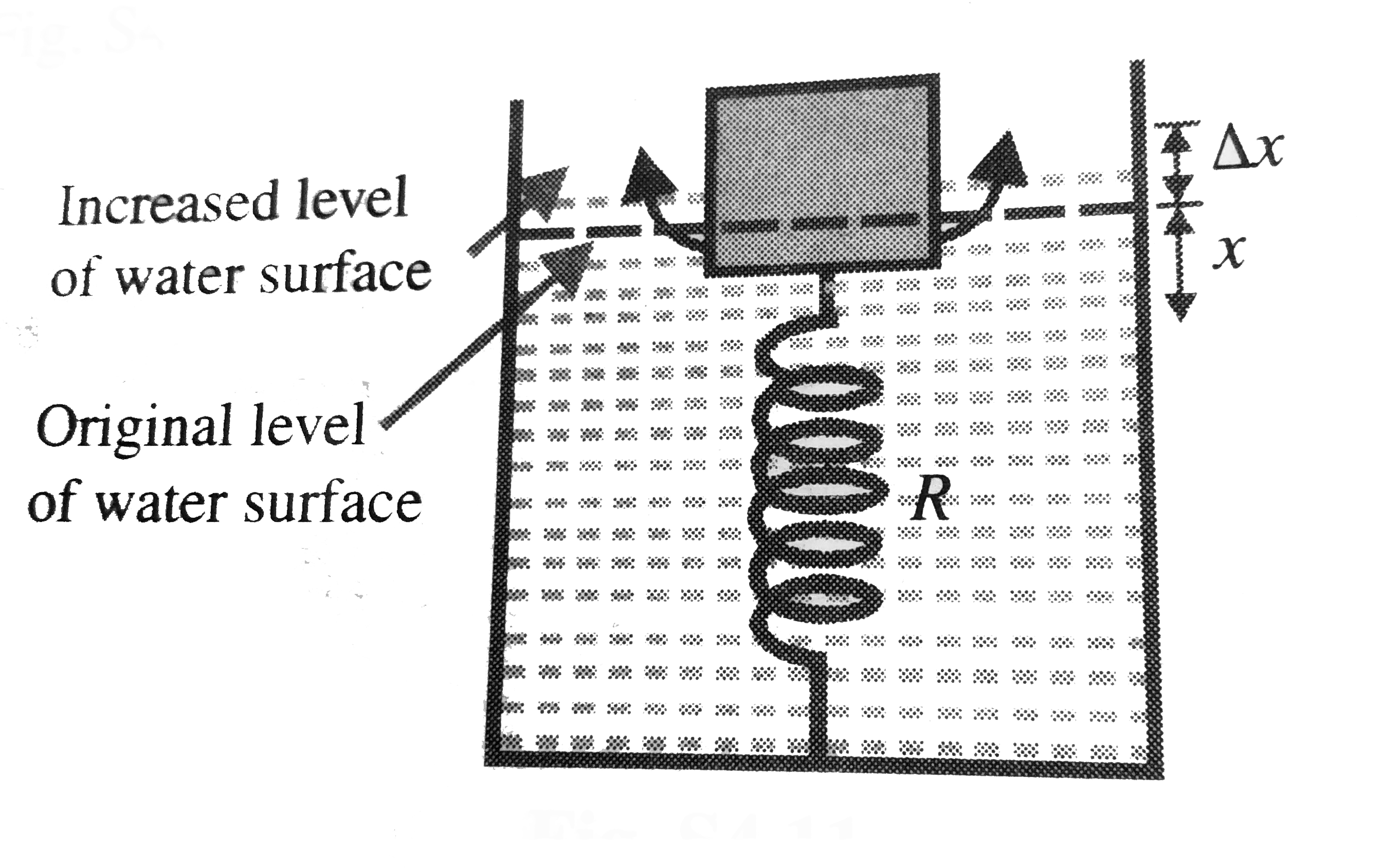
This rise level, `Delta x = (l^(2) x)/(A - l^(2))`
where `A = 15 cm xx 20 cm` (have area of tank).
`Delta x = 0.5 x`
Mass of water displaced by the block is
`l^(2) (x + Delta x) rho = 15 x kg`
Upthrust exerted by water = apparent weight of water displaced.
Upthrust `F_(1) = 1.5 x (g - a) = 120 x N`
Upward force exerted by spring. `F_(2) = kx = 280 x`
Considering free body diagram of the block .
`mg - (F_(1) - F_(2)) = ma`
Subsituting value of `F_(1) and F_(2), x = 0.04 m = 4 cm`
If the block is slightly pushes downwards by dx, both `F_(1) and F_(2)`, increase.
Increase in `F_(1) is dF_(1) = 120 dx`
Increase in `F_(2) is dF_(2) = 280 dx`
Restoring force on block = increase in `F_(1) + "increase in" F_(2)`
` dF_(1) + dF_(2) = (120 dx + 280 dx) = 400 dx`
Restoring acceleration `= (400 dx)/(m) = 200 dx`
Since restoring acceleration or displacement (dx), block performs SHM.
Hence, frequency
`f = (1)/(2 pi) sqrt(("displacement")/("acceleration")) = (1)/(2 pi) sqrt 200 = (5sqrt2)/(pi)` per second