A ring of radius R is first rotated with an angular velocity ω and then carefully placed on a rough horizontal surface. The coefficient of friction between the surface and the ring is μ. Time after which its angular speed is reduced to half is
ω0μR2g
ω0g2μR
2ω0Rμg
ω0R2μg
The correct Answer is:D
To solve the problem step by step, we will analyze the situation involving a ring rotating on a rough surface and determine the time it takes for its angular speed to reduce to half.
Step 1: Understand the Forces Acting on the Ring
When the ring is placed on the rough surface, the frictional force acts to oppose the motion of the ring. The forces acting on the ring are:
- The gravitational force (weight) acting downward, mg.
- The normal force N acting upward, which balances the weight, so N=mg.
- The frictional force f acting opposite to the direction of rotation.
Step 2: Calculate the Torque Due to Friction
The torque τ acting on the ring due to the frictional force can be expressed as:
τ=f⋅R
where f is the frictional force and R is the radius of the ring.
The maximum static frictional force is given by:
f=μN=μmg
Thus, the torque becomes:
τ=μmgR
Step 3: Relate Torque to Angular Acceleration
According to Newton's second law for rotation, the torque is also related to the moment of inertia I and angular acceleration α:
τ=Iα
For a ring, the moment of inertia I is given by:
I=mR2
Substituting this into the torque equation gives:
μmgR=mR2α
Step 4: Solve for Angular Acceleration
We can cancel m and R from both sides (assuming R≠0):
μg=Rα⇒α=μgR
Step 5: Set Up the Equation for Angular Velocity
The angular velocity of the ring changes with time according to the equation:
ω=ω0+αt
where ω0 is the initial angular velocity and α is the angular acceleration.
Step 6: Find the Time When Angular Velocity is Halved
We need to find the time t when the angular velocity is reduced to half:
ω02=ω0−αt
Rearranging gives:
ω02=ω0−μgRt
ω02−ω0=−μgRt
−ω02=−μgRt
t=ω0R2μg
Final Answer
The time after which the angular speed of the ring is reduced to half is:
t=ω0R2μg
---
When the ring is placed on the rough surface, the frictional force acts to oppose the motion of the ring. The forces acting on the ring are:
- The gravitational force (weight) acting downward, mg.
- The normal force N acting upward, which balances the weight, so N=mg.
- The frictional force f acting opposite to the direction of rotation.
The torque τ acting on the ring due to the frictional force can be expressed as:
τ=f⋅R
where f is the frictional force and R is the radius of the ring.
f=μN=μmg
Thus, the torque becomes:
τ=μmgR
According to Newton's second law for rotation, the torque is also related to the moment of inertia I and angular acceleration α:
τ=Iα
For a ring, the moment of inertia I is given by:
I=mR2
Substituting this into the torque equation gives:
μmgR=mR2α
We can cancel m and R from both sides (assuming R≠0):
μg=Rα⇒α=μgR
The angular velocity of the ring changes with time according to the equation:
ω=ω0+αt
where ω0 is the initial angular velocity and α is the angular acceleration.
We need to find the time t when the angular velocity is reduced to half:
ω02=ω0−αt
Rearranging gives:
ω02=ω0−μgRt
ω02−ω0=−μgRt
−ω02=−μgRt
t=ω0R2μg
The time after which the angular speed of the ring is reduced to half is:
t=ω0R2μg
Topper's Solved these Questions
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - More than one option is correct
36 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Comprehension Type Questions
40 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions
17 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Subjective Questions
2 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
Similar Questions
A uniform disc of radius R is spinned to the angular velocity ω and then carefully placed on a horizontal surface. How long will the disc be rotating on the surface if the friction coeffiecient is equal to k? The pressure exerted by the disc on the surface can be regarded as uniform.
View SolutionA body of mass m and radius r is rotated with angular velocity ω as shown in the figure & kept on a surface that has sufficient friction then the body will move
View Solution
Knowledge Check
A ring of radius R is rotating with an angular speed ω0 about a horizontal axis. It is placed on a rough horizontal table. The coefficient of kinetic friction is μk. The time after it starts rolling is.
AωoμkR2gBωog2μkRC2ωoRμkgDωoR2μkg
A uniform disc of mass m and radius R is rotated about an axis passing through its center and perpendicular to its plane with an angular velocity ω. It is placed on a rough horizontal plane with the axis of the disc keeping vertical. Coefficient of friction between the disc and the surface is μ, find
(a). The time when disc stops rotating
(b). The angle rotated by the disc before stopping.
View SolutionA disc is given an initial angular velocity ω0 and placed on a rough horizontal surface as shown Fig. The quantities which will not depend on the coefficient of friction is/are
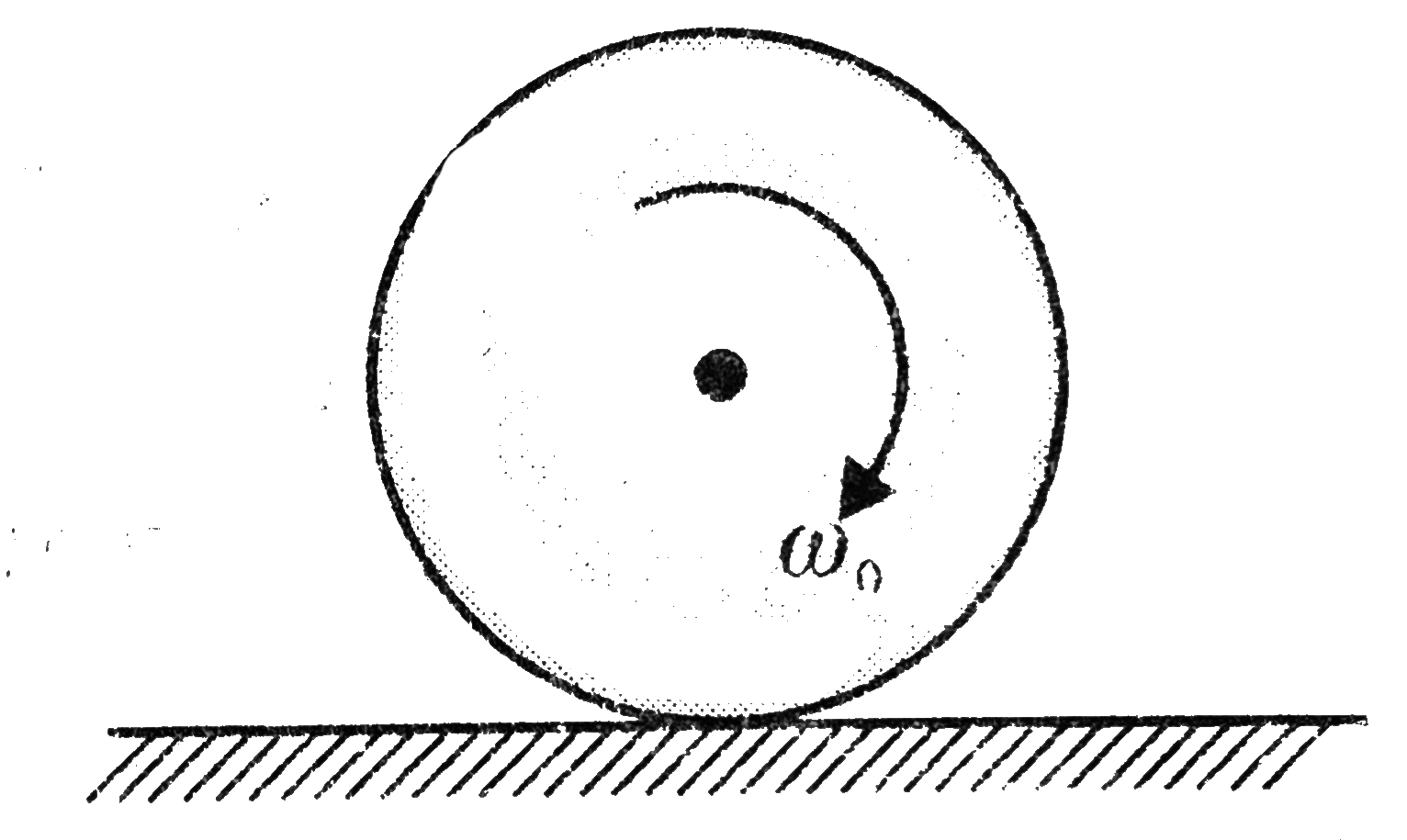
View SolutionA ring of radius 'r' and mass per unit length 'm' rotates with an angular velocity 'ω' in free space then tension will be :
View SolutionA block of mass m is pulled by a force of constant power P placed on a rough horizontal plane. The friction coefficient between the block and the surface is μ. Then
View SolutionA disc of mass m and radius R rotating with angular speed ω0 is placed on a rough surface (co-officient of friction =μ). Then

View SolutionA hoop of radius r mass m rotating with an angular velocity ω0 is placed on a rough horizontal surface. The initial velocity of the centre of the hoop is zero. What will be the velocity of the centre of the hoop when it cases to slip ?
View SolutionA block of mass m is moving on a rough horizontal surface. μ is the coefficient of kinetic friction between the block and the surface. What is the net force exerted by the surface on the block?
View Solution
