Find the area of the region enclosed by the parabola x2=y and the line y = x+ 2.
Video Solution
Text Solution
The correct Answer is:N/a
We have,x2=y and y=x+2
⇒x2=c+2
⇒x2−x−2=0
⇒x2−2x+x−2=0
⇒x(x−2)+1(x−2)=0
⇒(x+1)(x−2)=0
⇒x=−1,2
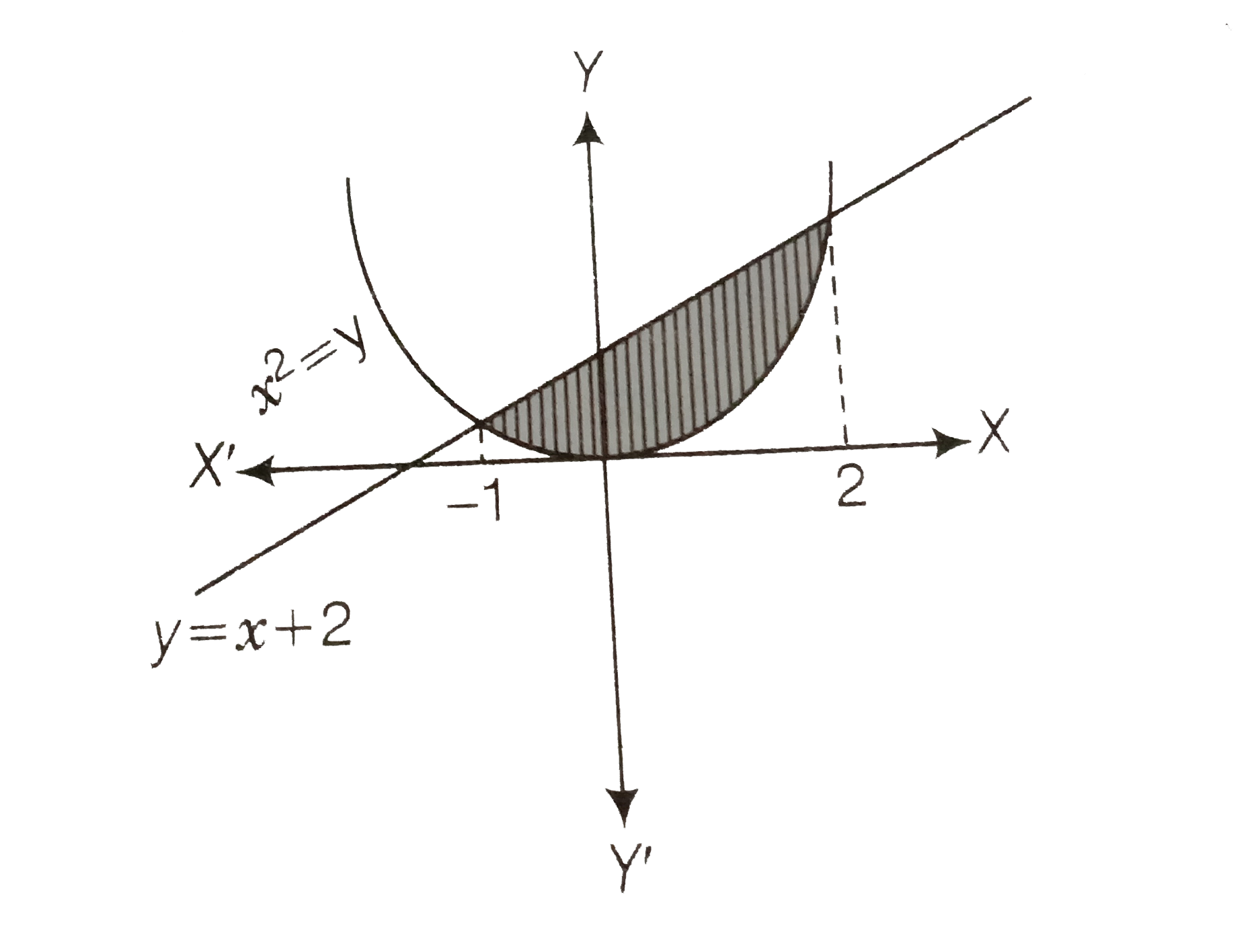
∴Required area of shaded region =∫2−1(x+2−x2)dx=[x22+2x−x33]2−1
=[42+4−83−12+2−13]
=6+32−93=36+9−186=276=92 sq units
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
The area of the region bounded by the parabola y=x2 and the line y = 4x is
A323sq.unitsB163sq.unitsC83sq.unitsD43sq.units
Find the area of the region bounded by the parabola y2=2x and the line x−y=4
View SolutionFind the area of the region bounded by the parabola x2=y, the line y=x+2 and the x-axis.
View SolutionFind the area of the region between the parabola x=y2−6y and the line x=−y
View SolutionFind the area of the region included between the parabola y2=x and the line x+y=2 .
View SolutionFind the area of the region bounded by the parabola y=x2 and y=|x|.
View SolutionFind the area of the region bounded by the parabola y=x2 and y=|x| .
View SolutionFind the area of the region bounded by the parabola y2=8x and the line x=2
View Solution
