In the given figure, ABCD is a quadrilateral in which AD= BC and ∠ADC=∠BCD. Show that the points A,B,C,D lie on a circle.
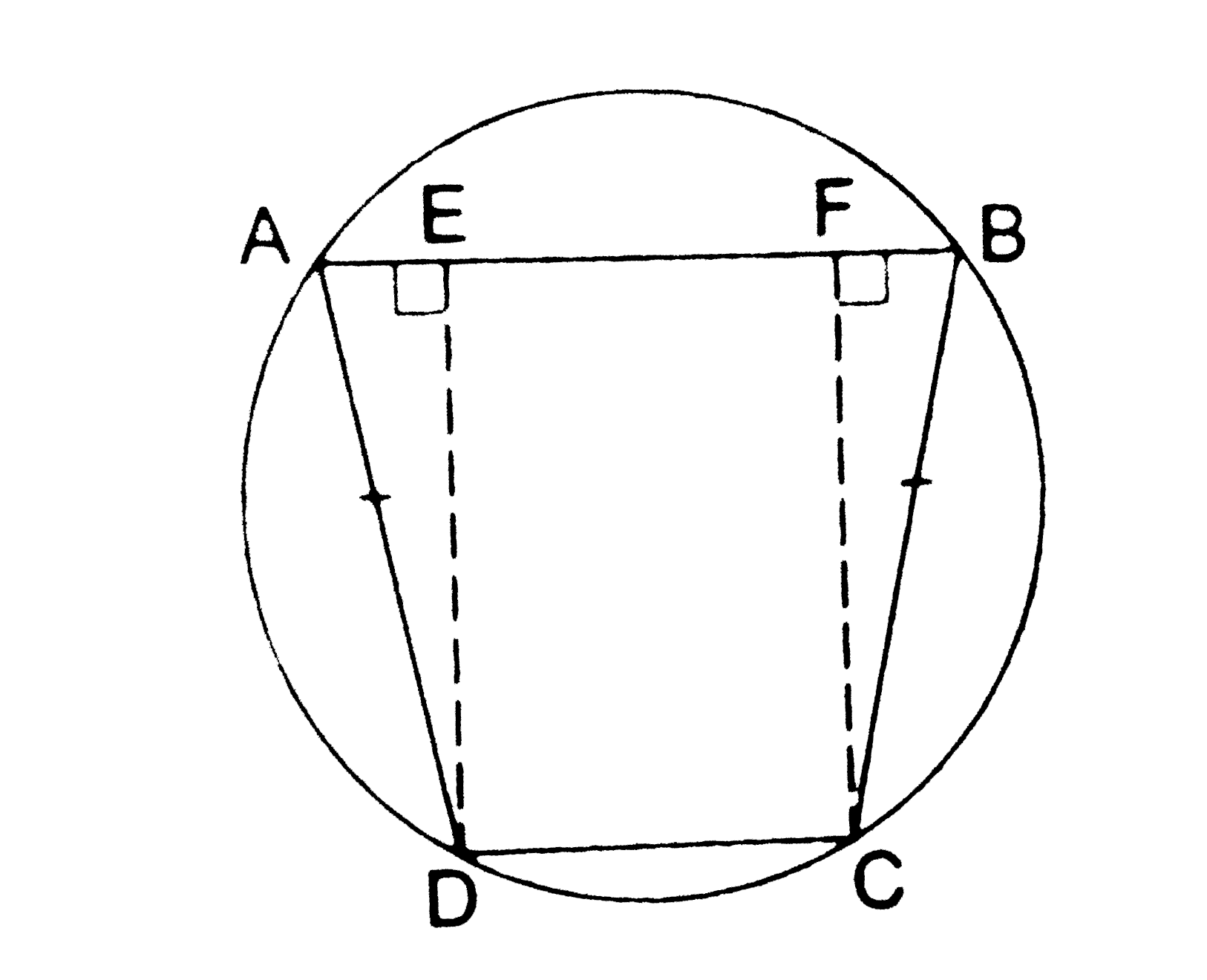
Draw DE ⊥ AB and CF ⊥ AB.
In ΔADE and ΔBCF, we have
∠ADE=∠ADC−90∘⇒∠BCD−90∘=∠BCF[∵∠ADC=∠BCD ( given) ],
and AD=BC [ given ]
∴ΔADE≅ΔBCF [ by RHS -congurence]
⇒∠A=∠B
∴∠A+∠B+∠C+∠D=360∘
⇒2∠B+2∠D=360∘[∵∠C=∠D ( given ) ]
⇒∠B+∠D=180∘ and therefore, ∠A+∠C=180∘
Hence, the points A,B,C,D are concyclic.
Topper's Solved these Questions
- CIRCLES BOOK - RS AGGARWALCHAPTER - CIRCLES EXERCISE - Multiple Choice Questions (Mcq)
5 Videos
- CIRCLES BOOK - RS AGGARWALCHAPTER - CIRCLES EXERCISE - MULTIPLE CHOICE QUESTION(MCQ)
37 Videos
- CIRCLES BOOK - RS AGGARWALCHAPTER - CIRCLES EXERCISE - EXERCISE 12B
18 Videos
- BAR GRAPH, HISTOGRAM AND FREQUENCY POLYGONBOOK - RS AGGARWALCHAPTER - BAR GRAPH, HISTOGRAM AND FREQUENCY POLYGONEXERCISE - EXERCISE 17B
16 Videos
- CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEBOOK - RS AGGARWALCHAPTER - CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEEXERCISE - Multiple Choice Questions (Mcq)
20 Videos
Similar Questions
The figure ABCD is a quadrilateral in which AB = CD and BC = AD. Its area is
View SolutionABCD is a quadrilateral in which AB=CD and AD=BC. show that it is a parallelogram.
View SolutionIf ABCD is a quadrilateral in which ABCD and AD=BC, prove that ∠A =∠B.
View SolutionABCD is a quadrilateral in which AB=BC and AD =CD ,Show that BD bisects boht the angle ABC and ADC
View SolutionIn the given figure ABCD is, ABCD is a quadrilateral in which AB∣∣DC and AD∣∣BC prove that ANGLEADC=ANGLEABC.
View SolutionABCD is a quadrilateral in which ∠ABC=∠BCD and CD>AB Prove that ∠BAD>∠ADC
View SolutionIn the given figure, ABCD is a quadrilateral in which AD = BC and ∠DAB=∠CBA.
Prove that (i) ΔABD≅ΔBAC,
(ii) BD = AC,
(iii) ∠ABD=∠BAC.
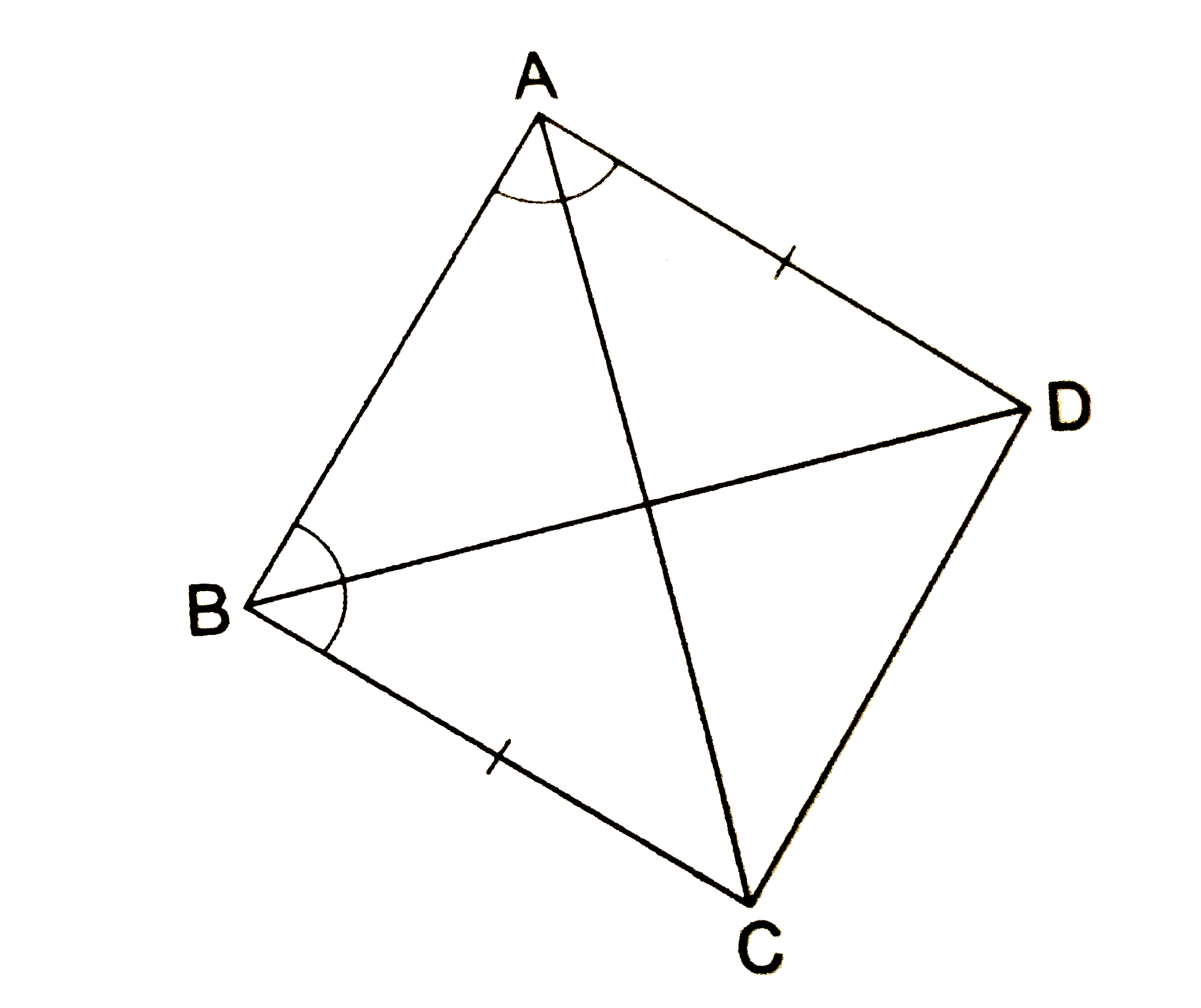
View SolutionIn the given figure, quadrilateral ABCD is a quadrilateral in which AB = AD and BC = DC. Prove that (i) AC bisects ∠A and ∠B, (ii) AC.is the perpendicular bisector of BD.
View SolutionIn quadrilateral ABCD, AB = AD and BC = CD. Show that ∠ABC=∠ADC.
View SolutionABCD is quadrilateral in which AD=BCand∠DAB=∠CBA (see figure). Prove that

BD=AC
View Solution
