Complete Solutions to Miscellaneous Examples of chapter SIMPLE HARMONIC MOTION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | SIMPLE HARMONIC MOTION |
| DC PANDEY | Miscellaneous Examples |
| Number Of Questions | 8 |
| Academic Year | 2023-24 |
For a two body oscillator system, prove the relation,
`T = 2pi sqrt((mu)/(k))`
where, `mu = (m_(1)m_(2))/(m_(1) + (m_(2))) =` reduced mass.
View SolutionTwo particles move parallel to `x-`axis about the origin with same amplitude 'a' and frequency `omega`. At a certain instant they are found at a distance `a//3` from the origin on opposite sides but their velocities are in the same direction. What is the phase difference between the two?
View SolutionFor the arrangement shown in figure, the spring is initially compressed by `3 cm`. When the spring is released the block collides with the wall and rebounds to compress the spring again.
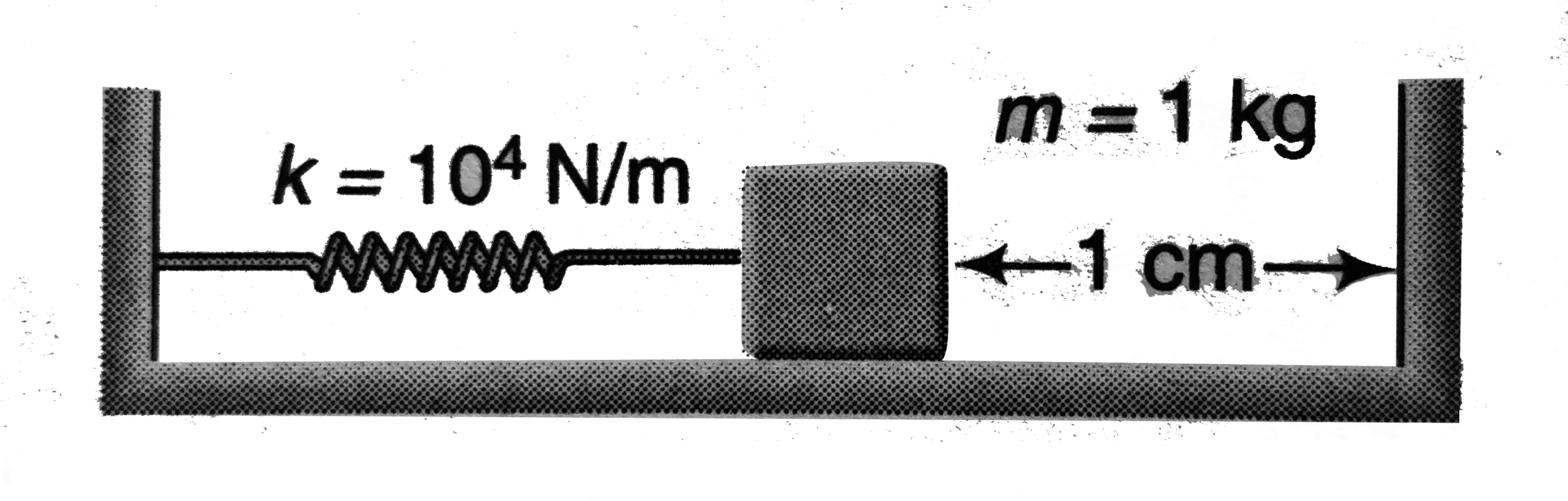
(a) If the coefficient of restitution is `(1)/sqrt(2)` , find the maximum compression in the spring after collision.
(b) If the time starts at the instant when spring is released, find the minimum time after which the block becomes stationary.
View SolutionA long uniform rod of length `L` and mass `M` is free to rotate in a horizontal plane about a vertical axis through its one end 'O'. A spring of force constant `k` is connected between one end of the rod and `PQ`. When the rod is in equilibrium it is parallel to `PQ`.
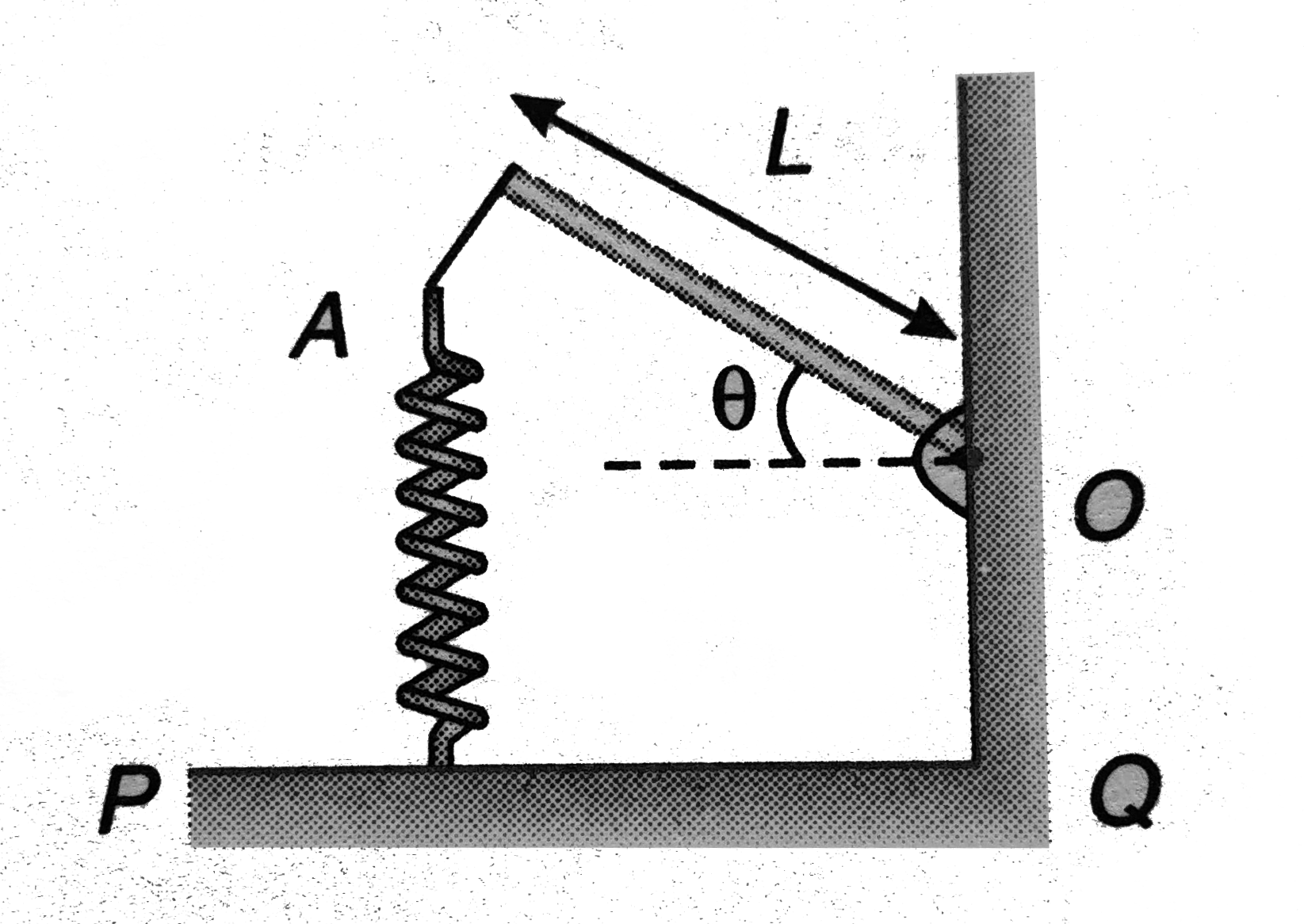
(a)What is the period of small oscillation that result when the rod is rotated slightly and released ?
(b) What will be the maximum speed of the displacement end of the rod, if the amplitude of motion is `theta_(0)`?
View SolutionA block with a mass of `2 kg` hangs without vibrating at the end of a spring of spring constant `500N//m`, which is attached to the ceiling of an elevator. The elevator is moving upwards with an acceleration `(g)/(3)`. At time `t = 0`, the acceleration suddenly ceases.
(a) What is the angular frequency of oscillation of the block after the acceleration ceases ?
(b) By what amount is the spring stretched during the time when the elevator is accelerating ?
(c )What is the amplitude of oscillation and initial phase angle observed by a rider in the elevator in the equation, `x = Asin (omega t + phi)` ? Take the upward direction to be positive. Take `g = 10.0 m//s^(2)`.
View SolutionCalculate the angular frequency of the system shown in fingure. Friction is absent everywhere and the threads, spring and pulleys are massless. Given that `m_(A) = m_(B) = m`.
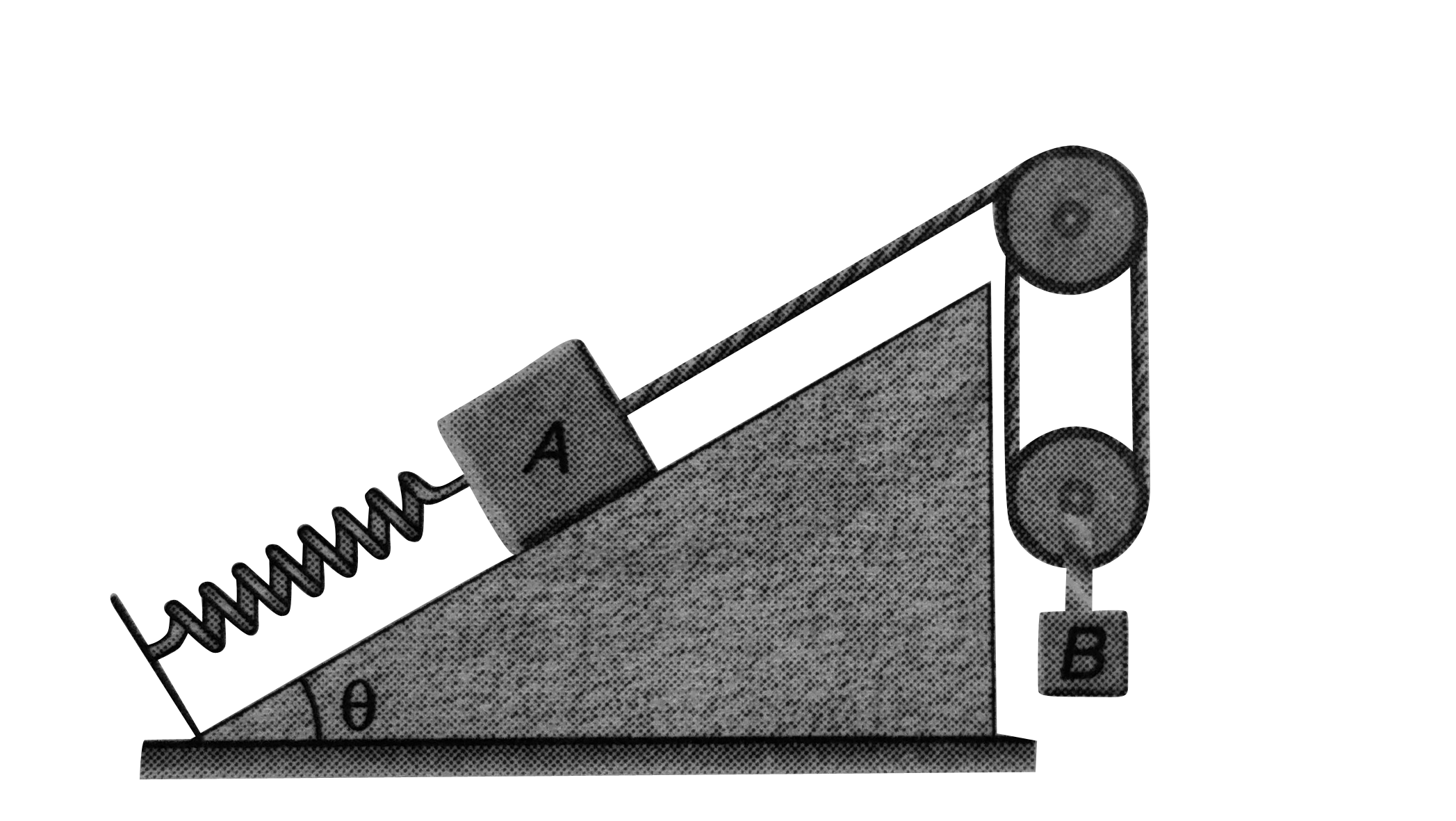
View SolutionA solid sphere `(radius = R)` rolls without slipping in a cylindrical throuh`(radius = 5R)`. Findthe time period of small oscillations.

View SolutionConsider the earth as a uniform sphere if mass `M` and radius `R`. Imagine a straight smooth tunnel made through the earth which connects any two points on its surface. Show that the motion of a particle of mass m along this tunnel under the action of gravitation would be simple harmonic. Hence, determine the time that a particle would take to go from one end to the other through the tunnel.
View Solution
Miscellaneous Examples questions and complete solutions for chapter SIMPLE HARMONIC MOTION of DC PANDEY ENGLISH of Class 11
All Exercises from DC PANDEY Class 11 PHYSICS SIMPLE HARMONIC MOTION
- Comprehension types
- Example Type 1
- Example Type 10
- Example Type 11
- Example Type 12
- Example Type 13
- Example Type 2
- Example Type 3
- Example Type 4
- Example Type 5
- Example Type 6
- Example Type 7
- Example Type 8
- Example Type 9
- Examples
- Exercise 14.1
- Exercise 14.2
- Exercise 14.3
- Exercise 14.4
- Integer type questions
- Intro. Exer.
- JEE Advanced
- Level 1 Assertion And Reason
- Level 1 Single Correct
- Level 1 Subjective
- Level 2 Comprehension
- Level 2 More Than One Correct
- Level 2 Single Correct
- Level 2 Subjective
- Matrix matching type questions
- Miscellaneous Examples
- More than one option is correct
- Only one question is correct