Complete Solutions to Exercises of chapter CENTRE OF MASS, LINEAR MOMENTUM, COLLISION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | CENTRE OF MASS, LINEAR MOMENTUM, COLLISION |
| HC VERMA | Exercises |
| Number Of Questions | 64 |
| Academic Year | 2023-24 |
Three particles of msses 1.0 kg 2.0 kg and 3.0 kg are placed at the corners A,B and C respectively of an equilateral triangle ABC of edge 1m. Locate the centre ofmass of the system.
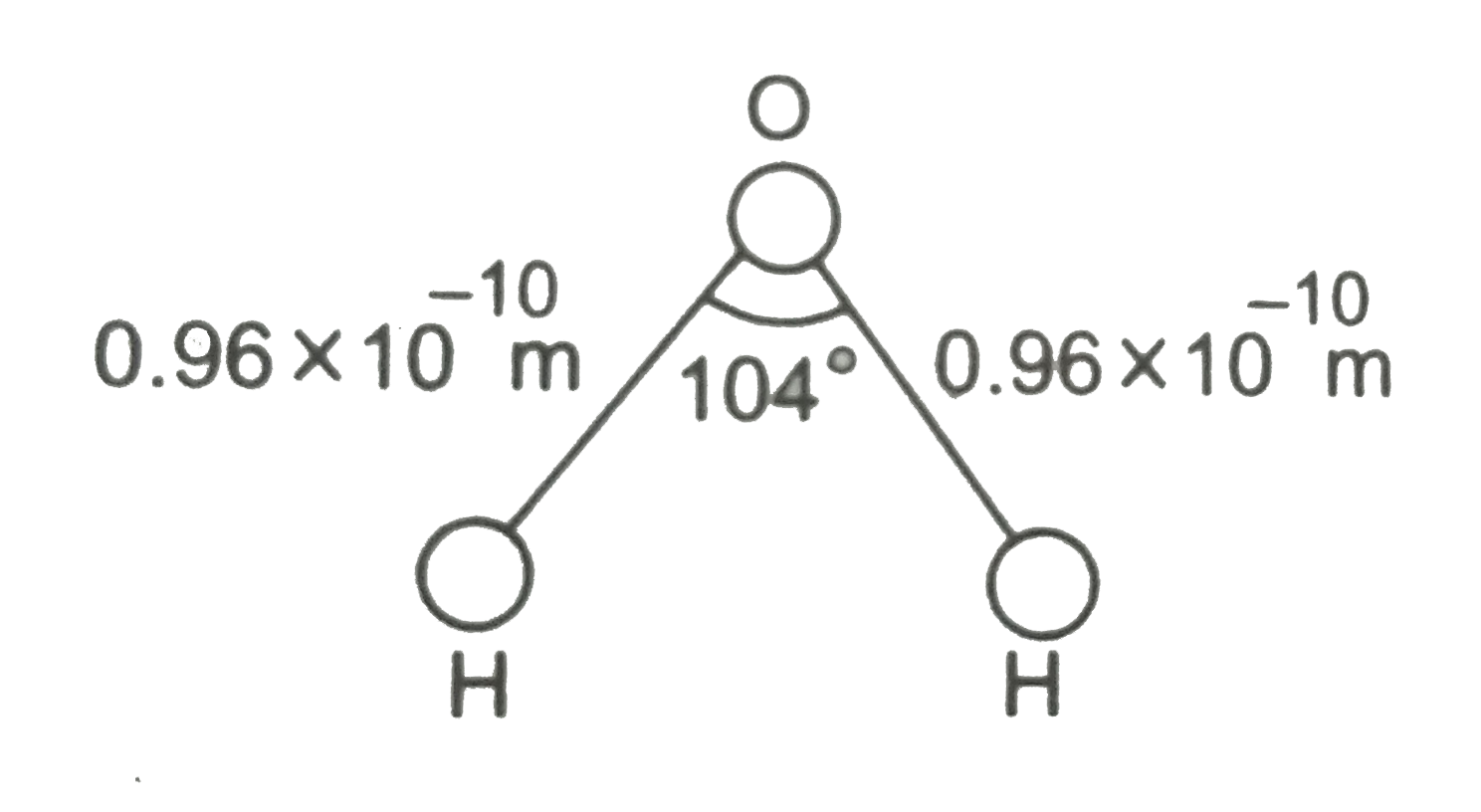
View SolutionThe structure of a water molecule is shown in figure. Find the distance of the distance of the centre of mass of the molecule from the centre of the oxygen atom.
View SolutionSeven homogeneous bricks, each of length L are arranged as shown in figure. Each brick is displaced with respect to the one in contact by `L/10`. Find the x-coordinate of the centre of mass relative to the origin shown.

View SolutionA uniform disc of radius R is put over another uniform disc of radius 2R of the same thickness and density. The peripheries of the two discs touch each other. Locate the centre of mass of the system.
View SolutionA disc of radis R is cut out from a larger disc of radius 2R in such a way that the edge of the hole touches the edge of the disc. Locate the centre of mass of the residual disc.
View SolutionA square plate of edge d and a circular disc of diameter d are placed toucing ech other at the midpoint of san edge of th plate as shown in figure. Locate the centre of mass of the combination assuming same mass per unit area for the two plates.
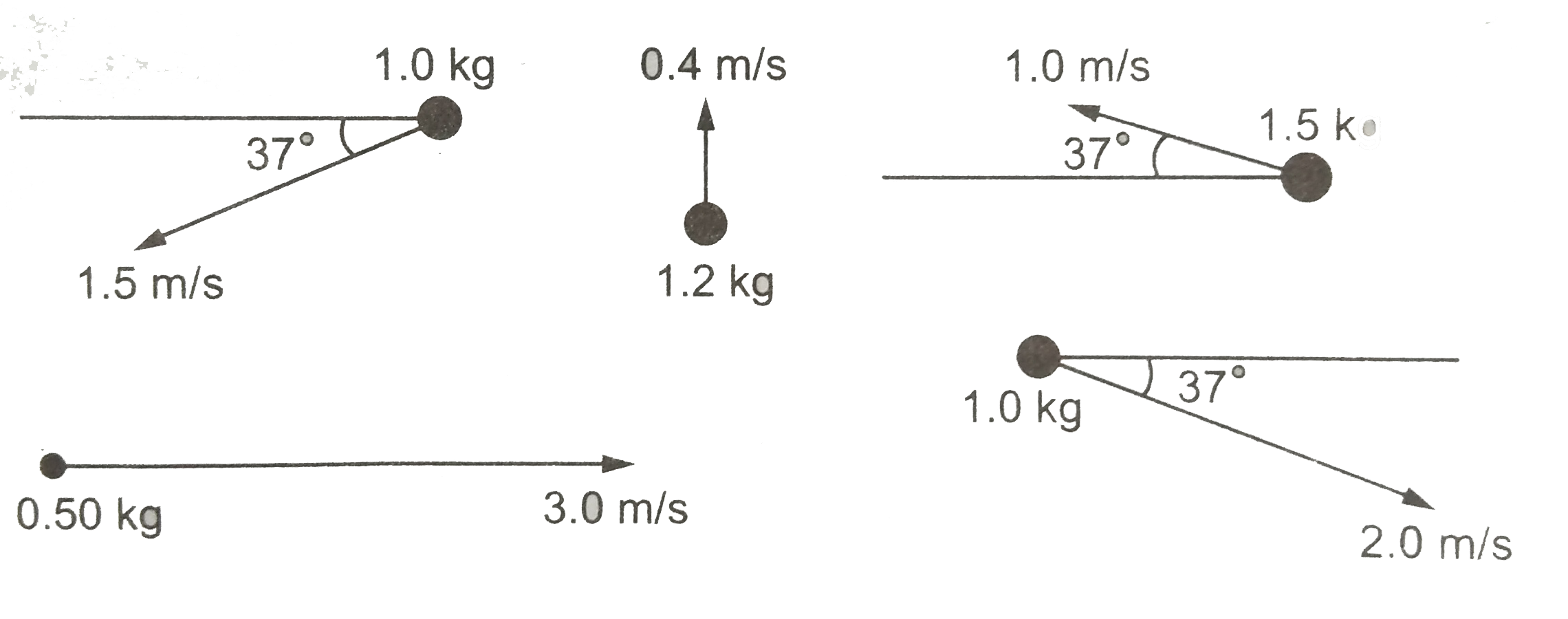
View SolutionCalculate the velocity of the centre of mss of the system of particles shown in figure.
View SolutionTwo bocks of masses 10 g and 20 kg are placed on the X-axis. The first mass is moved on the axis by a distance of 2 cm. By what distance should the second mass be moved to keep the position of the centre of mass unchanged?
View SolutionTwo blocks of masses 10 kg and 30 kg are placed along a vertical line. The first block is raised through a height of 7 cm. By what distance should the second mass be moved to raise the centre of mass by 1 cm?
View SolutionConsider a gravity free hall in which a tray of mas M, carrying a cubical block of icce of mas m and edge L, is at rest in the middle.. If the ice melts, by what distnce does the centre of mass of the tray plus the ice system descend?
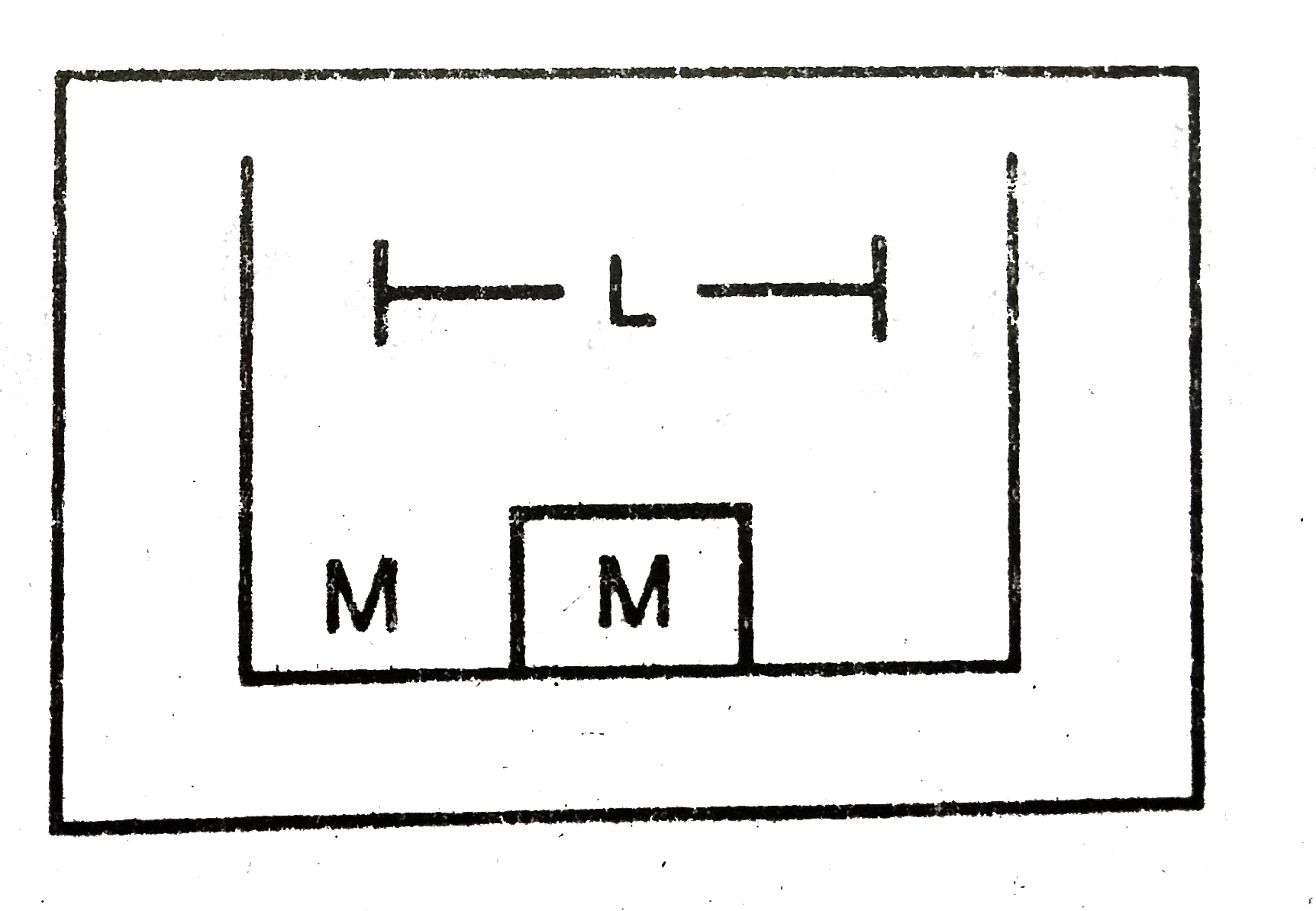
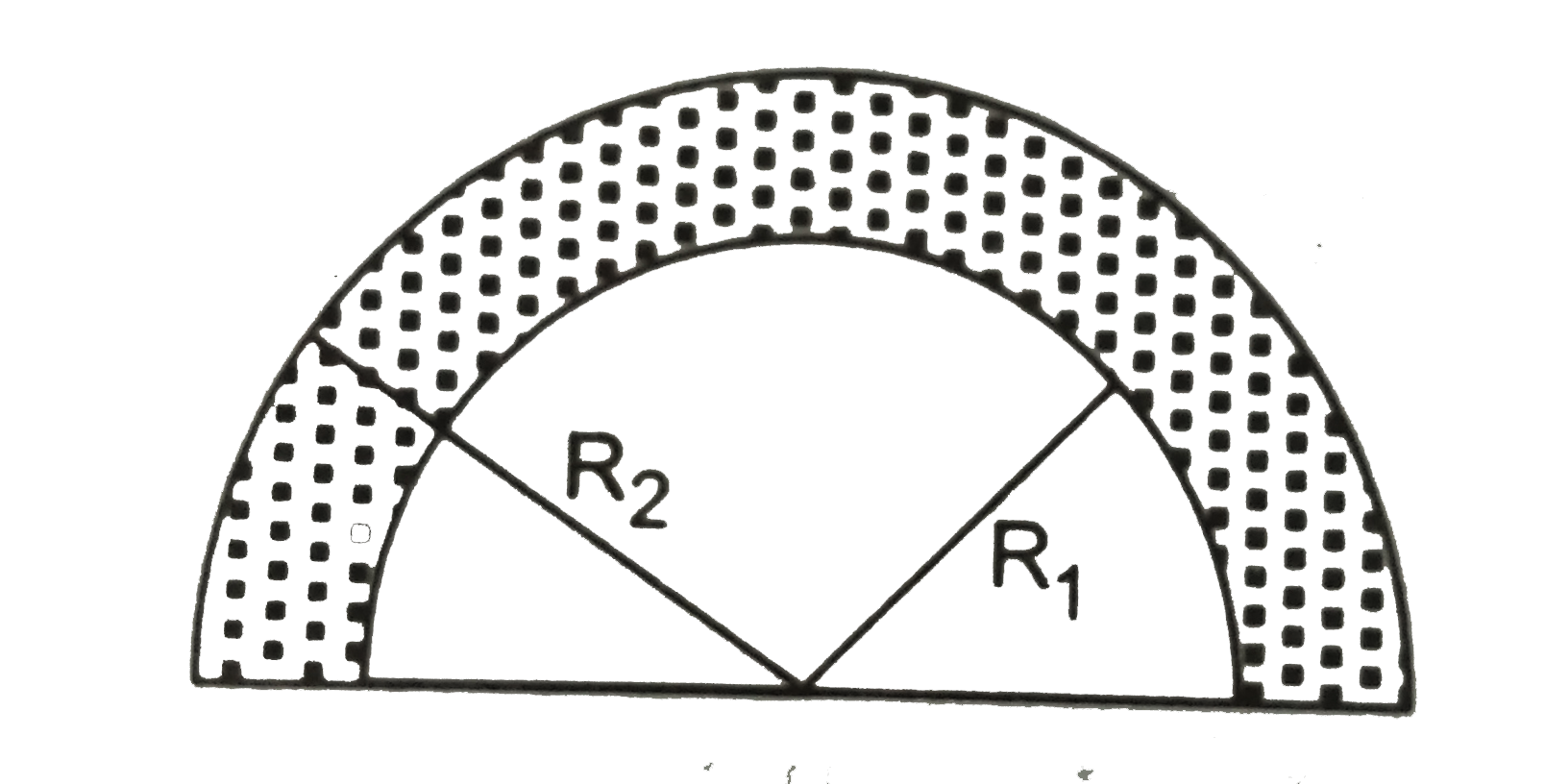
View SolutionFind the centre of mass of a uniform plate having semicircular inner and outer boundaries of radii `R_1 and R_2`.
View SolutionMr. Verma (50kg) and Mr. Mathur (60kg) are sitting at the two extremes of a 4 m long boat (40 kg) standing still in water. To discuss a mechanics problem, they come to the middle of the boat. Neglecting frictioin with water how far does the boat move on the water during the process?
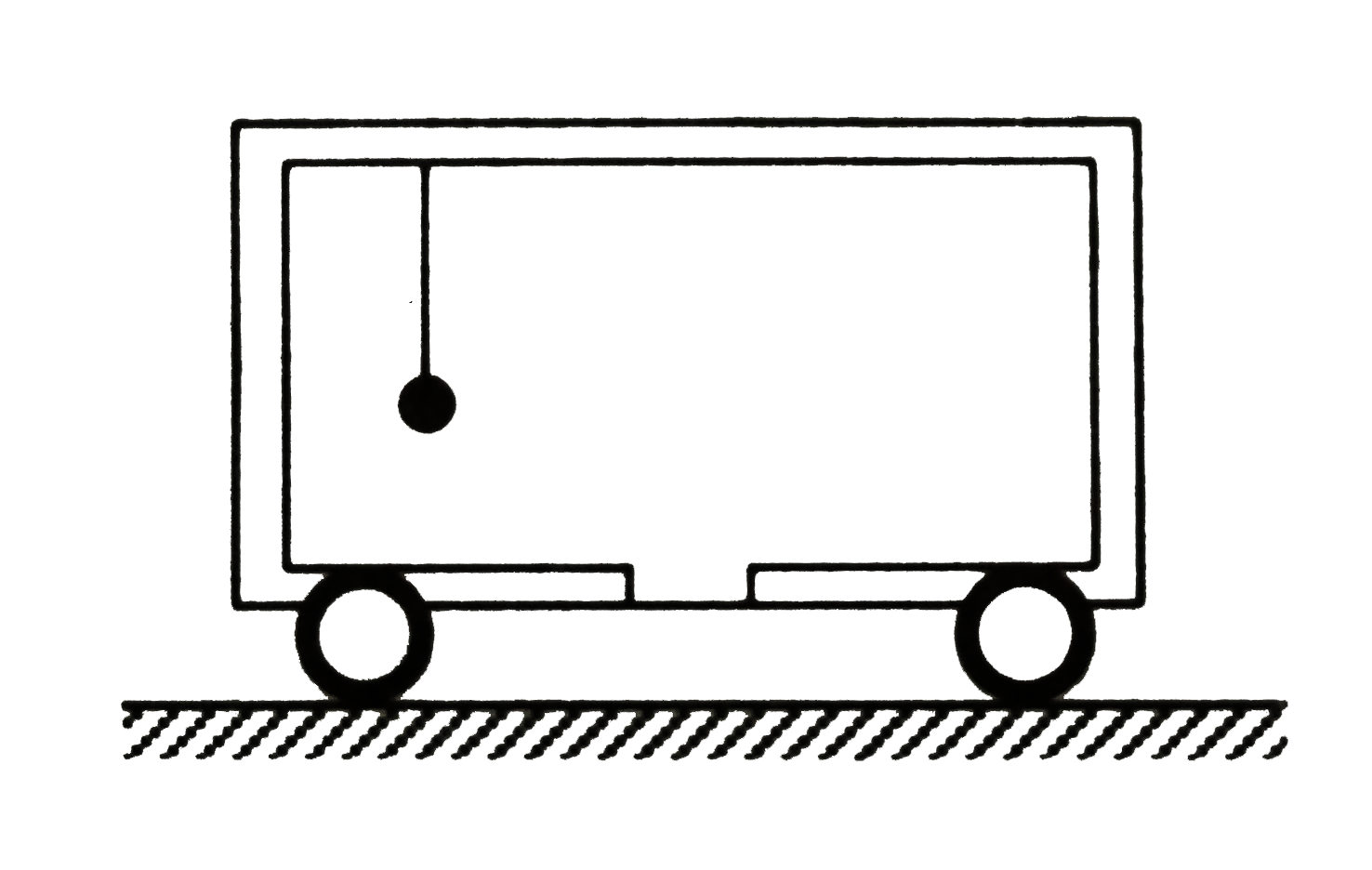
View SolutionA cart of mas M is at rest on a frictionless horizontal surface and a pendulum bob of mass m hangs from the roof of the cart figure. The string breaks, the bob falls on the floor, makes several collision on the floor and finally lands up in a small slot made in the floor. The horizontal distance between the string and the slot is L. Find the displacement of the cart during this process
View SolutionThe ballon the light rope and the monkey shown in figure are at rest in the air. If the monkey reaches the top of the rope, by what distnce does the ballon descend? Mass of the ballon =M mass of the monkey =m and the length of the rope ascended by the monkey =L
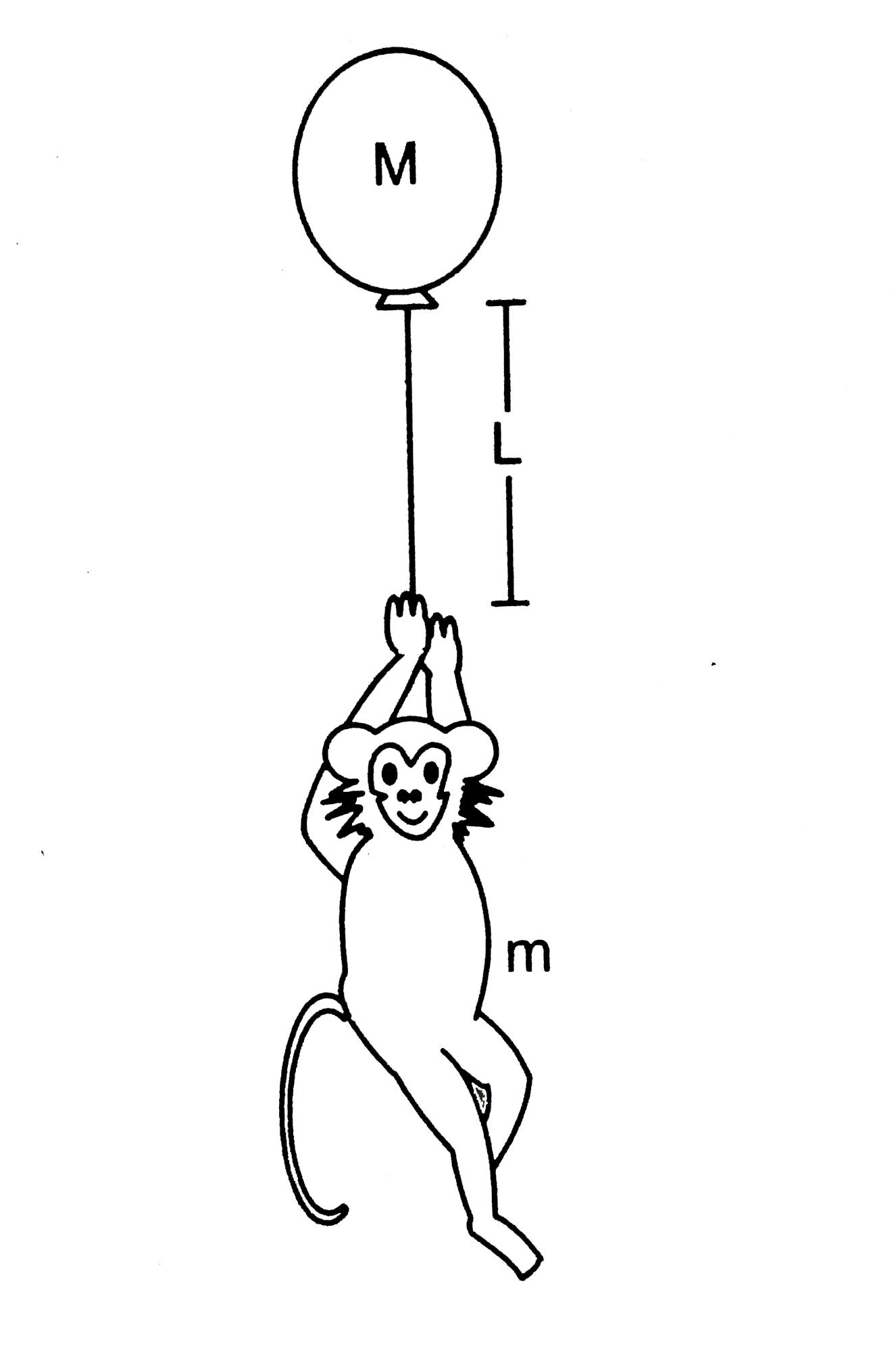
View SolutionFind the ratio of the linear omenta of two particles of masses 1.0 kg and 4.0 kg if their kinetic energies are equal.
View SolutionA uranium 238 nucleus, initially at rest emits n alpha particle with a speed of `1.4xx10^7m/s`. Calculte the rcoil speed of the residual nucleus thorium 234. Assume that the mas of a nucleus is proprotional to the mass number.
View SolutionA man of mass 50 kg starts moving on the earth and acquires speed of 1.8 m/s. with what speed does the earth recoil? Mass of earth =`6xx10^24kg.`
View SolutionA neutron initially at rest, decays into a proton, an electron and an antineutrino. The ejected electron has a momentum of `1.4xx10^-26` kg.m/s nd the antineutrino `6.4xx10^27` kgm/s. Find the recoil speed of the proton a. if the electron and the antineutrino are ejected along the same directioin and b. if they are ejected along perpendicular directions. Mass of the proton `1.67xx10^-27`kg.
View SolutionA man of mass m having a bag ofmas m slips from the roof of atall building of height H and starts falling vertically figure. When at a height h from the ground, he notices that the ground below him is pretty hard but there is a pond at a horizontal distnce x from the line of fall. In order to save himself he throws the bag horizontally (with respect to himself) in the direction opposite to the pond. Calculate the minimum horizontal velocity imparted to the bag so that the man lands in the water. If the man just suceeds toavoid the hard ground, where will the bag land?

View SolutionA ball of mass 50 g moving at a speed of 2.0 m/s strikes a placne surface at an angle of incidence `45^0`. The ball is reflected by the plane at equal angle of reflection with the same speed. Calculate a. the magnitude of the change in momentum of the ball b. the change in the magnitude of the mometum of the ball.
View SolutionLight in certain cases may be considered as a stream of paerticles called photons. Each photon has a linear momentum `h/lamda` where h is the Planck's constant and `lamda` is the wavelength `lamda` is incident on a plane mirror at an angle of incidene `theta`. Calculate the change in the linear momentum of a photon as the beam is reflected by the mirror.
View SolutionA block at rest explodes into three equal parts. Two parts start moving along X and Y axes respectively with equal speeds of 10 m/s. Find the initial velocity of the third part.
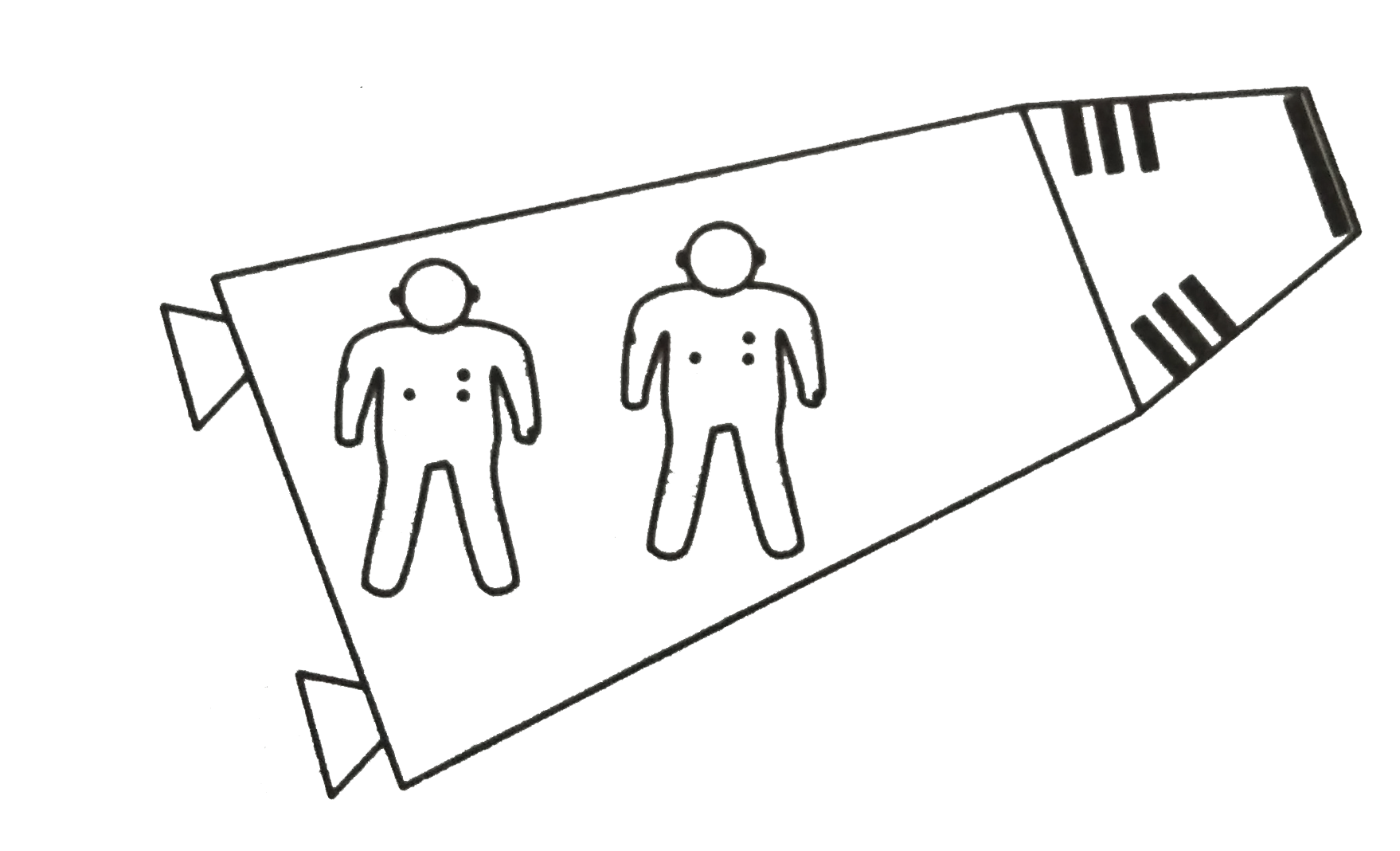
View SolutionTwo fat astronauts each of mass 120 kg are travelling in a closed spaceship moving at a sped of 15 km/s in the outer space far remvoed from tall other material objects. The total mass of the spaceship and its contents including the stronauts is 660 kg. If the astronuts do slimming exercise and thereby reduce theri mases to 90 kg, each with wht velocity will spaceship move?
View SolutionDureing a heavy rain hailstones of average size 1.0 cm in diameter fall with an average speed of 20 m/s. Suppose 2000 hailstones stgrike every square meter of a `10mxx10m` roof perpendicularly in one second and assume that the hailstones do not rebound. Calculate the average force exerted by the falling hailstones n the roof. Density of a hailstone is `900 km/s^3`
View SolutionA ball of mass m is dropped onto a floor from a certain height. The collision is perfectly elastic and the basll rebounds to the same height and again falls. Find the averge force exerted by the ball on the floor durig a long tie interval.
View SolutionA railroad car of mass M is at rest on frictionless rails when a man of mass m strts moving on the car towards the engine. If the car recoils with a speed v backward on the rails, with what velocity is the man aproaching the engine?
View SolutionA gun is monted on a railroad car. The mass of the car, the gun, the shells and the operator is 50m where m is the mass of one shell. If the velocity of het shell with respect to the gun (in its state before firing) is 200 m/s, what is the recoil speed of the car after the second shot? Neglect friction.
View SolutionTwo persns each of mass m are standing at the two extremes of a railroad car of mas M resting on a smooth track figure. The person on left jumps to the left with a horizontal speed u with respect to the state f the cr before the jump. Threafter, the other person jumps to the right, again with the same horizontl speed u with respect to teg state of the car before his jump. Find the vellcity of the car after both the person hae jumped off.

View SolutionFigure shows a small block of mass m which is started with a speed v on the horizontal part of the bigger block of mas m placed on a horizontal floor. The curved part of the surface shown is semicircular. All the surfaces are frictionless. Find the speed of the bigger block when the smaller block reaches the point A of the surface.

View SolutionIN a typical Indian Bugghi(a luxury cart drawn by horses), a wooden plate is fixed on the rear on which one person can sit. A bughi ofmas 200 kg is moving at a seed of 10 km/h. As it overtakes a school boy walking at a speed of 4 km/h,. the boy sits on the wooden plate. If the mass of the boy is 25 kg, what will be the new velocity of the bugghi?
View SolutionA ball of mass 0.50 kg moving at a speed of 5.0 m/s collides with another ball of mas 1.0 kg. After the collision the balls stick together ane remain motionless. What was the velocity of the 1.0 kg block before the collision?
View SolutionA 60 kg man skating with a speed of 10 m/s collides with a 40 kg skater ast rest and they cling to each other. Find the loss of kinetic energy duriung the collision.
View Solutionconsider a head on collision between two particles of masses `m_1 and m_2`. The initial speeds of the particles are `u_1 and u_2` in the same directioin. The collision starts at t=0 and the particles interact for a time inteval `/_\t.` Lduring the collision the speed o the first particle varies as
`v(t)=u_1+t//_\(v_1-u_1)` Find the speed of the second particle as as function of time during the collision.
View SolutionA bullet of mas m moving at a speed v hits as ball of mass M kept at rest. A small prt hving mass m breaks from the ball and sticks to the bullet. The remaining ball is found to move at a speed `v_1` in the direction of the bullet. Find the velocity of the ulet after the collision.
View SolutionA ball of mas m moving t a speed v makes a head on collisiobn with a identicalbl at rest. The kinetic energy of the blls after the collision is three fourths of the original. Find the coefficient of retitutioin.
View SolutionA block of mass 2.0 kg moving at 2.0 m/s collides head on with another block of equal mass kept at rest. a. Find the maximum possible loss in kinetic energhy due to the collision. b.If the actual loss in kinetic energy is half of this maximum, find the coefficient of restitution.
View SolutionA particle of mass 100 g moving ast an initial speed u collides with another particle of same mass kept initially at rest. If the totl kinetic energy becomes 0.2 J after the collision wht could be minimum and the maximum value of u.
View SolutionTwo friends A and B (each weighing 40 kg) are sitting on a frictionless pltform some distance d apart. A rolls a ball of mass 4 kg on the platform towrds B which B catches. Then B rolls the ball towards A and A catches it. The ball keeps on moving back and forth between A and B. The ball has a fixed speed of 5 m/s on the platform. a. Find the speed of A after he rolls the ball for the first time. b. Find the speed of A after he cathces teh ball for the first time. c. Find the speed s of A and B after the ball has made 5 round trips and is held by A. d. How many times can A roll theball? e. where is the centre of mas the system A+B+ ball at the end of the nth trip?
View SolutionA ball falls on the ground fro a height of 2.0 m and rebounds up to a height of 1.5 m. Find the coefficient of restitution.
View SolutionIn a gamma decay fprcess, the internal energy of a nucleus of mass M deceses, a gamma photon of energy E and linear momentum E/c is emitted and the nucleus recoils. Find the decrease in internal energy.
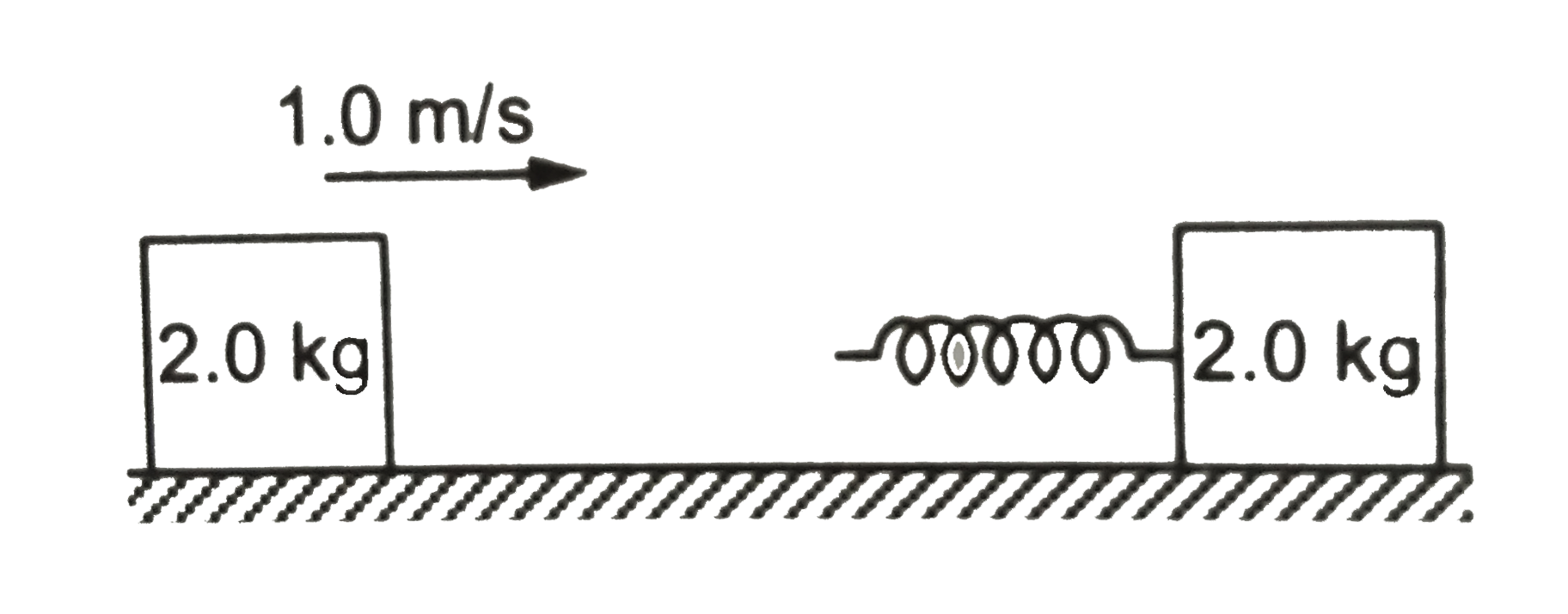
View SolutionA block of mass 2.0 kg is moving on a frictionless horizontal surface with a velocity of 1.0 m/s figure towards abnother block of equal mass kept at rest. The spring constant of the spring fixed at one end is 100 The spring constant of the spring fixed at one end is 10 N/m. Find the maximum compression of the spring.
View SolutionA bullet of mass 20 g travelling horizontally with a speed of 500 m/s passes through a wooden block of mass 10.0 kg initially at rest on a level surface. The bulet emerges with speed of 100 m/s and the block slides 20 cm on the surface before coming to rest. Find the friction cioefficient between the block and the surface figure.

View SolutionA projectile is fired with a pseed u atan angle `theta` above a hoerizontal fiedl. The coeffiient of restitution of collisiion between the projectile and the field is e. How far from the starting point does the projectile makes its second collision with the field?
View SolutionA ball falls on an inclined plane of inclinatioin `theta` from a height h above the pontof impact and makes a perfectly elastic collision. Where will it hit the plane again?
View SolutionSolve tehh previous problem if the coefficient of restitution is e. Use `theta=45^0, e=3/4 nd h=5m`
View SolutionA block of mass `180 g` is suspended by a massless spring. The spring extends by `1.8 cm` due to the weight of block. A particle of mass `20 g` is dropped from a height `80 cm` on the block. The collision is completely inelastic . Find the maximum elongation of the spring.
View SolutionA bullet of mass 25 g is fired horizontally into a ballistic pendulum of mas 5.0 kg and gets embedded in it figure. If the cwentre of the pendulum rises by a distance of 10 cm, find the speed of the bullet.
View SolutionA bullet of mass 20 g moving horizontally at speed of 300 m/s is fired into wooden block of mas 500 suspended by a long string. The bullet crosses the block and emerges on the other side. If the centre of mass of the block rises through a height of 20.0 cm, find the speed of the bullet as it emerges from the block.
View SolutionTwo masses `m_1 and m_2` re connected by a spring of spring constnt k and are placed on as frictionless horizontal surface. Initially the spring is tretched through a distance `x_0` when the system is released from rest. Find the distance moved by the tow masses before they again come to rest.
View SolutionTwo blocks of masses `m_1 and m_2` are connected by a spring of spring constant k figure. The block of mass `m_2` is given a shapr imulse so that it acquires a velocity `v_0` twoards right. Find a. the velocity of the cenre of mas b. the maximum elongation that teh spring will suffer.
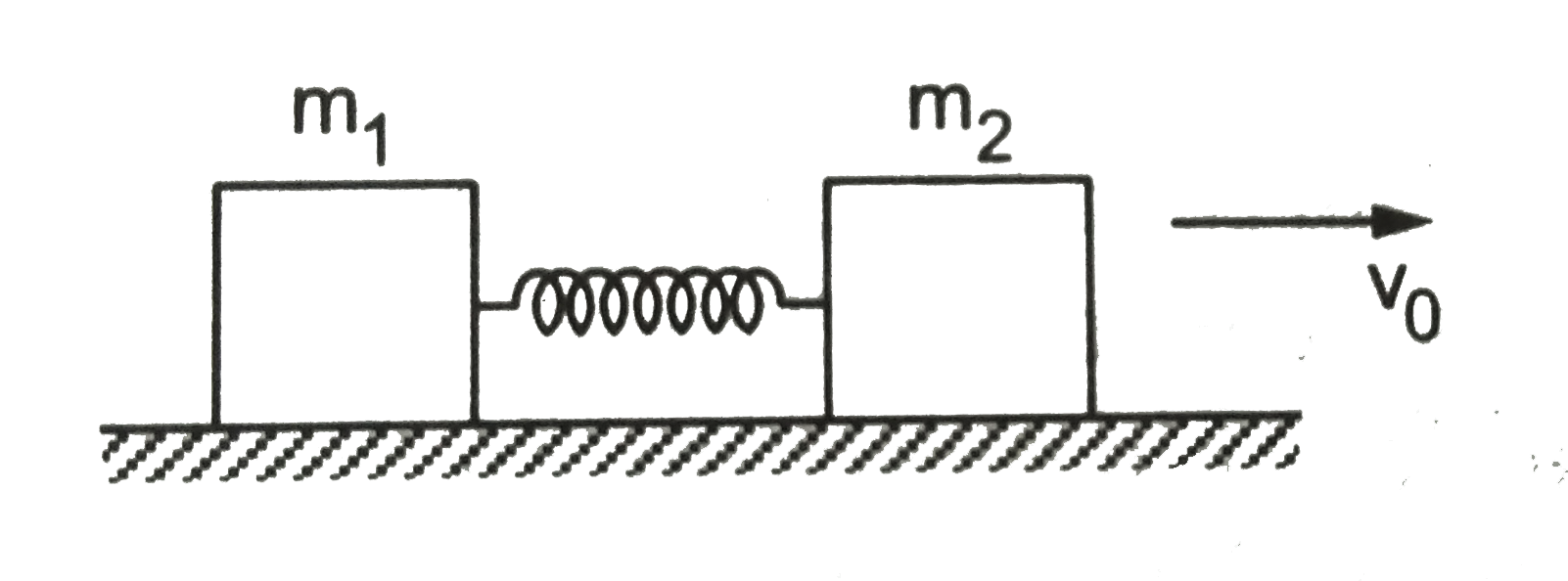
View SolutionCionsider the situation of the previous problem. Suppose each of tbe blocks is pulled by a constant foerce F instead of any impulse. Find the maximum elongation that the spring will suffer and the distancews moved by the two blocks in the process.
View Solutionconsider the situastion of the previous problem. Suppose the block of mass `m_1` is pulled by a constant force `F_1` and the other block is pulled by a constant force `F_2`. Find the maximum elongation that the spring will suffer.
View SolutionConsider a gravity free hall in which an experimetner of mass 50 kg is resting on a 5 kg pilow, 8 ft above the floor of the hall. He pushes the pilow down so that it starts falling at a pseed of 8 fft/s. The pillow makes a perfectly elastic collision with teh floor, rebounds and reaches the experimeter's head. Findthe time elapsed in the process.
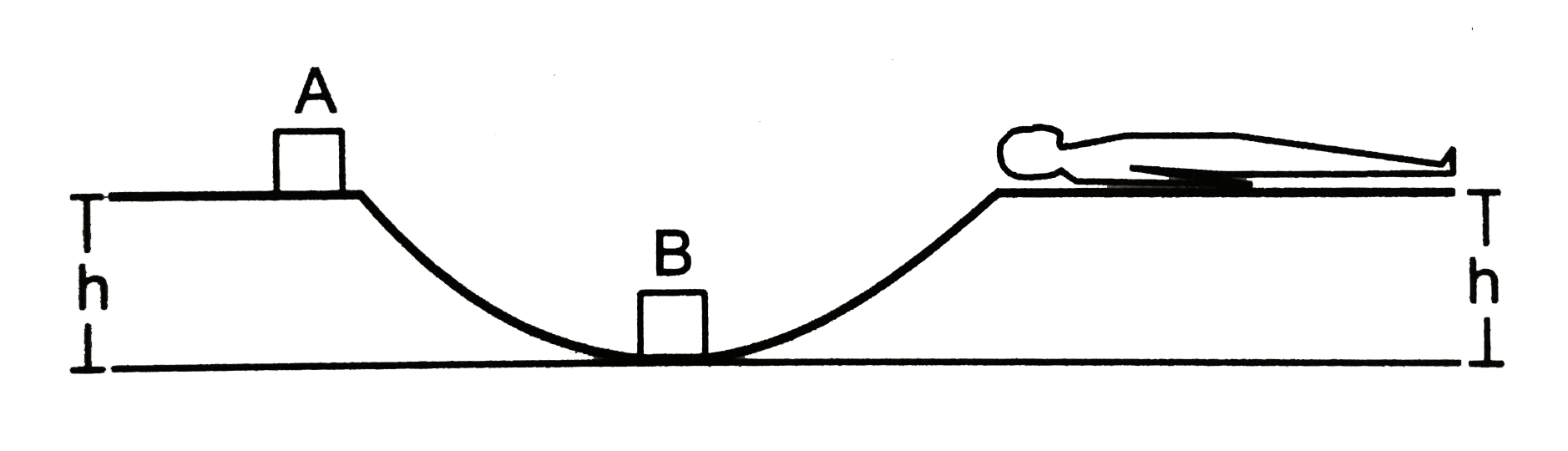
View SolutionThe track shown in figure is frictionles. The block B of mass 2m is lying at rest and the block A of mass m is pushed along the track with some speed. The collision etwen A and B is perfectly elastic. With what velocity should the block A be started to get teh sleepign mn awakenend?
View SolutionA bullet of mass 10 g moving horizontally at asped dof `50sqrt7m/s` strikes a block of mass 490 g kept on a frictionless track as shown in figure. The bullet remains inside the block and the system proceeds towards the semicircular tract o radius 0.2 m. Where will the block strike the horizontal part after leaving the semicircular track?

View SolutionTwo balls having masses m and 2m are fastened to two light strings of same length l figure. The other ends of the strings are fixed at O. The strings are kept in the same horizontal line and the system is released from rest. The collision between the balls is elastic. a. Find velocities of the balls just after their collision. b. How high will the balls rise after the collision.

View SolutionA uniform chain of mass M and length L is held verticallyi in such a way that its lower end just touches the horizontal floor. The chain is released from rest in this position. Any portion that strikes the floor comes to rest. Assuming that the chain does not form a heap on the floor, calculate the force exerted by it on the floor when a length x has reached the floor.
View SolutionThe blocks shown in figure have equal masses. The surface of A is smooth but that of B has a friction coefficient of 0.10 with the floor. Block A is moving at a speed of 10 m/s towards B which is kept at rest. Find the distance travelled by B if a. the collision is perfectly elastic and b. the collision is perfectly inelastic. Take `g=10 m/s^2`.

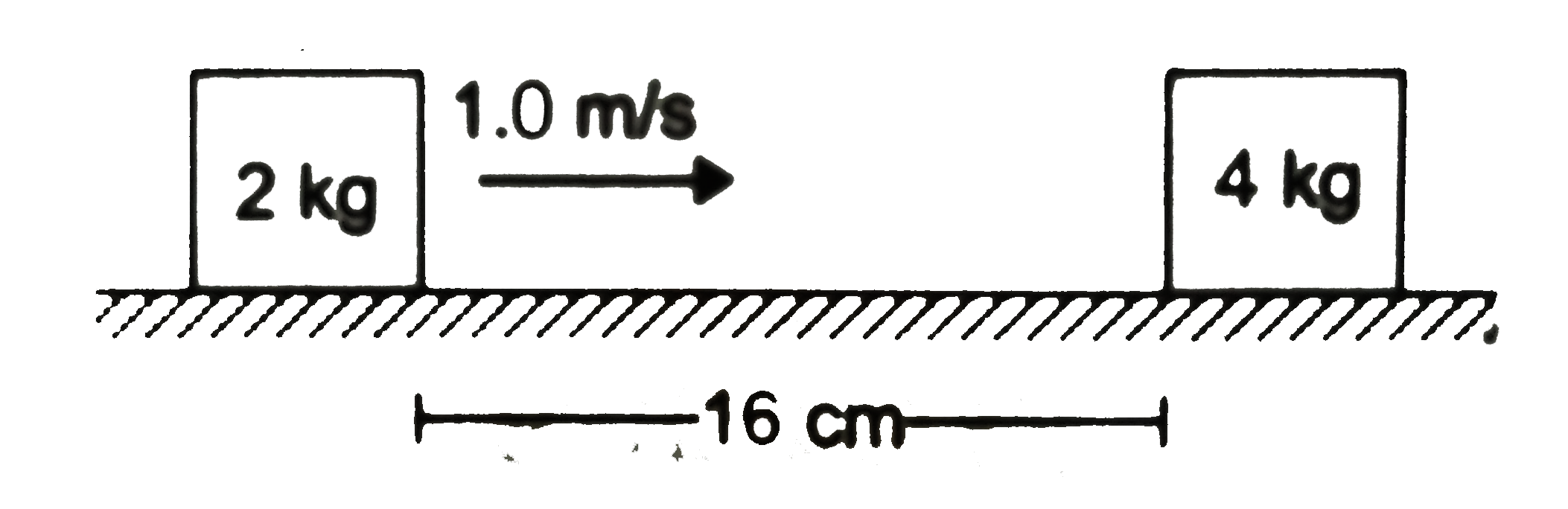
View SolutionThe friction coefficient between the horizontal surface and each of the blocks shown in figure is 0.20. The collision between the blocks is perfectly elastic. Find the separation between the two blocks when they come to rest. Take `g=10 m/s^2`.
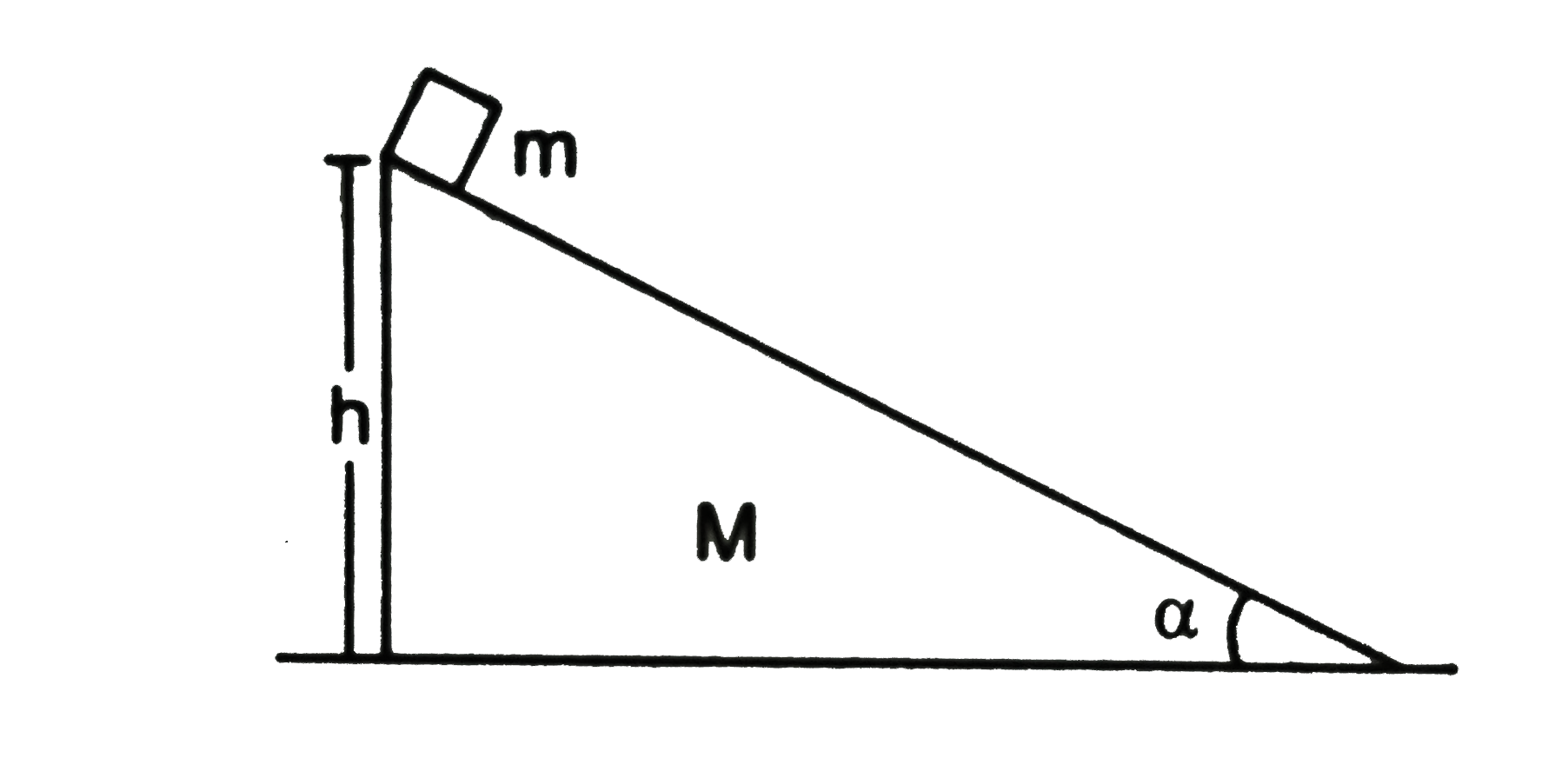
View SolutionA block of mas m is placed on a triangular block of mas m, which in tunis placed on a horizontal surface as shown in figure. Assuming frictionless surfaces find the velocity of the triangular block when the smaller block reaches the bottom end.
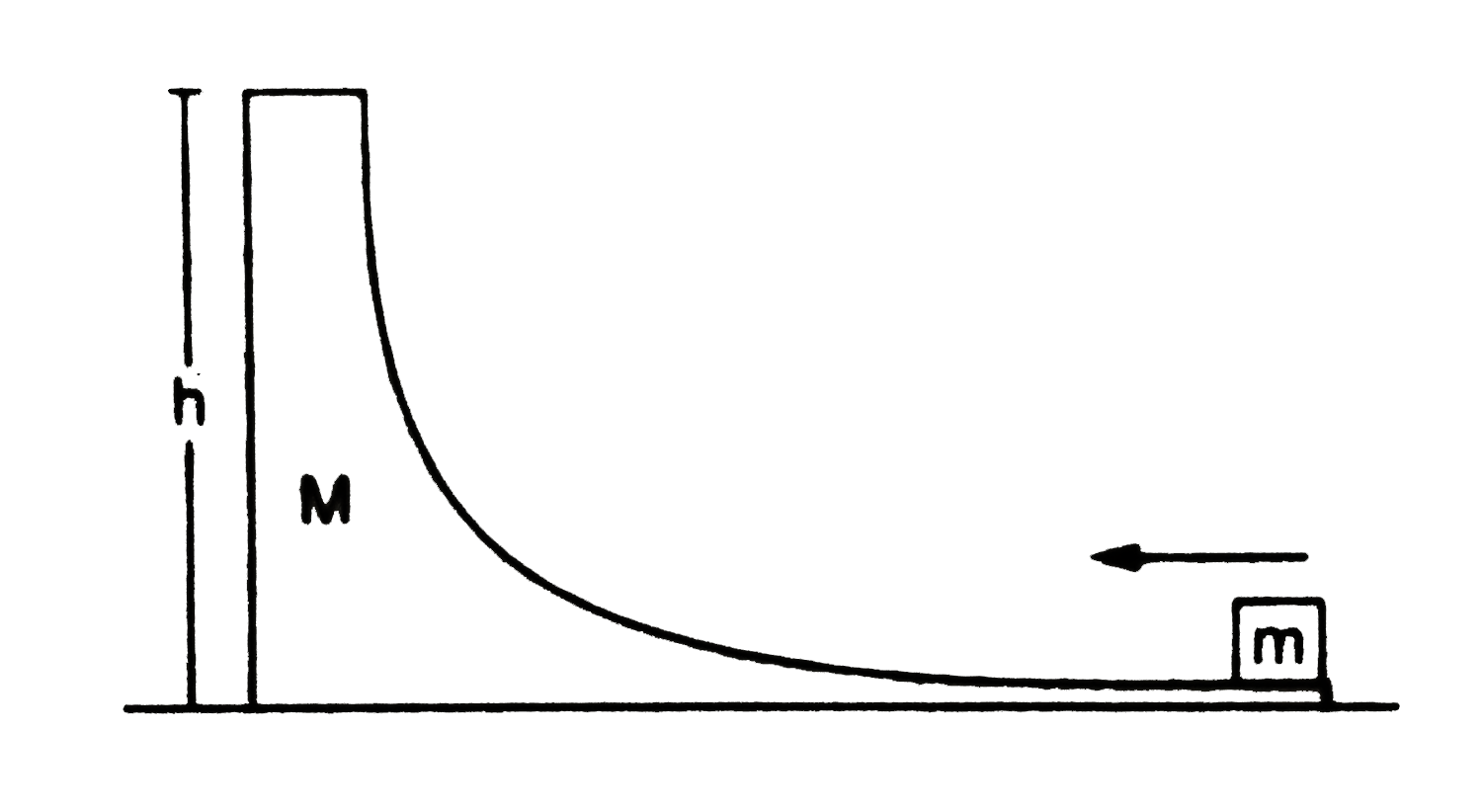
View SolutionFigure shows a small body of mass m placed over a larger mass M whose surface is horizontal near the smaller mass and gradually curves to become verticle. The smaller mass is pushed on the longer on at a speed v and the system is left to itself. Assume thast all the surfaces are frictionless. a. find teh speed of the larger block when the smaller block is sliding on the vertical part. b. find the speed of the smaller mass when it breaks off the larger mas at height h. c. find the maximum height (from the ground) that the smaller mass seconds. d show that the smaller mass will again land on the bigger one. Find the distane traversed by the bigger block during the time when the smaller block was in its flight under gravity.
View SolutionA small block of superdennse material has a mass of `3xx10^24` kg. It is situated at a height h (much smaller than the earth's radius) from where it falls on the earth's surface. Find its speed when its height from the earth's surface has reduced to `h/2`. The mass of the earth is `6xx10^24kg`
View SolutionA body of mass m makes an elastic collision with another identical body at rest. Show that if the collisionis not head on the bodiese to at right to each other after the collision.
View SolutionA small particle travelling with a velocity v collides elastically with a spbereical body of equal masss and of radius r initially kept at rest. The centre of this spherical body is located a distnce `rho(ltr)` away from the direction of motion of the particle figure. Find the final velocities of the two particles.

View Solution
Exercises questions and complete solutions for chapter CENTRE OF MASS, LINEAR MOMENTUM, COLLISION of HC VERMA PHYSICS (HINGLISH) of Class 11