Complete Solutions to Exercises of chapter WAVE MOTION AND WAVES ON A STRING of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | WAVE MOTION AND WAVES ON A STRING |
| HC VERMA | Exercises |
| Number Of Questions | 57 |
| Academic Year | 2023-24 |
A wave pulse passing on a string with speed of `40cms^-1` in the negative x direction has its maximum at `x=0 at t=0`. Where will this maximum be located at `t=5s?
View SolutionThe equation of a wve travelling on a string stretched along the X-axis is given by
`y=Ad^(-(x/at/T)^2)`
a. Write the dimensions of A, a and T. b. Find the wave speed. c.In which direction is the wave travelling? d.Where is the maximum of the pulse located at `t=T? At t=2T?`
View SolutionFigure shows a wave pulse at t=0. The pulse moves to the right with a speed of 10cms^-1. Sketch the shape of the string at t=s, 2s and 3s.

View SolutionA pulse travelling on a string is represented by the function
y=a^3/((x-vt)^2+a^2)`
where a=5 mm and v=20 cms^-1. Sketch the shape of the string at t=0, 1 s and 2s. Take x=0 in the middle of the string.
View SolutionThe displacement of the particle at x = 0 of a stretched string carrying a wave in the positive x-direction is given by `f(t)sin(t/T)`. The wave speed is v. Write the wave equation.
View SolutionA wave pulse is travelling on a string with a speed v towards the positive X-axis. The shape of the string at t = 0 is given by `g(x) = A sin(x /a)`, where A and a are constants. (a) What are the dimensions of A and a ? (b) Write the equation of the wave for a general time 1, if the wave speed is v.
View SolutionA wave propagates on a string in the positive x-direction at a velocity u. The shape of the string at `t=t_0` is given by `g(x, t_0)= A sin(x /a)` . Write the wave equation for a general time t.
View SolutionThe equation of a wave travelling on a string is `y=(0.10mm)sin[3.14m^-1)x+(314s^-1)t]`. (a) In which direction does the wave travel ? (b) Find the wave speed, the wavelength and the frequency of the wave. (c) What is the maximum displacement and the maximum speed of a portion of the string ?
View SolutionA wave travels along the positive x-direction with a speed of `20 ms^-1`. The amplitude of the wave is 0.20 cm and the wavelength 2.0 cm. (a) Write a suitable wave equation which describes this wave. (b) What is the displacement and velocity of the particle at x = 2.0 cm at time t = 0 according to the wave equation written ? Can you get different values of this quantity if the wave equation is written in a different fashion ?
View SolutionA wave is decribed by the equation `y = (1.0mm) sin pi ((x)/(2.0cm) - (t)/(0.1s)). (a) Find the time period and the wavelength ? (b) Write the equation for the velocity of the particles. Find the speed of the particle at x = 1.0cm at time t = 0.01s, (c ) What are the speeds of the particles at x =3.0 cm 5.0 cm aned 7.0cm at t = 0.01 ? (d) What are the speeds of the particles at x = 1.0 cm at t= 0.011, 0.012, and 0.013s ?
View SolutionA particle on a stretched string supporting a travelling wave, takes 5.0 ms to move from its mean position to the extreme position. The distance between two consecutive particles, which are at their mean positions, is 2.0 cm. Find the frequency, the wavelength and the wave speed.
View SolutionFigure shows a plot of the transverse displacements of the particles of a string at t = 0 through which a travelling wave is passing in the positive x-direction. The wave speed is `20 cms^-1`. Find (a) the amplitude, (b) the wavelength, (c) the wave number and (d) the frequency of the wave.
 .
.
View SolutionA wave travelling on a string at a speed of `10 ms^-1` causes each particle of the string to oscillate with a time period of 20 ms. (a) What is the wavelength of the wave ? (b) If the displacement of a particle is 1.5 mm at a certain instant, what will be the displacement of a particle 10 cm away from it at the same instant ?
View SolutionA steel wire of length 64 cm weighs 5 g. If it is stretched by a force of 8 N, what would be the speed of a transverse wave passing on it ?
View SolutionA string of length 20 cm and linear mass density `0.40 g cm^-1` is fixed at both ends and is kept under a tension of 16 N. A wave pulse is produced at t = 0 near an end as shown in figure, which travels towards the other end. (a) When will the string have the shape shown in the figure again ? (b) Sketch the shape of the string at a time half of that found in part (a).

View SolutionA string of linear mass density `0.5 g cm^-1` and a total length 30 cm is tied to a fixed wall at one end and to a frictionless ring at the other end. The ring can move on a vertical rod. A wave pulse is produced on the string which moves towards the ring at a speed of `20 cm s^-1`. The pulse is symmetric about its maximum which is located at a distance of 20 cm from the end joined to the ring. (a) Assuming that the wave is reflected from the ends without loss of energy, find the time taken by the string to regain its shape. (b) The shape of the string changes periodically with time. Find this time period. (c) What is the tension in the string ?

View SolutionTwo wires of different densities but same area of cross section are soldered together at one end and are stretched to a tension T. The velocity of a transverse wave in the first wire is double of that in the second wire. Find the ration of the denstiy of the first wire to that of the second wire.
View SolutionA transverse wave described by
`y=(0.02m)sin[(1.0m^-1)x+(30s^-1)t]`
propagates on a stretched string having a linear mass density of `1.2xx10^-4 kgm^-1`. Find the tension in the string.
View SolutionA travelling wave is produced on a long horizontal string by vibrating an end up and down sinusoidal. The amplitude of vibration is 1.0cm and the displacement becomes zero 200 times per second. The linear mass density of the string is `0.10 kg m^(-1)` and it is kept under a tension of 90 N. (a) Find the speed and the wavelength of the wave. (b) Assume that the wave moves in the positive x-direction and at t = 0 the end x= 0 is at its positive extreme position. Write the wave equation. (c ) Find the velocity and acceleration of the particle at x = 50 cm at time t = 10ms.
View SolutionA string of length 40 cm and weighing 10 g is attached to a spring at one end and to a fixed wall at the other end. The spring has a spring constant of `160 N m^-1` and is stretched by 1-0 cm. If a wave pulse is produced on the string near the wall, how much time will it take to reach the spring ?
View SolutionTwo blocks each having a mass of 3.2 kg are connected by a wire CD and the system is suspended from the ceiling by another wire AB . The linear mass density of the wire AB is 10 g m4 and that of CD is `8 g m^-1.` Find the speed of a transverse wave pulse produced in AB and in CD.
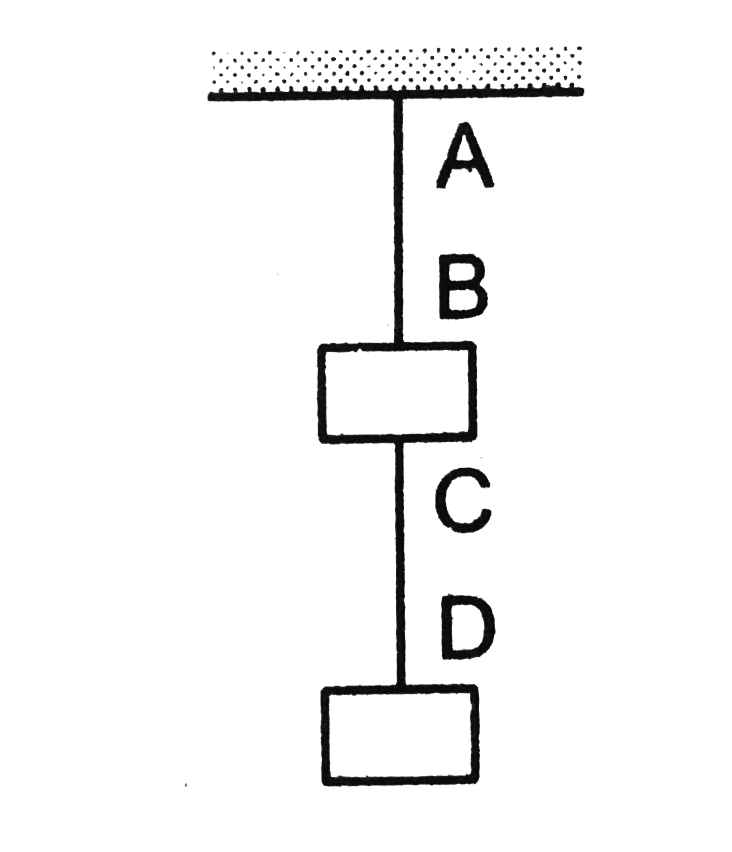
View SolutionIn the arrangement shown in figure, the string has a mass of 4.5 g. How much time will it take for a transverse disturbance produced at the floor to reach the pulley ? Take `g=10ms^-2`
 .
.
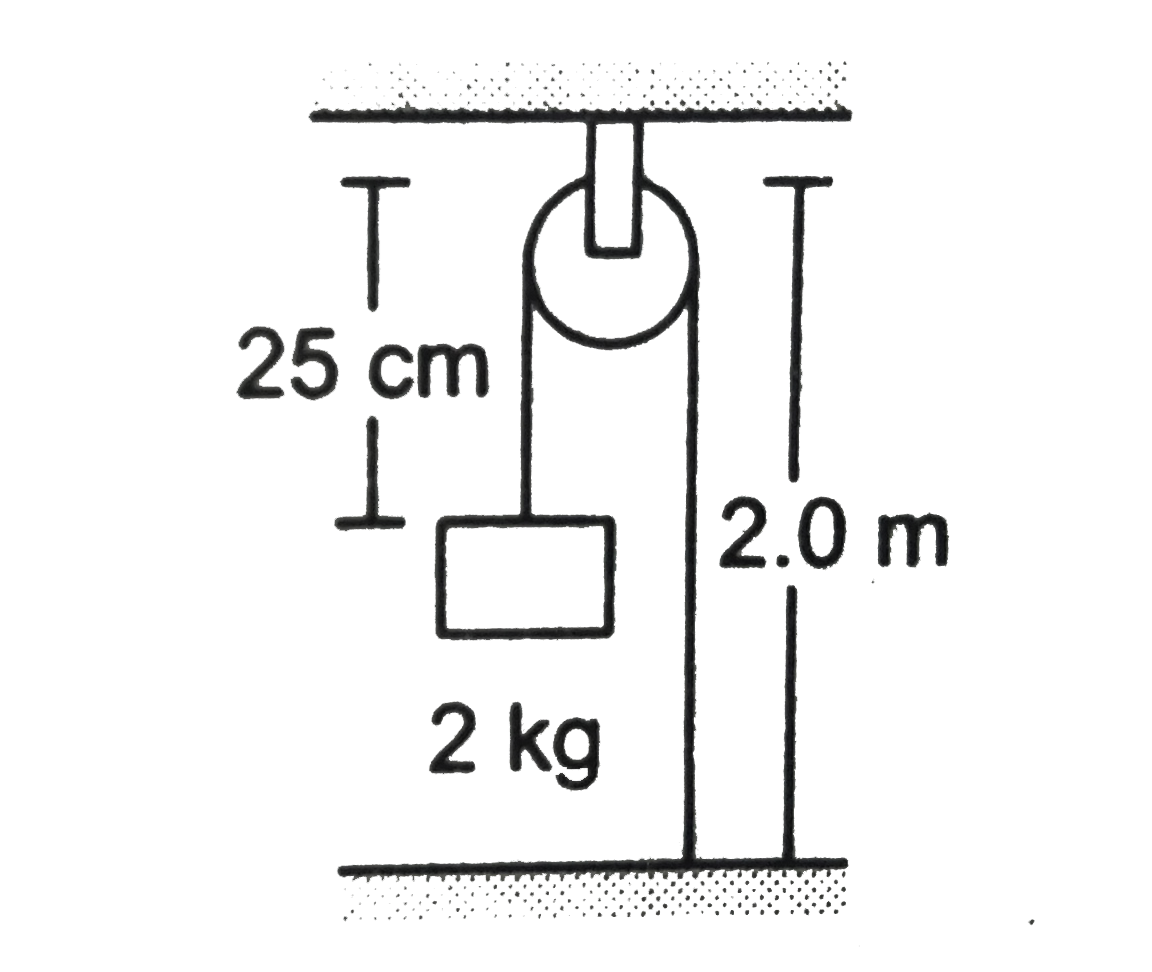
View SolutionA 4.0 kg block is suspended from the ceiling of an elevator through a string having a linear mass desity of `19.2xx10^-3 kgm^-1`. Find the speed (with respect to the string) with which a wave pulse can proceed on the string if the elavator accelerates up at the rate of `2.0ms^-2. Take g=10 ms^-2`.
View SolutionA heavy ball is suspended from the ceiling of a motor car through a light string. A transverse pulse travels at a speed of `60 cm s^-1` on the string when the car is at rest and `62 cm s^-1` when the car accelerates on a horizontal road. Find the acceleration of the car. Take `g = 10 ms^-2`
View SolutionA circular loop of string rotates about its axis on a frictionless horizontal plane at a uniform rate so that the tangential speed of any particle of the string is u. If a small transverse disturbance is produced at a point of the loop, with what speed (relative to the string) will this disturbance travel on the string ?
View SolutionA heavy but uniform rope of lenth L is suspended from a ceiling. (a) Write the velocity of a transverse wave travelling on the string as a function of the distance from the lower end. (b) If the rope is given a sudden sideways jerk at the bottom, how long will it take for the pulse to reach teh celling ? (c ) A particle is dropped from the ceiling at the instant the bottom end is given the jerk where will the particle meet the pulse ?
View SolutionTwo long strings A and B, each having linear mass density `1.2 xx 10 ^-2 kg m^-1`, are stretched by different tensions 4.8 N and 7.5 N respectively and are kept parallel to each other with their left ends at x = 0. Wave pulses are produced on the strings at the left ends at t = 0 on string A and at t = 20 ms on string B. When and where will the pulse on B overtake that on A ?
View SolutionA transverse wave of amplitude 0.50 mm and frequency 100 Hz is produced on a wire stretched to a tension of 100 N. If the wave speed is `100 m s^-1, what average power is the source transmitting to the wire ?
View SolutionA `200 Hz` wave with amplitude `1 mm` travels on a long string of linear mass density `6 g//m` keep under a tension of `60 N`.
(a) Find the average power transmitted across a given point on the string.
(b) Find the total energy associated with the wave in a `2.0 m` long portion of the string.
View SolutionA tuning fork of frequency 440 Hz is attached to a long string of linear mass density `0.01 kg m^-1` kept under a tension of 49 N. The fork produces transverse waves of amplitude 0.50 mm on the string. (a) Find the wave speed and the wavelength of the waves. (b) Find the maximum speed and acceleration of a particle of the string. (c) At what average rate is the tuning fork transmitting energy to the string ?
View SolutionTwo waves, travelling in the same direction through the same region, have equal frequencies, wavelengths and amplitudes. If the amplitude of each wave is 4 mm and the phase difference between the waves is `90^@`, what is the resultant amplitude ?
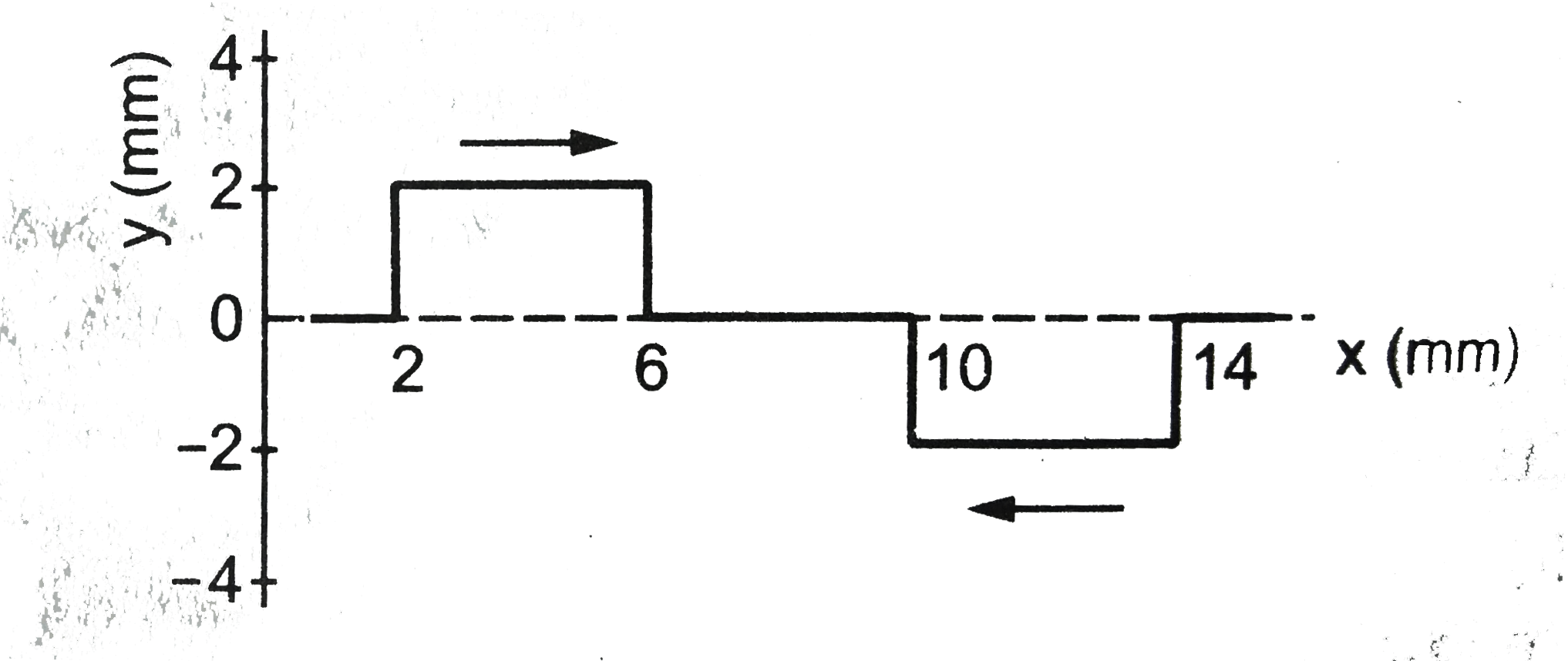
View SolutionFigure shows two wave opulses at t=0 travelling on a string i opposite directions with the same wave speed `50 cms^-1`. Sketch the shape of the string at t=4ms, 6ms, 8ms and 12 ms.
 .
.
View SolutionTwo waves, each having a frequency of 100 Hz and a wavelength of 2.0 cm, are travelling in the same direction on a string. What is the phase difference between the waves (a) if the second wave was produced 0.015 s later than the first one at the same place, (b) if the two waves were produced at the same instant but the first one was produced a distance 4.0 cm behind the second one ? (c) If each of the waves has an amplitude of 2.0 mm, what would be the amplitudes of the resultant waves in part (a) and (b) ?
View SolutionIf the speed of a transverse wave on a stretched string of length 1 m is `60 m s^-1`, what is the fundamental frequency of vibration ?
View SolutionA wire of length 2.00 m is stretched to a tension of 160 N. If the fundamental frequency of vibration is 100 Hz, find its linear mass density.
View SolutionA steel wire of mass 4.0 g and length 80 cm is fixed at the two ends. The tension in the wire is 50 N. Find the frequency and wavelength of the fourth harmonic of the fundamental.
View SolutionA piano wire weighing 6.00 g and having a length of 90.0 cm emits a fundamental frequency corresponding to the "Middle C" (v = 261.63 Hz). Find the tension in the wire.
View SolutionA sonometer wire having a length of 1.50 m between the bridges vibrates in its second harmonic in resonance with a tuning fork of frequency 256 Hz. What is the speed of the transverse wave on the wire ?
View SolutionThe length of the wire shown in figure between the pulley is 1.5 m and its mass is 12.0 g. Find the frequency of vibration with which the wire vibrates in two loops leaving the middle point of the wire between the pulleys at rest.

View SolutionA one-metre long stretched string having a mass of 40 g is attached to a tuning fork. The fork vibrates at 128 Hz in a direction perpendicular to the string. What should be the tension in the string if it is to vibrate in four loops ?
View SolutionA wire, fixed at both ends is seen to vibrate at a resonant frequency of 240 Hz and also at 320 Hz. (a) What could be the maximum value of the fundamental frequency ? (b) If transverse waves can travel on this string at a speed of 40 m s t, what is its length ?
View SolutionA string, fixed at both ends, vibrates in a resonant mode with a separation of 2.0 cm between the consecutive nodes. For the next higher resonant frequency, this separation is reduced to 1.6 cm. Find the length of the string.
View SolutionA 660 Hz tuning fork sets up vibration in a string clamped at both ends. The wave speed for a transverse wave on this string is `1220 m s^-1` and the string vibrates in three loops. (a) Find the length of the string. (b) If the maximum amplitude of a particle is 0.5 cm, write a suitable equation describing the motion.
View SolutionA particular guitar wire is 30.0 cm long and vibrates at a frequency of 196 Hz when no finger is placed on it. The next higher notes on the scale are 220 Hz, 247 Hz, 262 Hz and 294 Hz. How far from the end of the string must the finger be placed to play these notes ?
View SolutionA steel wire fixed at both ends has a fundamental frequency of 200 Hz. A person can hear sound of maximum frequency 14 kHz. What is the highest harmonic that can be played on this string which is audible to the person ?
View SolutionThree resonant frequencies of a string are 90, 150 and 210 Hz. (a) Find the highest possible fundamental frequency of vibration of this string. (b) Which harmonics of the fundamental are the given frequencies ? (c) Which overtones are these frequencies ? (d) If the length of the string is 80 cm, what would be the speed of a transverse wave on this string ?
View SolutionTwo wires are kept tight between the same pair of supports. The tensions in the wires are in the ratio 2 : 1, the radii are in the ratio 3 : 1 and the densities are in the ratio 1 : 2. Find the ratio of their fundamental frequencies.
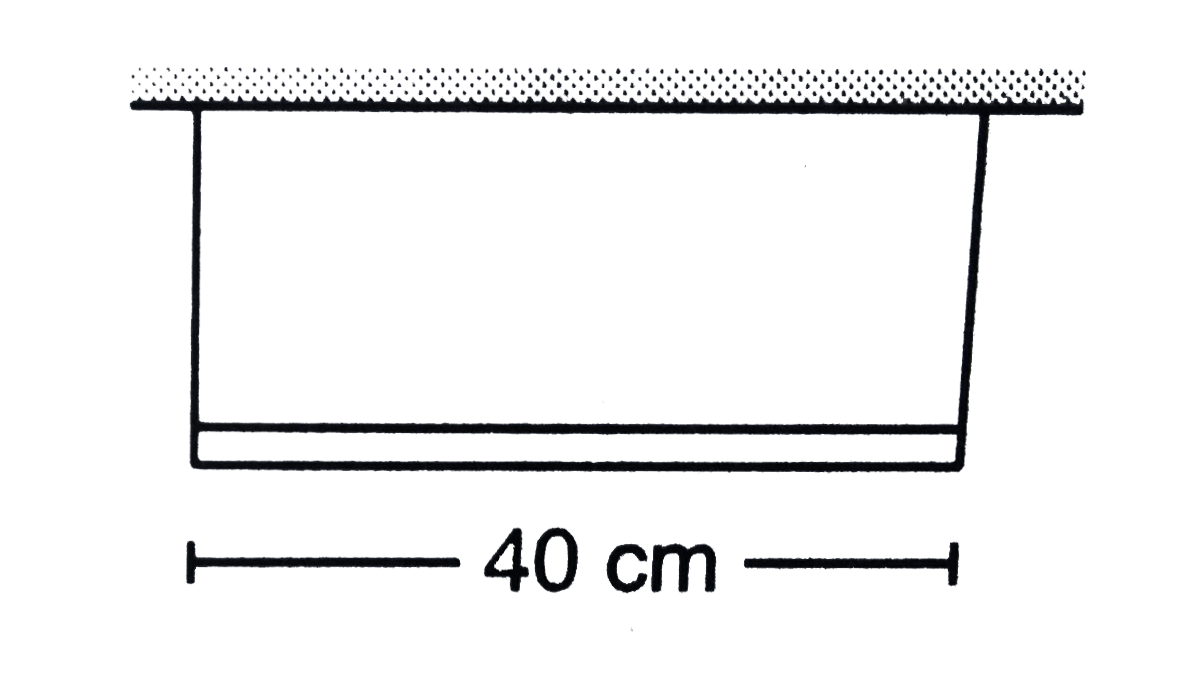
View SolutionA uniform horizontal rod of length 40 cm and mass 1.2 kg is supported by two identical wires as shown in figure. Where should a mass of 4.8 kg be placed on the rod so that the same tuning fork may excite the wire on left into its fundamental vibrations and that on right into its first overtone ? Take `g=10ms^-2`
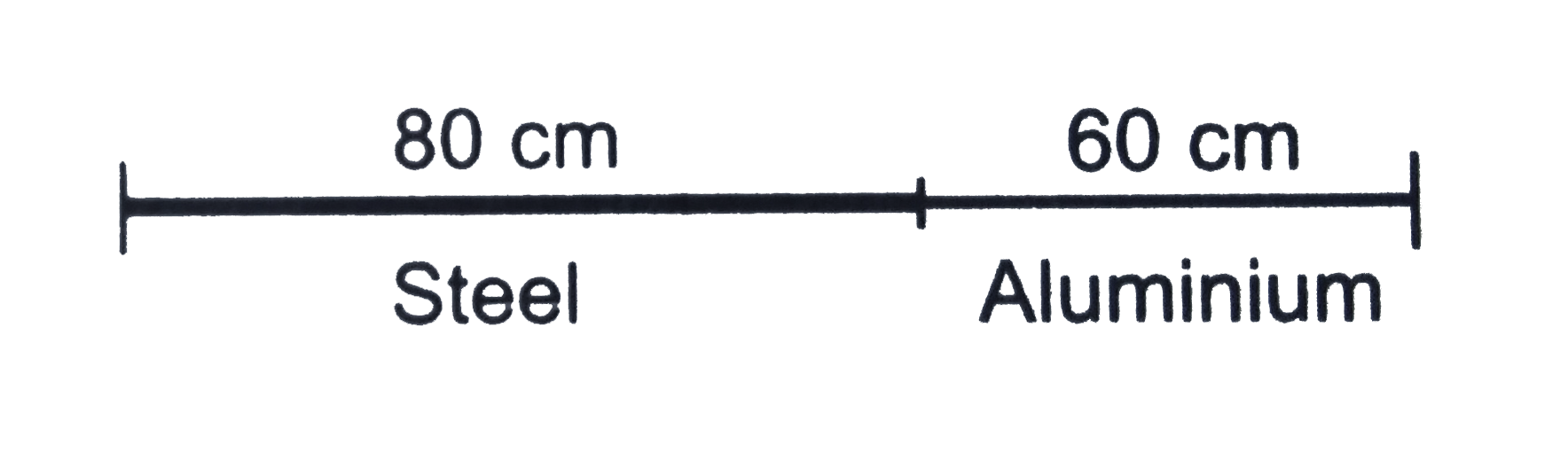
View SolutionFigure shows an aluminium wire of length 60 cm joined to a steel wire of length 80 cm and stretched between two fixed supports. The tension produced is 40 N. The cross-sectional area of the steel wire is .`1.0 mm^2 ` and that of the aluminium wire is 3.0 mm 2. What could be the minimum frequency of a tuning fork which can produce standing waves in the system with the joint as a node ? The density of aluminium is `2.6 g cm^-3`and that of steel is ` 7.8gcm^-3`
View SolutionA string of length L fixed at both ends vibrates in its fundamental mode at a frequency v and a maximum amplitude A. (a) Find the wavelength and the wave number k. (b) Take the origin at one end of the string and the X-axis along the string. Take the Y-axis along the direction of the displacement. Take t = 0 at the instant when the middle point of the string passes through its mean position and is going towards the positive y-direction. Write the equation describing the standing wave.
View SolutionA 2 m-long string fixed at both ends is set into vibrations in its first overtone. The wave speed on the string is 200 m s and the amplitude is 0.5 cm. (a) Find the wavelength and the frequency. (b) Write the equation giving the displacement of different points as a function of time. Choose the X-axis along the string with the origin at one end and t = 0 at the instant when the point x= 50 cm has reached its maximum displacement.
View SolutionThe equation for the vibration of a string, fixed at both ends vibrating in its third harmonic, is given by ` y = (0.4 cm) sin[(0.314 cm^-1) x] cos[f(600pis^-1)t]`.(a) What is the frequency of vibration ? (b) What are the positions of the nodes ? (c) What is the length of the string ? (d) What is the wavelength and the speed of two travelling waves that can interfere to give this vibration ?
View SolutionThe equation of a standing wave, produced on a string fixed at both ends, is ` y = (0.4 cm) sin[(0.314 cm^-1) x] cos[(600pis^-1)t]` What could be the smallest length of the string ?
View SolutionA 40 cm wire having a mass of 3.2 g is stretched between two fixed supports 40.05 cm apart. In its fundamental mode, the wire vibrates at 220 Hz. If the area of cross section of the wire is `1.0 mm^2`, find its Young modulus.
View SolutionFigure shows a string stretched by a block going over a pulley. The string vibrates in its tenth harmonic in unison with a particular tuning fork. When a beaker containing water is brought under the block so that the block is completely dipped into the beaker, the string vibrates in its eleventh harmonic. Find the density of the material of the block.
 .
.
View SolutionA 2.00 m-long rope, having a mass of 80 g, is fixed at one end and is tied to a light string at the other end. The tension in the string is 256 N. (a) Find the frequencies of the fundamental and the first two overtones. (b) Find the wavelength in the fundamental and the first two overtones.
View SolutionA heavy string is tied at one end to a movable support and to a light thread at the other end as shown in figure. The thread goes over a fixed pulley and supports a weight to produce a tension. The lowest frequency with which the heavy string resonates is 120 Hz. If the movable support is pushed to the right by 10 cm so that the joint is placed on the pulley, what will be the minimum frequency at which the heavy string can resonate ?
 .
.
View Solution
Exercises questions and complete solutions for chapter WAVE MOTION AND WAVES ON A STRING of HC VERMA PHYSICS (HINGLISH) of Class 11