Complete Solutions to Assignment (Section C) (PREVIOUS YEARS QUESTIONS) of chapter OSCILLATIONS of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | OSCILLATIONS |
| AAKASH INSTITUTE | Assignment (Section C) (PREVIOUS YEARS QUESTIONS) |
| Number Of Questions | 43 |
| Academic Year | 2023-24 |
A particle executes linear simple harmonic motion with an amplitude of `3 cm`. When the particle is at `2 cm` from the mean position, the magnitude of its velocity is equal to that of its acceleration. Then, its time period in seconds is
View SolutionA body of mass m is attached to the lower end of a spring whose upper end is fixed. The spring has negligible mass. When the mass m is slightly pulled down and released, it oscillates with a time period of 3s. When the mass m is increased by `1 kg`, the time period of oscillations becomes 5s. The value of m in kg is
View SolutionA particle is executing a simple harmonic motion. Its maximum acceleration is `alpha` and maximum velocity is `beta`. Then, its time period of vibration will be
View SolutionA particle is executing SHM along a straight line. Its velocities at distances `x_(1)` and `x_(2)` from the mean position are `v_(1)` and `v_(2)`, respectively. Its time period is
View SolutionWhen two displacement represented by `y_(1) = a sin (omega t)` and `y_(2) = b cos (omega t)` are superimposed, the motion is
View SolutionThe oscillation of a body on smooth horizontal surface is represented by the equation,`x=A"cos"omegat` where ,x=displacement at time t
`omega`=frequency of oscillation
which one of the following graphs shows correctly the variation of a with t?
Here , a=acceleration at time t
T =time period
View SolutionOut of the following functions representing motion of a particle which represents SHM?
1. `x=sin^(3)omegat`
2. `x=1+omegat+omega^(2)t^(2)`
3. `x=cosomegat+cos3omegat+cos5omegat`
4. `x=sinomegat+cosomegat`
View SolutionTwo particles execute simple harmonic motion of the same amplitude and frequency along close parallel lines. They pass each other moving in opposite directions each time their displacement is half their amplitude. Their phase difference is
View SolutionThe displacement of paritcle along the x-axis is given by x=a `sin^(2) omegat`.The motion of the particle corresponds to
View SolutionThe period of oscillation of mass M suspended from a spring of negligible mass is T. If along with it another mass M is also suspended, the period of oscillation will now be
View SolutionA simple pendulum performs simple harmonic motion about `x=0` with an amplitude a ans time period T. The speed of the pendulum at `x = (a)/(2)` will be
View SolutionWhich one of the following equations of motion represents simple harmonic motion ?
View SolutionTwo simple harmonic motions of angular frequency `100 rad s^(-1)` and `1000 rad s^(-1)` have the same displacement amplitude. The ratio of their maximum accelerations is
View SolutionA point performs simple harmonic oscillation of period T and the equation of motion is given by `x = a sin (omega t + (pi)/(6))`. After the elapse of what fraction of the time period, the velocity of the point will be equal to half of its maximum velocity ?
View SolutionA mass of `2.0 kg` is put on a flat pan attached to a vertical spring fixed on the ground as shown in the figure. The mass of the spring and the pan is negligible. When pressed slightly and released the mas executes a simple harmonic motion. The spring constant is `200 N//m`. What should be the minimum amplitude of the motion, so that the mass gets detached from the pan ? (Take `g = 10 m//s^(2)`)

View SolutionThe phase difference between the instantaneous velocity and acceleration of a particle executing simple harmonic motion is:-
View SolutionThe particle executing simple harmonic motion has a kinetic energy `K_(0) cos^(2) omega t`. The maximum values of the potential energy and the energy are respectively
View SolutionA particle executes simple harmonic oscillation with an amplitudes a. The period of oscillation is T. The minimum time taken by the particle to travel half of the amplitude from the equilibrium position is
View SolutionA rectangular block of mass m and area of cross-section A floats in a liquid of density `rho`. If it is given a small vertical displacement from equilibrium, it undergoes oscillation with a time period T. Then
View SolutionThe circular motion of a particle with constant speed is
View SolutionA particle executing simple harmonic motion of amplitude `5 cm` has maximum speed of `31.4 cm//s`. The frequency of its oscillation is
View Solutionwhich one of following is a simple harmonic motion?
View SolutionA particle is moving along the x-axis and force acting on it is given by `F=F_0sinomegaxN`, where `omega` is a constant. The work done by the force from `x=0` to `x=2` will be
View SolutionWhich one of the following statements is true for the speed v and the acceleration `alpha` of a particle axecuting simple harmonic motion?
View SolutionA particle of mass `m` is released from rest and follow a particle part as shown Assuming that the displacement of the mass from the origin is small which graph correctly depicts the position of the particle as a function of time?
View SolutionIn a simple harmonic motion, when the displacement is one-half the amplitude, what fraction of the total energy is kinetic ?
View SolutionA linear harmonic oscillator of force constant `2 xx 10^(6) Nm^(-1)` and amplitude 0.01 m has a total mechanical energy of 160 J. Its
View SolutionDisplacement between maximum potential energy position and maximum kinetic energy position for a particle executing `S.H.M` is
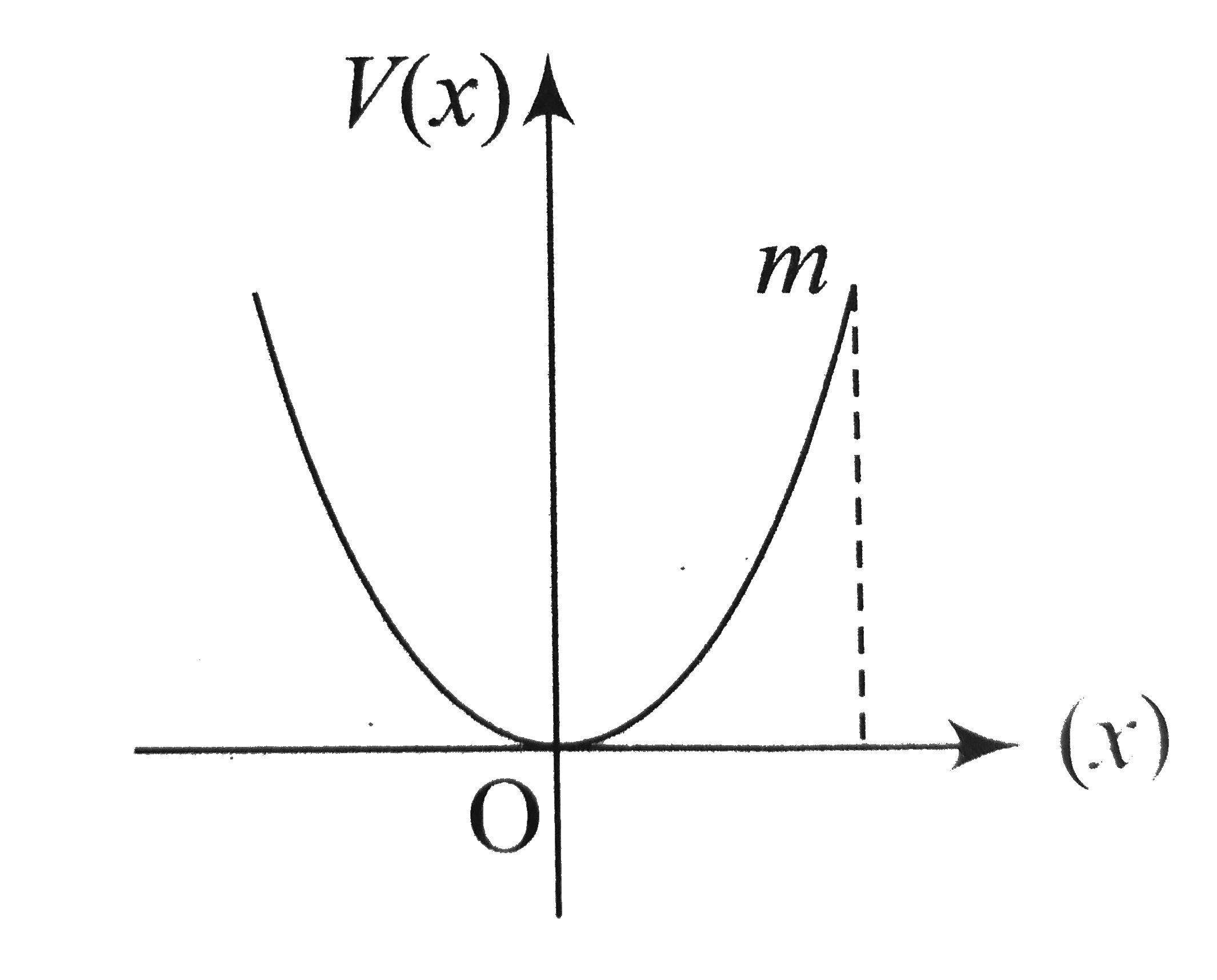
View SolutionA particle of mass m oscillates with simple harmonic motion between points `x_(1)` and `x_(2)` , the equilibrium position being at O. Its potential energy is plotted . It will be given below in the graph
View SolutionThe potential energy of a simple harmonic oscillator when the particle is half way to its end point is
(where, E is the total energy)
View SolutionIf the length of a simple pendulum is increased by 2%, then the time period
View SolutionTwo simple pendulums of length `0.5 m` and `0.2 m` respectively are given small linear displacement in one direction at the same time. They will again be in the same phase when the pendulum of shorter length has completed oscillations
View SolutionTwo sttings A and B length `l_(A) and l_(B) ` and carry masses `M_(A) and M_(B)` at their lower ends . The upper ends being supported by rigid supports. If `n_(A) and n_(B)` are the frequencies of their vibrations and `n_(A) = 2n_(B)` , then
View SolutionA mass m is vertically suspended from a spring of negligible mass, the system oscillates with a frequency n. what will be the frequency of the system, if a mass `4m` is suspended from the same spring?
View SolutionA mass is suspended separately by two springs of spring constant `k_(1)` and `k_(2)` in successive order. The time period of oscillations in the two cases are `T_(1)` and `T_(2)` respectively .If the same mass be suspended by connecting the two springs in parallel, (as shown in figure ) then the timer period of oscillations is T. The correct relation is

View SolutionThe time period of a mass suspended from a spring is T. If is the spring is cut into four equal parts and the same mass is suspend from one of the parts, then the new time period will be -
View SolutionA particle, with restoring force proportional to displacement and resulting force proportional to velocity is subjected to a force `F sin omega t`. If the amplitude of the particle is maximum for `omega = omega_(1)`, and the energy of the particle is maximum for `omega=omega_(2)`, then
View SolutionWhen an oscillator completes 100 oscillation its amplitude reduced to `1/3` of initial value. What will be its amplitude, when it completes 200 oscillation : -
View SolutionInc ase of a forced oscillation, the resonance peak becomes very sharp when the
View SolutionTwo SHM's with same amplitude and time period, when acting together in perpendicular directions with a phase difference of `(pi)/(2)` give rise to
View SolutionThe equations of two waves given as `x = a cos (omega t = delta)` and `y = a cos (omega t + alpha)`, where `delta = alpha + pi/2`, then resultant wave represent:
View SolutionThe damping force on an oscillator is directly proportional to the velocity. The units of the constant to proportionality are
View SolutionA wave has SHM (simple harmonic motion) whose period is 4s while another periods 3 s. If both are combined, then the resultant wave will have the period equal to
View Solution
Assignment (Section C) (PREVIOUS YEARS QUESTIONS) questions and complete solutions for chapter OSCILLATIONS of AAKASH INSTITUTE ENGLISH of Class 12
All Exercises from AAKASH INSTITUTE Class 12 PHYSICS OSCILLATIONS
- ASSIGNMENT ( SECTION-E(ASSERTION-REASON TYPE QUESTION) )
- ASSIGNMENT (SECTION -D( Comprehension) )
- ASSIGNMENT (SECTION-B )
- ASSIGNMENT ( SECTION -A)
- ASSIGNMENT ( SECTION-C )
- ASSIGNMENT ( SECTION-F( MATRIX MATCH TYPE QUESTIONS) )
- ASSIGNMENT ( Section-G( Integers Answer Type Question) )
- ASSIGNMENT ( SECTION-H ( MULTIPLE TRUE-FALSE TYPE QUESTIONS) )
- ASSIGNMENT ( SECTION-I(SUBJECTIVE TYPE QUESTIONS) )
- ASSIGNMENT ( SECTION-J )
- Assignment (Section - A) (OBJECTIVE TYPE QUESTIONS)
- Assignment (Section - B) (OBJECTIVE TYPE QUESTIONS)
- Assignment (Section C) (PREVIOUS YEARS QUESTIONS)
- Assignment (Section D) (ASSERTION-REASON TYPE QUESTIONS)
- EXAMPLE
- EXAMPLE 1
- Exercise
- Try Yourself