Multiple Choice Questions II. from PRADEEP PHYSICS (HINGLISH) SYSTEMS OF PARTICLES AND ROTATIONAL MOTION for Class 11
| Class 11 | PHYSICS |
| Chapter | SYSTEMS OF PARTICLES AND ROTATIONAL MOTION |
| PRADEEP PHYSICS (HINGLISH) | Multiple Choice Questions II. |
| Medium | English |
| Academic Year | 2023-2024 |
Question 32
Fig. shown a three particle system with masses m1=3.0kg,m2=4.0kg and m3=8.0kg. The scales on the axes are set by xs=2.0m and ys=2.0m. What are the co-ordinates of centre if mass ? If m3 is gradually increased, does the centre of mass of the system shift towards or away from that particle or does it remain stationary ?
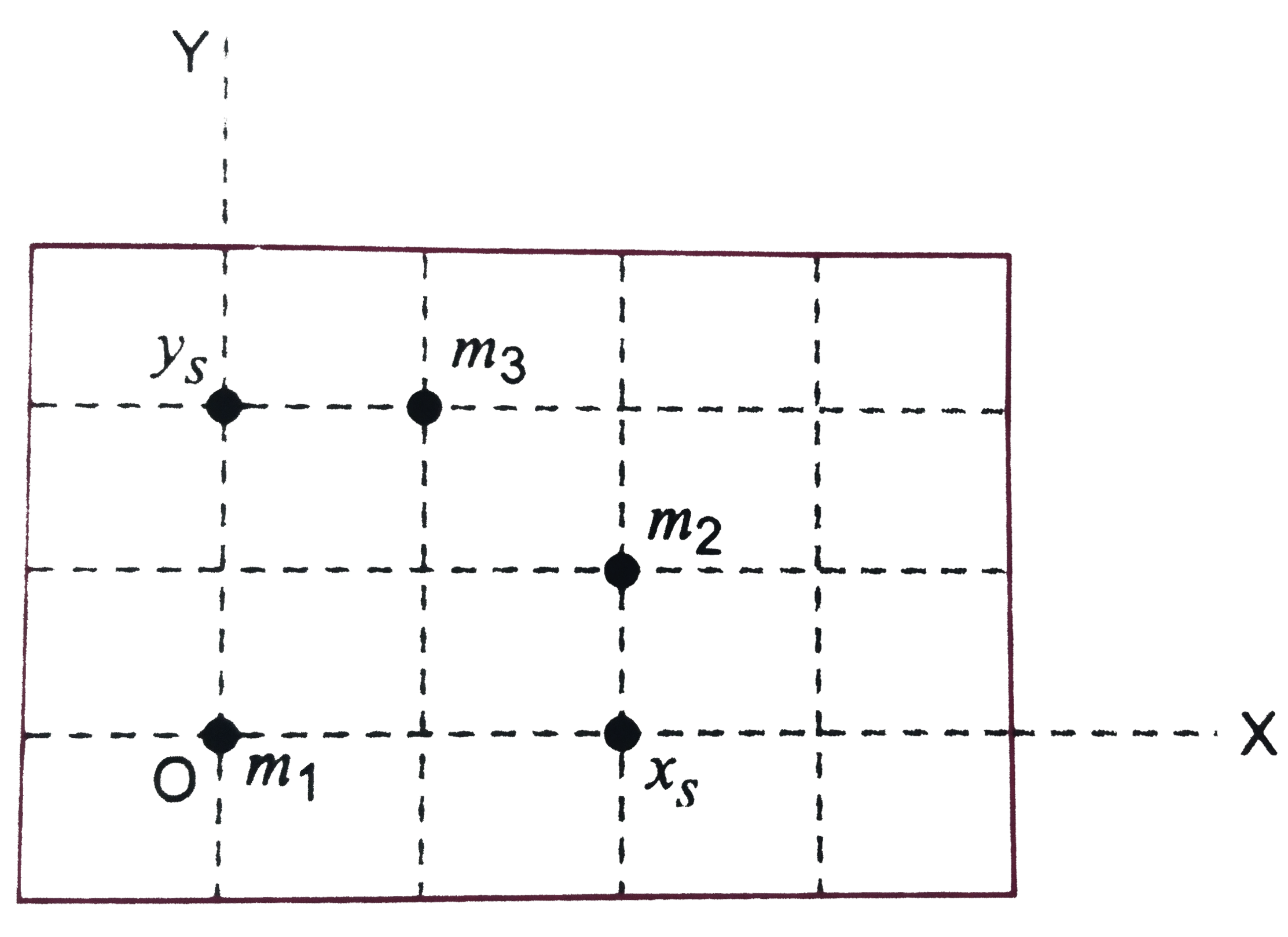 View Solution
View SolutionQuestion 34
A flywheel of mass 25kg has a radius of 0.2m. It is making 240 rpm. What is the torque necessary to bring it to rest in 20s ? If the torque is due to a force applies tangentially on the rim of the wheel, what is the magnitude of the force ? Assume that mass of flywheel is concentrated at its rim.View SolutionQuestion 47
A cord is wound around the circumference of a bicycle wheel (without tyre) of diameter 1m. A mass of 2kg is tied at the end of the cord and it is allowed to fall from rest. The weight falls 2m in 4s. The axle of the wheel is horizontal and the wheel rotates which its plane vertical. if g=10ms−2, what is the angular acceleration of the wheel ?View SolutionQuestion 49
An autmobile travelling at 80km/h has tyres of 75.0cm diameter. What is the angualr speed of the tyres about their axles ? If the car is brought to stop uniformly in 30 complete turns of the tyres without skidding, what is the magnitude of angular acceleration of the wheels ? How far does the car move during the braking ?View SolutionQuestion 52
The centre of gravity of a loaded texi is 1.5m above the ground, and the distance between the wheels is 2m. What is the maximum speed with which it can go round an unbanked curve of radius 100m without being turned upside down. What minimum value of coefficient of friction is needed at this speed ?View SolutionQuestion 81
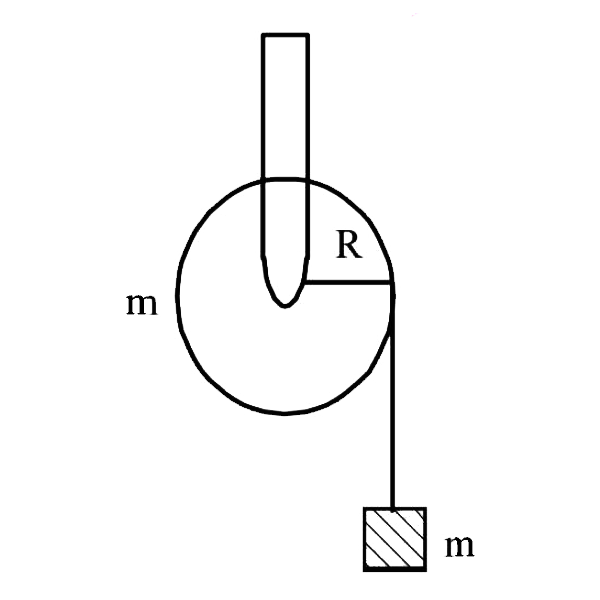
A bucket of mass 8kg is supported by a light rope wound around a solid wooden cylinder of mass 12kgandradius20cm, free to rotate about its axis. A man holding the free end of the rope with the bucket and the cylinder at rest initially, lets go the bucket freely downward in a well 50m deep. Neglecting friction, obtain the speed of bucket and angualr speed of the cylinder just before the bucket enters water. Take g=10m/s2. View SolutionQuestion 89
A wheel of radius 10 cm can rotate freely about its centre as shown in figure. A stirng is wrapped over its rim and is ulle dby a force of 5.0 N. It is found that the torque roduces an angular acceleration 2.0 rads2 in the wheel. Calculate the moment of inertia of the wheel. View Solution
View SolutionQuestion 94
A flywheel of moment of inertia 5.0 kg−m2 is rotated at a speed of 60 rad/s. Because of the friction at the axle, it comes to rest in 5.0 minutes. Find a. The average torque of the friction. B. the total work done by the friction and c. the angular momentum of the wheel 1 minute before it stops rotating.View SolutionQuestion 101
A circular disc of moment of inertia It is rotating in a horizontal plane about its symmetry axis with a constant angular velocity ωi. Another disc of moment of inertia Ib is dropped co-axially onto the rotating disc. Initially, the second disc has zero angular speed. Eventually, both the discs rotate with a constant angular speed ωf. Calculate the energy lost by the initially rotating disc due to friction.View SolutionQuestion 104
Calculate moment of inertia of a uniform circular disc of mass 700g and diameter 20cm about
(i) an axis through the centre of disc and perpendicular to its plane, (ii) a diameter of disc, (iii) a tangent in the plane of the disc, (iv) a tangent perpendicular to the plane of the disc.View SolutionQuestion 108
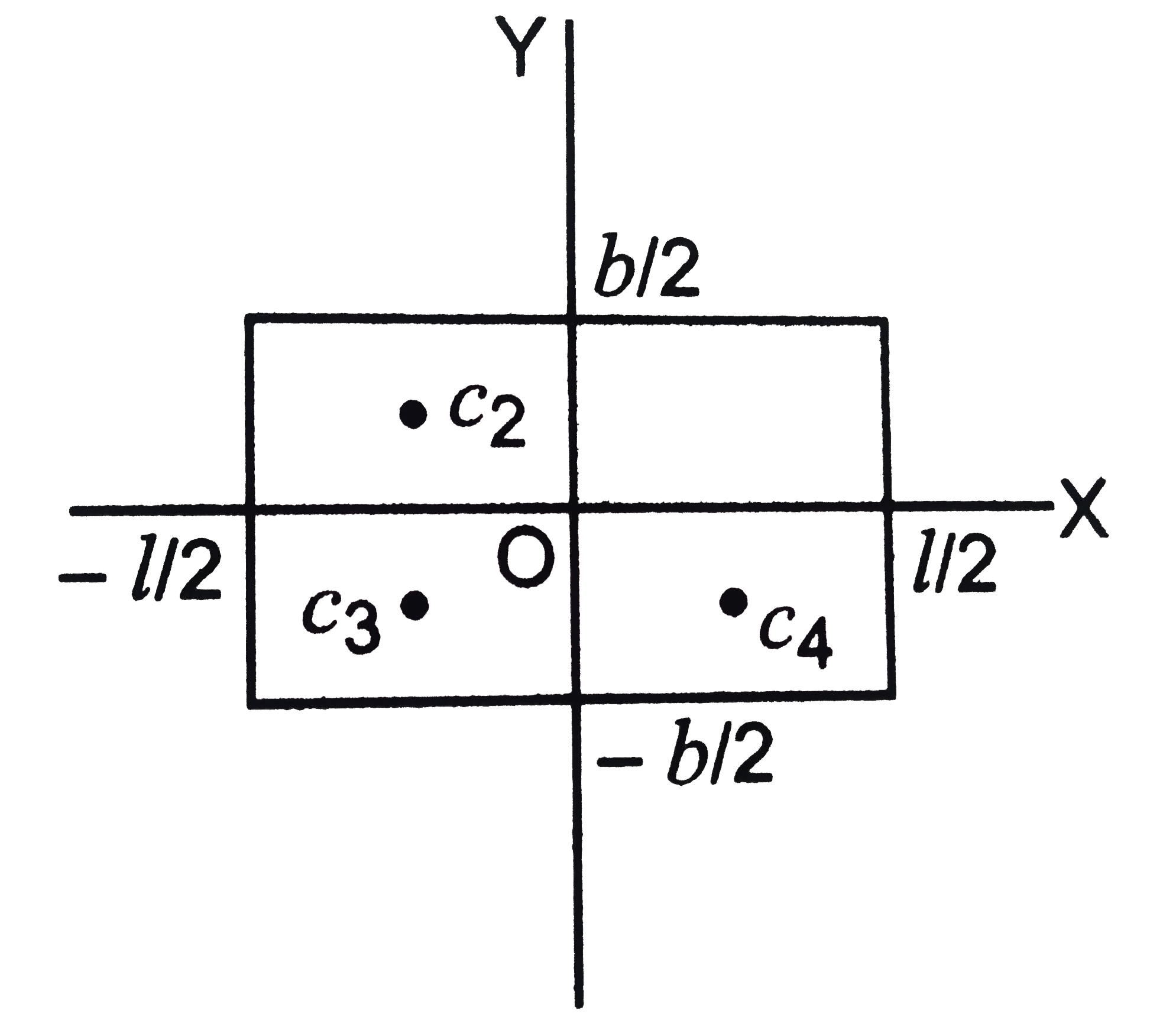
Four particles of masses 4kg,2kg,3kgand5kg are respectively located at the four corners A,B,C,D of a square of side 1m. Calculate the moment of inertia of the system about
(i) the axis passing through point of intersection of the diagonals and perpendicualr to the plane of the square. (ii) side AB (ii) diagonal BD.View SolutionQuestion 116
Two small balls A and B each of mass m, are attched erighdly to the ends of a light rod of length d. The structure rotates about the perpendicular bisector of the rod at an angular speed ω. Calculate the angular momentum of the individual balls and of the system about the axis of rotation.View SolutionQuestion 117
A cylinder of mass 5kg and radius 30cm is rolling down an inclined plane at an angle of 45∘ with the horizontal. Calculate (i) force of friction (ii) acceleration of cylinder (iii) minimum value of coeff. of static friction so that cylinder does not slip while rolling down the plane.View SolutionQuestion 118
A wheel of radius 6cm is mounted so as to rotate about a horizontal axis through its centre. A string of negligibe mass wrapped round its circumference carries a mass of 0.2kg attrached to its free end. When let fall, the mass descends through 1m in 5s. Calculate the angular acceleration of the wheel, its moment of inertia and tension in the cord.View SolutionQuestion 126
A Meery -go-round, made of a ring-like plarfrom of radius RandmassM, is revolving with angular speed ω. A person of mass M is standing on it. At one instant, the person jumps off the round, radially awaay from the centre of the round (as see from the round). The speed of the round after wards isView SolutionQuestion 128
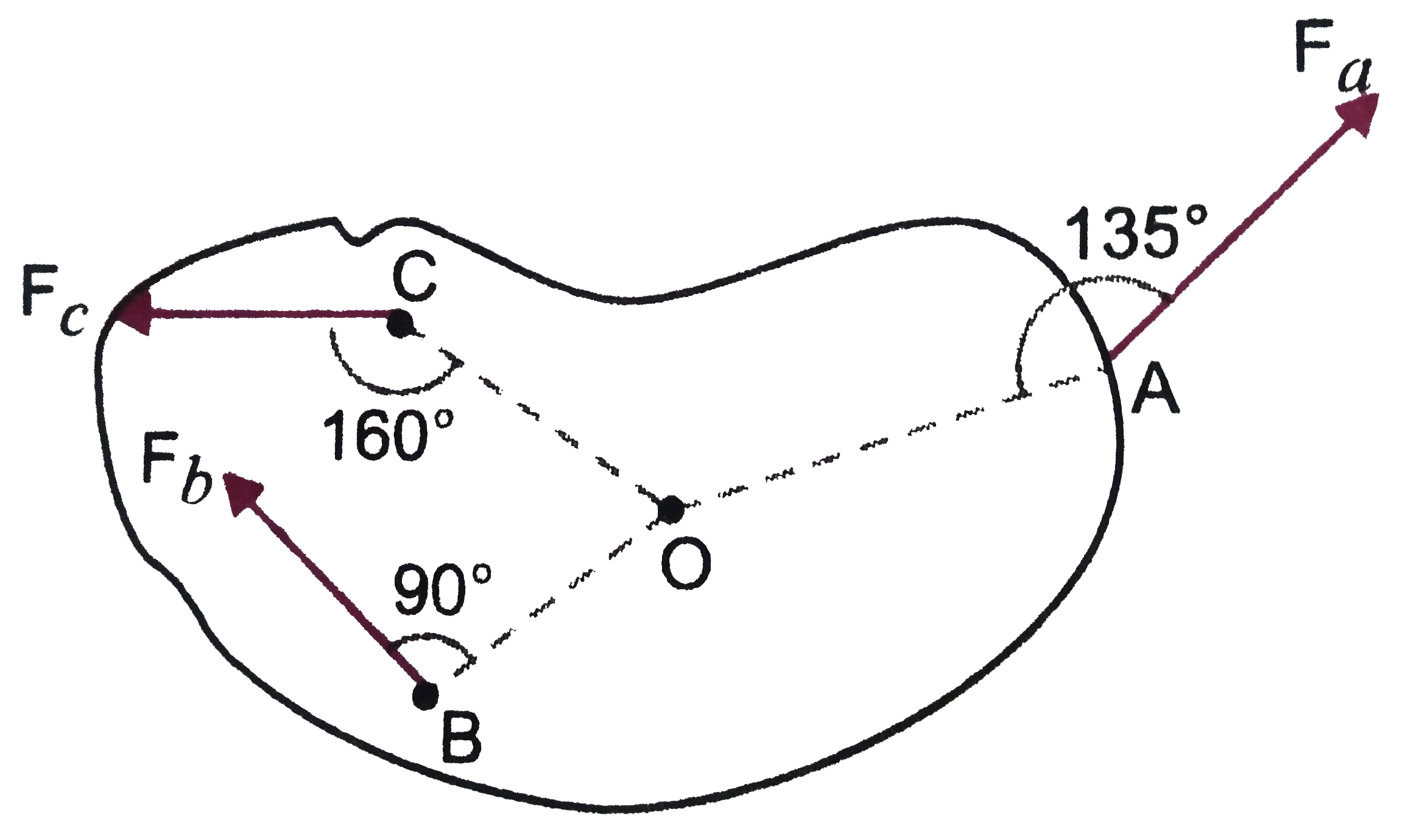
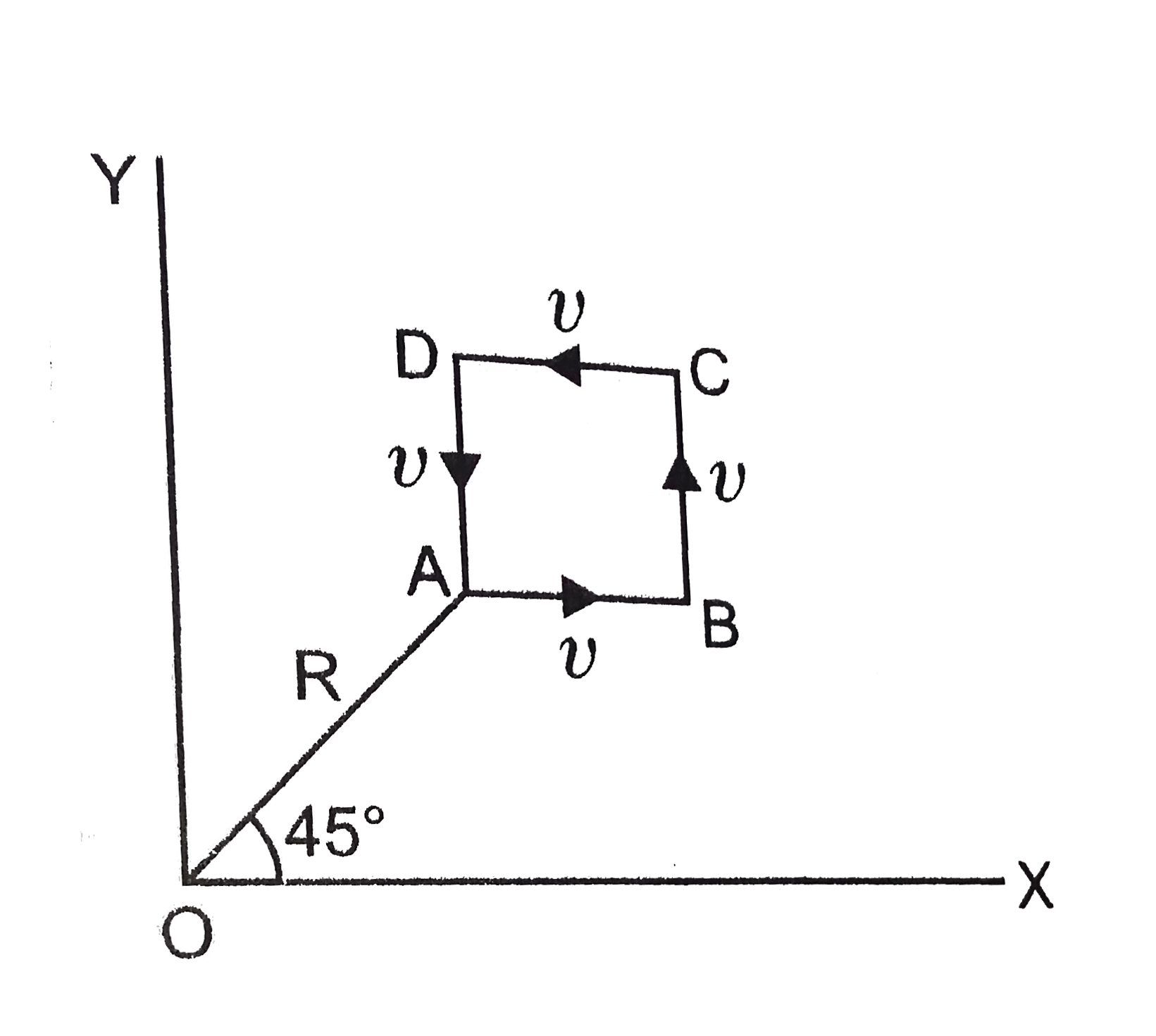
Figure shows two identical particles 1 and 2, each of mass m, moving in opposite directions with same speed →V along parallel lines. At a particular instant, →r1 and →r2 are their respective position vectors drawn from point A which is in the plane of the parallel lines. Which of the following is the correct statement ?
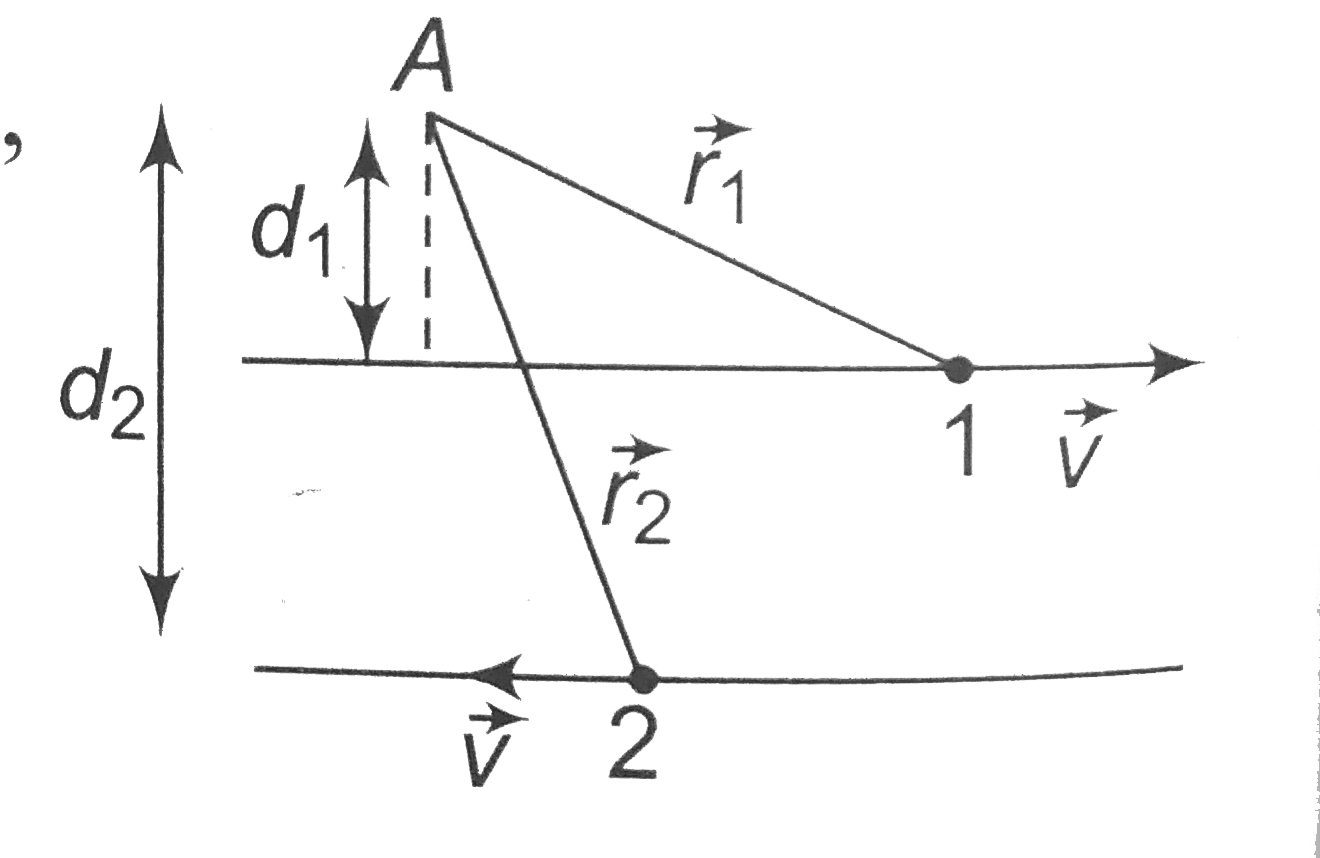 .View Solution
.View SolutionQuestion 136
A thin rod of length 'L' is lying along the x-axis with its ends at x=0 and x=L its linear (mass/length) varies with x as k(xL)n, where n can be zero of any positive number. If to position xCM of the centre of mass of the rod is plotted against 'n', which of the following graphs best apporximates the dependence of xCM on n?View SolutionQuestion 139
(1) Centre of gravity (C.G.) of a body is the point at which the weight of the body acts,
(2) Centre of mass coincides with the centre of gravity if the earth is assumed to have infinitely large radius,
(3) To evaluate the gravitational field intensity due to any body at an external point, the entire mass of the body can be cosidered to be concentrated at its C.G..,
(4) The radius of gyration of any body rotating about ab axis is the length of the perpendicular dropped from thr C.G. the body to the axis. which one of the following paires of statements is correct ?View SolutionQuestion 144
The densities of two solid spheres A and B of the same radii R very with radial distance raspA(r)=k(rR)andpB(r)=k(r(R)5, respectively, where k is a constant . The moments of inertia of the inividual spheres about axes passing throgh their centres are IAandIB respectively. if IBIA=n10, the value of n is View SolutionQuestion 146
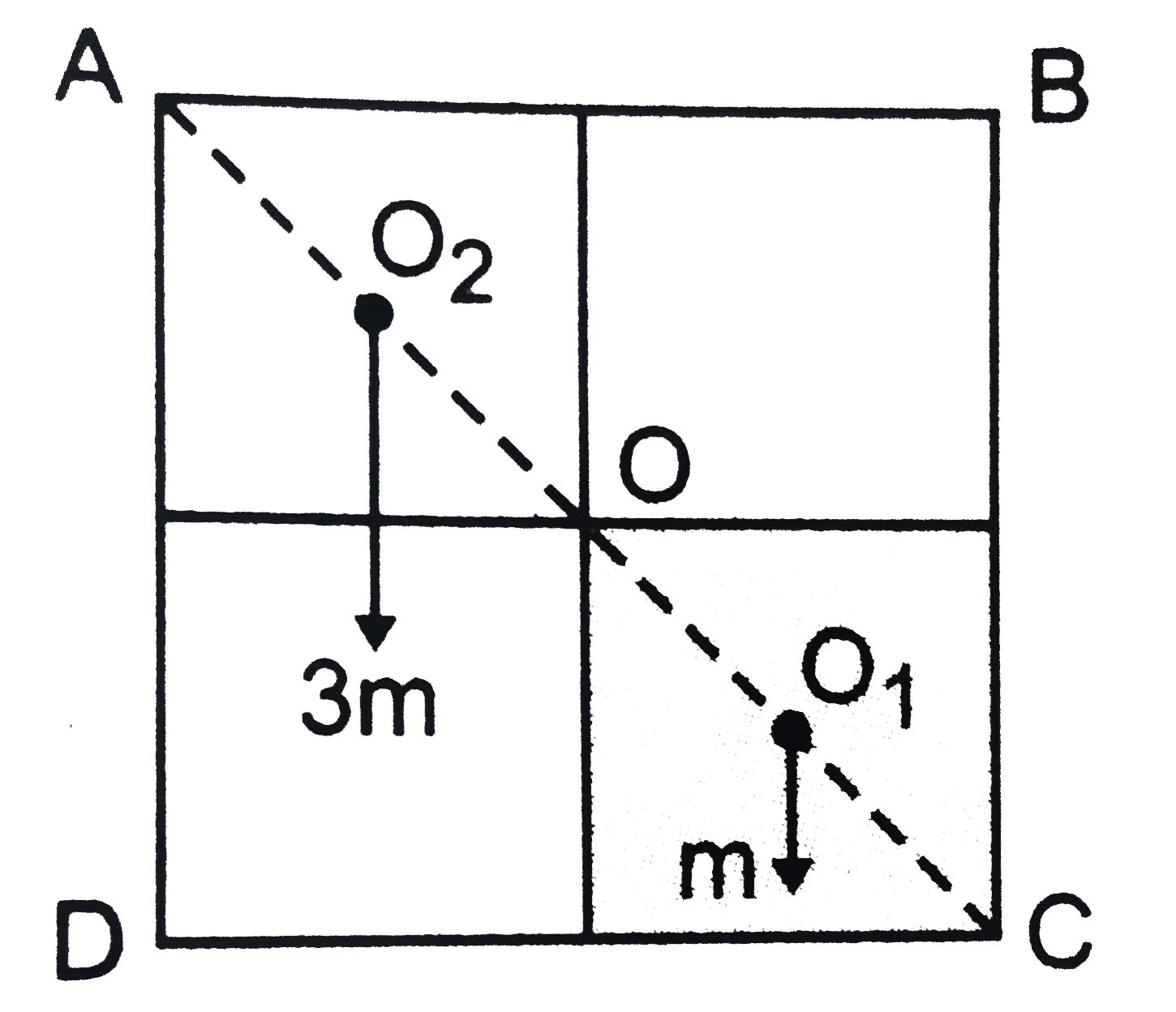
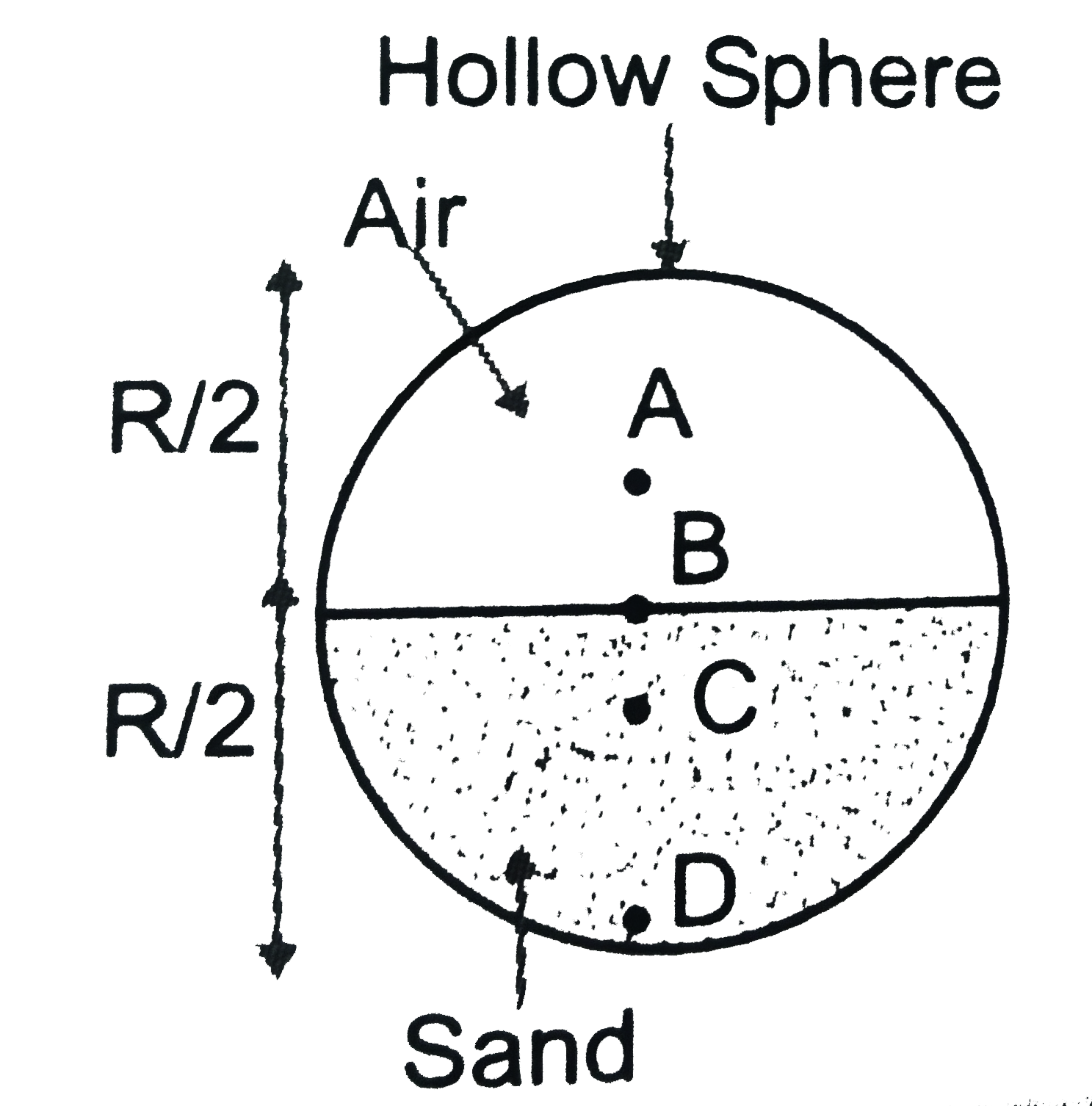
Three idential spherical shells each of mass m and radius r are placed as shown in Fig. Consider an axis XX' which is touching the two shells and passing through diameter of third shell. Moment of Inertia of the system consisting of these three spherical shells about XX' as axis is :
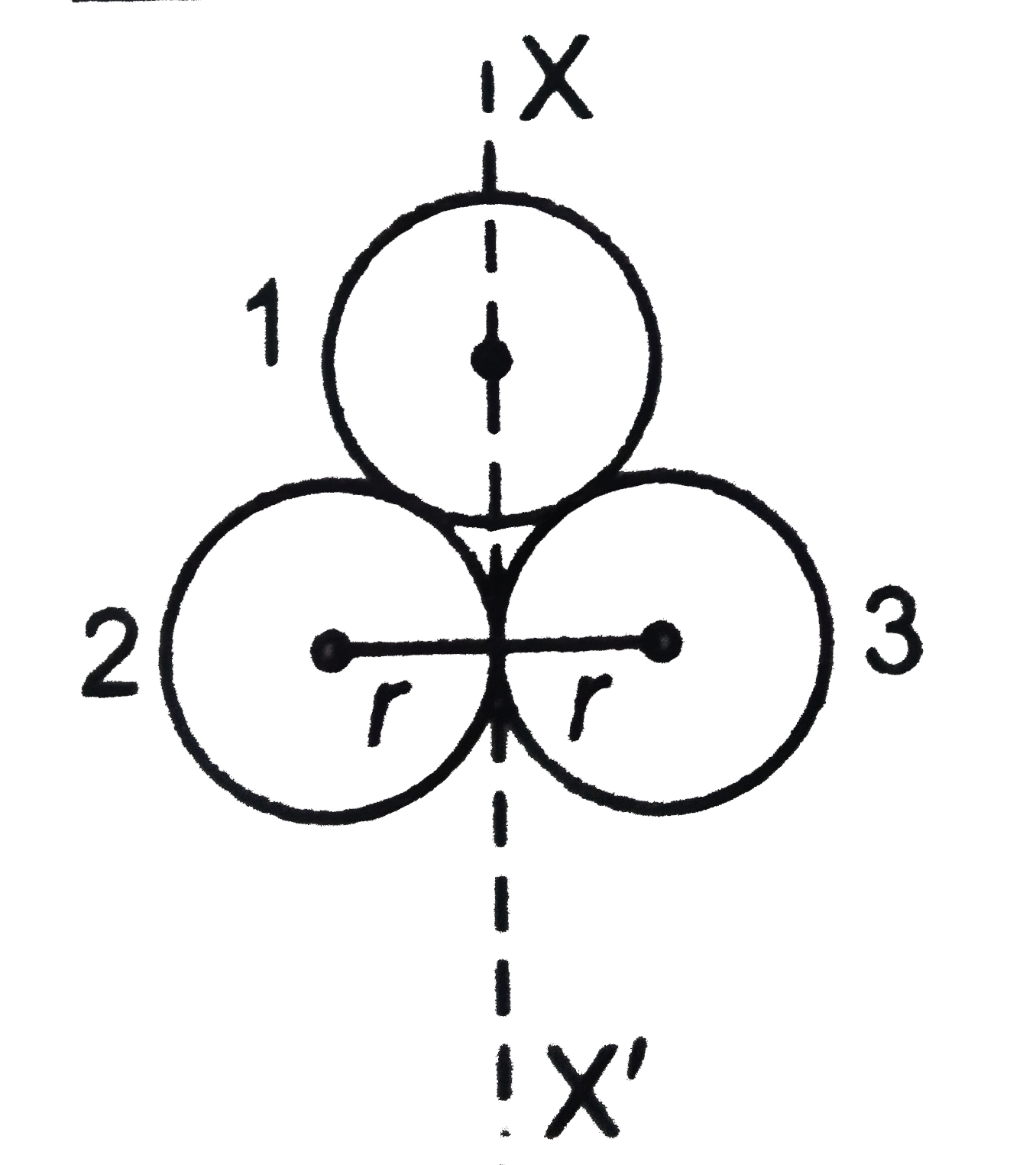 View Solution
View SolutionQuestion 147
Point masses m1andm2 are placed at the opposite ends of a rigid rod of length L, and negligible mass. The rod is to be set rotating about an axis perpendicualr to it. The position of point P on this rod through which the axis should pass so that the work required to set the rod rotating with angular velocity ω0 is minimum, is given by :
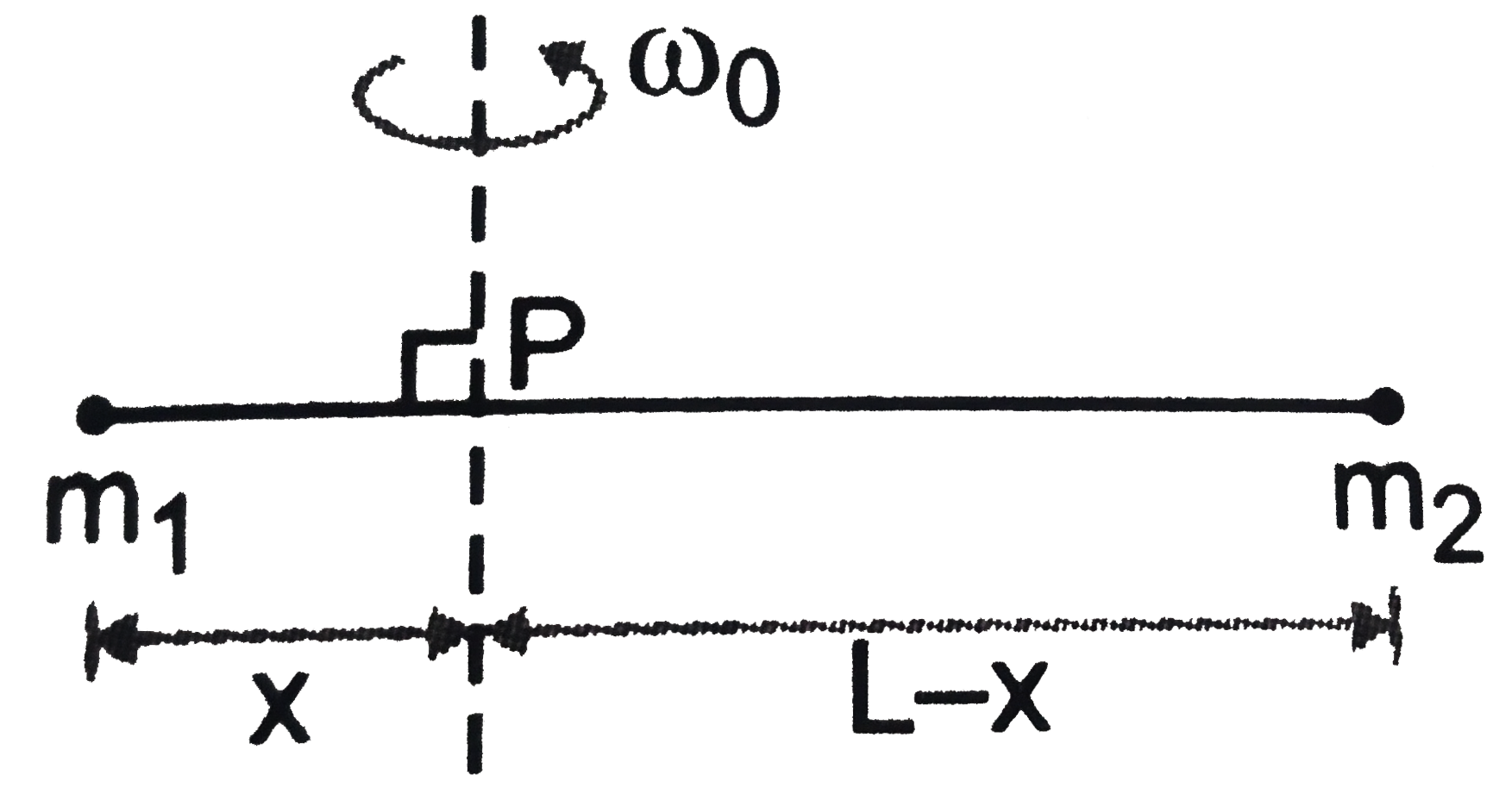 View Solution
View SolutionQuestion 148
If l1 is the moment of inertia of a thin rod about an axis perpendicular to its length and passing thorugh its centre of mass and l2 the moment of inertia of the ring formed by the same rod about an axis passing through the centre of mass of the ring and perpendicular to the plane of the ring. then find the ratio l1l2.View SolutionQuestion 155
A circular disc of moment of inertia It is rotating in a horizontal plane about its symmetry axis with a constant angular velocity ωi. Another disc of moment of inertia Ib is dropped co-axially onto the rotating disc. Initially, the second disc has zero angular speed. Eventually, both the discs rotate with a constant angular speed ωf. Calculate the energy lost by the initially rotating disc due to friction.View SolutionQuestion 157
A pulley has radius 2m is rotated about its axis by a force F=(20t−5t2) newton (where t is measured in seconds) applied tangentially. If the moment of inertia of the pulley about its axis of rotation is 10kgm2 the number of rotations made by the pulley before its direction of motion is reversed, is:View SolutionQuestion 160
An autmobile moves on road with a speed of 54km/h. The radius of its wheel is 0.45m and the moment of inertia of the wheel about its axis of rotation is 3kgm2. If the vehicle is brought to rest in 15s, the magnitude of average torque tansmitted by its brakes to the wheel is : View SolutionQuestion 163
A point P moves in counter - clockwise direction on a circular path as shown in the figure . The movement of P is such that it sweeps out a length s=t3+5 , where s is in metres and t is in seconds . The radius of the path is 20m . The acceleration of P when t=2s is nearly .
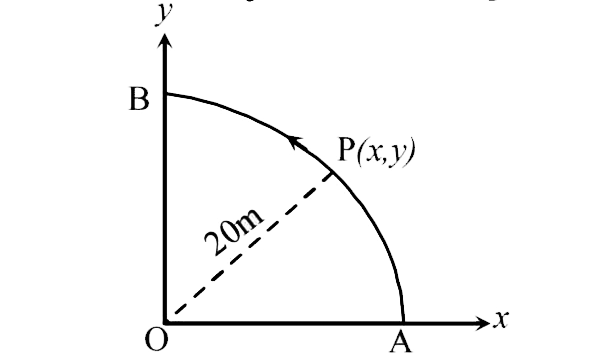 View Solution
View SolutionQuestion 164
A ball of mass (m) 0.5g is attached to the end of a string having length (L) 0.5m. The ball is rotated on a horizontal circular path about vertical axis. The maximum tension that the string can bear is 324N. The maximum possible value of anguar velocity of ball(in radian/s) is
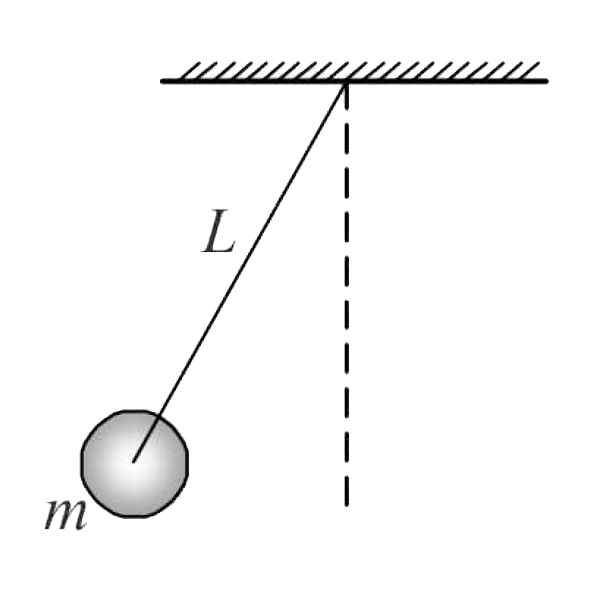 View Solution
View SolutionQuestion 166
A slender uniform rod of mass M and length l is pivoted at one ens so that it can rotate in a vertical plane, Fig. There is negligible friction at the pivot. The free end is held vertically above the pivot and then released. The angular acceleration of the rod when it makes an angle θ with the vertical is
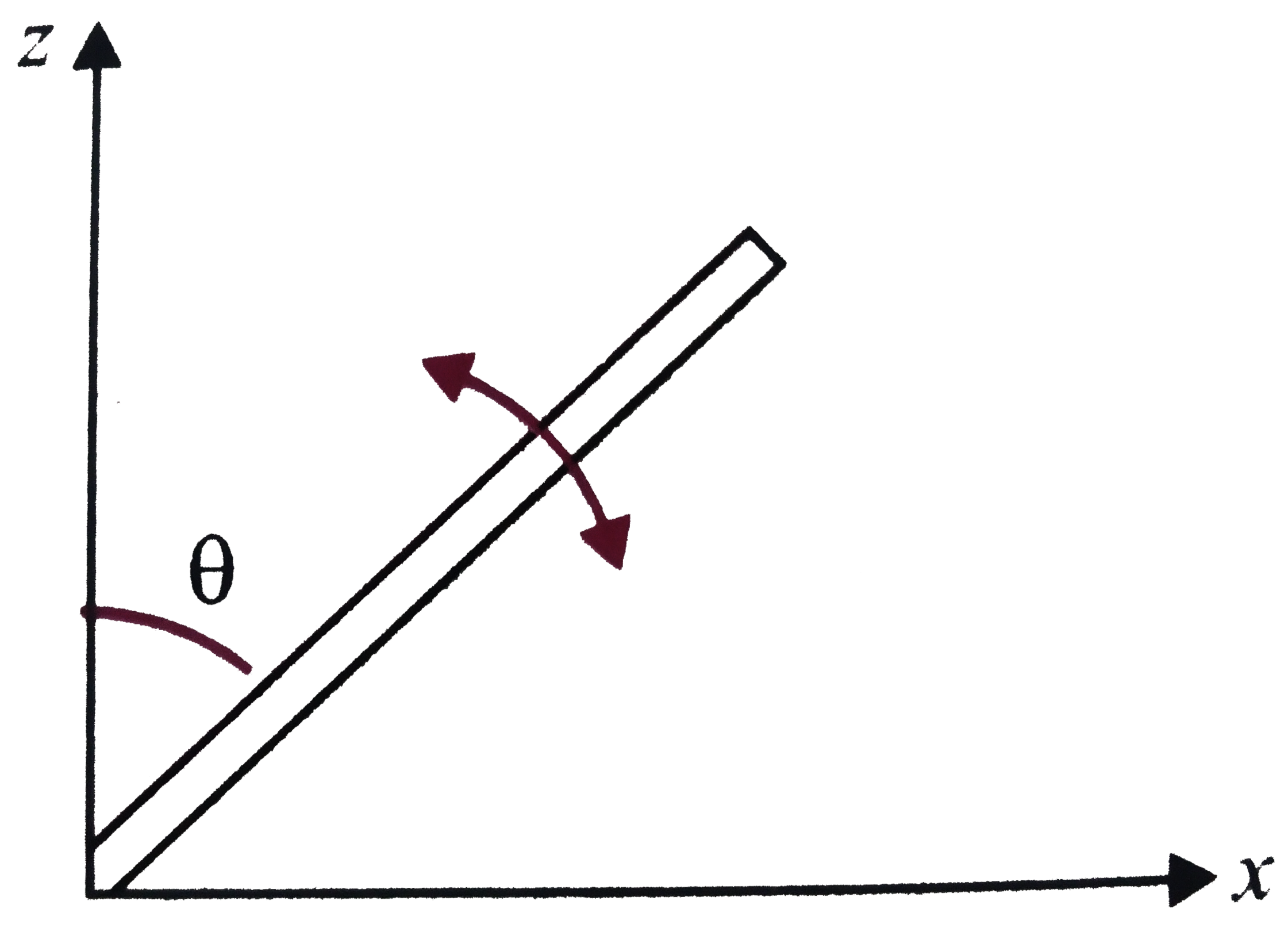 View Solution
View SolutionQuestion 172
A unifrom disc is acted by two equal forces of magnitude F. One of them, acts tangentially to the disc, while other one is acting at the central point of the disc. The friction between disc surface and ground surface in nF. If r be the radius of the disc, then the value of n would be (in N)View SolutionQuestion 174
A hemispherical bowl of radius R si set rotating abouv its axis of symmetry whichis kept vertical. A small blcok kept in the bowl rotates with the bowl without slippingn on its surface. If the surfaces of the bowl is mooth, and the abgel made by the radius through the block with the vertical is θ, find the angular speed at which the bowl is rotating.View SolutionQuestion 175
A uniform circular disc of radius 50cm at rest is free to turn about an axis, which is perpendicular to the plane and passes through its centre. It is subjected to a torque which produces a constant angular acceleration of 2.0rad/s2. Its net acceleration in m/s2 at the end of 2.0s is approximatelyView SolutionQuestion 179
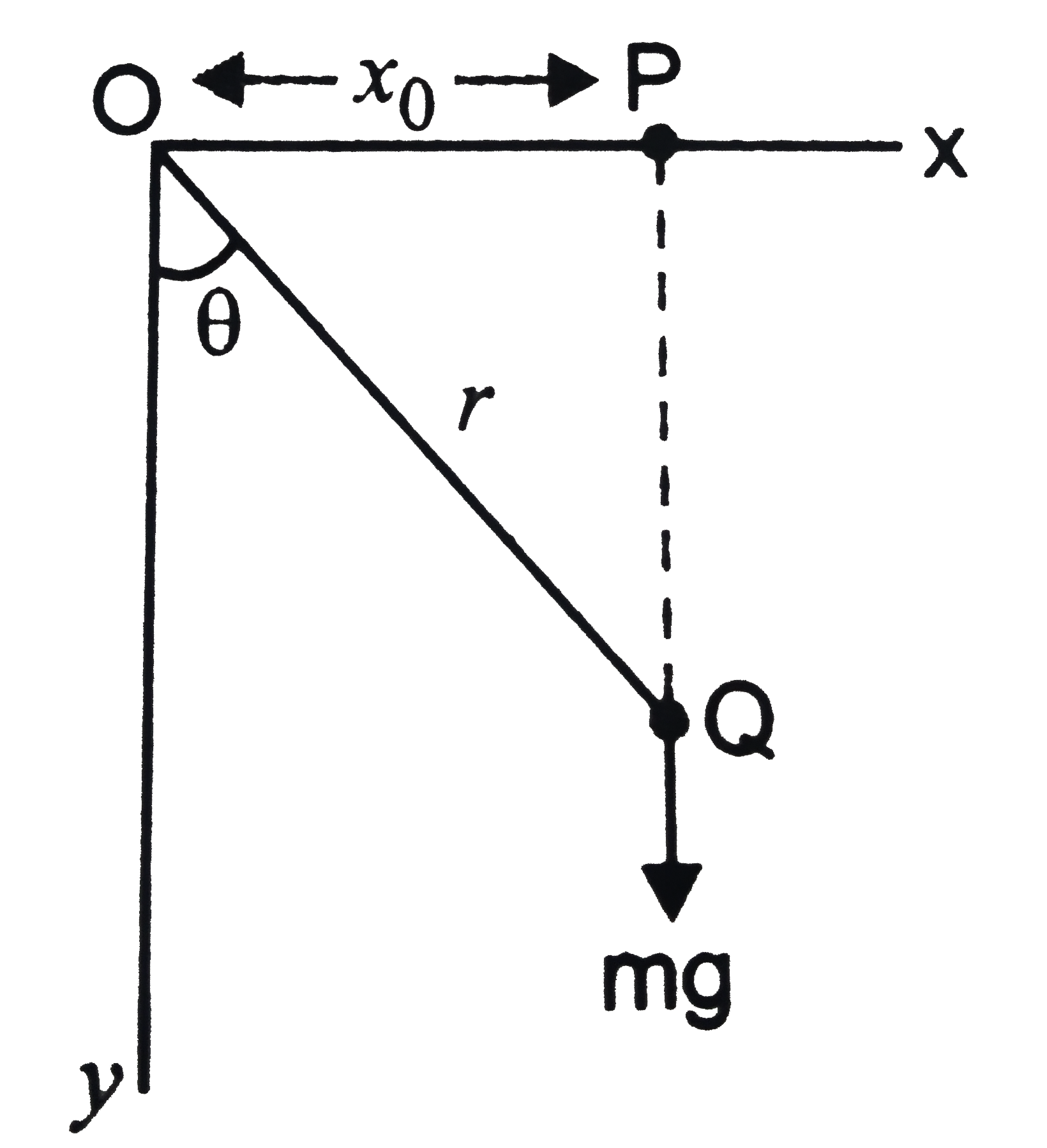
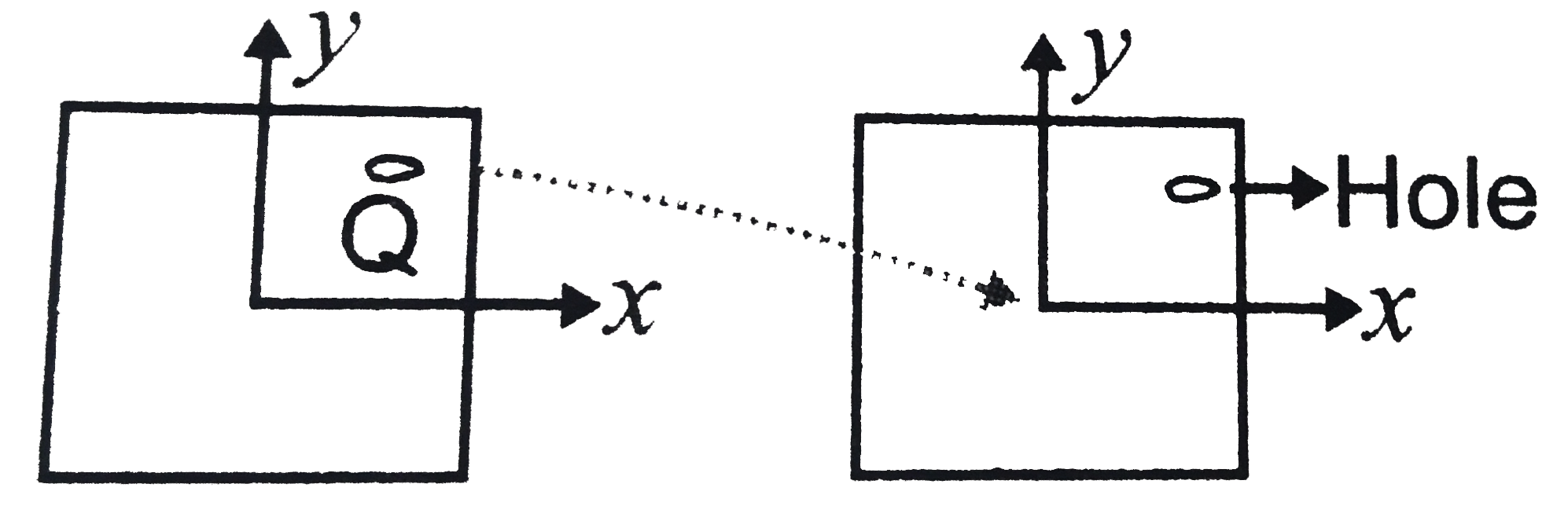
A wire, which passes through the hole in a small bead, is bent in the form of quarter of a circle. The wire is fixed vertically on ground as shown in the figure. The bead is released from near the top of the wire and it slides along the wire without friction. As the bead moves from A to B, the force it applies on the wire is
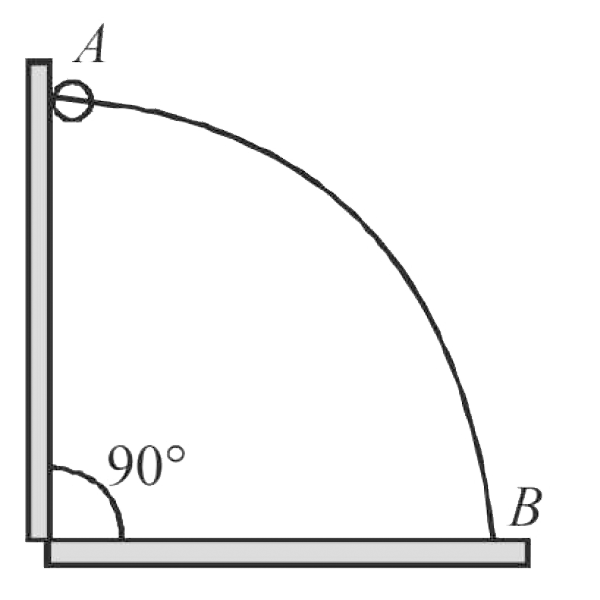 View Solution
View SolutionQuestion 180
A ring of mass M and radius R is rotating with angular speed ω about a fixed vertical axis passing through its centre O with two point masses each of mass M8 at rest at O. These masses can move radially outwards along two massless rods fixed on the ring as shown in the figure. At some instant the angular speed of the system is 89ω and one fo the masses is at a distance of 35R from O. At this instant the distance of the other mass from O is
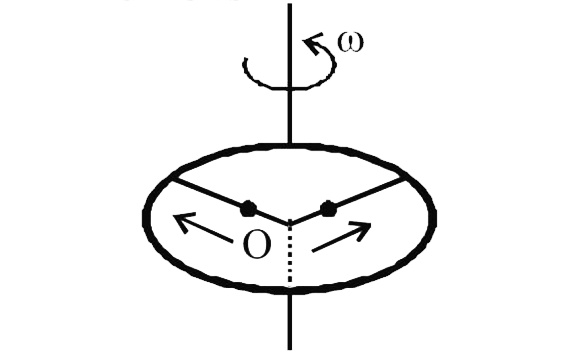 View Solution
View SolutionQuestion 181
A unifrom circular disc of radius 50cm at rest is free to turn about an axis, which is perependicular to the plane and passes through its centre. It is subjected to a torque which produces a constant angualr acceleration of 2.0rad/s2. Its net acceleration in m/s2 at the end of 2.0s is approximatelyView SolutionQuestion 182
A paritcal of mass 10g moves along a circle of radius 6.4cm with a constant tangennitial acceleration. What is the magnitude of this acceleration . What is the magnitude of this acceleration if the kinetic energy of the partical becomes equal to 8×10−4J by the end of the second revolution after the beginning of the motion?View SolutionQuestion 184
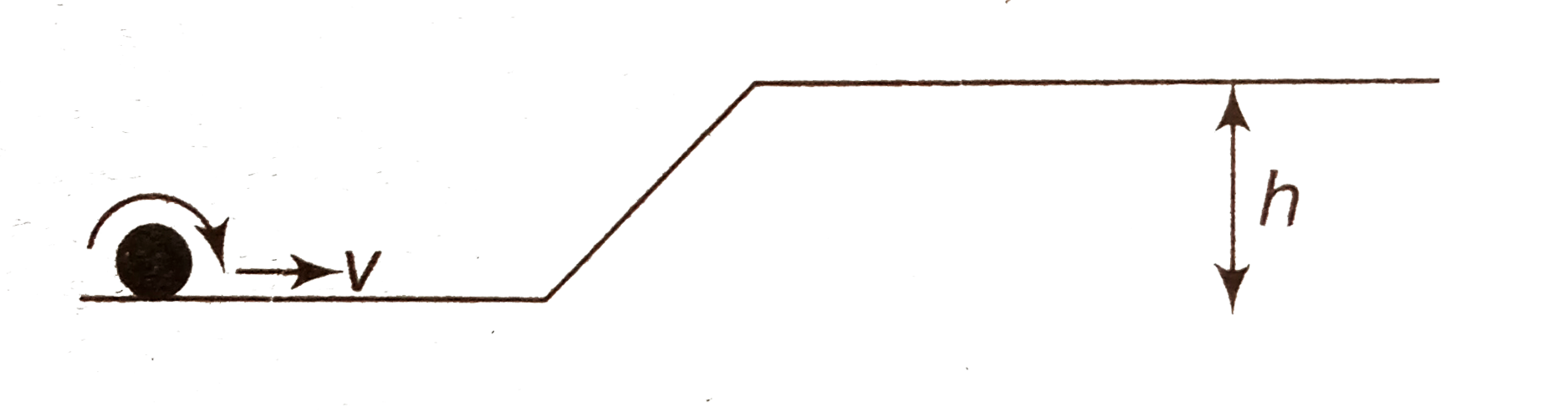
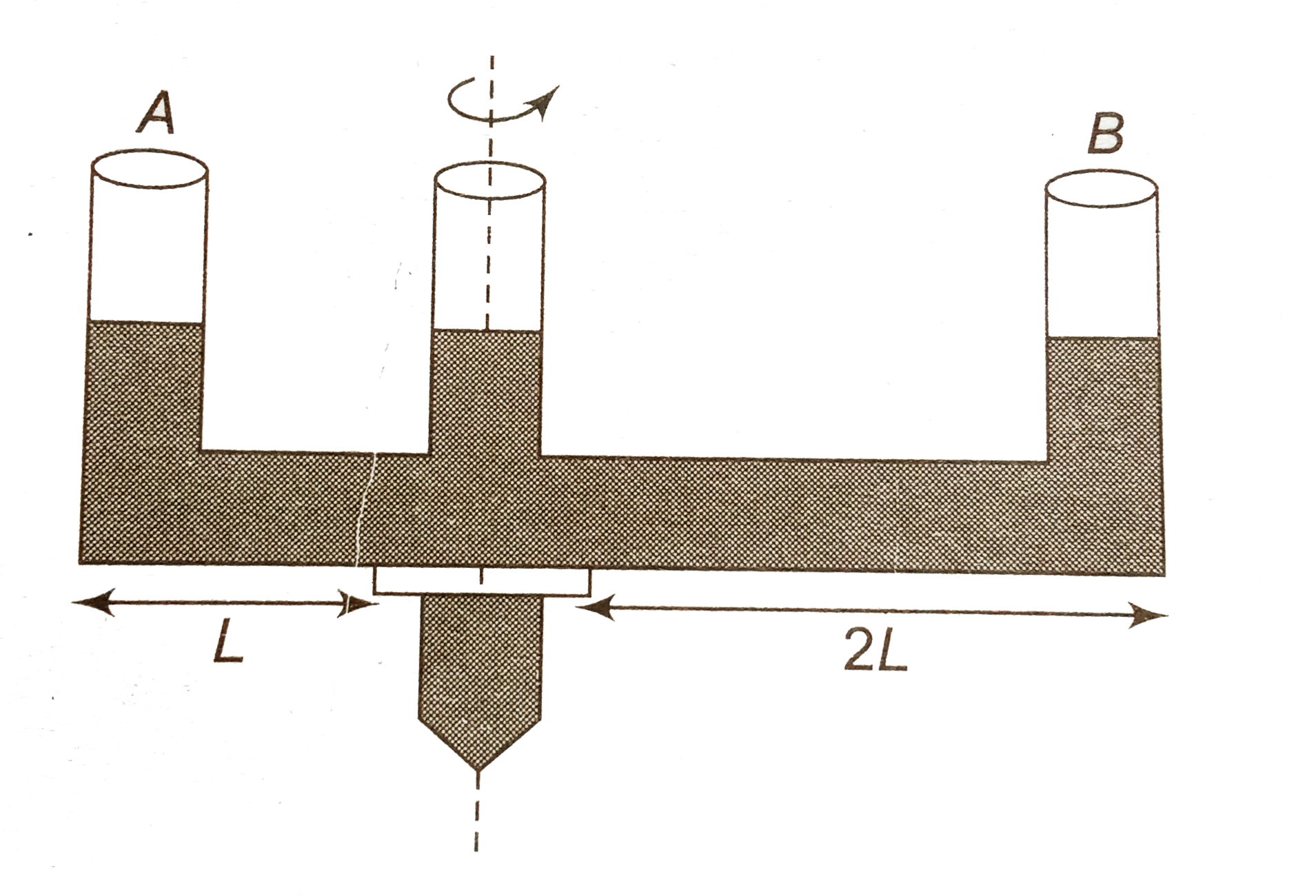
A roller is made by joining together two cones at their vertices O, ti is kept on two rails AB and CD, which are placed asymmetrically with its axis perpendiuclar to CD and its center O at the centre of line joining AB and Cd it is given a light push so that it starts rolling with its centre O moving parallel to CD in the direction shown As it moves, the roller wil tand to:
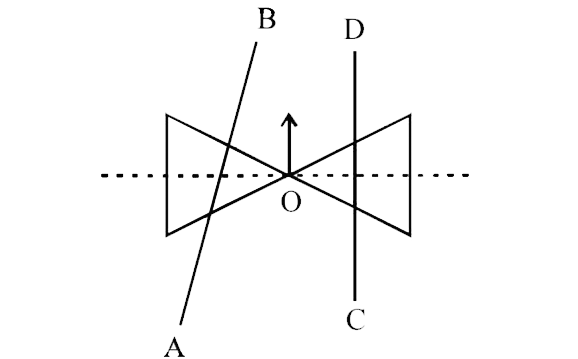 View Solution
View SolutionQuestion 185
A satellite is revolving in a circular orbit at a height 'h' from the earth's surface (radius of earth R, h ltltR). The minimum increase in its orbital velocity required, So that the satellite could escape from the erth's gravitational field, is close to :(Neglect the effect of atomsphere.)View SolutionQuestion 206
A rigid body is made of three indentical thin rods, each of length L, fastened together in the form of letter H, Fig. The body is free to rotate about a horizontal axis that tuns along the length of one of legs of H. The body is allowed to fall from rest from a position in which plane of H is horizontal. the angular speed of body when plane of H is vertical is
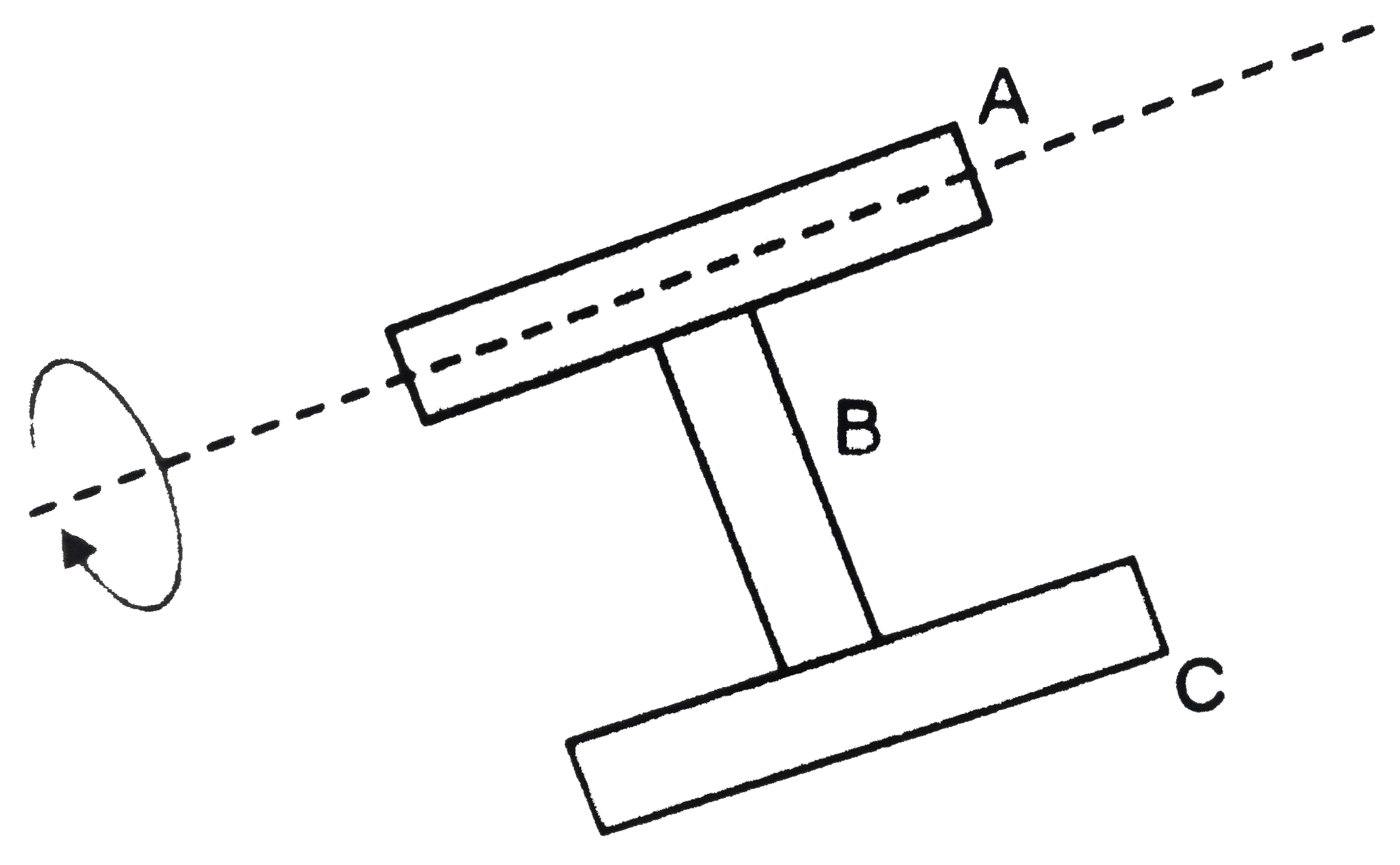 View Solution
View SolutionQuestion 212
A boy is pushng a ring of mass 2kg and radius 0.5 m with a stick as shwon in figure. The stick applies a force of 2N on the ring and rolls it without slipping with an accelertaion of 0.3ms2. The coefficinet of friction between the ground and the ring is large enough that rolling always occurs and the coefficient of friction between the stick and the ring of (P/10). The value of P is
 View Solution
View SolutionQuestion 213
A thin horizontal circular disc is roating about a vertical axis passing through its centre. An insect is at rest at a point near the rim of the disc. The insect now moves along a diameter of the disc to reach its other end. During the journey of the insect, the angular speed of the disc.View SolutionQuestion 217
A solid cylinder of mass 50kg and radius 0.5m is free to rotate about the horizontal axis. A massless string is wound round the cylinder with one end attached to it and other end hanging freely. Tension in the string required to produce an angular acceleration of 2 revolution s−2 isView SolutionQuestion 221
A uniform circular disc of mass 1.5 kg and raius 0.5 m is initially ar rest on a horiozntal frictonless surface. Three forces of equal matgnitude F = 0.5 N are applied simultaneously along the three sides of an equilateral triangle XYZ with its vertices on the perimeter of the disc (see figure). One second after applying the forces the angular speed of the disc in rads−1 is
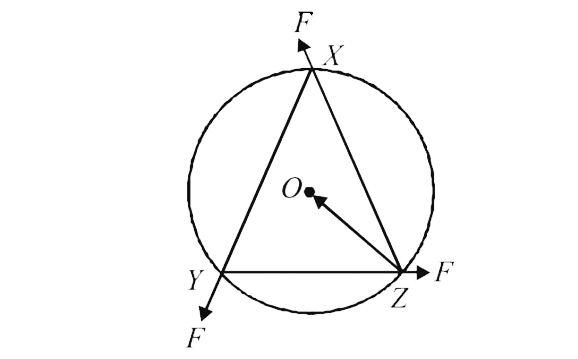 View Solution
View SolutionQuestion 222
A horizontal circular platform of radius 0.5 m and mass axis. Two massless spring toy-guns, each carrying a steel ball of mass 0.05 kg are attached to the platform at a distance 0.25m from the centre on its either sides along its diameter (see figure). Each gun simultaneously fires the balls horizontally and perpendicular to the diameter in opposite directions. After leaving the platform, the balls have horizontal speed of 9ms−1 with respect to the ground. The rotational speed of the platform in rads−1 after the balls leace the platform is
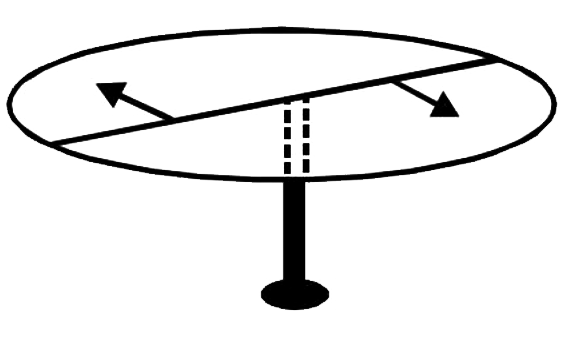 View Solution
View SolutionQuestion 223
A thin uniform rod, pivoted at O, is rotating in the horizontal plane with constatn angular speed ω, as shown in the figure. At time t = 0, a small insect starts from O and moves with constant sped v, with respect to the rod towards the other end. It reaches the end of the rod at t =T and stops. The angular speed of the system remains ω throughout. The magnitude of the torque (|→π|) about O, as a function of time is best represented by which plot?
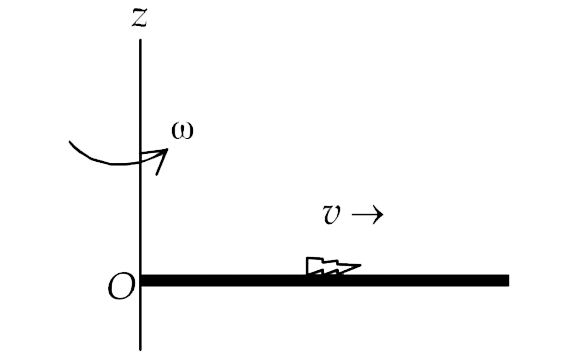 View Solution
View SolutionQuestion 224
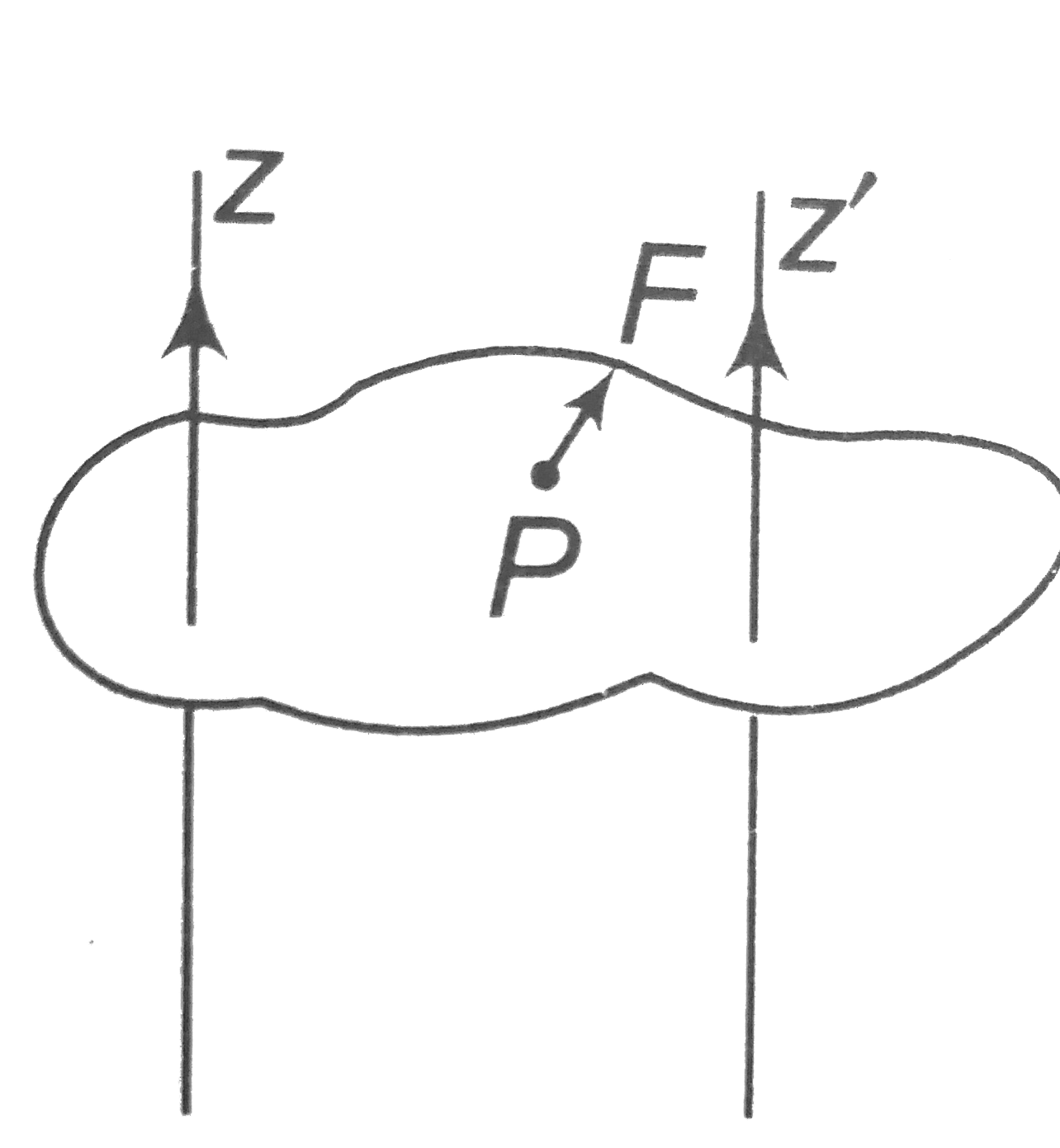
A small mass m is attached to a massless string whose other end is fixed at P as shown in the figure. The mass is undergoing circular motion in the x-y plane with centre at O and constant angular speed ω. If the angular momentum of the system. calculated about O and P are denoted. by →LOand→LP respectively, then.
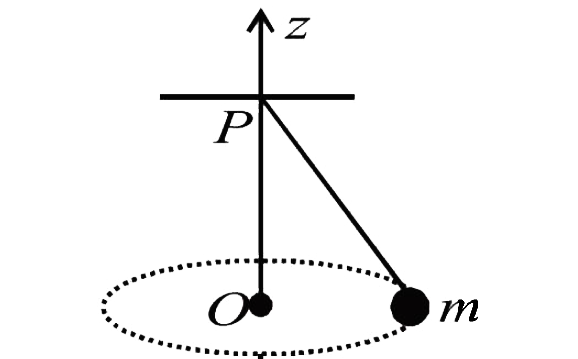 View Solution
View SolutionQuestion 225
Two identical discs of same radius R are rotating about their axes in opposite directions with the same constant angular speed ω . The discs are in the same horizontal plane. At time t=0 , the points P and Q are facing each other as shown in the figure. The relative speed between the two points P and Q is vr. In one time period (T) of rotation of the discs , vr as a function of time is best represented by
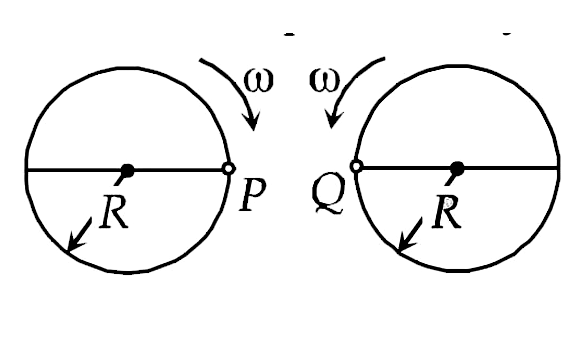 View Solution
View SolutionQuestion 226
A circular platform is mounted on a frictionless vertical axle. Its radius R=2m and its moment of inertia about the axle is 200kgm2. It is initially at rest. A 50kg man stands on the edge at the platform and begins to walk along the edge at the speed of 1ms−1 relative to the ground. Time taken by the man to complete one revolution is :View SolutionQuestion 228
An explosion blows a rock into three parts. Two parts go off at right angles to each other . These two are 1kg first part moving with a velocity of 12ms−1and2kg second part moving with a velocity of 8ms−1. If the third part flies off with a velocity of 4ms−1. Its mass would beView SolutionQuestion 233
A uniform bar of length 6a and mass 8m lies on a smooth horizontal table. Two point masses m and 2m moving in the same horizontal plane with speed 2v and v, respectively, strike the bar [as shown in the fig.] and stick to the bar after collision. Denoting angular velocity (about the centre of mass), total energy and centre of mass velocity by ω, E and vc respecitvely, we have after collison
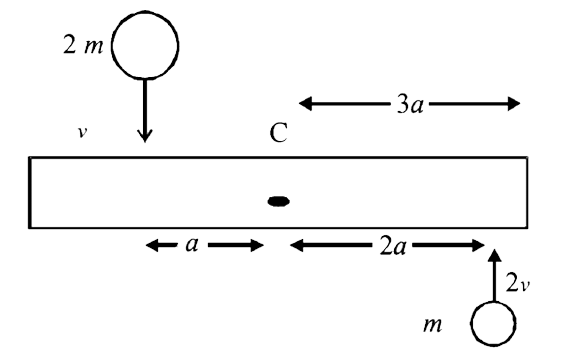 View Solution
View SolutionQuestion 236
A simple pendulum of length L and mass (bob) M is oscillating in a plane about a vertical line between angular limit −ϕ and +ϕ. For an angular displacement θ(|θ|<ϕ), the tension in the string and the velocity of the bob are T and V respectively. The following relations hold good under the above conditions:View SolutionQuestion 239
Two spherical bodies of mass M and 5M & radii R & 2R respectively are released in free space with initial separation between their centres equal to 12R. If they attract each other due to gravitational force only, then the distance covered by the smallar body just before collision is View SolutionQuestion 247
Two solid cylinders P and Q of same mass and same radius start rolling down a fixed inclined plane from the same height at the same time. Cylinder P has most of its mass concentrated near its surface, while Q has most its mass concentrated near the axis. Which statement(s) is (are) correct?View SolutionQuestion 248
The centre of mass of a body is a point at which the entire mass of the body is supposed to be concentrated. The position vector →r of c.m of the system of tow particles of masses m1 and m2 with position vectors →r1 and →r2 is given by
→r=m1→r1+m2→r2m1+m2
For an isolated system, where no external force is acting, −−→vcm= constant
Under no circumstances, the velocity of centre of mass of an isolated system can undergo a change
With the help of the comprehension given above, choose the most appropriate alternative for each of the following questions :
Two bodies of masses 1kg and 2kg are located at (1,2) and (−1,3) respectively. the co-ordinates of the cetre of mass are :View SolutionQuestion 249
The centre of mass of a body is a point at which the entire mass of the body is supposed to be concentrated. The position vector →r of c.m of the system of tow particles of masses m1 and m2 with position vectors →r1 and →r2 is given by
→r=m1→r1+m2→r2m1+m2
For an isolated system, where no external force is acting, −−→vcm= constant
Under no circumstances, the velocity of centre of mass of an isolated system can undergo a change
With the help of the comprehension given above, choose the most appropriate alternative for each of the following questions :
Two bocls of masses 5kg and 2kg are placed on a frictionless surface and connected by a spring. an external kick gives a velocity of 14m/s to heavier block in the direction of lengter one. the velocity gained by the centre of mass is View SolutionQuestion 250
The centre of mass of a body is a point at which the entire mass of the body is supposed to be concentrated. The position vector →r of c.m of the system of tow particles of masses m1 and m2 with position vectors →r1 and →r2 is given by
→r=m1→r1+m2→r2m1+m2
For an isolated system, where no external force is acting, −−→vcm= constant
Under no circumstances, the velocity of centre of mass of an isolated system can undergo a change
With the help of the comprehension given above, choose the most appropriate alternative for each of the following questions :
An electron and a proton move towards eachother with velocities v1 and v2 respectively. the velocity of their centre of mass is View SolutionQuestion 251
The centre of mass of a body is a point at which the entire mass of the body is supposed to be concentrated. The position vector →r of c.m of the system of tow particles of masses m1 and m2 with position vectors →r1 and →r2 is given by
→r=m1→r1+m2→r2m1+m2
For an isolated system, where no external force is acting, −−→vcm= constant
Under no circumstances, the velocity of centre of mass of an isolated system can undergo a change
With the help of the comprehension given above, choose the most appropriate alternative for each of the following questions :
A bomb dropped from an aeroplane in level flight explodes in the middle. the centre of mass of the fragmentsView SolutionQuestion 252
Moment of inertia of a body about a given axis is the rotational inertia of the body about that axis. It is respresented by I=MK2, where M is mass of body and K is radius of gyration of the body about that axis. It is a scalar quantity, which is measured in kgm2. when a body rotates about a given axis, and teh axis of rotates also moves, then total K.E. of body =K.E. of translation +K.E. of rotation
E=12mv2+12Iω2
Which the help of the compreshension given above, choose the most apporpriate altermative for each of the following questions :
Moment of inertia of a body depends on (i) mass of body (ii) size and shape of body (iii) axis of rotation of body (iv) all the above View SolutionQuestion 253
Moment of inertia of a body about a given axis is the rotational inertia of the body about that axis. It is respresented by I=MK2, where M is mass of body and K is radius of gyration of the body about that axis. It is a scalar quantity, which is measured in kgm2. when a body rotates about a given axis, and teh axis of rotates also moves, then total K.E. of body =K.E. of translation +K.E. of rotation
E=12mv2+12Iω2
Which the help of the compreshension given above, choose the most apporpriate altermative for each of the following questions :
A circular disc and a circular ring of same mass and same diameter have, about a given axis, View SolutionQuestion 254
Moment of inertia of a body about a given axis is the rotational inertia of the body about that axis. It is respresented by I=MK2, where M is mass of body and K is radius of gyration of the body about that axis. It is a scalar quantity, which is measured in kgm2. when a body rotates about a given axis, and teh axis of rotates also moves, then total K.E. of body =K.E. of translation +K.E. of rotation
E=12mv2+12Iω2
Which the help of the compreshension given above, choose the most apporpriate altermative for each of the following questions :
A 40kg flywheel in the from of a unifrom circular disc of diameter 1m is making 120r±. Its moment of inertia about a transverse axis through its centre isView SolutionQuestion 255
Moment of inertia of a body about a given axis is the rotational inertia of the body about that axis. It is respresented by I=MK2, where M is mass of body and K is radius of gyration of the body about that axis. It is a scalar quantity, which is measured in kgm2. when a body rotates about a given axis, and teh axis of rotates also moves, then total K.E. of body =K.E. of translation +K.E. of rotation
E=12mv2+12Iω2
Which the help of the compreshension given above, choose the most apporpriate altermative for each of the following questions :
Kinetic energy of rotation of the flywheel in the above case isView SolutionQuestion 266
A uniform circular disc of mass 1.5 kg and raius 0.5 m is initially ar rest on a horiozntal frictonless surface. Three forces of equal matgnitude F = 0.5 N are applied simultaneously along the three sides of an equilateral triangle XYZ with its vertices on the perimeter of the disc (see figure). One second after applying the forces the angular speed of the disc in rads−1 is
 View Solution
View SolutionQuestion 267
A horizontal circular platform of radius 0.5 m and mass axis. Two massless spring toy-guns, each carrying a steel ball of mass 0.05 kg are attached to the platform at a distance 0.25m from the centre on its either sides along its diameter (see figure). Each gun simultaneously fires the balls horizontally and perpendicular to the diameter in opposite directions. After leaving the platform, the balls have horizontal speed of 9ms−1 with respect to the ground. The rotational speed of the platform in rads−1 after the balls leace the platform is
 View Solution
View SolutionQuestion 268
Two identical uniform discs roll without slipping on tow different sufaces AB and CD (see figure) starting at A and C with linear speeds v1andv2 respectively, and always remain in contact with the surfaces. If they reach B and D with the same linear speed v1=3m/sthenv2∈m/sis(g=10m/s2)
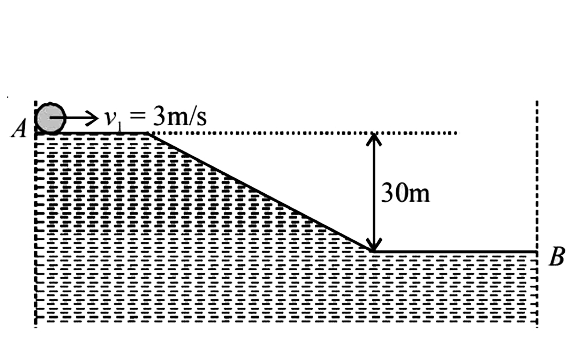
 View Solution
View Solution
All Exercises from PRADEEP PHYSICS (HINGLISH) Class 11 PHYSICS SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- ASSERTION- REASON TYPE QUESTIONS
- FILL IN THE BLANKS
- FOCUS MULTIPLE CHOICE QUESTIONS I.
- INTEGER TYPE QUESTIONS
- MULTIPLE CHOICE QUESTIONS
- MULTIPLE CHOICE QUESTIONS COMPREHENSION 2
- MULTIPLE CHOICE QUESTIONS II.
- MULTIPLE CHOICE QUESTIONS III. COMPREHENSION 1
- MULTIPLE CHOICE QUESTIONS-I
- MULTIPLE CHOICE QUESTIONS-II
- PROBLEMS FOR PRACTICE
- PROBLEMS FOR PRACTICE TYPE A
- PROBLEMS FOR PRACTICE TYPE B
- PROBLEMS FOR PRACTICE TYPE C
- PROBLEMS FOR PRACTICE TYPE D
- PROBLEMS FOR PRACTICE TYPE E
- ADDITIONAL EXERCISES
- ADVANCED PROBLEMS FOR COMPETITIONS
- CONCEPTUAL PROBLEMS
- CONCEPTUAL PROBLEMS I.
- CONCEPTUAL PROBLEMS II.
- CURIOSITY QUESTIONS
- LONG ANSWER QUESTIONS
- NCERT
- QUESTIONS
- SAMPLE PROBLEM
- SHORT ANSWER QUESTIONS
- SHORT ANSWER QUESTIONS I.
- SHORT ANSWER QUESTIONS II.
- SOLVED EXAMPLES
- SOLVED EXAMPLES TYPE A
- SOLVED EXAMPLES TYPE B
- SOLVED EXAMPLES TYPE C
- SOLVED EXAMPLES TYPE D
- SOLVED EXAMPLES TYPE E
- VALUE BASED QUESTIONS
- VERY SHORT ANSWER QUESTIONS
- VERY SHORT ANSWER QUESTIONS I.
- VERY SHORT ANSWER QUESTIONS II.


 .
.


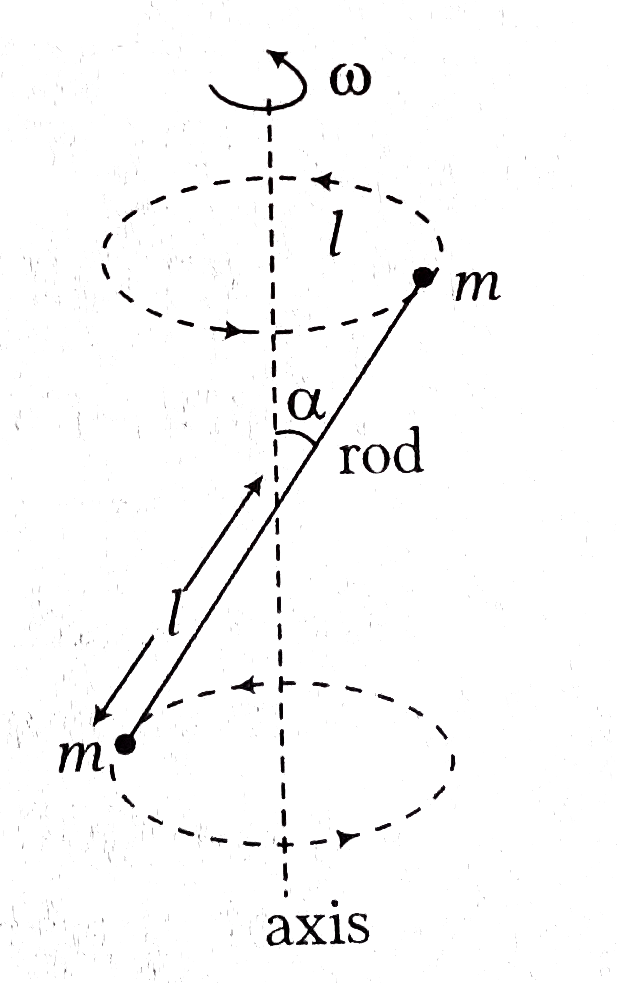


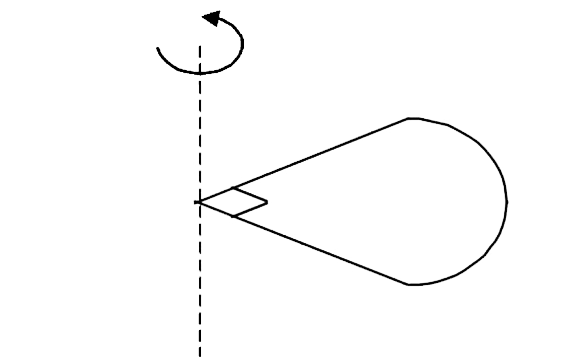
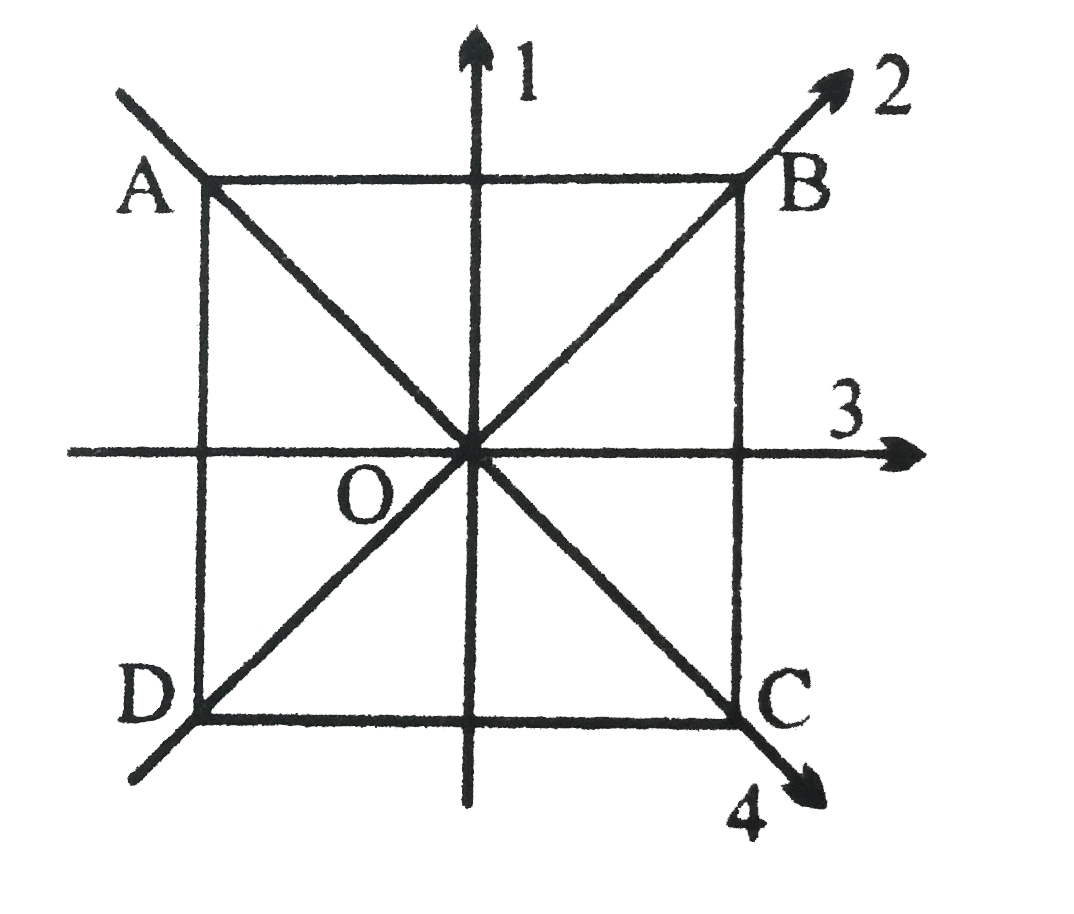 .
.