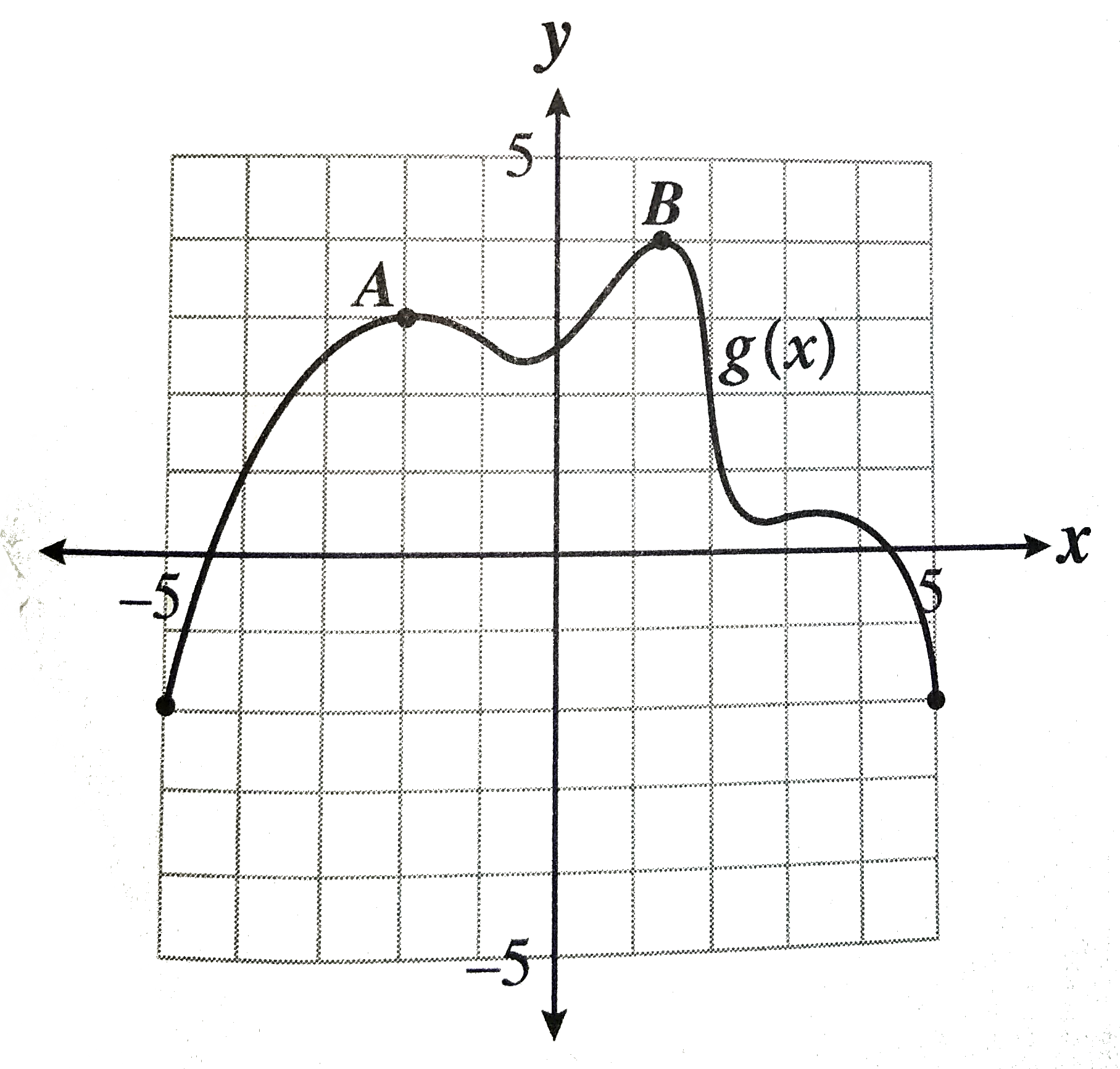
If the function shown in the graph is represented by f(x)=a(x−h)2+k, which of the following statements is NOT true ?
A
The value of a is negative.
B
f (x) is symmetrical across the line y=3.
C
The function g(x)=2x3 intersects f (x) at its vertex.
D
The value of h is positive.
Text Solution
The correct Answer is:B
Keep in mind that the question asks for the statement that's false, so eliminate the true choices as you go. The parabola opens downward, so the a term should be negetive. Eliminate (A). The parabola's vertex is in quadrant l, so h and k are both positive. That makes choice (D) true as well, eliminate it. The axis of symmetry is x=h,sox=3. According to the graph, the vertex is (3,2). Choice (B), however, claims that the axis of symmetry is y=3. Choice (B) is false and is therefore the correct answer.
For the record, (C ) is true statement because the line g(x)=2x3 passes through the point (3,2), which is indeed the vertex of f (x).
|
Topper's Solved these Questions
- QUADRATICSBOOK - KAPLANCHAPTER - QUADRATICSEXERCISE - SOLVING QUADRATICS BY FACTORING
1 Video
- QUADRATICSBOOK - KAPLANCHAPTER - QUADRATICSEXERCISE - CLASSIC QUADRATICS
1 Video
- QUADRATICSBOOK - KAPLANCHAPTER - QUADRATICSEXERCISE - SYSTEMS OF QUADRATIC AND LINEAR EQUATIONS
1 Video
- PAIRED PASSAGES AND PRIMARY SOURCE PASSAGES BOOK - KAPLANCHAPTER - PAIRED PASSAGES AND PRIMARY SOURCE PASSAGES EXERCISE - HOW MUCH HAVE YOU LEARNED
11 Videos
- SAT MATH: TIMING ANS SECTION MANAGEMENT STRATEGIESBOOK - KAPLANCHAPTER - SAT MATH: TIMING ANS SECTION MANAGEMENT STRATEGIESEXERCISE - TRY ON YOU OWN
5 Videos
Similar Questions
Knowledge Check
For function f(x)=lnxx, which of the following statements are true?
Af(x) has horizontal tangent at x = eBf(x) cuts the X-axis only at one pointCf(x) is many-one functionDf(x) has one vertical tangentFor the function f(x) = x2/3 , which of the following statement(s) is/are true ?
Adydx at the origin is non existentBequation of the tangent at the origin is x = 0Cf(x) has an extremum at x = 0Dorigin is the point of inflectionFor the function f(x)=cos−1x+cos−1x2 which of the following statements is/are true
Af(x) is always decreasingBf(x) is decreasing in [0,1] and increasing in [-1, 0]Cf(x) has only one local maximaDf(x) has only one local minima
Which of the following graphs represent the function of x ? Why
View SolutionLet f (x) be a polynomial function of degree 3 where a<b<candf(a)=f(b)=f(c). If the graph of f (x) is as shown, which of the following statements are INCORRECT ? (Where c>|a|)
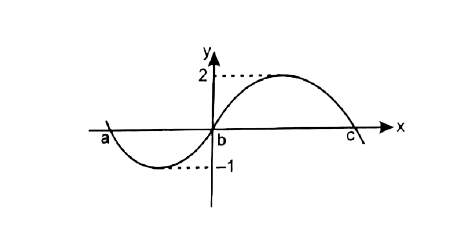
View SolutionWhat is the range of the function f(x)=|x|x,x≠0?
View SolutionLet f (x) be a polynomial function of degree 3 where a<b<candf(a)=f(b)=f(c). If the graph of f (x) is as shown, which of the following statements are INCORRECT ? (Where c>|a|)
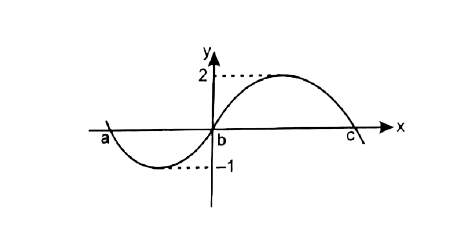
View SolutionIf f(x) and g(x) are odd functions of x, then which of the following is true?
View Solution