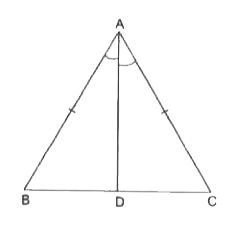
If the bisector of the vertical angle of a triangle bisects the base of the triangle. then the triangle is isosceles. GIVEN : A ABC in which AD is the bisector of ∠A meeting BC in D such that BD=DC.
Given:A△ABCin which AD is the bisector of ∠A meetingBC in D such that BD=CD
To Prove: △ACD is an isosceles triangle.
Construction: We produce AD to E such that AD=DE and join EC.
Proof: In △ADB and △EDC we have
AD=DE [By construction]
∠ADB=∠CDE[Vertically opposite angles] BD=DC [Given]
By SAS criterion of congruence, we get
△ADB≅△EDC
AB=EC.......(i) And,
∠BAD=∠CED [By cpctc]
But, ∠BAD=∠CAD
∠CAD=∠CED
AC=EC [Sides opposite to equal angles are equal]
AC=AB [By eg. (i)]
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
ΔABC ,angle B external angle C meets at P ∠A=80 then ∠BPC = ?
A50∘B160∘C100∘D130∘
JoinA→D
Hence,AD∥BC
∠ACB=∠CAD(alternateangle
∠BAC=∠ACD(alternateangle)
HenceAB=AC (by ASA congurency )so, it is isosceles triangle.View SolutionIf the bisector of the exterior vertical angle of a triangle be parallel to the base. Show that the triangle is isosceles.
View SolutionProve that the bisector of the vertical angle of an isosceles triangle bisects the base at right angles.
 View Solution
View SolutionShow that the bisector of vertical angle of an isosceles triangle bisects the base at right angles.
View SolutionIf the bisector of an angle of a triangle bisects the opposite side, prove that the triangle is isosceles.
View SolutionIf the altitude from one vertex of a triangle bisects the opposite side, then the triangle is isosceles. GIVEN : A ABC such that the altitude AD from A on the opposite side BC bisects BC i.e., BD=DC. TO PROVE : AB=AC i.e. the triangle ABC is isosceles.
View SolutionThe bisectors of the angles B and C of a triangle ABC , meet the opposite sides in D and E respectively. If DE||BC , prove that the triangle is isosceles.
View Solution

