D and E are, respectively, the points on equal sides AB and AC of an isosceles triangle ABC such that B, C, E, and D are concyclic as shown in Figure. If O is the point of intersection of CD and BE, prove that AO is the bisector of line segment DE
Since points B,C,DandE are Concyclic. Sum of opposite angles of cyclic quadrilateral is 180∘.
So, ∠1+∠3=180°
and, ∠22+∠24=180°
Also, AABC is an Isosceles Triangle.
AB=AC(Given)
∠3=∠4→when opposite sides are equal angle opposite to them are equal.
Gives, ∠1+∠4=180∘
∠2+∠3=180∘
But these are interior angles on the same side of transversal B D and EC. So, if sum of interior angles on the same side of transversal is 180∘, then lines are parallel.
DE || BC
∠4=∠5'<br>'∠3=∠6
when lines are parallel, corresponding angles are equal.
But, ∠3=∠4
Which gives, ∠5=∠6
AD=A E→If opposite angles in a triangle are equal, side opposite to them are equal.
Draw, AM⊥DE
In ΔAMD and ΔΑΜΕ
AD=AE→Proved above
∠AMD=∠AME→each being 90∘.
AM is common.
△AMD = △AME→[R HS congruency rule]
→DM ME→[CPCT]
Join ,AO,which will pass through M, as ∠AME +∠OME=180∘, showing points A,M and O are collinear
Which shows, Segment AO is the bisector of line segment DE because, DM = ME.
Topper's Solved these Questions
Similar Questions
DandE are points on equal sides ABandAC of an isosceles triangle ABC such that AD=AE . Prove that B,C,D,E are concylic.
View SolutionD and E are points on equal sides AB and AC of an isosceles triangle ABC such that AD=AE. Prove that B, C, D, E are concyclic.
View SolutionABC and DBC are two isosceles triangles on the same bas BC and vertices A and D are on the same side of BC . If AD is extended to intersect BC at P, show that ABD ≅ACD (ii) ABP ≅ACP
View SolutionABC and DBC are two isosceles triangles on the same bas BC and vertices A and D are on the same side of BC . If AD is extended to intersect BC at P, show that AP bisects \ ∠A as well as ∠D AP is the perpendicular bisector of BC
View SolutionIn ABC, D, E and F are, respectively, the mid-points of BC, CA and AB . If the lengths of side AB, BC and CA are 7cm, 8cm, and 9cm, respectively, find the perimeter of DEF
View SolutionIn Figure, AD and BE are medians of ABC and BE∣∣DF . Prove that CF=14AC
Consider △ABC Since AD is Median
⇒BD=BC⋯(1)
Since BE is Median⇒AE=EC⋯(2)
Consider △BECSince BE∣∣DF
By Basic Proportionality theorm, ⇒BDDC=EFFC
From (1)⇒CF=EF
⇒CF=12AE
From (2)⇒CF=14ACView SolutionIn a ABC,EandF are the mid-points of ACandAB respectively. The altitude AP to BC intersects FE at Q Prove that AQ=QP
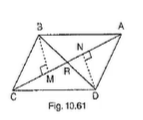
View SolutionIn Figure, BM and DN are both perpendiculars to the segments AC and BM=DN . Prove that AC bisects BD
 View Solution
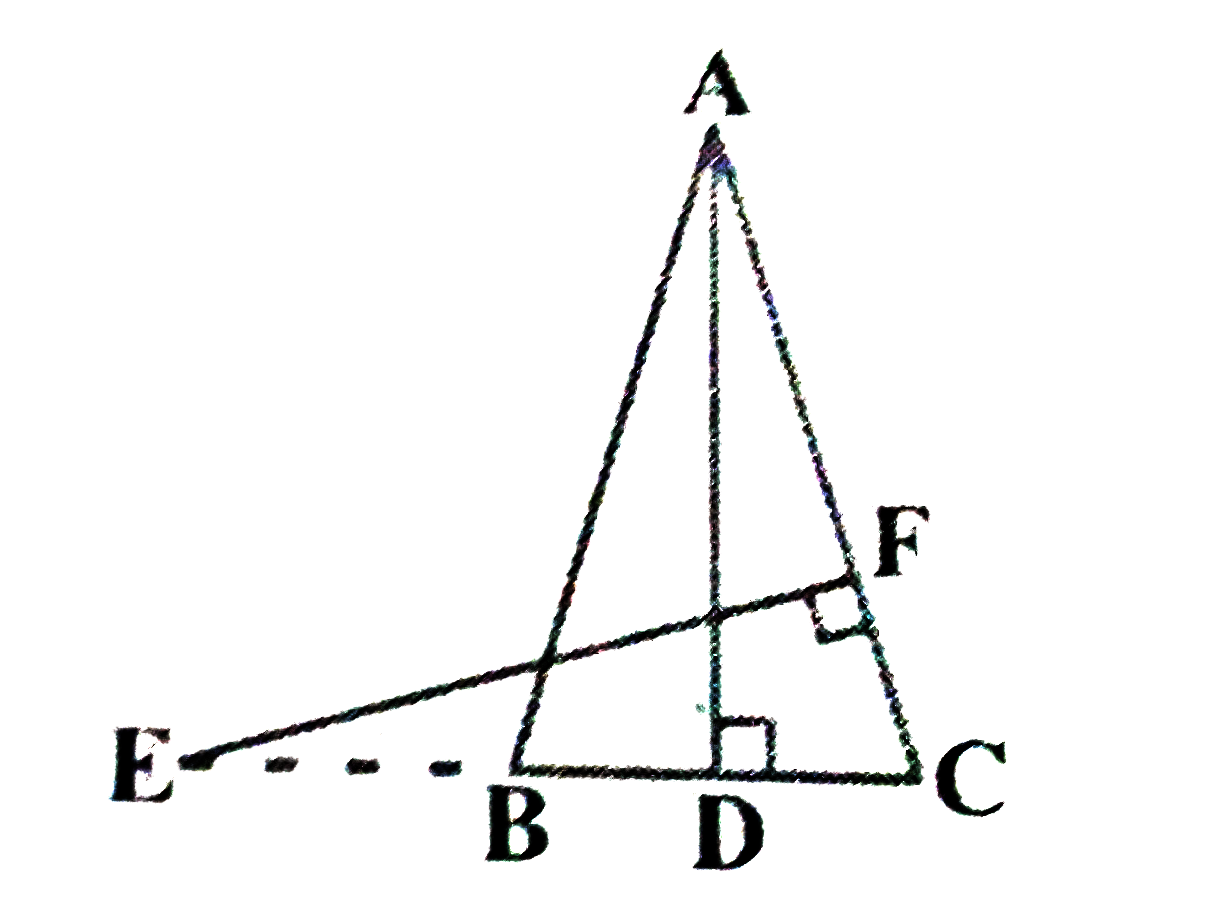
View SolutionIn figure E is a point on side CB produced of an isosceles triangle ABC with AB = AC. If AD⊥BCandEF⊥AC, prove thatΔABD~ΔECF.
 View Solution
View SolutionLet ABC be an isosceles triangle with AB=AC and let D, E, F be the mid points of BC, CA and AB respectively. Show that AD⊥FE and AD is bisected by FE
View Solution

