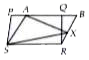
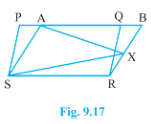
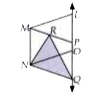
In the adjoining figure, MNPQ and ABPQ are parallelogram and T is any point on the side BP. Prove that
(i) $a r (M N P Q) = a r (A B P Q)$
(ii) $a r (△ A T Q) = \frac{1}{2} a r (M N P Q)$ .

Video Solution

Text Solution

Verified by Experts

$a r (M N P Q) = a r (A B P Q)$ ( they have same base and lie between same parallels).
$a r (△ A T Q) = \frac{1}{2} a r (A B P Q)$ (they have same base and lie between same parallels).

Updated on:21/07/2023

Class 9 MATHS AREA OF PARALLELOGRAMS AND TRIANGLES

Topper's Solved these Questions

AREAS OF PARALLELOGRAMS AND TRIANGLES BOOK - RS AGGARWALCHAPTER - AREAS OF PARALLELOGRAMS AND TRIANGLES EXERCISE - MULTIPLE-CHOICE QUESTIONS (MCQ)
32 Videos
AREAS OF PARALLELOGRAMS AND TRIANGLES BOOK - RS AGGARWALCHAPTER - AREAS OF PARALLELOGRAMS AND TRIANGLES EXERCISE - ASSERTION & REASON TYPE
5 Videos
AREAS OF PARALLELOGRAMS AND TRIANGLES BOOK - RS AGGARWALCHAPTER - AREAS OF PARALLELOGRAMS AND TRIANGLES EXERCISE - ASSERTION & REASON TYPE
5 Videos
AREAS OF TRIANGLES AND QUADRILATERALSBOOK - RS AGGARWALCHAPTER - AREAS OF TRIANGLES AND QUADRILATERALSEXERCISE - Multiple Choice Questions (Mcq)
16 Videos

Knowledge Check

In the given figure, ABCD and AEFD a re two parallelograms.

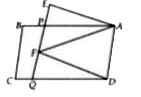
ar( $Δ$ PEA) =
Aar( $∣ ∣^{g m} Δ$ PEA)
Bar( $Δ$ PFD)
Car( $Δ$ QFD)
Dar( $∣ ∣^{g m} Δ$ CQPB)
In the figure, ABCD and EFGD are two parallelograms and G is the mid-point of CD. Then, $a r (Δ D P C) = a r (E F G D)$ .

ATrue
BFalse
CPartially true
DNone of these

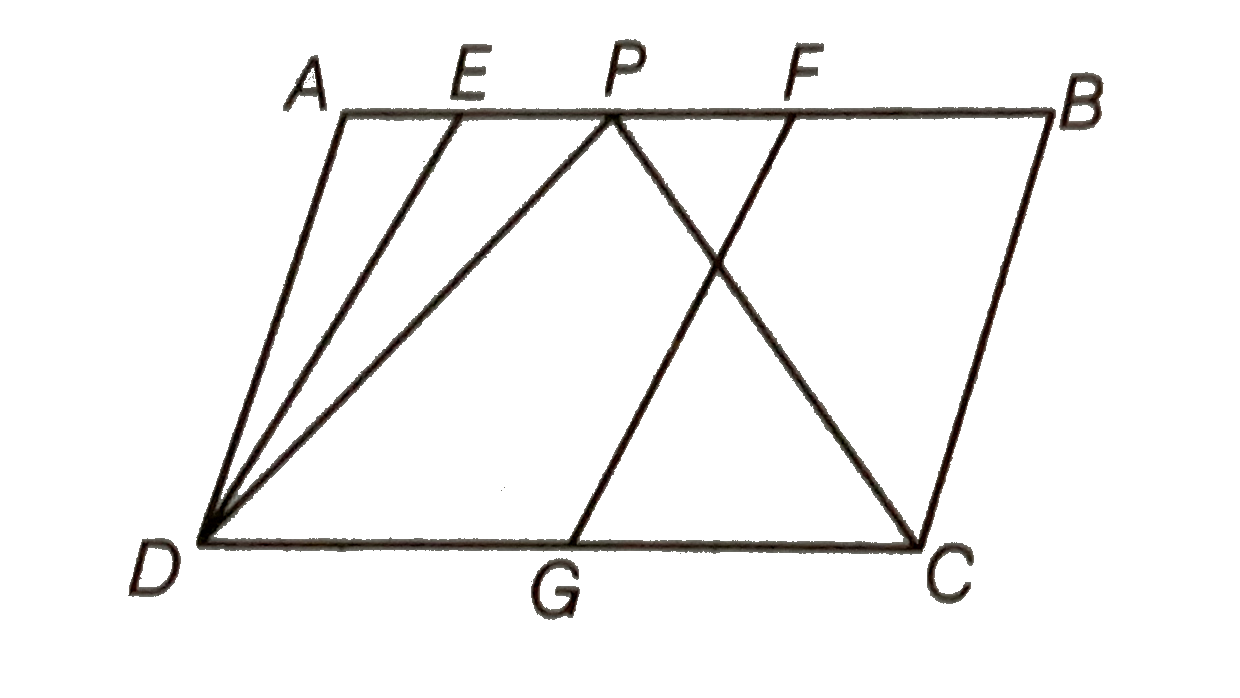
In the adjoining figure, ABCD is a parallelogram and P is any points on BC. Prove that
$a r (△ A B P) + a r (△ D P C) = a r (△ P D A)$ .

View Solution
In the adjoining figure, ABCD is a parallelogram. Points P and Q on BC trisect BC. Prove that
$a r (△ A P Q) = a r (△ D P Q) = \frac{1}{6} a r (△ A B C D)$ .

View Solution
In the given figure, ABCD is a parallelogram. E and F are any two points on AB and BC, respectively. Prove that $a r (△ A D F) = a r (△ D C E)$ .
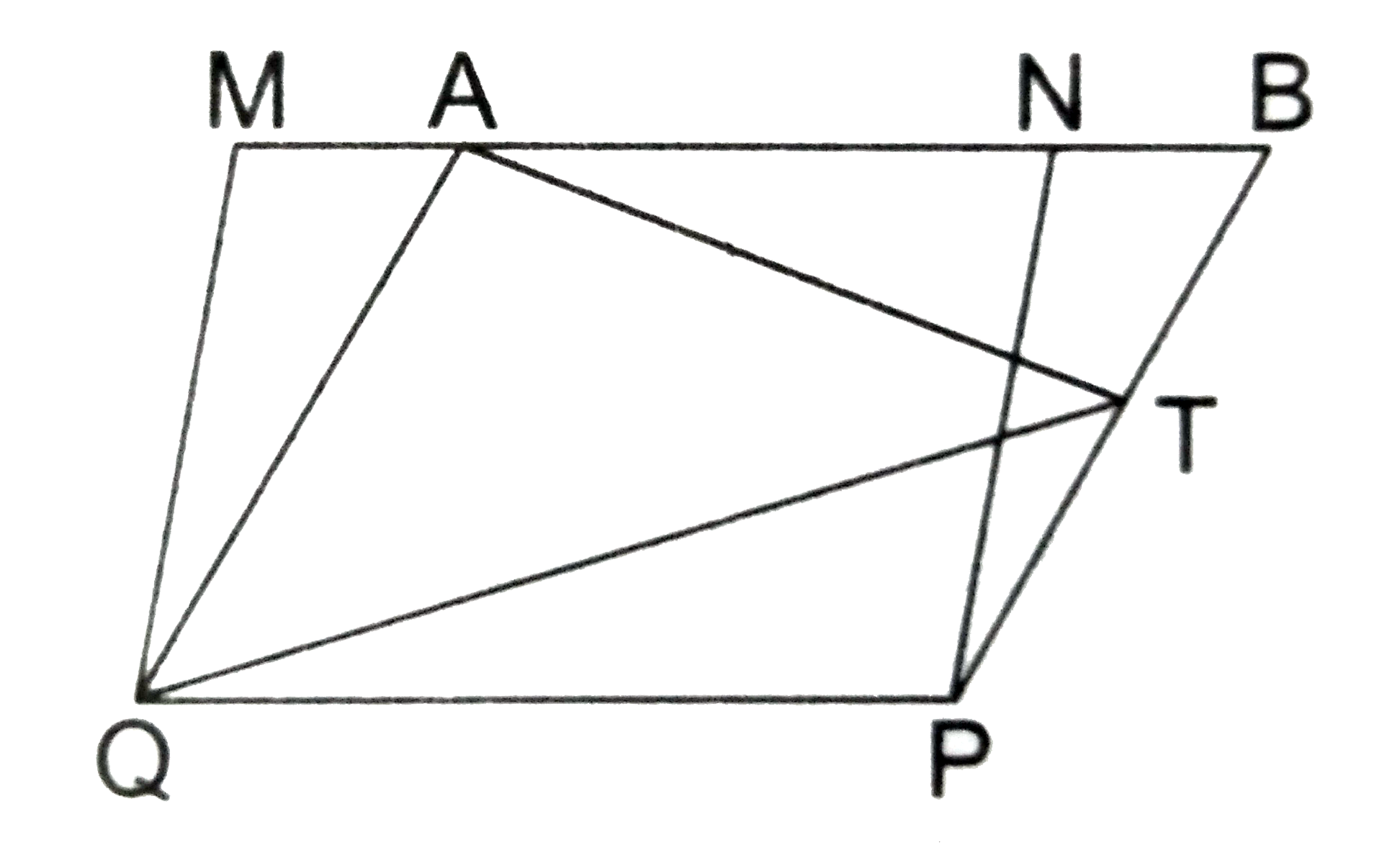
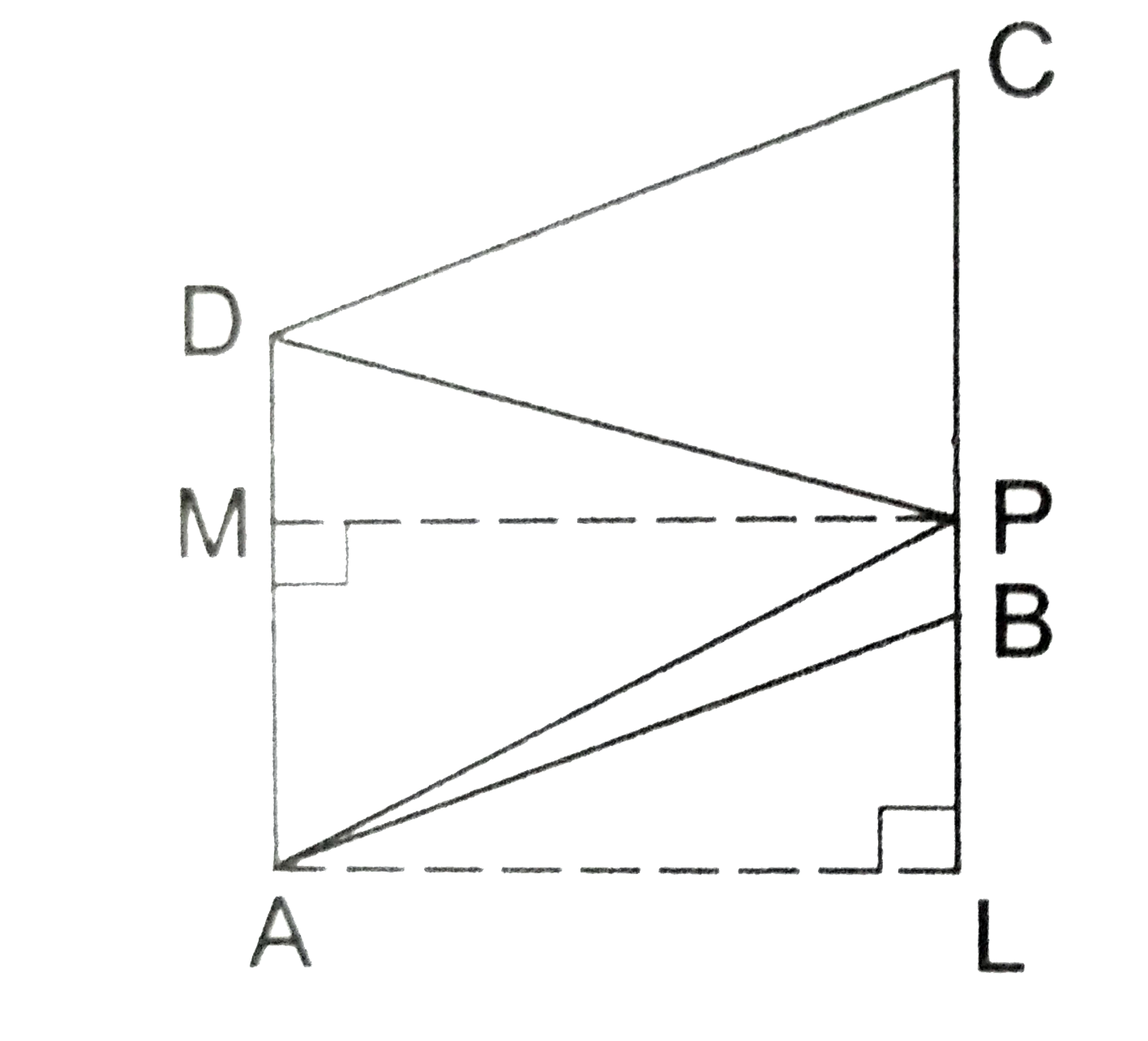
View Solution
In the given figure LMNO and PMNQ are two parallelograms. R is any point on side MP. If ar( $Δ$ NRQ) = k[ar( $I I^{g m}$ LMNO)] then 2k equals

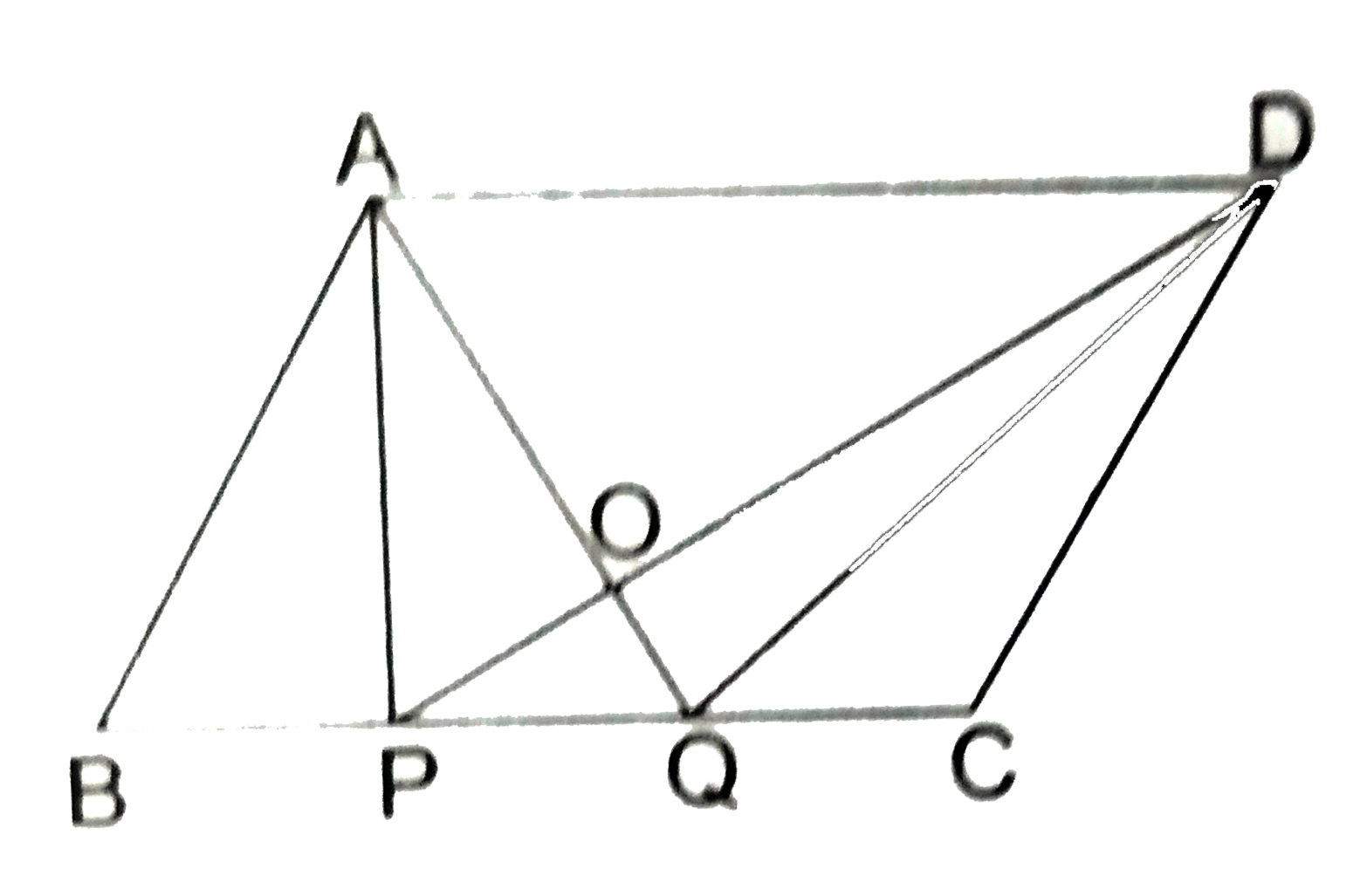
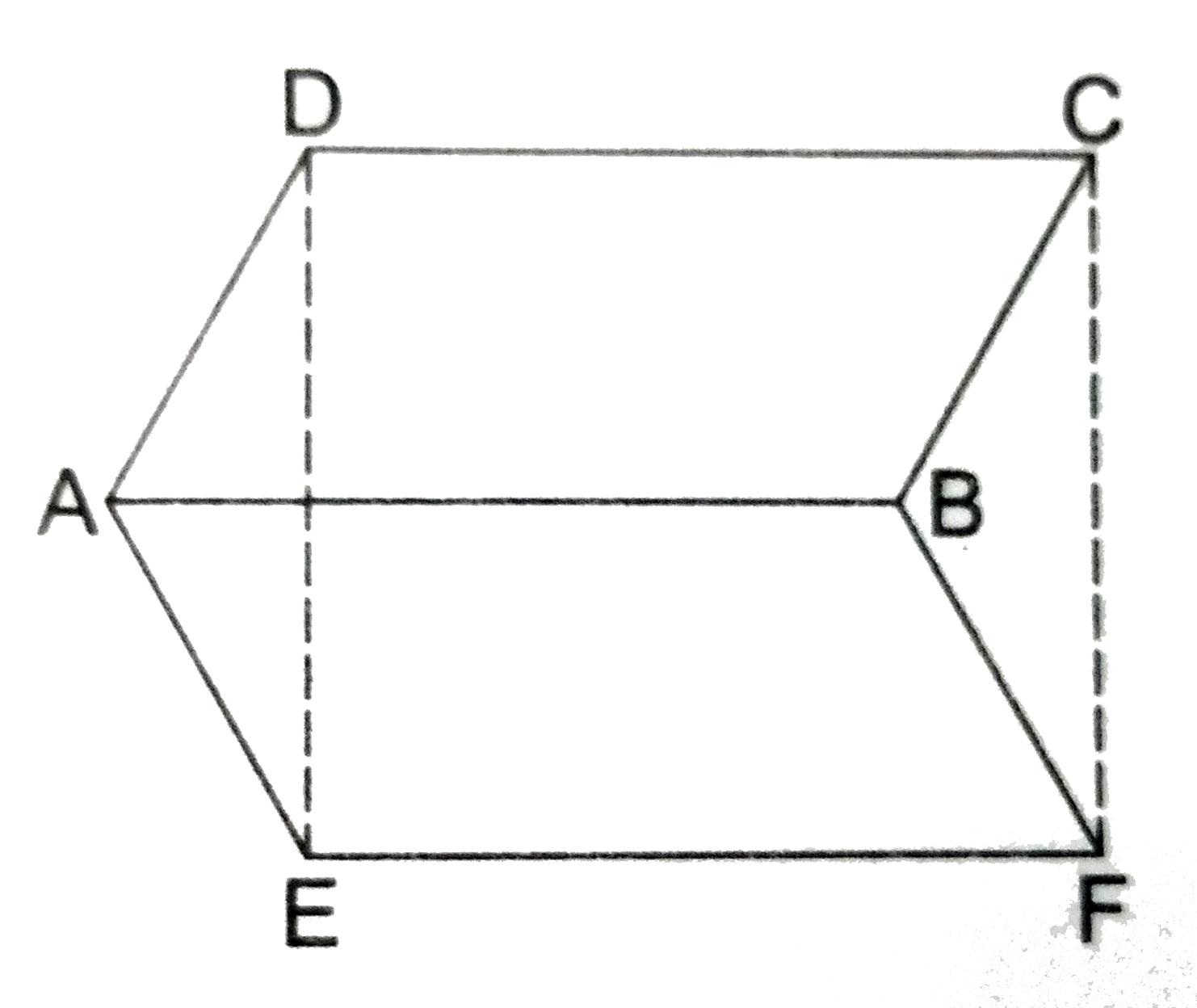
View Solution
In the adjoing figure, two parallelograms ABCD and AEFB are drawn on opposite sides of AB. Prove that
$a r (||gm ABCD) + a r (||gm AEFB)$
$= a r (||gm EFCD)$ .

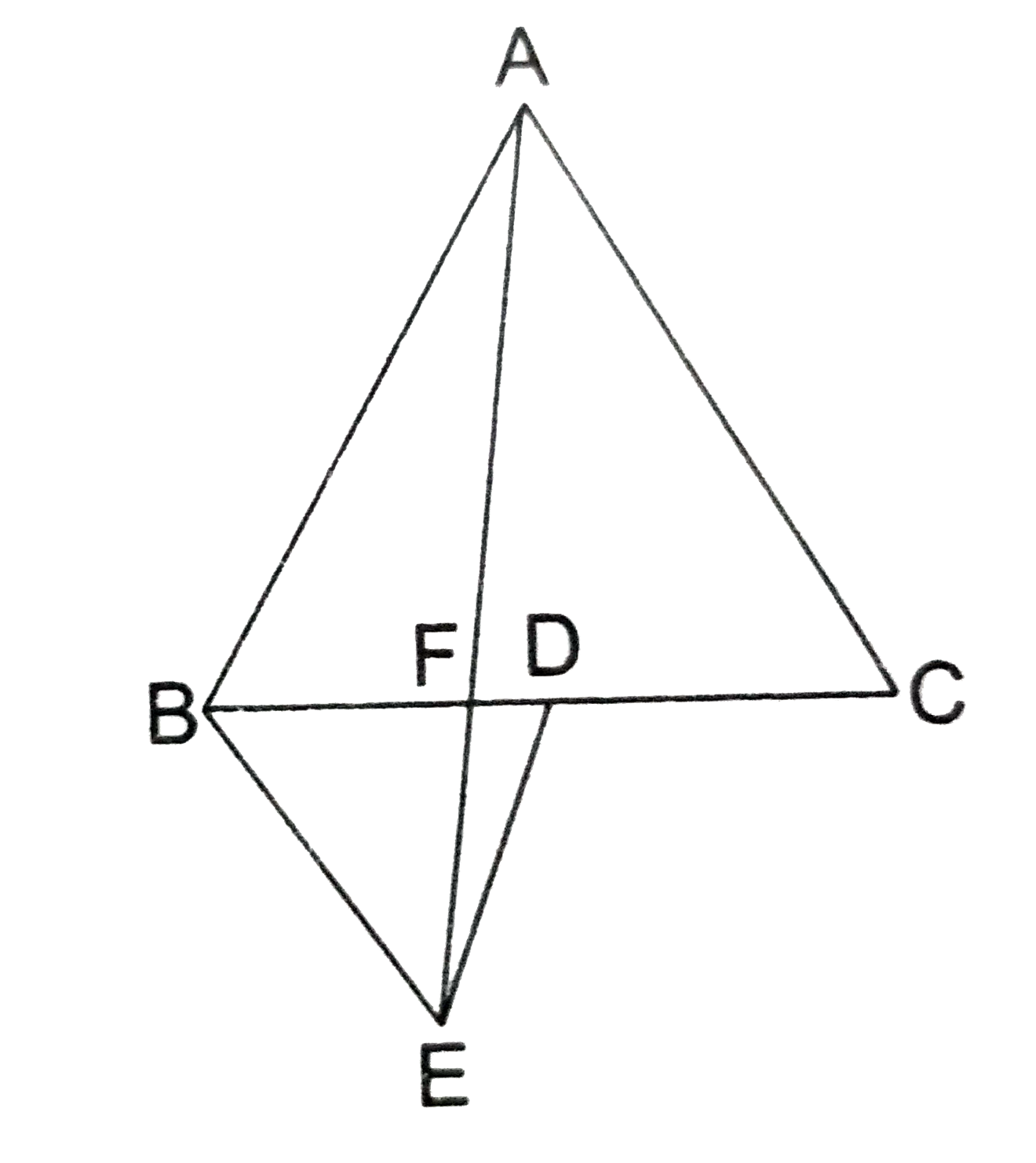
View Solution
In the adjoining figure, ABC and BDE are two equilateral triangle such that D is the midpoint of BC. If AE intersects BC at F, show that
$(i) a r (△ B D E) = \frac{1}{4} a r (△ A B C)$
$(i i) a r (△ B D E) = \frac{1}{2} a r (△ B A E)$
$(i i i) a r (△ A B C) = 2 a r (△ B E C)$
$(i v) a r (△ B F E) = a r (△ A F D)$
$(v) a r (△ B F E) = 2 a r (△ F E D)$
$(v i) a r (△ F E D) = \frac{1}{8} a r (△ A F C)$ .

View Solution

RS AGGARWAL-AREAS OF PARALLELOGRAMS AND TRIANGLES -Exercise 11

In the adjoining figure, ABCD is a quadrilateral in which diag. BD = 1...
01:42
P and Q are any two points lying on the sides DC and AD respectively ...
03:46
In the adjoining figure, MNPQ and ABPQ are parallelogram and T is any ...
03:13
Assertion (A) : In a trapezium ABCD, we have AB ||DC and the diagonals...
02:05
In figure, if DEabs()BC, then find the ratio of ar (DeltaADE) and ar(D...
04:52
Show that a median of a triangle divides it into two triangles of e...
03:04
A diagonal of a parallelogram divides it into two triangles of equal a...
02:41
In Fig. 9.24, ABC and ABD are two triangles on the same base AB. If l...
04:46
The points D and E are respectively located on AB and AC on the sides ...
03:56
O is the any point on diagonal PR of parallelogram PQRS. Prove that Ar...
05:53
In the adjoining figure, the diagonals AC and BD of a quadrilateral AB...
02:42
The vertex A of triangle ABC is joined to a point D on the side BC. Th...
03:48
D is the midpoint of side BC of triangleABC and E is the midpoint of ...
03:44
In trapezium ABCD, AB || DC and L is the mid-point of BC. Through L, a...
05:13
In the adjoining figure, ABCD is a quadrilateral. A line through D, p...
02:23
In the adjoining figure, triangle ABC and triangleDBC are on the same ...
03:24
ABCD is a parallelogram in which BC is produced to E such that CE = BC...
06:01
A point E is taken on the side BC of a parallelogram ABCD. AE and DC a...
05:45
P, Q, R, S are respectively the midpoints of the sides AB, BC, CD and ...
10:21
किसी त्रिभुज ABC की मधिकाएँ CD और BE एक दूसरे को O पर कटती है , तो अन...
02:44

Home
Ask Doubt
Profile

In the adjoining figure, MNPQ and ABPQ are parallelogram and T is any point on the side BP. Prove that (i) ar(MNPQ)=ar(ABPQ) (ii)ar(△ATQ)=12ar(MNPQ).

ar(MNPQ)=ar(ABPQ) ( they have same base and lie between same parallels). ar(△ATQ)=12ar(ABPQ) (they have same base and lie between same parallels).

Topper's Solved these Questions

Similar Questions

Knowledge Check

In the given figure, ABCD and AEFD a re two parallelograms. ar(ΔPEA) =

In the figure, ABCD and EFGD are two parallelograms and G is the mid-point of CD. Then, ar(ΔDPC)=ar(EFGD).

RS AGGARWAL-AREAS OF PARALLELOGRAMS AND TRIANGLES -Exercise 11

In the adjoining figure, MNPQ and ABPQ are parallelogram and T is any point on the side BP. Prove that
(i) $a r (M N P Q) = a r (A B P Q)$
(ii) $a r (△ A T Q) = \frac{1}{2} a r (M N P Q)$ .

$a r (M N P Q) = a r (A B P Q)$ ( they have same base and lie between same parallels).
$a r (△ A T Q) = \frac{1}{2} a r (A B P Q)$ (they have same base and lie between same parallels).

In the given figure, ABCD and AEFD a re two parallelograms.

ar( $Δ$ PEA) =

In the figure, ABCD and EFGD are two parallelograms and G is the mid-point of CD. Then, $a r (Δ D P C) = a r (E F G D)$ .