The interior bisector of ∠B and exterior bisector of ∠C of ΔABC meet at D. Through D, a line parallel to CB meets AC at L and AB at M. If the measures of legs LC and MB of trapezoid CLMB are 5 cm and 7 cm respectively. Find the measure of base 'LM'
3 cm
2 cm
32cm
5 cm
Step by step video & image solution for The interior bisector of angleB and exterior bisector of angleC of DeltaABC meet at D. Through D, a line parallel to CB meets AC at L and AB at M. If the measures of legs LC and MB of trapezoid CLMB are 5 cm and 7 cm respectively. Find the measure of base 'LM' by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
AD is perpendicular to the internal bisector of ∠ABC of triangle ABC. DE is drawn through D and parallel to BC to meet AC at E. If the length of AC is 12cm. Then the length of AE (in cm) is.
A3B8C4D6The external bisector of ∠Band∠C of ΔABC(where AB and AC extended to E and F respectively) meet at point P. If ∠BAC=100∘, then the measure of ∠BPC is
A50∘B80∘C40∘D100∘The side BC of ΔABC is produced to D. The bisectors of ∠ABCand∠ABD meet at E. If AB = AC and ∠BEC=24∘. then the measure of ∠ABC is :
AA)60∘BB)72∘CC)48∘DD)66∘
In ΔABC, the bisector of ∠A interseats BC at D. A perpendicular to AD from B intersects AD at E. A line segment through E and parallel to AC intersects BC at G and AB at H. If AB = 26, BC = 28, AC = 30. Find the measure of DG.
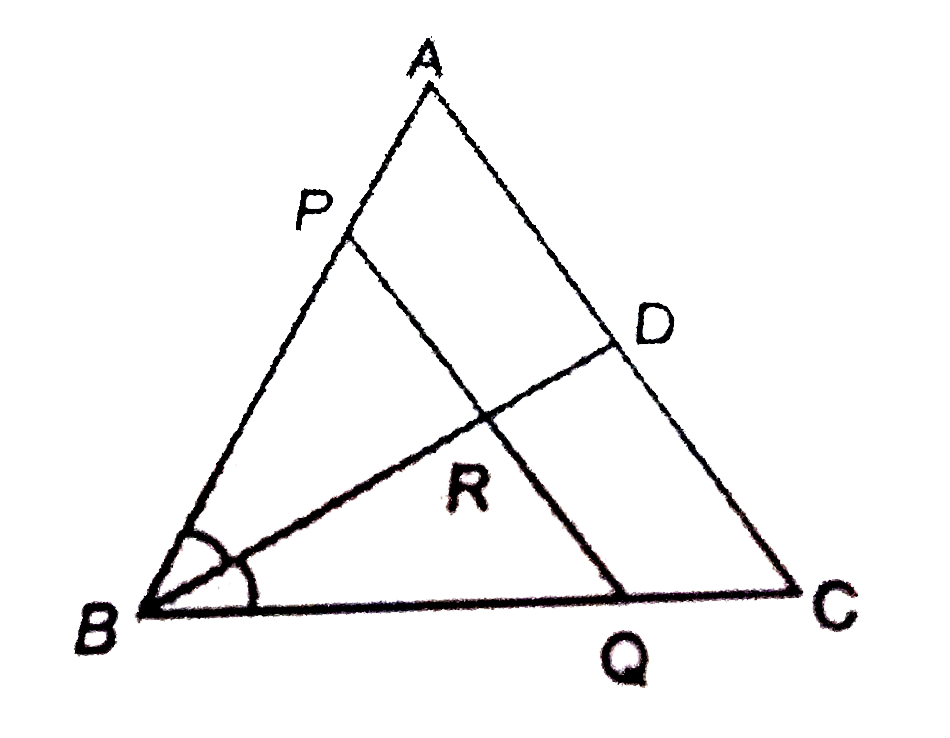
View SolutionIn △ABCthe bisector of ∠B meets AC at D. A line PQ||AC meets AB,BC and BD at P, Q and R respectively.
show that PR×BQ=QR×BP.
View SolutionInternal bisector of ∠A of triangle ABC meets side BC at D. A line drawn through D perpendicular to AD intersects the side AC at E and the side AB at F. If a, b, c represent sides of ΔABC, then
View SolutionIn ΔABC, the bisector of ∠B meets AC at D. A line PQ∣∣AC meets AB,BC and BD at P,Q and R respectively. Show that BP×QR=BQ×PR.
View SolutionIn ΔABC,AB=AC and the bisectors of ∠B and ∠C meet AC and AB at point D and E respectively. Prove that BD=CE.
View Solution
