A point charge q is situated at a distance r from one end of a thin conduction rod of length L having a charge Q (uniformly distributed a long its length).find the magnitudes of electric force between the two.
Kqqr2
2KQr(r+L)
KQqr(r−L)
KQqr(r+L)
The correct Answer is:D
To find the electric force between a point charge q situated at a distance r from one end of a thin conducting rod of length L with a uniformly distributed charge Q, we can follow these steps:
Step 1: Define Charge Density
The charge per unit length λ of the rod is given by:
λ=QL
Step 2: Consider an Element of the Rod
Take an infinitesimal element of the rod at a distance x from the point charge q with thickness dx. The charge dq on this element is:
dq=λdx=QLdx
Step 3: Calculate the Electric Field Due to dq
The electric field dE at the location of charge q due to the charge dq is given by Coulomb's law:
dE=kdqx2=kQLdxx2
where k is Coulomb's constant.
Step 4: Set Up the Integral for the Total Electric Field
The total electric field E at the location of charge q due to the entire rod can be found by integrating dE from x=r to x=r+L:
E=∫r+LrdE=∫r+LrkQLdxx2
Step 5: Perform the Integration
The integral can be simplified as follows:
E=kQL∫r+Lrdxx2
The integral ∫dxx2 evaluates to −1x:
E=kQL(−1x)r+Lr=kQL(−1r+L+1r)
E=kQL(1r−1r+L)
Step 6: Calculate the Force on Charge q
The force F on the charge q due to the electric field E is given by:
F=qE
Substituting the expression for E:
F=q⋅kQL(1r−1r+L)
Step 7: Simplify the Expression
Now, simplifying the expression for force:
F=kqQL((r+L)−rr(r+L))=kqQLLr(r+L)
Thus, the final expression for the electric force F is:
F=kqQr(r+L)
Final Result
The magnitude of the electric force between the point charge q and the conducting rod with charge Q is:
F=kqQr(r+L)
The charge per unit length λ of the rod is given by:
λ=QL
Take an infinitesimal element of the rod at a distance x from the point charge q with thickness dx. The charge dq on this element is:
dq=λdx=QLdx
The electric field dE at the location of charge q due to the charge dq is given by Coulomb's law:
dE=kdqx2=kQLdxx2
where k is Coulomb's constant.
The total electric field E at the location of charge q due to the entire rod can be found by integrating dE from x=r to x=r+L:
E=∫r+LrdE=∫r+LrkQLdxx2
The integral can be simplified as follows:
E=kQL∫r+Lrdxx2
The integral ∫dxx2 evaluates to −1x:
E=kQL(−1x)r+Lr=kQL(−1r+L+1r)
E=kQL(1r−1r+L)
The force F on the charge q due to the electric field E is given by:
F=qE
Substituting the expression for E:
F=q⋅kQL(1r−1r+L)
Now, simplifying the expression for force:
F=kqQL((r+L)−rr(r+L))=kqQLLr(r+L)
Thus, the final expression for the electric force F is:
F=kqQr(r+L)
The magnitude of the electric force between the point charge q and the conducting rod with charge Q is:
F=kqQr(r+L)
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Match the columns
5 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
A thin straight rod of length l carrying a uniformly distributed charge q is located in vacuum. Find the magnitude of the electric force on a point charge 'Q' kept as shown in the figure.

View SolutionA 10 cm long rod carries a charge of +150μC distributed uniformly along its length. Find the magnitude of the electric field at a point 10 cm from both the ends of the rod.
View SolutionA point charge q is located at the centre fo a thin ring of radius R with uniformly distributed charge −q, find the magnitude of the electric field strength vectro at the point lying on the axis of the ring at a distance x from its centre, if x>>R.
View SolutionA rod has a total charge Q uniformly distributed along its length L. If the rod rotates with angular velocity ω about its end, compute its magnetic moment.

View SolutionA rod has a total charge Q uniformly distributed along its length L. If the rod rotates with angular velocity ω about its end, compute its magnetic moment.

View SolutionA particle of mass m is situated at a distance d from one end of a rod of mass M and length L as shown in diagram. Find the magnitude of the gravitational force between them
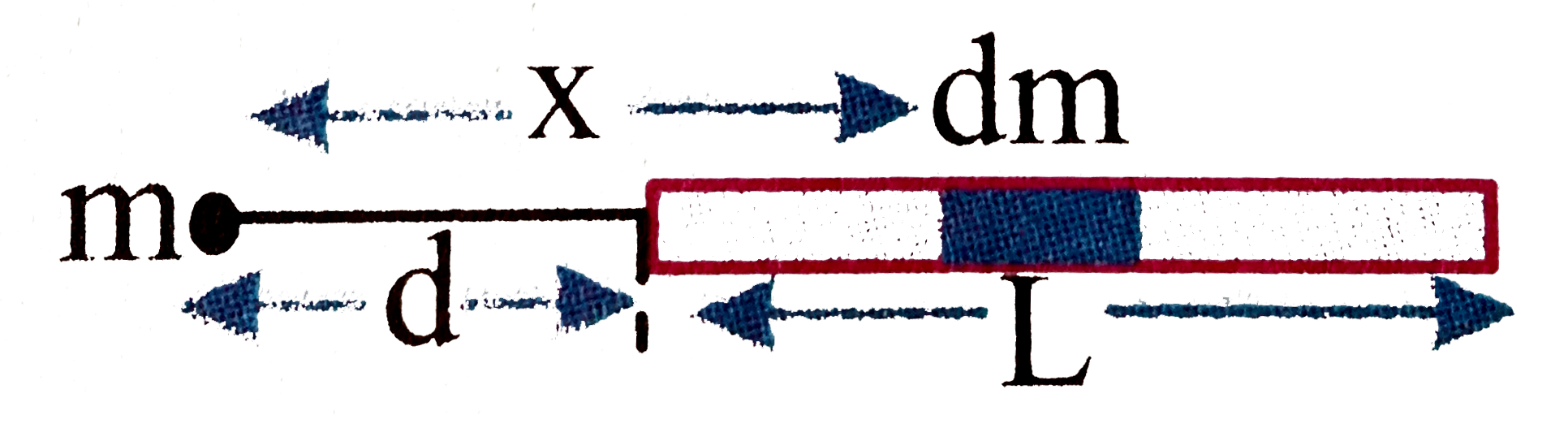
View SolutionA wire of length l and charge q is bent in form of a semicircle. The charge is uniformly distributed over the length. The electric field at the centre of semicircle is
View SolutionCharge q is uniformly distributed over a thin half ring of radius R. The electric field at the centre of the ring is
View SolutionPotential at a point x-distance from the centre inside the conducting sphere of radius R and charged with charge Q is
View SolutionFigure shows a uniformly charged sphere of radius R and total charge Q. A point charge q is situated outside the sphere at a distance r from centre of sphere. Find out the following :
(i) Force acting on the point charge q due to the sphere.
(ii) Force acting on the sphere due to the point charge.

View Solution
