Electric potential at a point P, r distance away due to a point charge q kept at point A is V. If twice of this charge is distributed uniformly on the surface of a hollow sphere of radius 4r with centre at point A the potential at P now is
V
V/2
V/4
V/8
The correct Answer is:B
To solve the problem, we need to determine the electric potential at point P due to a hollow sphere with a uniformly distributed charge. Here’s a step-by-step breakdown of the solution:
Step 1: Understand the Initial Situation
Initially, we have a point charge q located at point A. The electric potential V at point P, which is at a distance r from charge q, is given by the formula:
V=kqr
where k is Coulomb's constant.
Step 2: Determine the New Charge Distribution
The problem states that twice the initial charge q (i.e., 2q) is now uniformly distributed over the surface of a hollow sphere with a radius of 4r centered at point A.
Step 3: Calculate the Potential Due to the Hollow Sphere
For a hollow sphere with a uniform charge distribution, the electric potential at any point outside the sphere (or on its surface) is given by:
V′=kQR
where Q is the total charge and R is the distance from the center of the sphere to the point of interest.
In this case, since point P is at a distance r from point A and the radius of the sphere is 4r, point P is inside the hollow sphere.
Step 4: Use the Formula for Potential Inside a Hollow Sphere
For any point inside a hollow charged sphere, the potential is constant and equal to the potential on the surface of the sphere. Thus, we need to calculate the potential at the surface of the sphere:
V′=k2q4r
Simplifying this gives:
V′=kq2r
Step 5: Relate the New Potential to the Original Potential
From the original potential V=kqr, we can express the new potential V′ in terms of V:
V′=12(kqr)=V2
Conclusion
Thus, the potential at point P due to the hollow sphere is:
V′=V2
Final Answer
The potential at point P now is V2.
---
Initially, we have a point charge q located at point A. The electric potential V at point P, which is at a distance r from charge q, is given by the formula:
V=kqr
where k is Coulomb's constant.
The problem states that twice the initial charge q (i.e., 2q) is now uniformly distributed over the surface of a hollow sphere with a radius of 4r centered at point A.
For a hollow sphere with a uniform charge distribution, the electric potential at any point outside the sphere (or on its surface) is given by:
V′=kQR
where Q is the total charge and R is the distance from the center of the sphere to the point of interest.
For any point inside a hollow charged sphere, the potential is constant and equal to the potential on the surface of the sphere. Thus, we need to calculate the potential at the surface of the sphere:
V′=k2q4r
Simplifying this gives:
V′=kq2r
From the original potential V=kqr, we can express the new potential V′ in terms of V:
V′=12(kqr)=V2
Thus, the potential at point P due to the hollow sphere is:
V′=V2
The potential at point P now is V2.
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Subjective
15 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - SUBJECTIVE_TYPE
6 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Assertion And Reason
19 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
Calculate the self potential energy of charge q distributed over the surface of a hollow sphere of radius R. What happens to this self energy when R=0, or when the charge is an ideal point charge?
View SolutionShow that electric potential at a point P, at a distance .r. from a fixed point charge Q, is given by :
V=(14πε0)Qr
View Solution
Knowledge Check
Metallic sphere of radius R is charged to potential V. Then charge q is proportional to
AVBRCboth V and RDnone of theseElectric field intensity at a point B due to a point charge Q kept at point A is 24NC−1, and electric potential at B due to the same charge is 12JC−1. Calculate the distance AB and magnitude of charge.
A10−6CB10−7CC10−10CD10−9C
Potential at a point x-distance from the centre inside the conducting sphere of radius R and charged with charge Q is
View SolutionA positive point charge q is placed at a distance 2 R from the surface of a metallic shell of radius R. the electric field at centre of shell due to indouced charge has magnitude
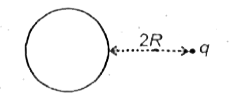
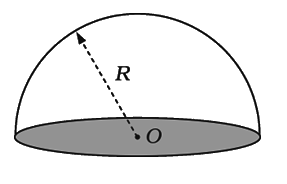
View SolutionCharge Q is uniformly distributed throughout the volume of a solid hemisphere of radius R metres. Then the potential at centre O of hemisphere in volts is:
View SolutionObtain an expression for electric potential .V due to a point charge .Q at a distance r.
View SolutionFind potential at a point 'P' at a distance 'x' on the axis away from centre of a uniform ring of mass M and radius R .
View SolutionAssertion: Electric potential on the surface of a charged sphere of radius R is V. Then electric field at distance r=R2 from centre is V2R. Charge is distributed uniformly over the volume.
Reason: From centre to surface, electric field varies linearly with r. Here r is distance from centre.
View Solution
