Complete Solutions to Solved Examples of chapter GRAVITATION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | GRAVITATION |
| DC PANDEY | Solved Examples |
| Number Of Questions | 16 |
| Academic Year | 2023-24 |
Three particles, each of the mass `m` are situated at the vertices of an equilateral triangle of side `a`. The only forces acting on the particles are their mutual gravitational forces. It is desired that each particle moves in a circle while maintaining the original mutual separation `a`. Find the initial velocity that should be given to each particle and also the time period of the circular motion. `(F=(Gm_(1)m_(2))/(r^(2)))`
View SolutionIn the above problem, find total mechanical energy of the system .
View SolutionTwo concentric shells of masses `M_(1)` and `M_(2)` are concentric as shown. Calculate the gravitational force on m due to `M_(1)` at points P,Q and `R`.

View SolutionGravitational potential energy between two points masses is
`U = -(Km_(1)m_(2))/(r^(n))`
where, K is a positive constant. With what power of 'r' time period of a satellite of mas 'm' varies in circular orbit if mass of planet is `M` ?
View SolutionImagine a light planet revolving around a very massive star in a circular orbit of radius R with a period of revolution T. if the gravitational force of attraction between the planet and the star is proportational to `R^(-5//2)`, then
(a) `T^(2)` is proportional to `R^(2)`
(b) `T^(2)` is proportional to `R^(7//2)`
(c) `T^(2)` is proportional to `R^(3//3)`
(d) `T^(2)` is proportional to `R^(3.75)`.
View SolutionThere is a smooth tunnel upto centre C of a solid sphere of mass 'M' and radius R.A particle of mass `m(ltltM)` is released from point P along the line CP. Find velocity of 'm' while striking at `C`.
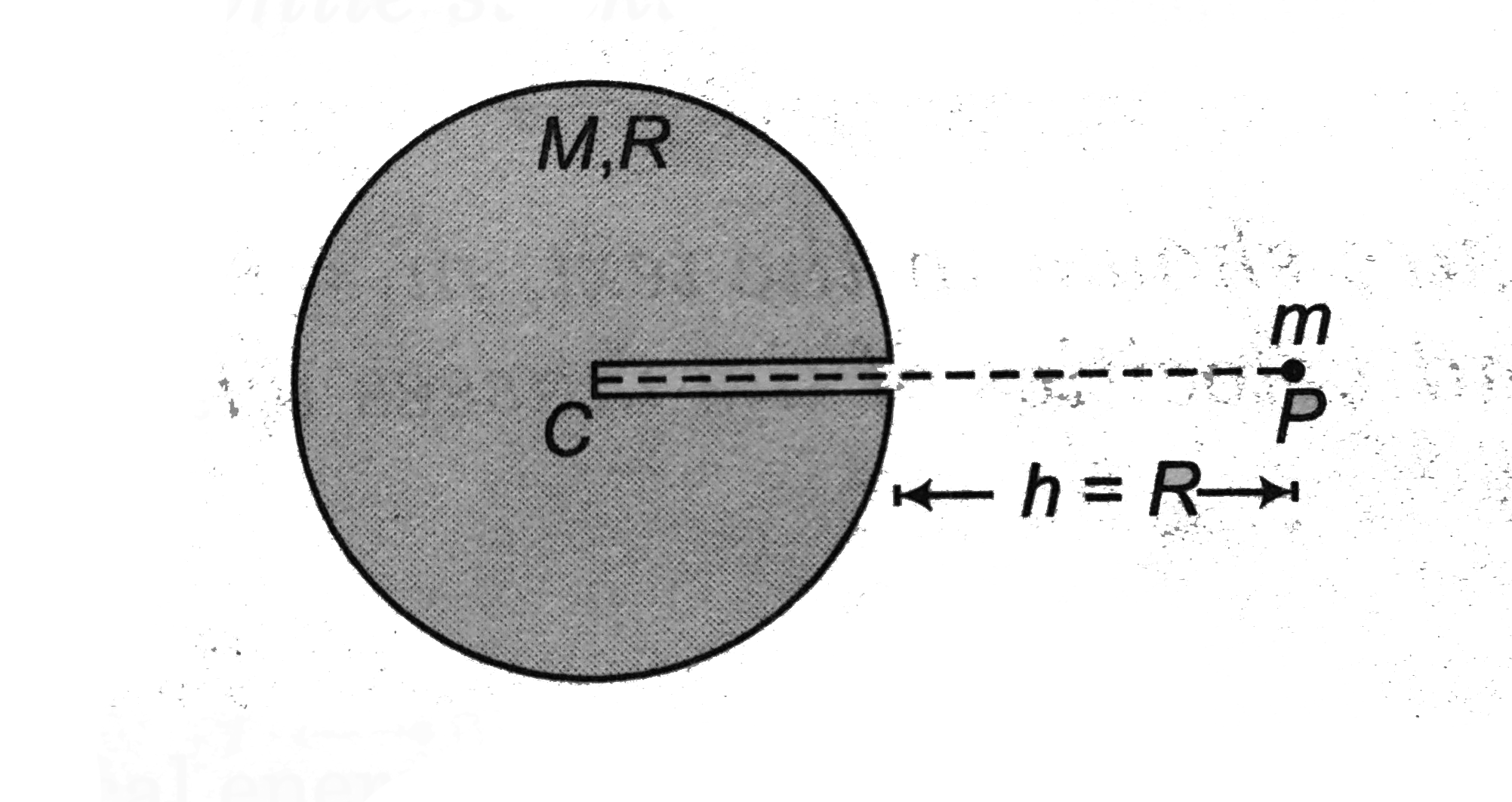 .
.
View SolutionA particle of mass 'm' is projected from the surface of earth with velocity`upsilon =2upsilon_(e)`, where `upsilon_(e)` is the value of escape velocity from the surface of earth . Find velocity of the particle on reaching to interstellar space (at infinity) in terms of `upsilon_(e)`.
View SolutionIn the figure shown in text, `m_(1) = m`, `m_(2) = 2m` and initial distance between them is `r_(0)`. Find velocities of the masses when separation between them becoms `r_(0)/(2)`.
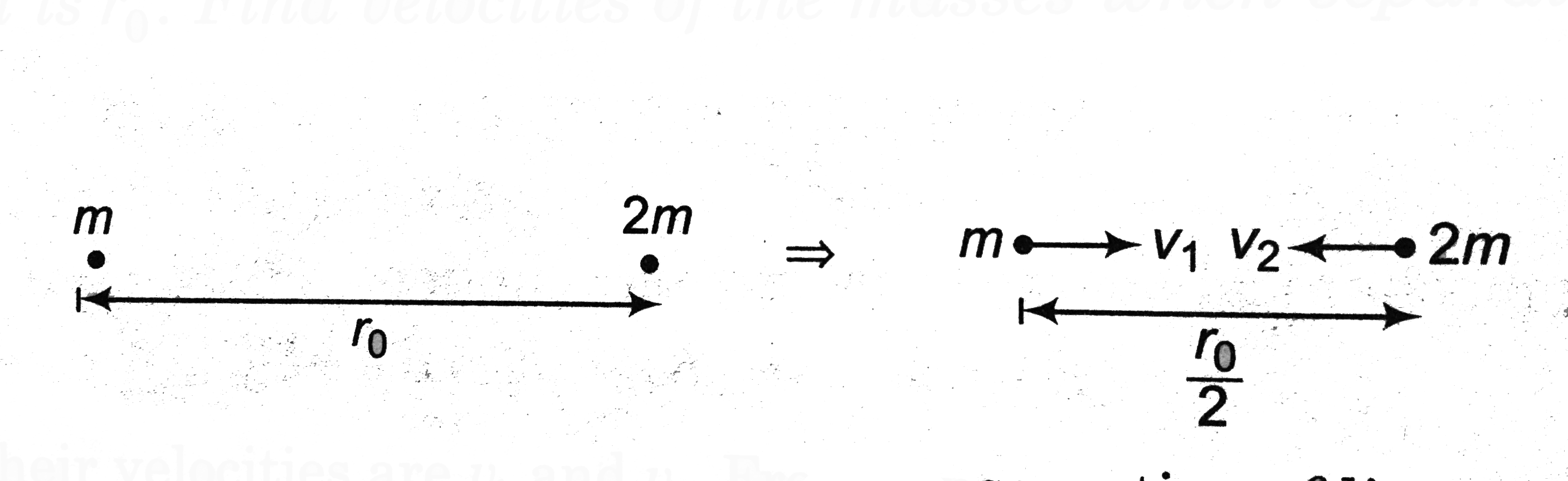
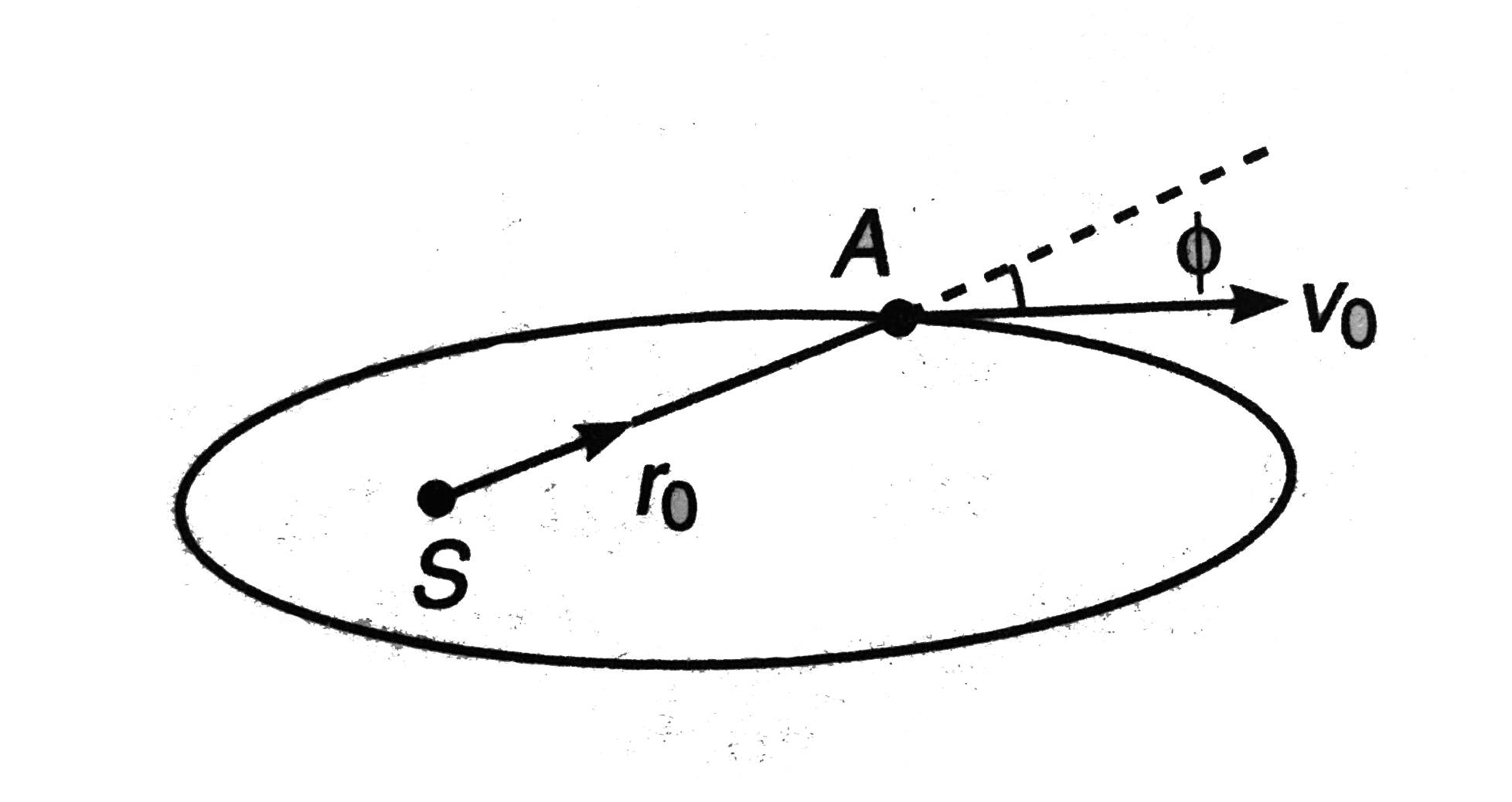
View SolutionFind the maximum and minimum distances of the planet `A` from the sun `S`, if at a certain moment of times it was at a distance `r_(0)` and travelling with the velocity `upsilon_(0)`. With the angle between the radius vector and velocity vector being equal to `phi`.
View SolutionA projectile of mass `m` is fired from the surface of the earth at an angle `alpha = 60^(@)` from the vertical. The initial speed `upsilon_(0)` is equal to `sqrt((GM_(e))/(R_(e))`. How high does the projectile rise ? Neglect air resistance and the earth's rotation.
View SolutionA planet of mass `m_(1)` revolves round the sun of mass `m_(2)`. The distance between the sun the planet is `r`. Considering the motion of the sun find the total energy of the system assuming the orbits to be circular.
View SolutionIn the problem discussed in the text, find `E - r` expressions for inside and outside points.
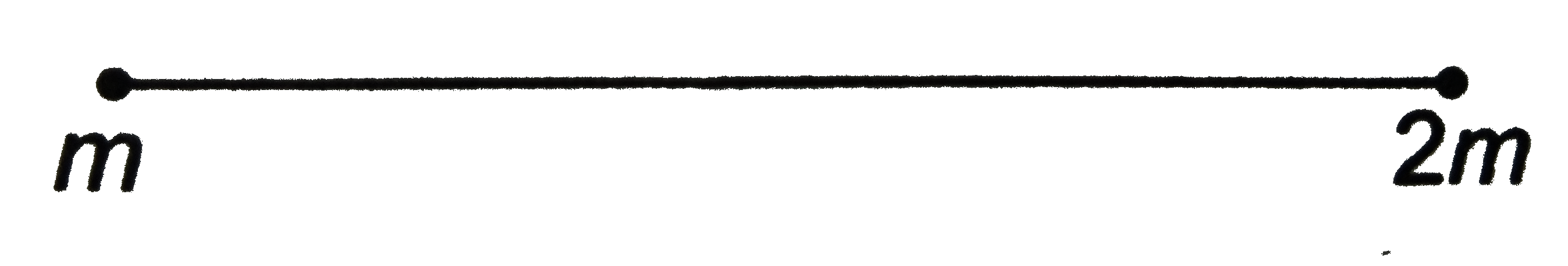
View SolutionTwo points masses 'm' and `2m` are kept at certain distance as shows in figure. Draw `E - r` and `V - r` graphs along the line joining them corresponding to given mass system.
View SolutionIn the problem discussed in the text, find the values of `E` and `V` at `p` dua to the remaining mass.
View SolutionDistance between the centres of two stars is `10a`. The masses of these stars are `M` and `16 M` and their radii a and `2a` respectively. A body of mass `m` is fired straight from the surface of the larger star towards the surface of the smaller star. What should be its minimum initial speed to reach the surface of the smaller star? Obtain the expression in terms of `G`, `M` and a.
View SolutionThree spherical shells of masses `M`, `2M` and `3M` have radii `R`, `3R` and `4R` as shows in figure. Find net potential at point `P`,
View Solution
Solved Examples questions and complete solutions for chapter GRAVITATION of DC PANDEY ENGLISH of Class 11
All Exercises from DC PANDEY Class 11 PHYSICS GRAVITATION
- (A) Chapter Exercises
- (B) Chapter Exercises
- (C) Chapter Exercises
- Check Point 10.1
- Check Point 10.2
- Check Point 10.3
- Check Point 10.4
- Check Point 10.5
- Check Point 10.6
- Example
- Exercise 13.1
- Exercise 13.2
- Exercise 13.3
- Exercise 13.4
- Exercise 13.5
- Exercise 13.6
- Exercise 13.7
- Level 1 Assertion And Reason
- Level 1 Single Correct
- Level 1 Subjective
- Level 2 More Than One Correct
- Level 2 Single Correct
- Level 2 Subjective
- Miscellaneous Examples
- Solved Examples