Complete Solutions to Try yourself of chapter MOTION IN A PLANE of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | MOTION IN A PLANE |
| AAKASH INSTITUTE | Try yourself |
| Number Of Questions | 48 |
| Academic Year | 2023-24 |
The resultant of ` vecp and vecq` makes angle `alpha "with " vecp and beta " with " vecq` . Then
View SolutionTwo forces 12 N and 16 N are acting upon a body, what can be the maximum and minimum resultant force on the body ?
View SolutionA motorboat is racing towards north at 25 `km//h` and the water current in that region is `10km//h` in the directioni of `60^(@)` east of south. Find the resultant velocity of the boat.
View SolutionA hydrogen balloon is flying eastward with speed `12 m.s^(-1)` . When wind starts blowing from north to south with speed `5 m.s^(-1)` what is the resultant velocity of balloon ?
View SolutionA boat is sent across a river with a velocity 8 `kmh^(-1)` If the resultant velocity of boat is `10h^(-1)`,then velocity of the river is
View SolutionA vector of magnitude 10 has its rectangular components as 8 and 6 along x and y axes. Find the angles it make with these axes.
View SolutionOne of the rectangular components of a force of 40 N is 20 N. Find the angle it makes with the component.
View SolutionTwo perpendicular forces of magnitudes 10 N and 5 N act at a point. Find their resultant.
View SolutionThe x and y components of a vector are ` 4sqrt3 m` and 4 m respectively. What angle does the vector make with positive x-axis ?
View SolutionA vector of magnitude 13 makes an angle ` 65.37^(@)` with the x-axis . What is its component along positive y-axis ?
View SolutionWhat is the resultant of unit vector s ` hatj and hatk` ?
[ Hint : ` |hatj| = |hatk| =1`]
View SolutionA particle lies in space at point ( 2,3,4) find the magnitude of its position vector.
View SolutionFind the sum of the vectors ` 10hati + 6hatj and 4hati - 2hatj`
View SolutionThe vectors are given below
` veca = hati + 2hatj +3hatk vecb = 2hati + 4hatj + 6hatk and vecc = 3hati + 6hatj + 9 hatk`
find the components of the vector ` veca + vecb -vecc`
View SolutionRain is falling at the speed of `25sqrt(3)` m/s vertically . The wind blows west to east at a speed of 25 m/s . Find the velocity of rain as experienced by a person standing on the ground .
View SolutionTwo vectors having magnitude 12 and 13 are inclined at and angle` 45^(@)` to each other.find their resultant vector.
View SolutionA resultant of two vectors makes ` 30^(@)` with one vector and ` 45^(@)` with the other. Find the two vectors if the resultant has the magnitude 15
View SolutionThe velocity of an object is given by ` vecv = ( 6 t^(3) hati + t^(2) hatj) m//s` . Find the acceleration at t = 2s.
View SolutionThe velocity (in m/s) of an object changes from ` vecv_(1) = 10 hati + 4hatj + 2hatk` to ` vecv_(2) = 4hati + 2hatj + 3hatk` in 5 second. Find the magnitude of average acceleration.
View SolutionThe position of an object is given by ` vecr= ( 9 thati + 4t^(3)hatj) ` m. find its velocity at time t= 1s.
View SolutionThe position of an object changes from ` vecr = ( 2hati + hatj) " m to " vecr_(1) = ( 4hati + 3hati)` m in 2s. Find its average velocity.
View SolutionThe velocity of an object at t =0 is ` vecv_(0) =- 4 hatj ` m/s . It moves in plane with constant acceleration ` veca = ( 3hati + 8 hatj) m//s^(2)`. What is its velocity after 1 s?
View SolutionAn object starts from rest, and moves under the acceleration ` veca = 4hati` . Its position after 3 s is given by ` vecr = 7 hati + 4hatj` . What is its initial position ?
View SolutionA boat is moving with a velocity `3 hat i+ 4hat j` with respect to ground. The water in the river is moving with a velocity `-3 hat i - 4 hat j` with respect to ground. The relative velocity of the boat with respect to water is.
View SolutionA boat is moving towards east velocity 4 m/s w.r.t still water and river is flowing towards north with velocity 2 m/s and the wind blowing towards north with velocity 6 m/s. the direction of the flag blown over by the wind hpisted on the boat is
View SolutionRain is falling vertically with a speed of `35 m s^(-1)` . A woman rides a bicycle with a speed of ` 12 m s^(-1)` in east to west direction. In which direction should she hold her umbrella ?
View SolutionA ship is steaming towards east with a speed of 8 m/s. A women runs across the deck at a speed of `6 ms^(-1)` towards north. What is the velocity of the women relative to the sea ?
View SolutionA boat is moving with a velocity `3 hat i+ 4hat j` with respect to ground. The water in the river is moving with a velocity `-3 hat i - 4 hat j` with respect to ground. The relative velocity of the boat with respect to water is.
View SolutionA bus appears to move northwards at a speed of ` 20sqrt3 " km h"^(-1)` to a man driving his car eastwards with speed ` 20 " km h "^(-1)`. Find the velocity of the bus w.r.t ground.
View SolutionFind the velocity of a projectile at the highest point, if it is projected with a speed ` 15 m s^(-1)` in the direction `45^(@)` above horizontla. (take g = ` 10 m s^(-2))`
View SolutionA cricket ball is thrown at a speed of ` 28 ms^(-1)` in a direction `30 ^(@)` above the horizontal. Calculate (a)the maximum height (b) the time taken by ball to return to the same level, and (c )the distance from the thrower to the point where the ball returns to the same level.
View SolutionA bullet fired at an angle of ` 60^(@)` with the vertical hits the levelled ground at a distance of 200 m . Find the distance at which the bullet will hit the ground when fired at angle of ` 30^(@)`. (with same speed).
View SolutionAn object is projected with velocity `vecv_(0) = 15hati + 20hatj` . Considering x along horizontal axis and y along vertical axis. Find its velocity after 2s.
View SolutionA player kicks a ball at an angle of `37^(@)` to the horizontal with an initial speed of ` 15 ms^(-1)` . Find its time of fight.
View SolutionFind the ratio of maximum horizontal range and the maximum height attained by the projectile. i.e. for ` theta_(0) = 45^(@)`
View SolutionA hiker stands on the edge of a cliff 490 m above the ground and throws a stone horizontally with a speed of 15 m `s^(-1)`. The time taken by the stone to reach the ground is
View SolutionA a certain instant of time a ballon is moving downwards with speed of 5m/s. It has a constant retardation of 5m/s2. A man in the ballon drops a stone at that instant. It hits the ground after 2s. Then the speed with which the stone hits the ground is.
View SolutionAn insect trapped in a circular groove of radius, 12 cm moves along the groove steadily and completes 7 revolutions in 100s. What is the angular speed and the linear speed of the motion ?
View SolutionAn insect trapped in a circular groove of radius, 12 cm moves along the groove steadily and completes 7 revolutions in 100s. What is the angular speed and the linear speed of the motion ?
What is the magnitude of the centripetal acceleration in above problem ?
View SolutionA mark on the rim of a rotating cicular wheel of 0.50 m radius is moving with a speed of `10 ms^(-1)` . Find its angular speed.
View SolutionFind the angular speed of the minute hand of a clock.
View SolutionA dust particle lying at the rim of a wheel has an angular speed of 22 rad/s. Find the time period.
View SolutionIf and object completes 49 revolutions in a minute around a circular path with a speed ` 7 ms ^(-1)` find the radius of the path.
View SolutionA particle goes uniformly in circular motion with an angualr speed `pi/8 rad s^(-1)` . What is its time period ?
View SolutionThe angualr displacement of an object having uniform cirular motion is ` pi/4` rad in every 3s. Find its frequency of revolution.
View SolutionA man is coming down an incline of angle `30^@`. When he walks with speed `2(sqrt(3)) m s^-1` he has to keep his umbrella vertical to protect himself from rain. The actual speed of rain is `5 ms^-1`. At angle with vertical should he keep his umbrella when he is at rest so that he does not getb drenched ?
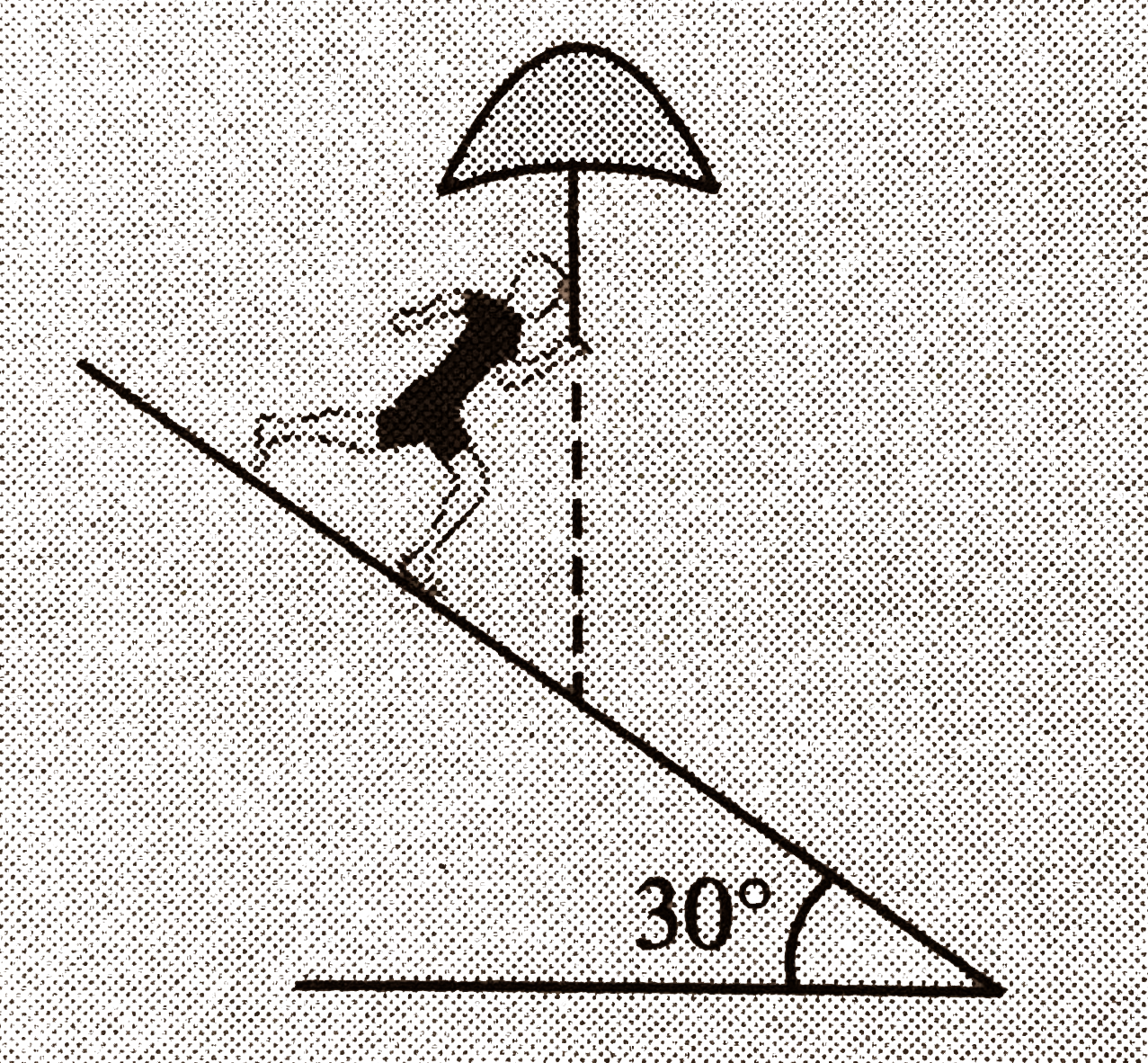 .
.
View SolutionIf the helicopter is flying at constant velocity then where will it be when the packet strikes the ground.
View SolutionFour particles `A, B, C and D` are situated at the corner of a square `ABCD` of side a at t-0. Each of particles moves with constant speed (v). A always has its velocity along `AB, B` along `BC, C` along `CD~ and D` along `DA`. At what time will these particles meet each other ?
View Solution
Try yourself questions and complete solutions for chapter MOTION IN A PLANE of AAKASH INSTITUTE ENGLISH of Class 12
All Exercises from AAKASH INSTITUTE Class 12 PHYSICS MOTION IN A PLANE
- Assignement section -A Objective (one option is correct)
- Assignement section -B Objective (one option is correct)
- Assignement section -C Objective (More than one option is correct)
- Assignement section -D (Linked Comprehension)
- Assignement section -E (Assertion-Reason)
- Assignement section -F (Matrix-Match)
- Assignement section -G (Integer)
- Assignement section -H (Multiple True-False)
- Assignement section -I (Subjective )
- Assignement section -J (Aakash Challengers Questions)
- Example
- llustration 1 :
- Try yourself