Complete Solutions to Try Yourself of chapter SYSTEM OF PARTICLES AND ROTATIONAL MOTION of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | SYSTEM OF PARTICLES AND ROTATIONAL MOTION |
| AAKASH INSTITUTE | Try Yourself |
| Number Of Questions | 63 |
| Academic Year | 2023-24 |
Two bodies of masses `1kg` and `3kg` are lying in `xy` plane at `(0,0)` and `(2,-1)` respectively. What are the coordinates of the centre of mass ?
Hint. `x_(cm)=(m_(1)x_(1)+m_(2)x_(2))/(m_(1)+x_(2))`, `y_(cm)=(m_(1)y_(1)+m_(2)y_(2))/(m_(1)+m_(2))`
View SolutionThree point masses of `1kg`, `2kg` and `3kg` lie at `(0,0)`, `(1,2)`, `(3,-1)` respectively. Calculate the coordinates of the centre of mass of the system.
View SolutionThree particles of masses `m`,`m` and `4kg` are kept at a verticals of triangle `ABC`. Coordinates of `A`, `B` and `C` are `(1,2)` , `(3,2)` and `(-2,-2)` respectively such that the centre of mass lies at origin. Find the value of mass `m`.
Hint. `x_(cm)=(sum_(i-1)^(3)m_(i)x_(i))/(sum_(i=1)^(3)m_(i))`, `y_(cm)=(sum_(i=1)^(3)m_(i)y_(i))/(sum_(i=1)^(3)m_(i))`
View SolutionThree particles having their masses in the ratio `1 : 3 : 5` are kept at the vertices of a triangle `ABC`. Coordinate of `A`, `B` and `C` are `(9,-3)`, `(3,4)` and `(0,0)`. Find the coordinates of the centre of mass.
Hint. `x_(cm)=(sum_(i-1)^(3)m_(i)x_(i))/(sum_(i=1)^(3)m_(i))`, `y_(cm)=(sum_(i=1)^(3)m_(i)y_(i))/(sum_(i=1)^(3)m_(i))`
View SolutionCentre of mass of the system lies inside disc or square plate and why ?
View SolutionTwo particles of equal mass are moving along the same line with the same speed in the same direction. What is the speed of the centre of mass of the system ?
View SolutionTwo particles of equal mass are moving along the same straight line with the same speed in opposite direction. What is the speed of the centre of mass of the system ?
View SolutionA shell following a parabolic path explodes somewhere in its flight. The centre of mass of fragements will move in
View SolutionAll the particles are situated at a distance `R` from the origin. The distance of centre of mass of the body from the origin will be
`(1)` More than `R`
`(2)` Less than `R`
`(3)` Equal to `R`
`(4)` At the origin
View SolutionWill the velocity and acceleration of centre of mass change if particles `A`, `B` and `C` are projected from different locations but with same velocities ?
View Solution`vecA=(3hati+2hatj-6hatk)` and `vecB=(hati-2hatj+hatk)`, find the scalar product `vecA` and `vecB`
View Solution`vecA=(hati-2hatj+6hatk)` and `vecB=(hati-2hatj+hatk)`, find the cross product between `vecA` and `vecB`.
View SolutionFind a unit vector in the direction of vector `vecA=(hati-2hatj+hatk)`
View SolutionFind a vector perpendicular to vector `vecA=(hati+2hatj-3hatk)` as well as `vecB=(hati+hatj-hatk)`
View SolutionThe angular displacement of a particle is `24` rad in `10` seconds. Calculate its angular velocity.
View SolutionThe angular velocity of a rigid body is `24` rad `s^(-1)`, Calculate the time it will take to rotate `72` rad.
View SolutionThe angular velocity of circular disc of radius `2cm` is `20` rad `s^(-1)`. Calculate the linear velocity of the disc.
View SolutionWhat is the angular velocity of a particle lying on the axis of rotation ?
View SolutionWhat is the angular acceleration of a particle moving with constant angular velocity ?
View SolutionA wheel is rotating with an angular velocity of `3 rad s^(-1)`. If the angular acceleration is `2 rad s^(-1)` then calculate its angular velocity after `5` second.
View SolutionA force `(hati-2hatj+3hatk)` acts on a particle lying at origin. What is the torque acting on the particle about the origin.
View SolutionA force `(hati-2hatj+3hatk)` acts on a particle of position vector `(3hati+2hatj+hatk)`. Calculate the `hatj^(th)` component of the torque acting on the particle.
View SolutionA particle of mass `1kg` is moving about a circle of radius `1m` with a speed of `1m//s`. Calculate the angular momentum of the particle.
View SolutionWhat is the angular momentum of a particle at rest ?
Hint. `vecv=0`.
View SolutionA torque of `5Nm` is applied on a particle for `2` seconds, calculate the change in its angular momentum.
View SolutionThe angular momentum of a particle changes from `4kg m^(2)s^(-1)` to `8kg m^(2)s^(-1)` is `2` seconds. Calcualte the torque acting on the particle
View SolutionGive an example of a body in rotational equilibrium but not in translational equilibrium.
View SolutionGive conditions for mechanical equilibrium.
View SolutionIf the parallel forces acting on a lever are in the ratio `3 : 5` then what is the mechanical advantage of the lever ?
View SolutionDefine mechanical advantage of a lever.
View SolutionDefine the term centre of gravity of a body?
View SolutionWhy the centre of gravity coincides with the centre of mass only if the size of the body is small ?
View SolutionCalculate the moment of inertia of a ring of mass `2kg` and radius `2cm` about an axis passing through its centre and perpendicular to its surface.
View SolutionThe moment of inerta of a ring of mass `1kg` about an axis passing through its centre perpendicular to its surface is `4kgm^(2)`. Calculate the radius of the ring.
View SolutionCalculate the radius of gyration of a uniform circular ring about an axis passing through its diameter.
View SolutionCalculate the radius of gyration of a circular disc about its diameter.
View SolutionCalculate the moment of inertia of a disc about its any diameter ?
View SolutionThe moment of inertia of a uniform ring about an axis passing through its centre and perpendicular to its plane is `100kgm^(2)`. What is the moment of inertia of the ring about its diameter ?
View SolutionCalculate the moment of inertia of a ring about a tangent to the circle of the ring normal to its plane.
View SolutionCalculate the moment of inertia of the given rod about an axis `CD`
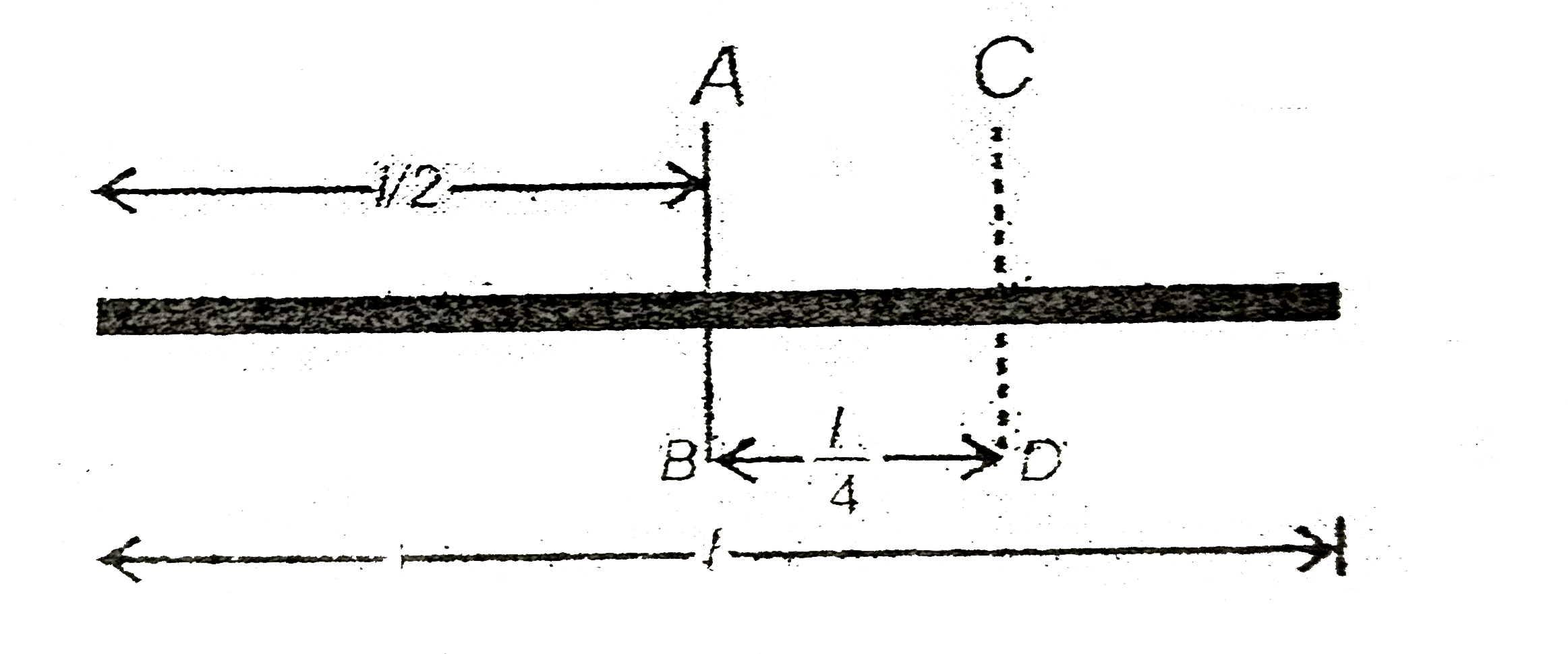
View SolutionWhat is the angular acceleration of a particle if the angular velocity of a particle becomes `4` times of its initial angular velocity `1` rad s in `2` seconds
View SolutionA particle moving with an angular velocity of `200` rad s^(-1)` and start de accelerating at a rate of 2 rad s^(-2)`. Calculate the time in which it will come to rest.
View SolutionThe angular velocity of a motor wheel changes from `180` rpm to `300` rpm in `4` seconds. Calculate the no. of revolutions does the engine make during this time.
View SolutionThe angular velocity of flywheel changes from `240` rpm to `120` rpm in `6` seconds. Calculate the no of revolutions it will make before coming to rest.
View SolutionCalculate the angular velocity with which a wheel is rotating. If its instanteous power is `500W`, and an external torque of `50Nm` is applied to it.
Hint : `P=tau omega`
View SolutionCalculate the instantaneous power of a body rotating with angular velocity of `20rad//s^(-2)`, when an external torque of `5 Nm` is applied to it.
Hint : `P=tau omega`.
View SolutionFind the speed of the block after moving down by `x`
View SolutionWhat are the forces acting on the disc?
View SolutionTwo masses M and m are connect by a light string gong over a pulley of radis r. The pulley is free to rotate about its axis which is kept horizontal. The moment of inertia of the pulley about the axis is I. The system is releaed from rest. Find the angular momentum fo teh system when teh mass Mhas descended through a height h. The string does not slip over the pulley.
View SolutionWhat is the torque acting on a body moving with constant angular velocity ?
View SolutionCompute the torque acting on a wheel of moment of inertia `10kgm^(2)`, moving with angular acceleration `5 rad s^(-2)`.
View SolutionAn ice skater having moment of inertia `l` rotating with angular speed `omega` suddenly opens her arms, which reduces her angular velocity to `(omega)/(4)`. Calculate the change in moment of inertia of the dancer.
View SolutionThe moment of inertia of a body moving with angular velocity `omega` decreases from `I` to `(I)/(3)` without any external torque. Calculate the new angular velocity of the body.
View SolutionFind the kinetic energy of the disc when it is fallen of a height `h`.
View SolutionA solid sphere and a hollow cylinder have the same radius and the same mass. They start from rest at the top of an incline. Which reaches the bottom first? Which has the greater speed at the bottom? Which has the greater total kinetic energy of the bottom ? Which has the greater rotational `KE`?
View SolutionTwo spheres look identical and have the same mass. However one is hollow and the other is solid. Describe an experiment to determine which is which.
View SolutionWill the ring roll purely if force `F` is applied in between topmost point and centre and the surface is smooth.
View SolutionIf the ring slips then will it be backward slipping or forward slipping.
View Solution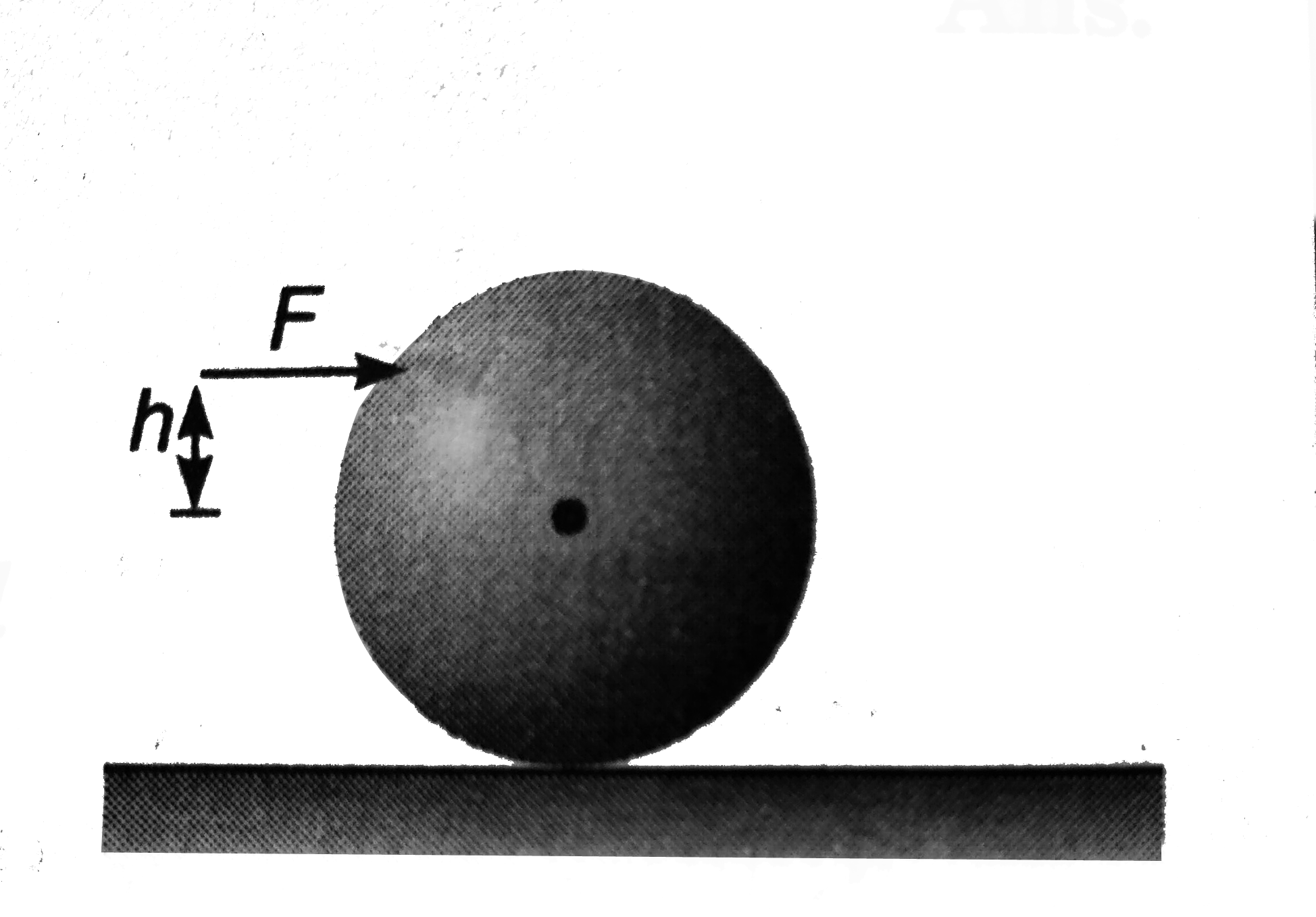
A billiard ball, initially at rest, is given a sharp impulse by a cue. The cue is held horizontally a distance `h` above the centre line as shown in figure. The ball leaves the cue with a speed `v_(0)` and because of its backward slipping eventually acquires a final
speed `(9)/(7)v_(0)` show that `h=(4)/(5)R`
Where `R` is the radius of the ball.
View SolutionCalculate the kinetic energy of a solid sphere of mass `2kg` rolling down an inclined plane with a velocity of `2m//s` about an axis passing through its diameter.
View SolutionThe kinetic energy of a rolling solid cylinder of mass `4kg` about it axis of rotation is `20kgm^(2)s^(-2)`. Calculate the velocity with which it is moving.
View SolutionCalculate the velocity with which a solid cylinder rolls down an inclined plane of height `10m` with slipping (take `g=10m//s^(2)`)
View SolutionA uniform ring rolls down an inclined plane without slipping. If it reaches the bottom of a speed of `2m//s`, then calculate the height of the inclined plane (use `g=10m//s^(2)`)
View Solution
Try Yourself questions and complete solutions for chapter SYSTEM OF PARTICLES AND ROTATIONAL MOTION of AAKASH INSTITUTE ENGLISH of Class 12
All Exercises from AAKASH INSTITUTE Class 12 PHYSICS SYSTEM OF PARTICLES AND ROTATIONAL MOTION
- Assignment (Section - A) Objective Type Questions (One option is correct)
- Assignment (Section - B) Objective Type Questions (One option is correct)
- Assignment (Section - C) Objective Type Questions (More than one option are correct)
- Assignment (Section - D) Linked Comprehension Type Questions
- Assignment (Section - E) Assertion-Reason Type Questions
- Assignment (Section - F) Matrix-Match Type Questions
- Assignment (Section - G) Integar Type Questions
- Assignment (Section - H) Multiple True-False Type Questions
- Assignment (Section - I) Subjective Type Questions
- Assignment (Section - J) Aakash Challengers Questions
- Example
- Illustration
- Try Yourself