Complete Solutions to Circles of chapter CIRCLES of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | CIRCLES |
| NCERT EXEMPLAR | Circles |
| Number Of Questions | 52 |
| Academic Year | 2023-24 |
AD is a diameter of a circle and AB is a chord. If AD = 34cm, AB = 30cm, the distance of AB form the centre of the circle is
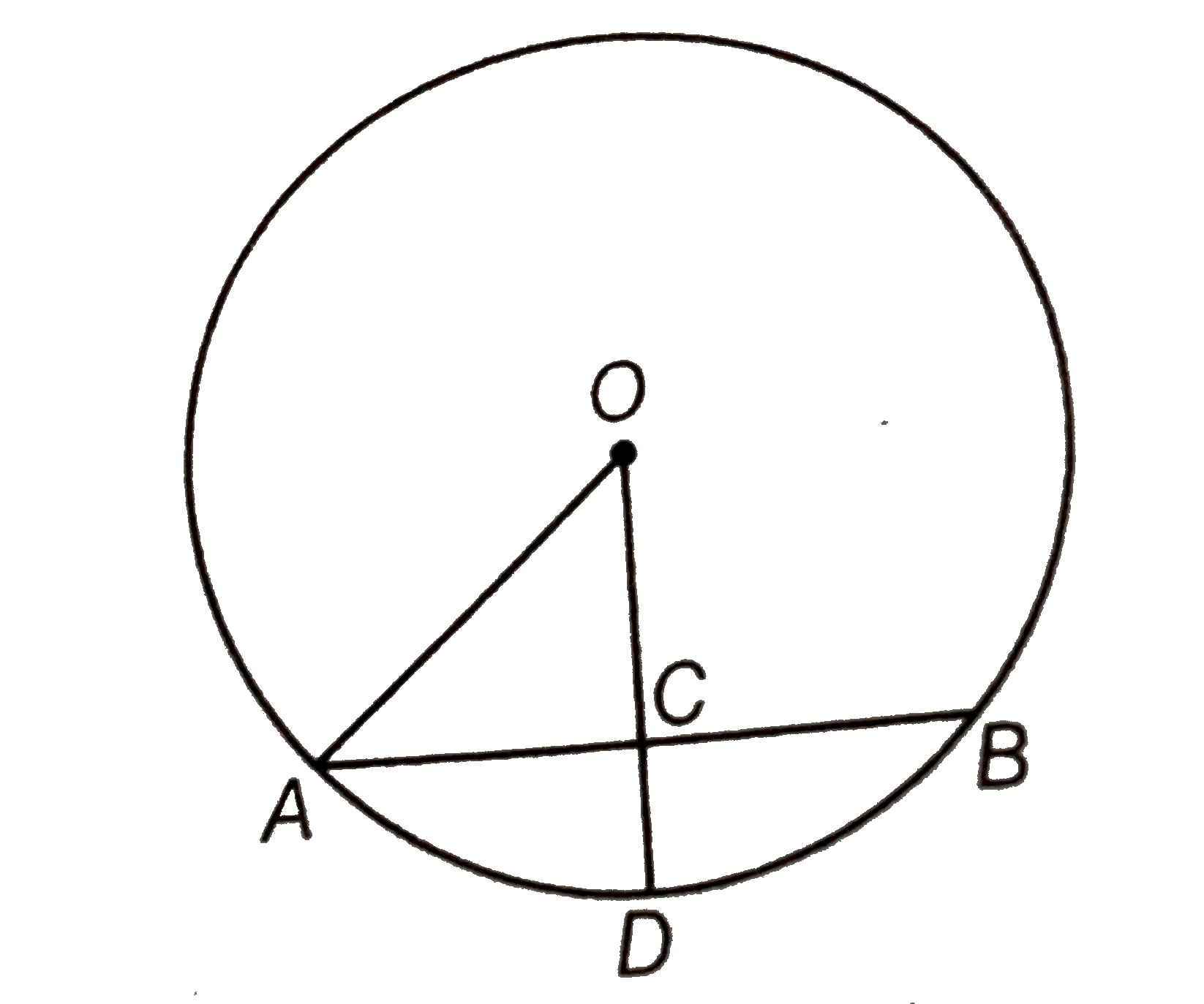
View SolutionIn figure, if OA=5cm, AB=8 cm and OD is perpendicular to AB, then CD is equal to
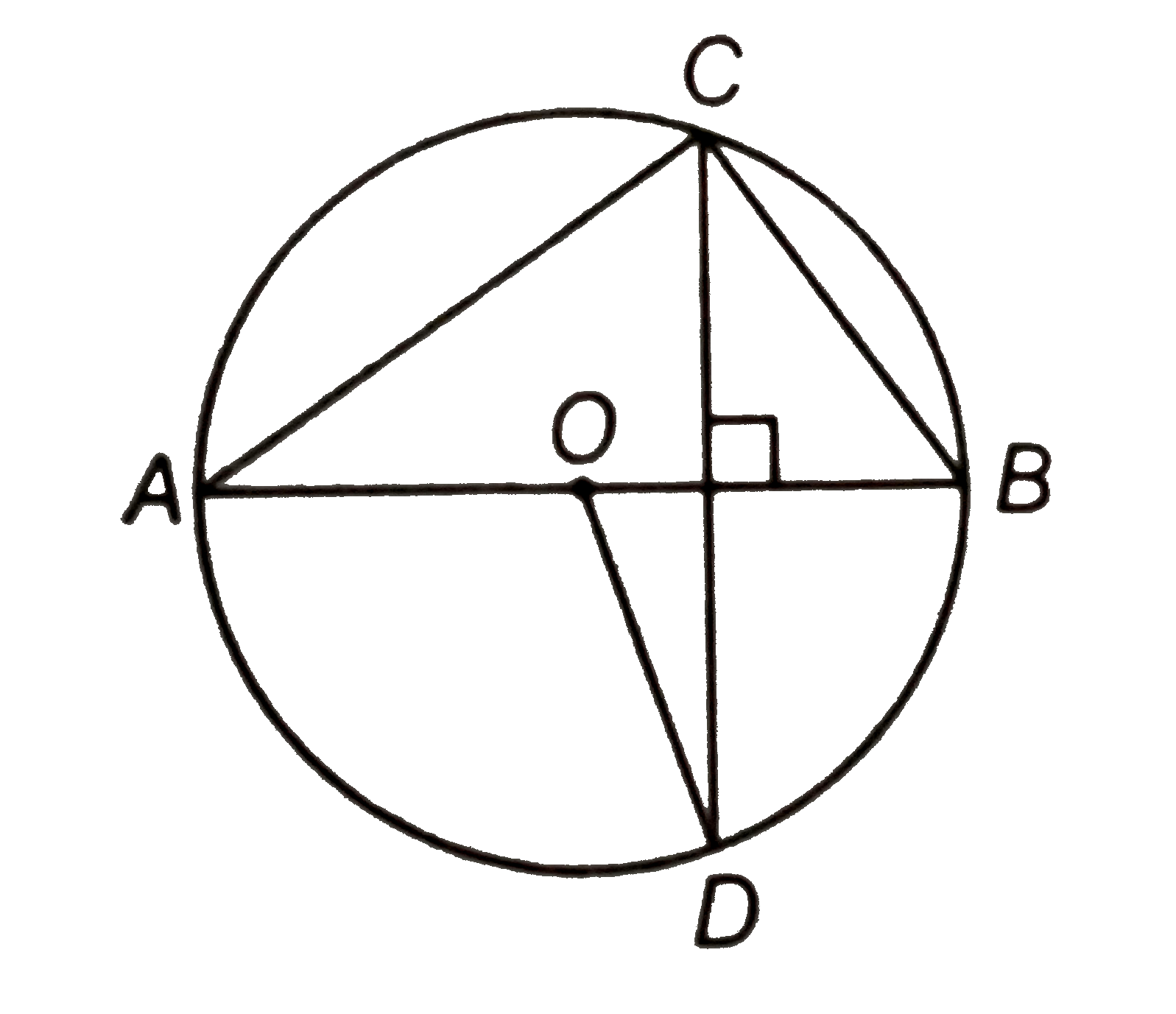
View SolutionIf AB =112cm, BC=16 cm and AB is perpendicular to BC, then the radius of the circle passing through the points A, B and C is
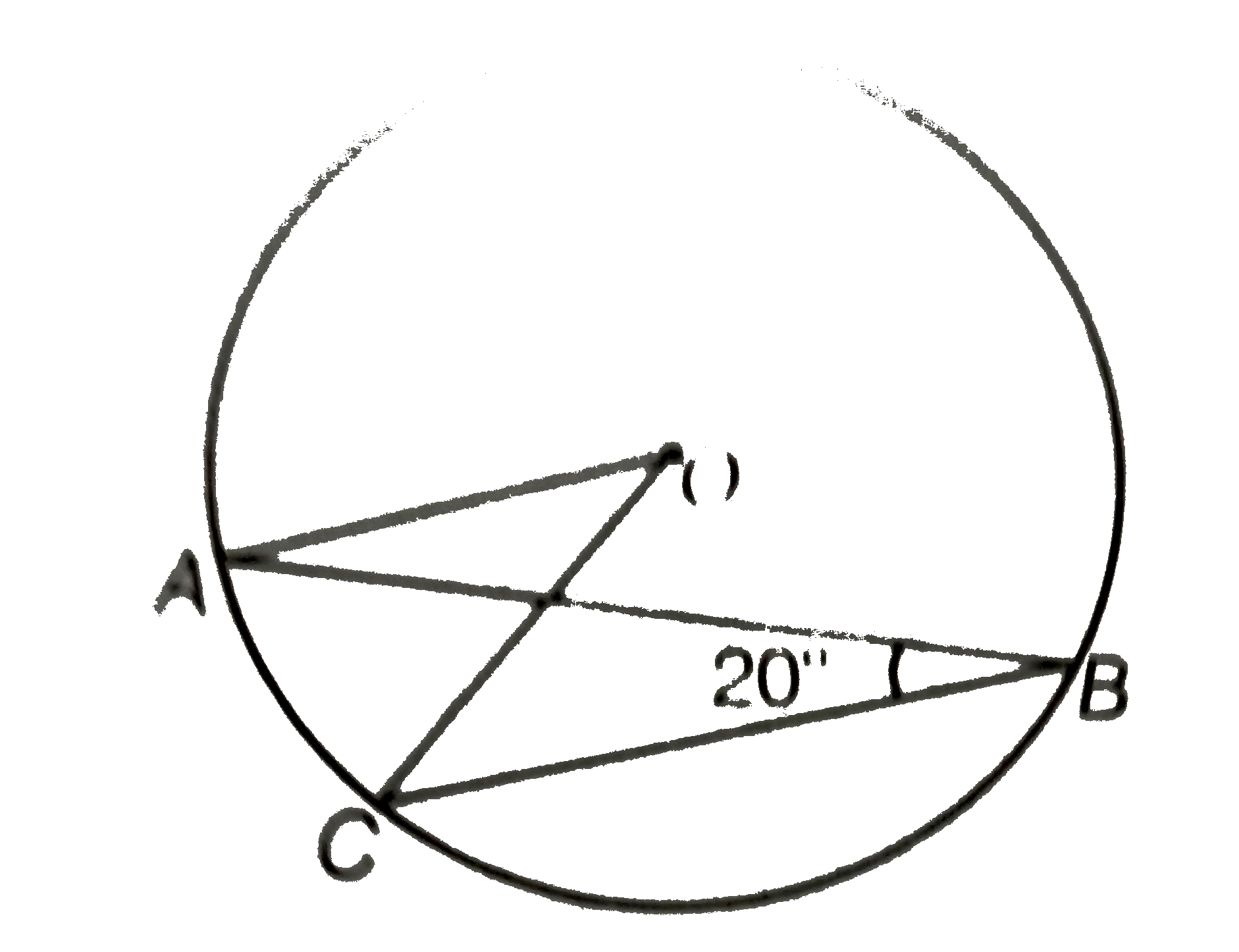
View SolutionIf figure, if`angleABC=20^(@),"then"angleAOC" is equal to"`
View SolutionIf AOB is a diameter of the circle and AC=BC, then `angle CAB` is equal to
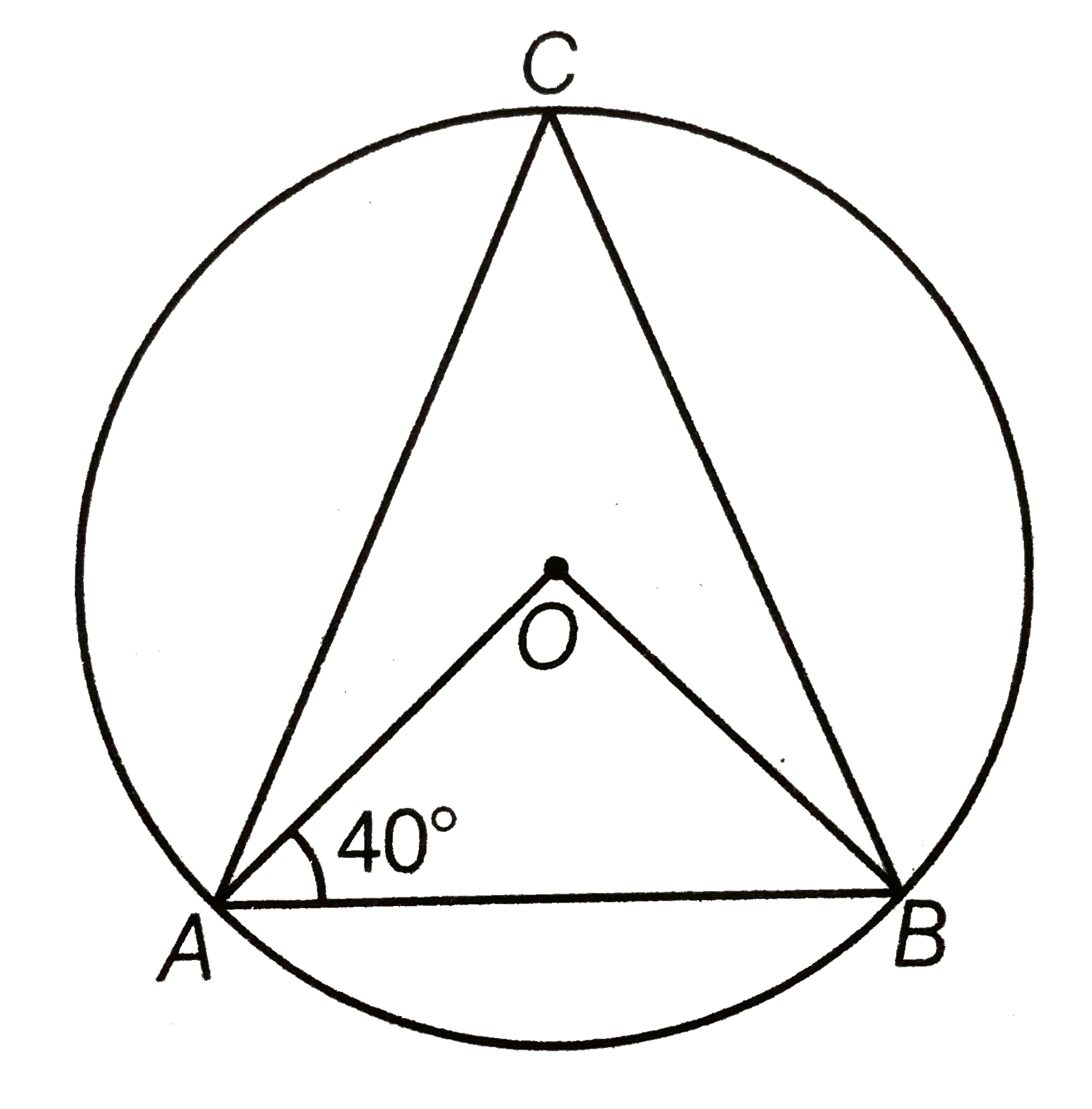
View SolutionIn figure, if `angleOAB=40^(@),"then"angleACB " is equal to "`
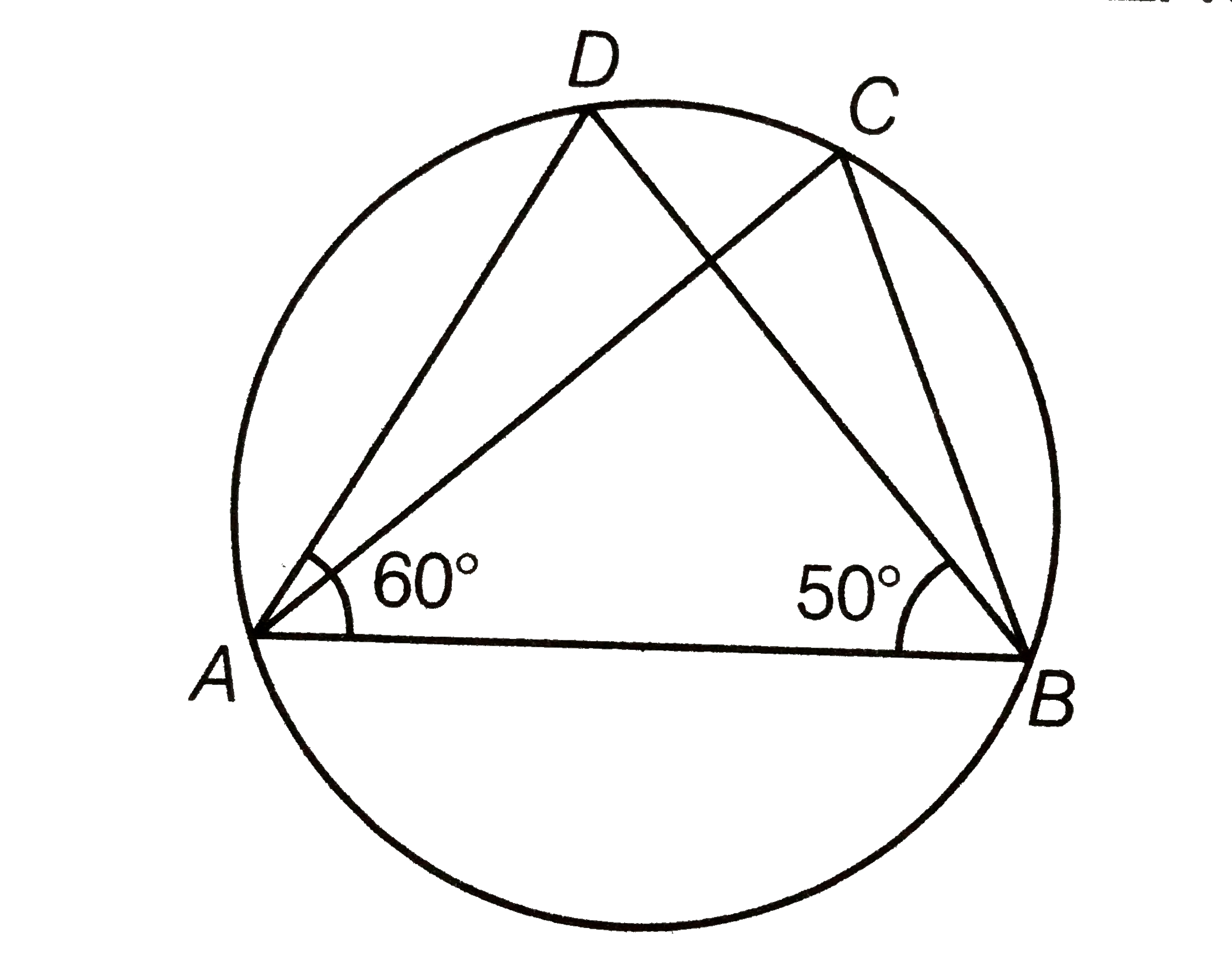
View SolutionIn figure, if `angleDAB=60^(@),angleABD=50^(@),"then "angleACB ` is equal to
View SolutionABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and `angleADC=140^(@)," than "angleBAC ` is equal to
View SolutionIn figure, BC is a diameter of the circle and `angleBAO=60^(@)." Then", angleADC`is equal to
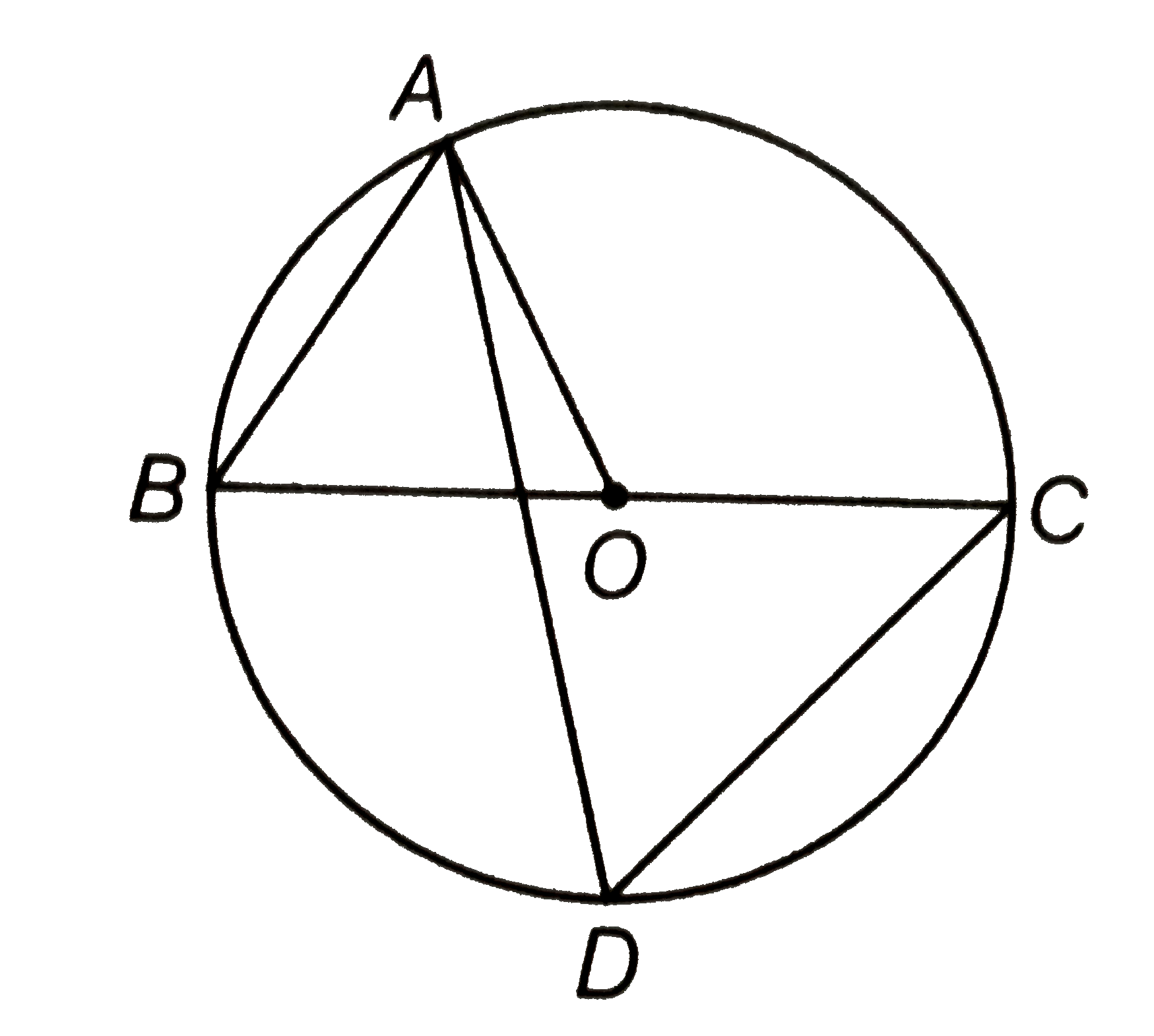
View SolutionIn figure, if `angleAOB=90^(@) and angleABC=30^(@)," then" angleCAO` is equal to
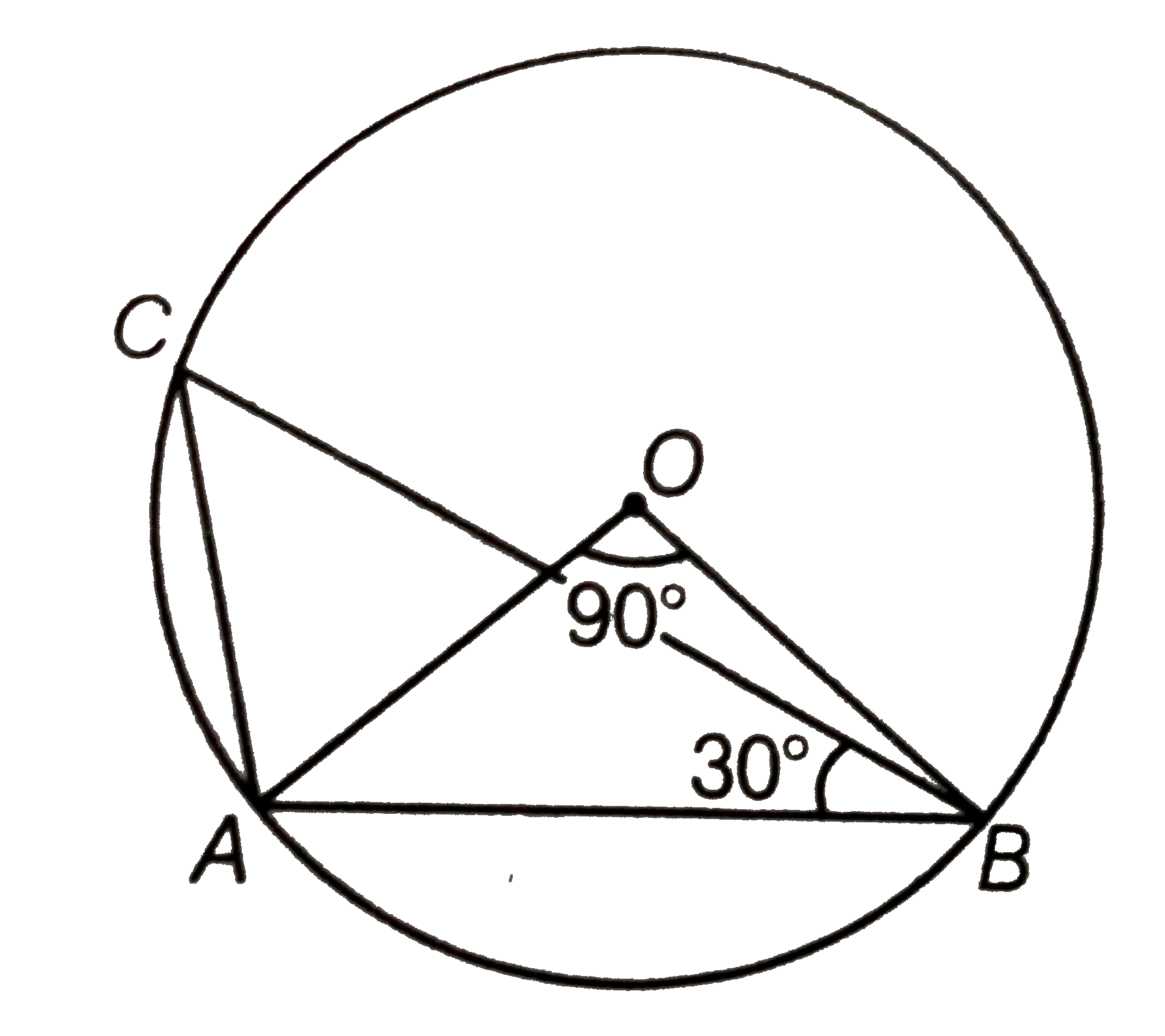
View SolutionTwo chords AB and CD of a circle are each at distances 4 cm from the centre. Then,
View SolutionTwo chords AB and AC of a circle with centre O are on the opposite sides of OA. Then, `angleOAB=angleOAC`.
View SolutionThe congruent circles with centres Oand O' intersect at two points A and B. Then, `angleAOB=angleAO'B`.
View SolutionThrough three collinear points a circle can be draw.
View SolutionA circle of radius 3 cm can be drawn through two points A, B such that AB=6 cm.
View SolutionIf AOB is a diameter of a circle and C is a point on the circle, then `AC^(2)+BC^(2)=AB^(2)`.
View Solutionstate true of false
`angleA=90^(@),angleB=70^(@),angleC=95^(@) and angleD=105^(@)` can be the vertex of cyclic quadrilateral
View SolutionIf A, B, C and D are four points such that `angleBAC=30^(@)and angleBDC=60^(@)`,then D is the centre of the circle through A, B and C.
View SolutionIf A, B, C and D are four points such that `angleBAC=45^(@) and angleBDC=45^(@)`, then A, B, C and D are concyclic.
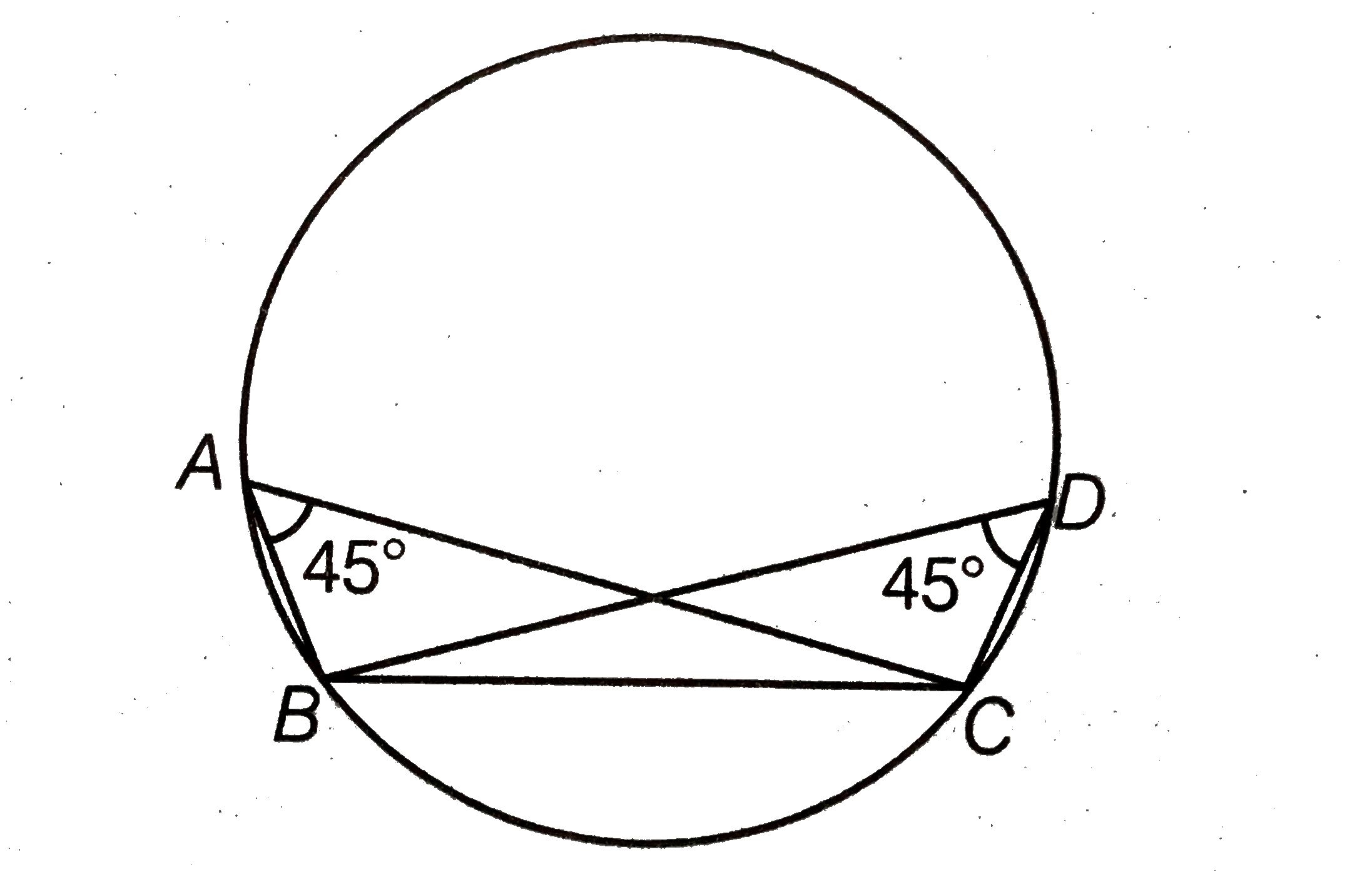
View SolutionIn figure, if AOB is a diameter and `angleADC=120^(@),"then"angleCAB=30^(@)`.
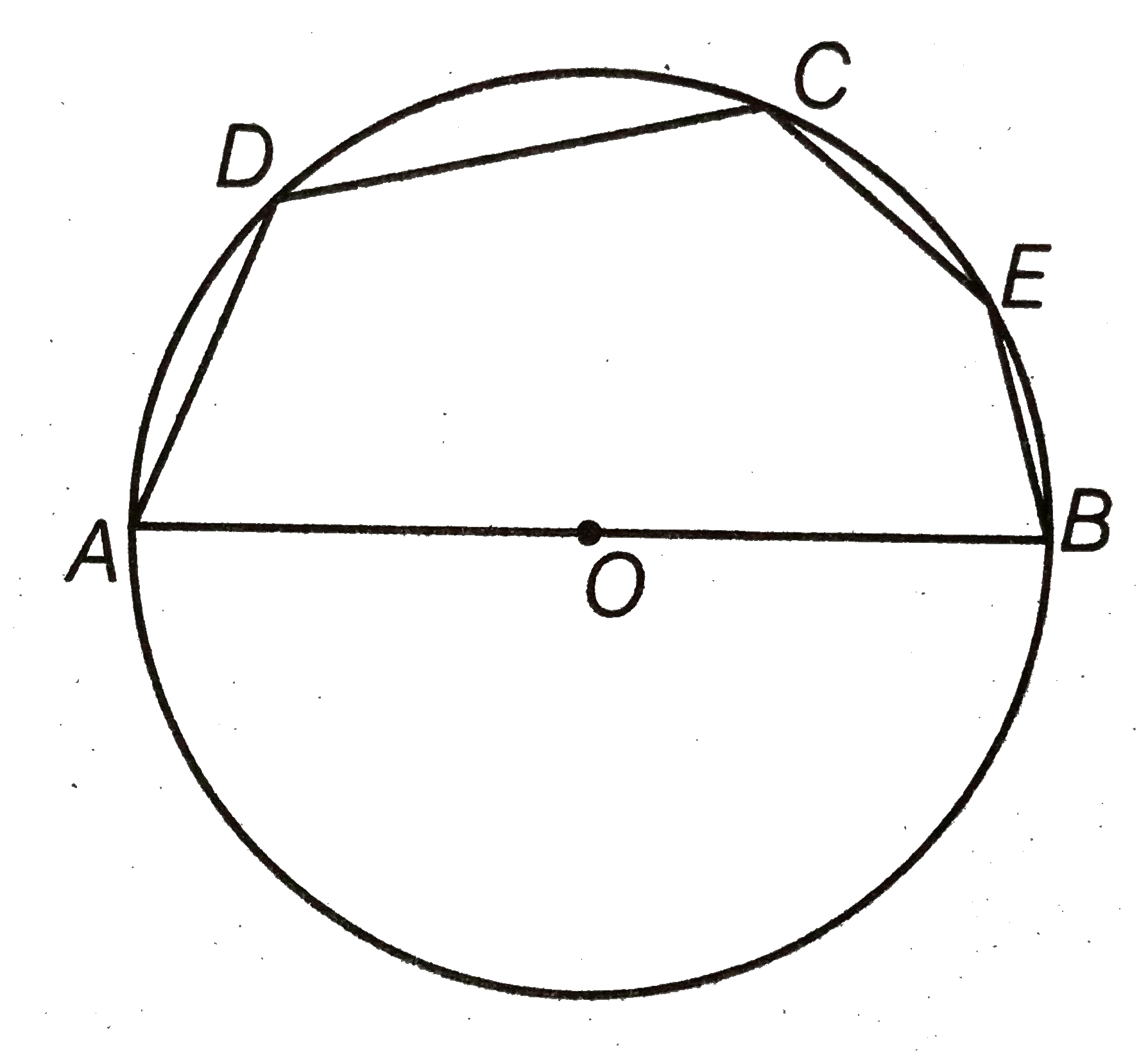
View SolutionIf two arcs of a circle (or of congruent circles) are congruent, then corresponding chords are equal.
View SolutionIf the perpendicular bisector of a chord AB of a circle PXAQBY intersects the circle at P and Q, prove that arc `PXA cong"arc "PYB`.
View Solution`A`, `B` and `C` are three points on a circle. Prove that the perpendicular bisectors of `AB`, `BC` and `CA` are concurrent.
View SolutionTwo chords `A B` and `A C` of a circle are equal. Prove that the centre of the circle lies on the angle bisector of `/_B A Cdot`
View SolutionIf a line segment joining mid-points of two chords of a circle passes through the centre of the circle, prove that the two chords are parallel.
View Solution`ABCD` is such a quadrilateral that A is the centre of the circle passing through `B, C and D`. Prove that `/_CBD+ /_CDB =1/2 /_BAD`.
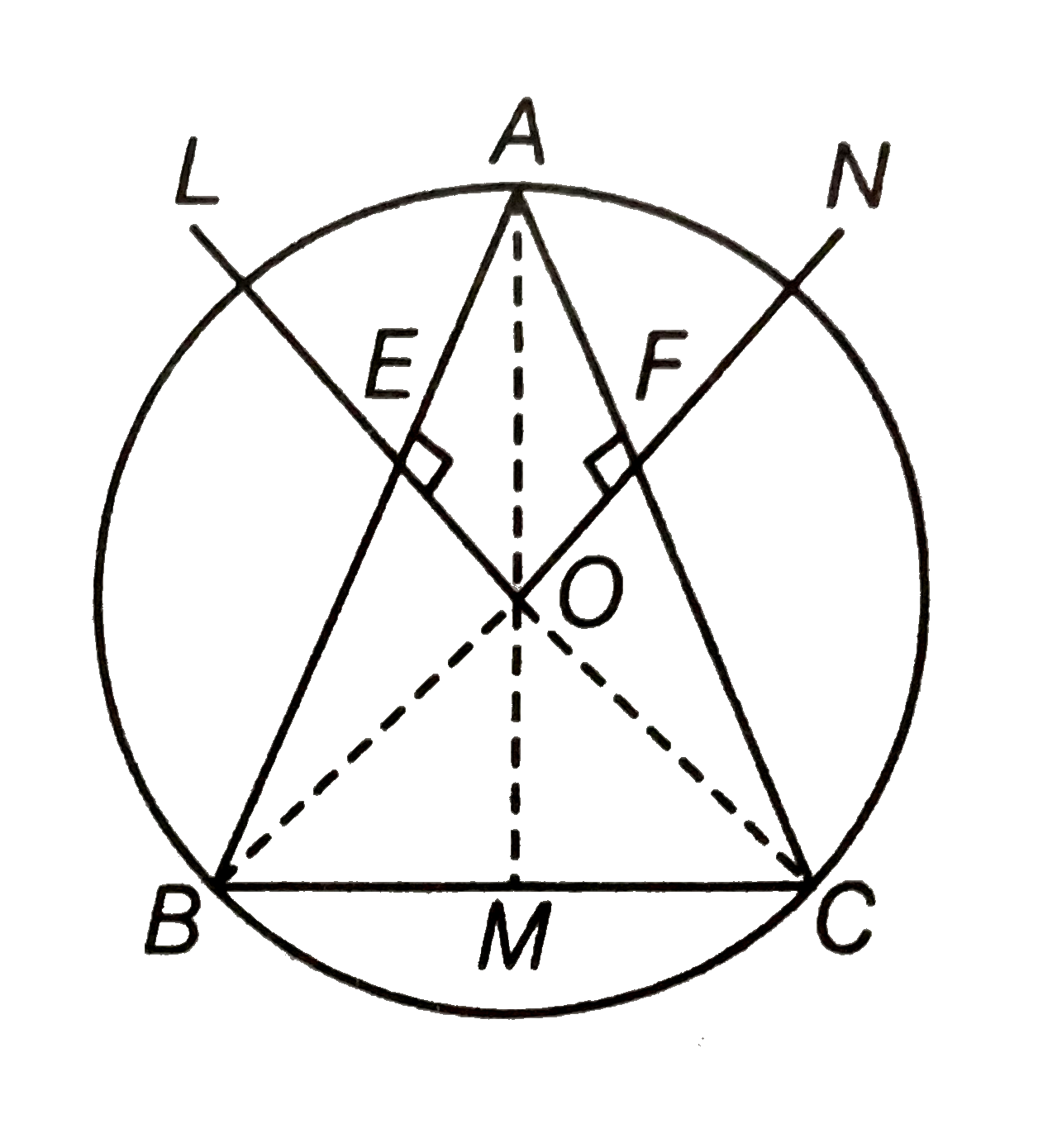
View SolutionIf `O` is the circumcentre of a ` A B C` and `O D_|_B C ,` prove that `/_B O D=/_adot`
View SolutionOn a common hypotenuse AB, two right angled triangles, ACB and ADB are situated on opposite sides. Prove that `angleBAC=angleBDC`.
View SolutionTwo chords AB and AC of a circle subtends angles equal to `90^(@) and 150^(@)`, respectively at the centre. Find`angleBAC`, if AB and AC lie on the opposite sides of the centre.
View SolutionIf BM and CN are the perpendiculars drawn on the sides AC and BC of the `Delta ABC`, prove that the points B, C, M and N are concyclic.
View SolutionIf a line is drawn parallel to the base of an isosceles triangle to intersect its equal sides, prove that the quadrilateral, so formed is cyclic.
View SolutionIf the two sides of a pair of opposite sides of a cyclic quadrilateral are equal, then
View SolutionThe circumcentre of the `DeltaABC" is O. Prove that "angleOBC+angleBAC=90^(@)`.
View SolutionA chord of a circle is equal to the radius of the circle find the angle subtended by the chord at a point on the monor arc and also at a point on the major arc.
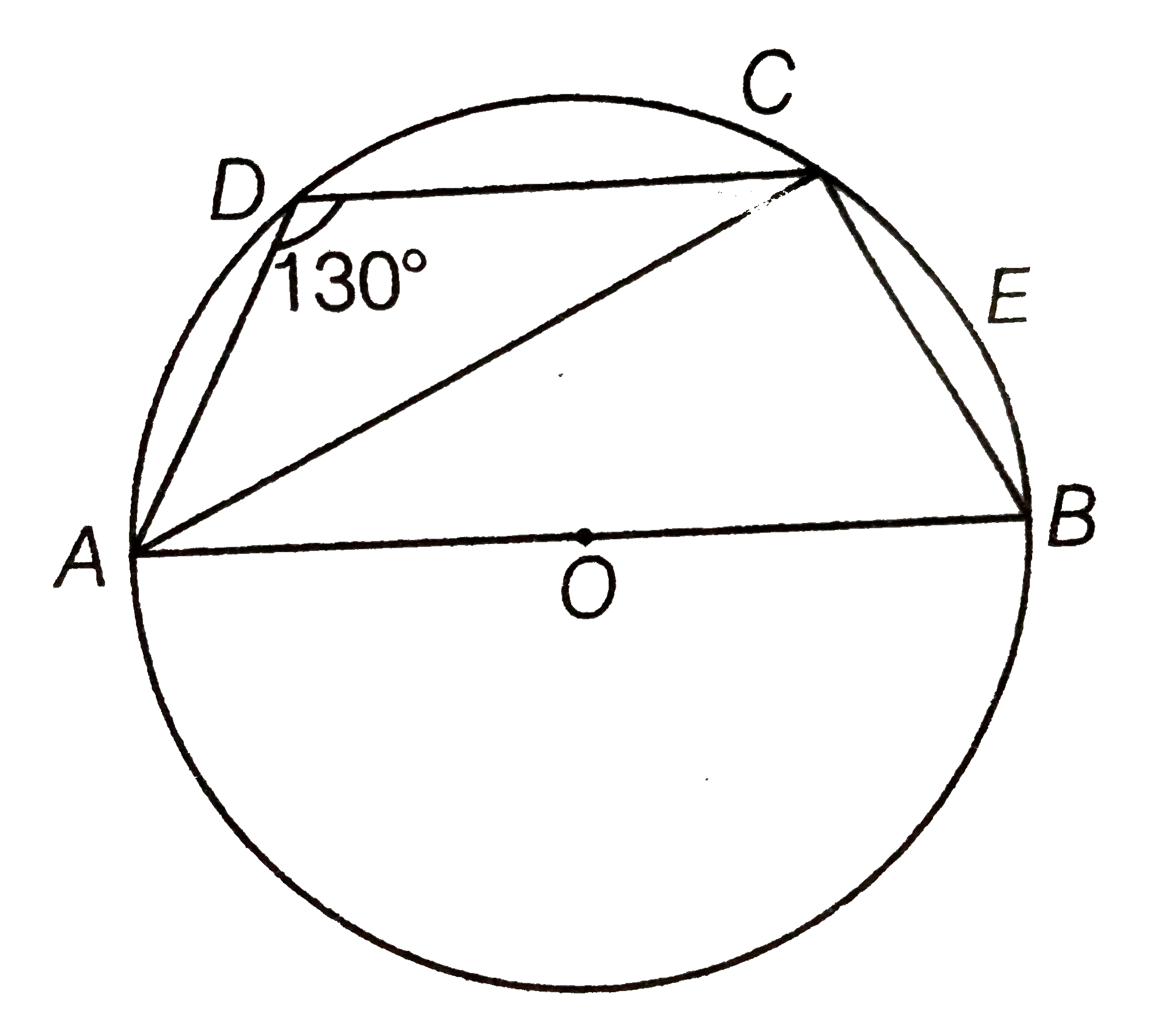
View SolutionIn figure, `angleADC=130^(@)" and chord BC=chord BE. Find "angleCBE`.
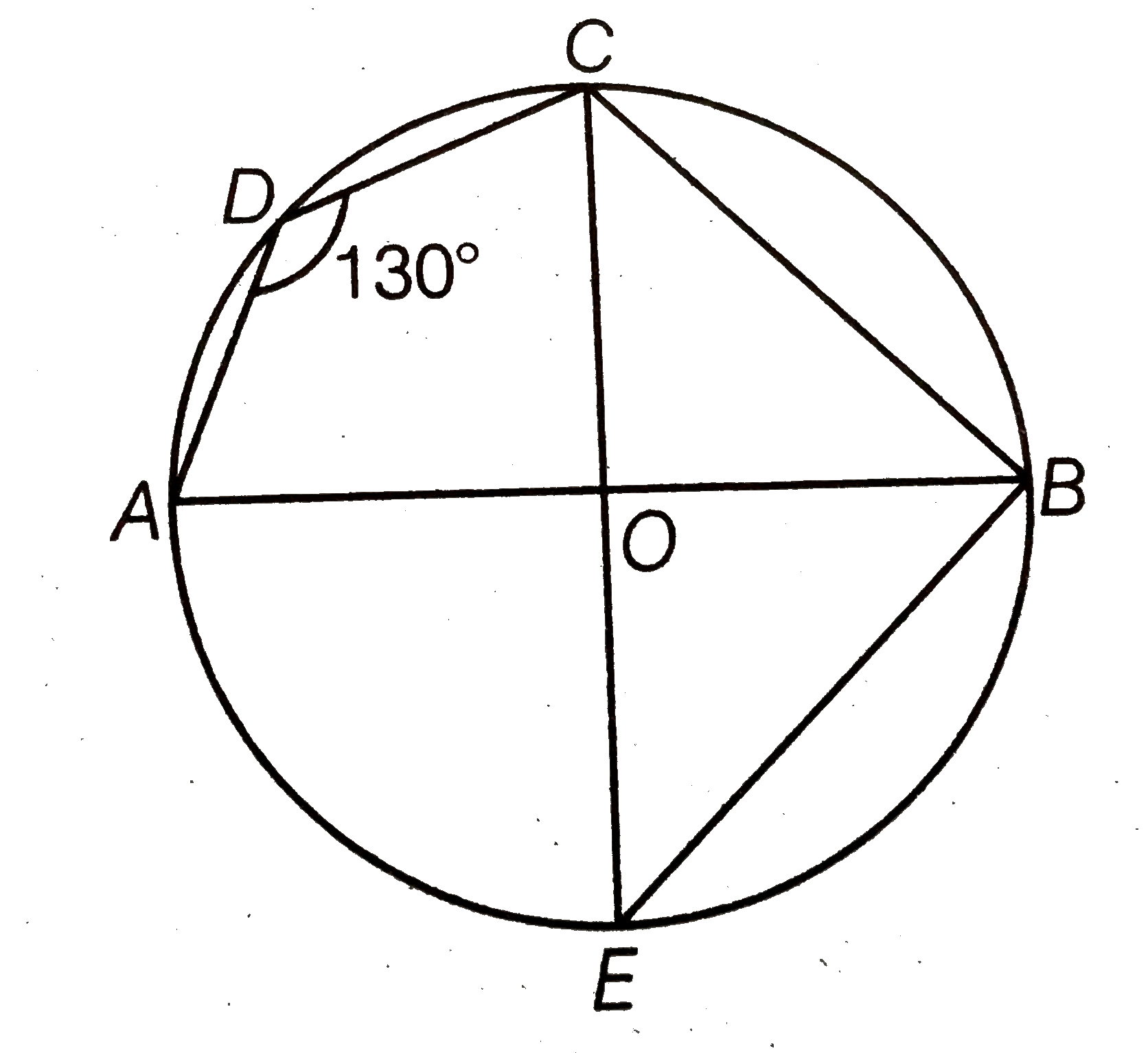
View SolutionIn figure, `angleACB=40^(@) ." Find "angleOAB`.
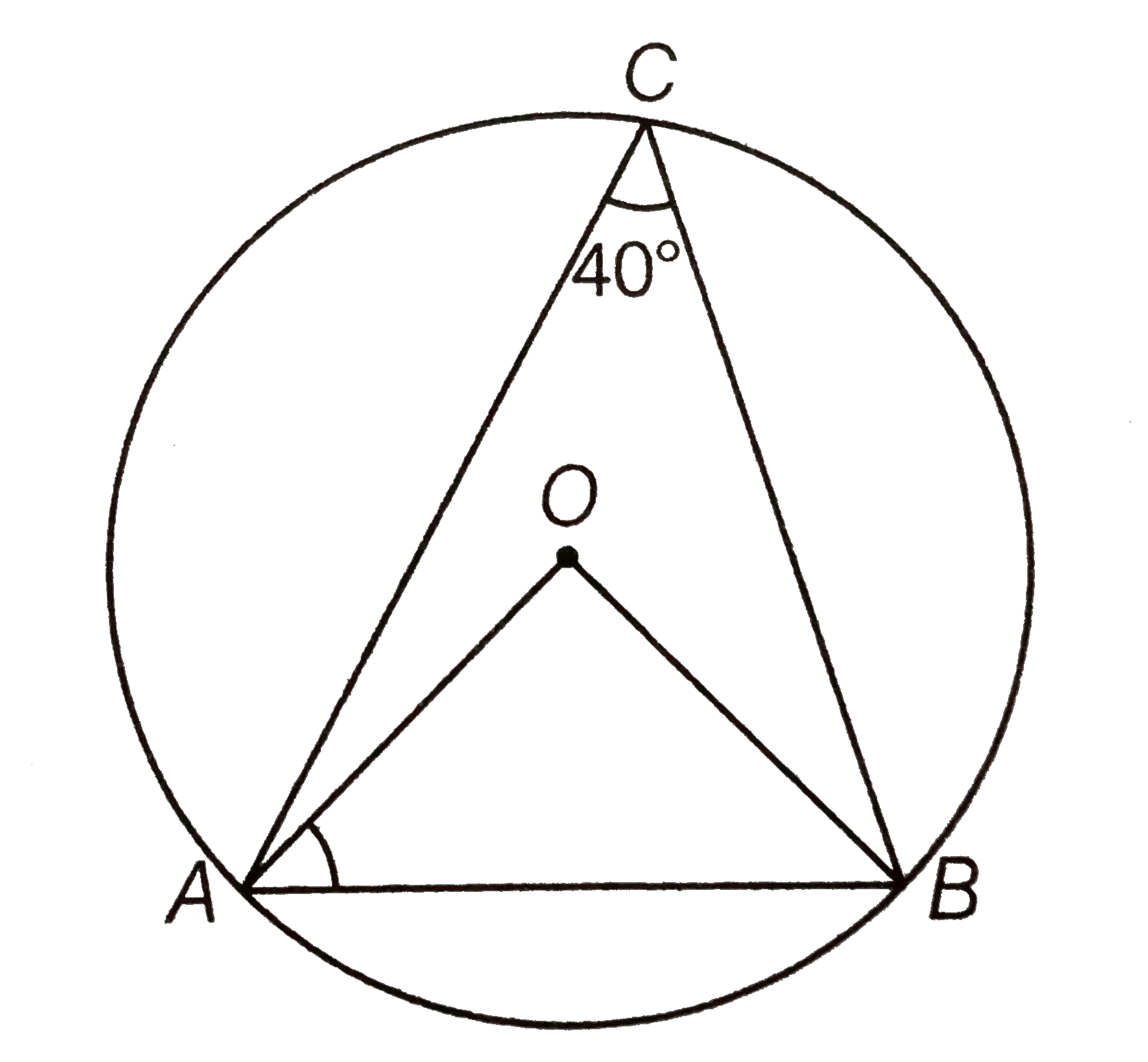
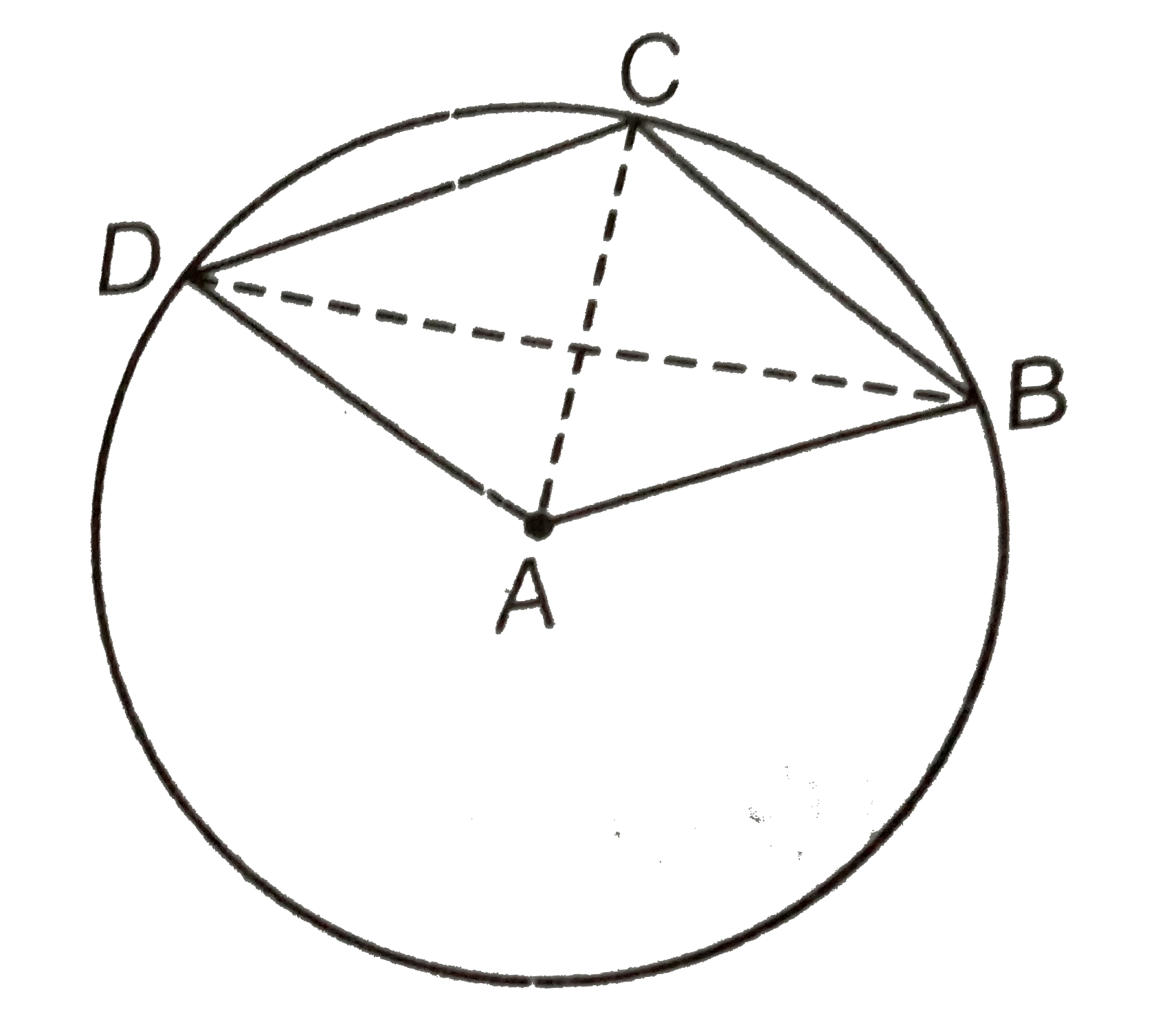
View SolutionIn Figure, `A B C D` is a cyclic quadrilateral whose side `A B` is a diameter of the circle through `A ,\ B ,\ C ,\ Ddot` If `(/_A D C)=130^0,`find `/_B A C`
View SolutionTwo circles whose centres are `O` and `O '` intersect at `Pdot` Through `P ,` a line `l` parallel to `O O '` intersecting the circles at `C\ a n d\ D` is drawn. Prove that `C D=2\ O O '`
View SolutionIn figure, AOB is a diameter of the circle and C, D, E are any three points on the semi-circle. Find the value of `angleACD+ angleBED`.
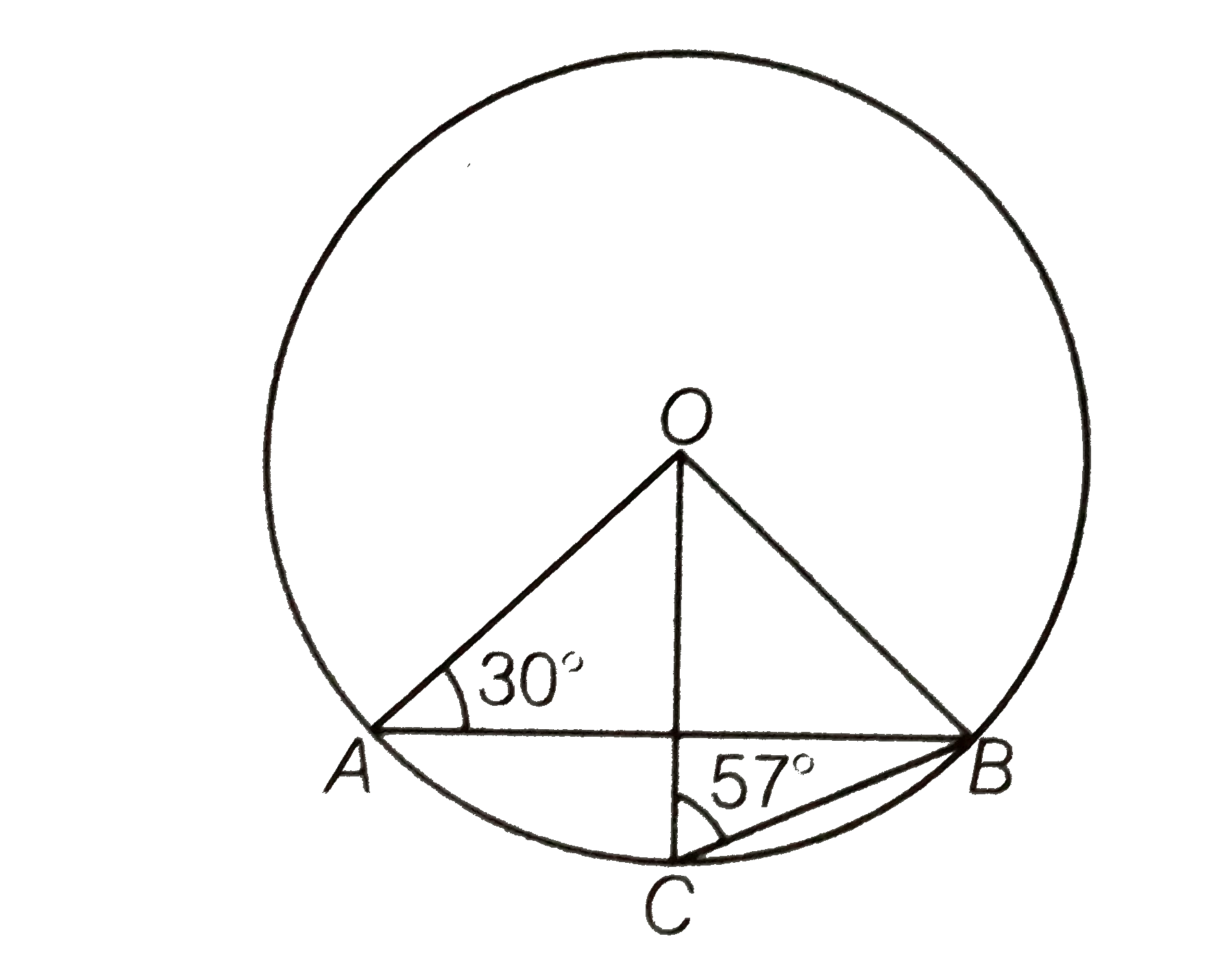
View SolutionIn figure, `angleOAB=30^(@) and angleOCB=57^(@)." Find "angleBOC `
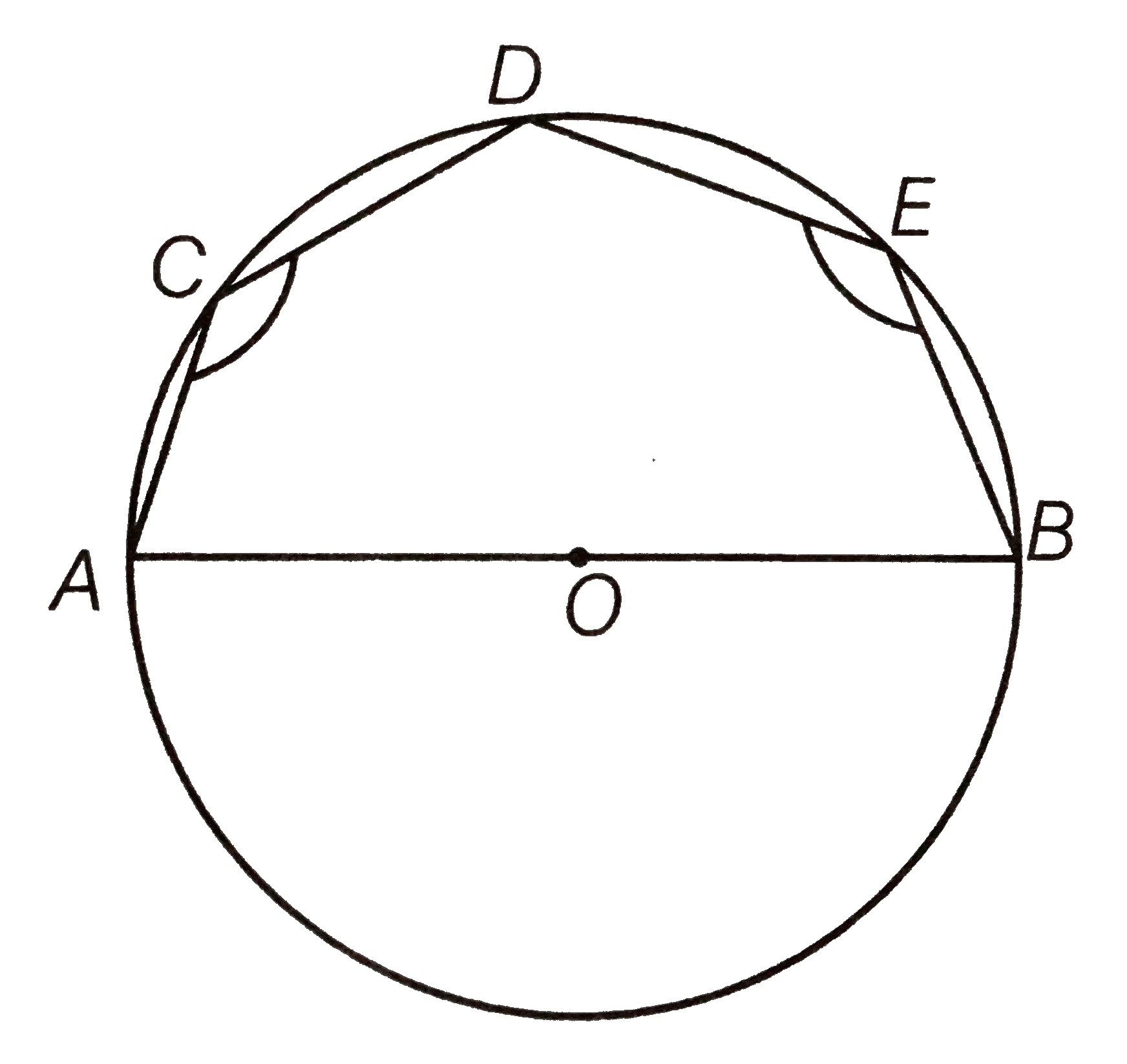
View SolutionIf two equal chords of a circle intersect within the circle, prove that the segments of one chord are equal to corresponding segments of the other chord.
View SolutionIf the non-parallel sides of a trapezium are equal, then
View Solution`P ,\ Q\ a n d\ R` are, respectively, the mid points of sides `B C ,\ C A\ a n d\ A B` of a triangle `A B C`
View SolutionProve that If the bisector of any angle of a triangle and the perpendicular bisector of its opposite side intersect, they will intersect on the circumcircle of the triangle.
View SolutionIf ABC is an equilateral triangle inscribed in a circle and P be any point on the minor arc BC which does not coincide with B or C, then prove that PA is angle bisector of `angleBPC`.
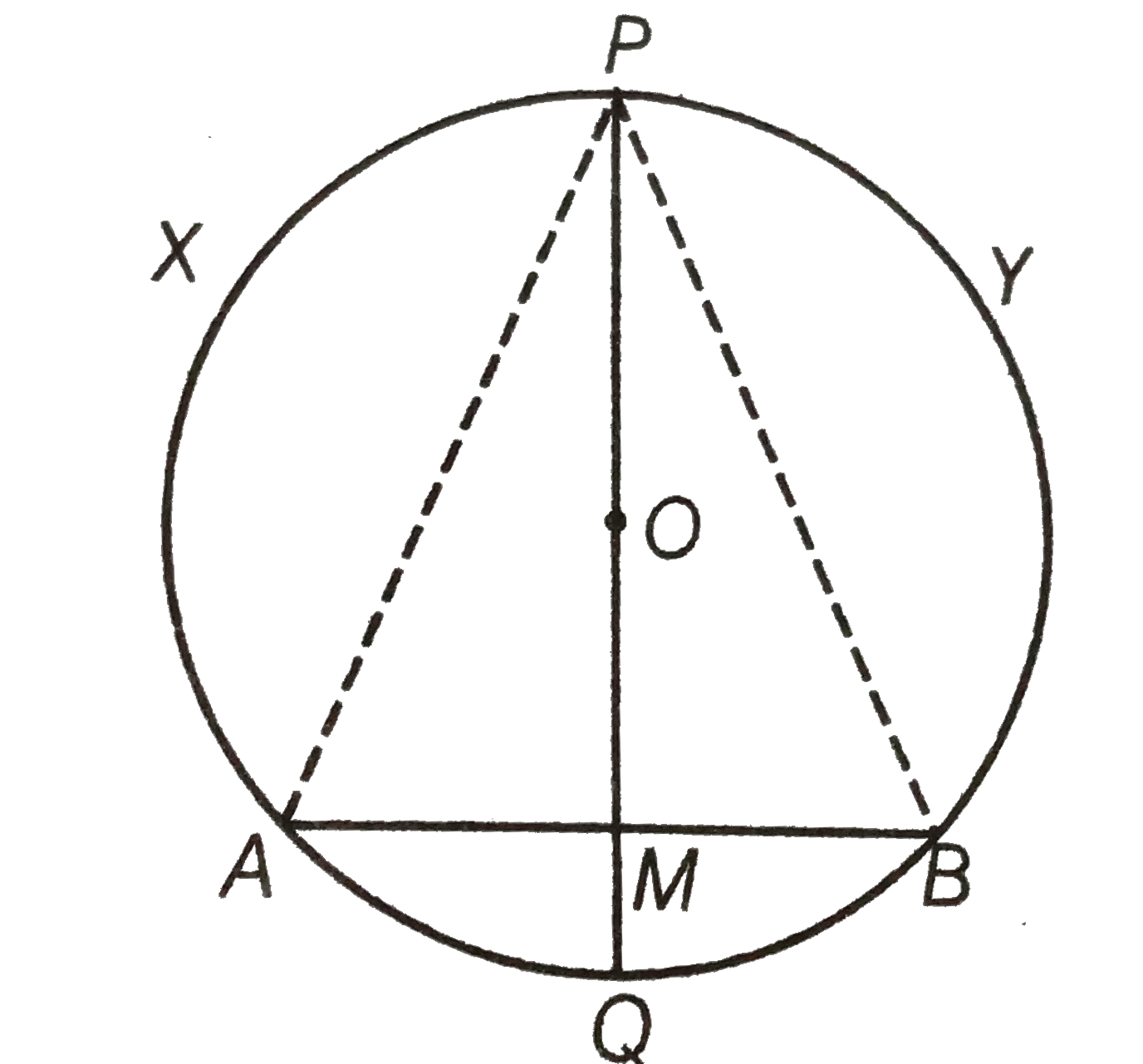
View SolutionIn the figure, AB and CD are two chords of a circle, interacting each other at a point E. Prove that `angle AEC` = `1/2`(angle subtended by arc `CXA`. at the center + angle subtended by arc `DYB` at the center).
View SolutionIf bisectors of opposite angles of a cyclic quadrilateral ABCD intersect the circle, circumscribing it at the points P and Q, prove that PQ is a diameter of the circle.
View SolutionA circle has radius `sqrt2`cm it is divided into 2 segments by a chord of length 2cm prove that angle subtended by the chord at a point in major segment is `45^@`
View Solution`AB and CD` are equal chords of a circle whose centre is O, when produced these chords meet at E,Prove that `EB=ED`.
View SolutionAB and AC are two chords of a circle of radius r such that AB=2AC. If p and q are the distances of AB and AC from the centre Prove that `4q^(2)=p^(2)+3r^(2)`.
View SolutionIn figure, O is the centre of the circle ` angleBCO=30^(@)` . Find X and Y.
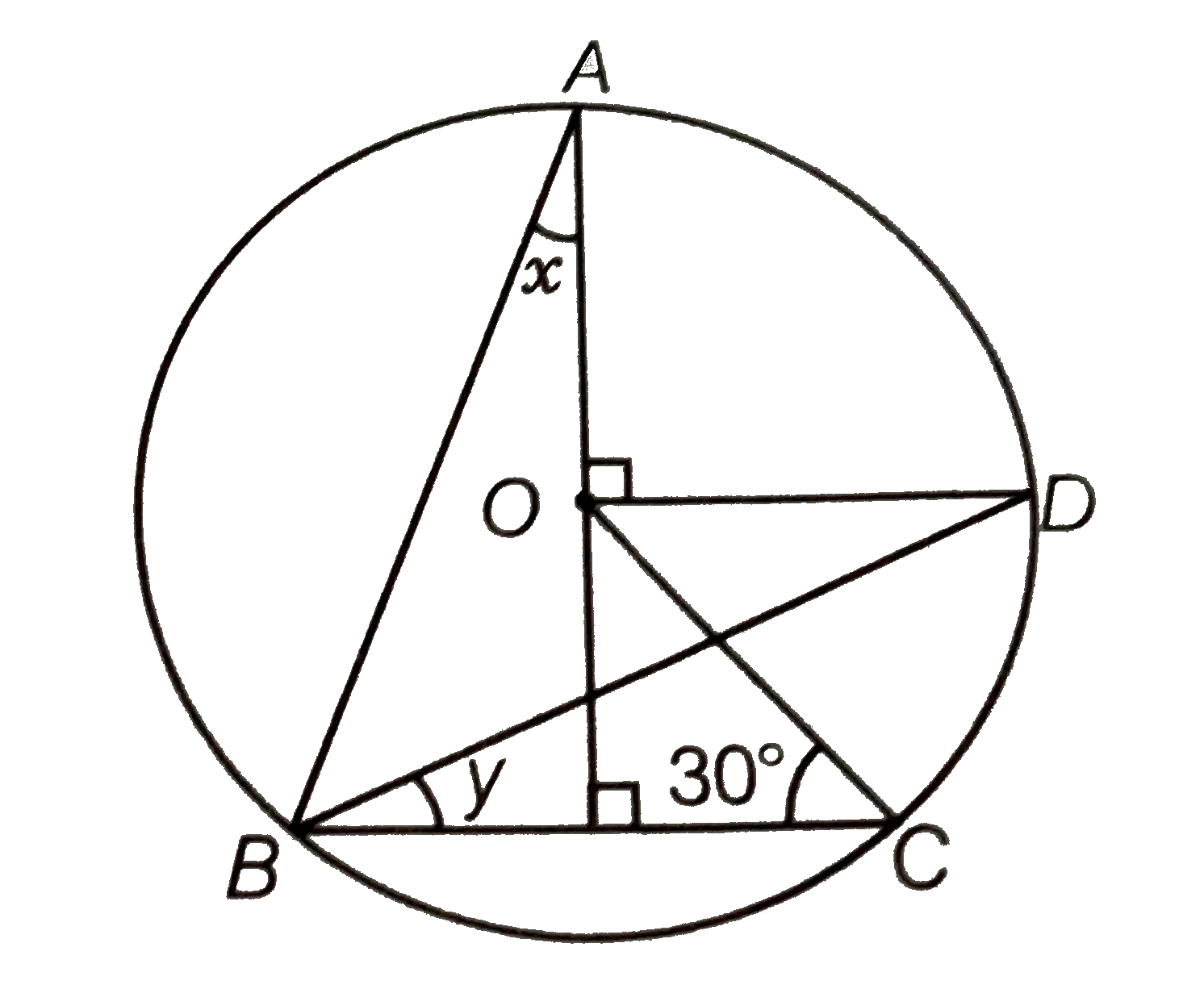
View SolutionIf figure, O is the centre of the circle, `BD=OD and CD bot AB." Find" angleCAB`.
View Solution
Circles questions and complete solutions for chapter CIRCLES of NCERT MATHS (HINGLISH) of Class 9