A uniformly charged thin ring has radius 10.0 cm and total charge +12.0nC. An electron is placed on the ring's axis a distance 25.0cm from the centre of the ring and is constrained to stay on the axis of the ring. The electron is then released from rest, then
(i) describe the subsequent motion of the electron
(ii) find the speed of the electron when it reaches the centre of the ring.
To solve the problem step by step, we will break it down into two parts as requested:
Part (i): Describe the Subsequent Motion of the Electron
1. Understanding the Initial Conditions:
- The electron is placed on the axis of a uniformly charged ring, 25 cm away from the center. The ring has a radius of 10 cm and a total charge of +12 nC.
- The electron is negatively charged and is initially at rest.
2. Forces Acting on the Electron:
- The positively charged ring exerts an attractive force on the negatively charged electron. This force will pull the electron towards the center of the ring.
3. Motion Description:
- Upon release, the electron will start moving towards the center of the ring due to the electrostatic attraction.
- As it moves closer to the ring, it will gain kinetic energy and speed up.
- When the electron reaches the center of the ring, it will have maximum kinetic energy and will continue moving past the center due to inertia.
4. Oscillatory Motion:
- After passing the center, the electron will be attracted back towards the ring and will oscillate about the center point.
- The motion will be periodic, with the electron moving to and fro along the axis of the ring.
Part (ii): Find the Speed of the Electron When It Reaches the Centre of the Ring
1. Using Conservation of Energy:
- The total mechanical energy (potential energy + kinetic energy) of the system remains constant.
- Initially, when the electron is at a distance of 25 cm from the center, its kinetic energy is zero (since it starts from rest).
2. Potential Energy Calculation:
- The potential energy (U) at a distance r from the charge can be calculated using the formula:
U=−k⋅Q1⋅Q2r
- Here, k is Coulomb's constant (9×109N m2/C2), Q1 is the charge of the ring (+12 nC), Q2 is the charge of the electron (-1.6 x 10−19 C), and r is the distance from the center of the ring to the electron.
3. Calculating Initial Potential Energy:
- The initial distance from the center to the electron is 0.25m.
- The potential energy when the electron is at 25 cm:
UA=−(9×109)⋅(12×10−9)⋅(−1.6×10−19)0.25
- Calculate UA.
4. Potential Energy at the Center:
- At the center of the ring, the potential energy UC is zero because the distance to the charge is zero (the potential due to a ring at its center is constant).
5. Applying Conservation of Energy:
- The total energy at point A (initial) equals the total energy at point C (final):
UA+KA=UC+KC
- Since KA=0 (the electron starts from rest), we have:
UA=KC
- The kinetic energy KC at the center is given by:
KC=12mv2
- Where m is the mass of the electron (9.1×10−31kg).
6. Solving for Speed v:
- Rearranging gives:
v=√2|UA|m
- Substitute the calculated value of |UA| and the mass of the electron to find v.
Final Calculation:
- After calculating UA and substituting into the equation for v, we find the speed of the electron when it reaches the center of the ring.
- The electron is placed on the axis of a uniformly charged ring, 25 cm away from the center. The ring has a radius of 10 cm and a total charge of +12 nC.
- The electron is negatively charged and is initially at rest.
- The positively charged ring exerts an attractive force on the negatively charged electron. This force will pull the electron towards the center of the ring.
- Upon release, the electron will start moving towards the center of the ring due to the electrostatic attraction.
- As it moves closer to the ring, it will gain kinetic energy and speed up.
- When the electron reaches the center of the ring, it will have maximum kinetic energy and will continue moving past the center due to inertia.
- After passing the center, the electron will be attracted back towards the ring and will oscillate about the center point.
- The motion will be periodic, with the electron moving to and fro along the axis of the ring.
- The total mechanical energy (potential energy + kinetic energy) of the system remains constant.
- Initially, when the electron is at a distance of 25 cm from the center, its kinetic energy is zero (since it starts from rest).
- The potential energy (U) at a distance r from the charge can be calculated using the formula:
U=−k⋅Q1⋅Q2r
- Here, k is Coulomb's constant (9×109N m2/C2), Q1 is the charge of the ring (+12 nC), Q2 is the charge of the electron (-1.6 x 10−19 C), and r is the distance from the center of the ring to the electron.
- The initial distance from the center to the electron is 0.25m.
- The potential energy when the electron is at 25 cm:
UA=−(9×109)⋅(12×10−9)⋅(−1.6×10−19)0.25
- Calculate UA.
- At the center of the ring, the potential energy UC is zero because the distance to the charge is zero (the potential due to a ring at its center is constant).
- The total energy at point A (initial) equals the total energy at point C (final):
UA+KA=UC+KC
- Since KA=0 (the electron starts from rest), we have:
UA=KC
- The kinetic energy KC at the center is given by:
KC=12mv2
- Where m is the mass of the electron (9.1×10−31kg).
- Rearranging gives:
v=√2|UA|m
- Substitute the calculated value of |UA| and the mass of the electron to find v.
- After calculating UA and substituting into the equation for v, we find the speed of the electron when it reaches the center of the ring.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.1
15 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.2
15 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
Similar Questions
A uniformly charged thin ring has radius 10.0cm and total charge +12.0μC. An electron is placed on the ring's axis a distance 25.0cm from the centre of the ring and is constrained to stay on the axis of the ring. The electron is then released from rest.
a. describe the subsequent motion of the electron
b. find the speed of the electron when it reaches the centre of the ring
View SolutionElectric charge Q is uniformly distributed around a thin ring of radius a. find the potential a point P on the axis of the ring at a distance x from the centre of the ring .

View SolutionA ring of radius R carries a uniformly distributed charge +Q. A point charge −q is placed on the axis of the ring at a distance 2R from the centre of the ring and released from rest. The particle executes a simple harmonic motion along the axis of the ring. State True or False.
View SolutionTwo identicaln thin rings each of radius 10 cm carrying charges 10 C and 5 C are coaxially placed at a distance 10 cm apart. The work done in moving a charge q from the centre of the first ring to that of the second is
View SolutionA circular ring carries a uniformly distributed positive charge .The electric field(E) and potential (V) varies with distance (r ) from the centre of thr ring along its axis as
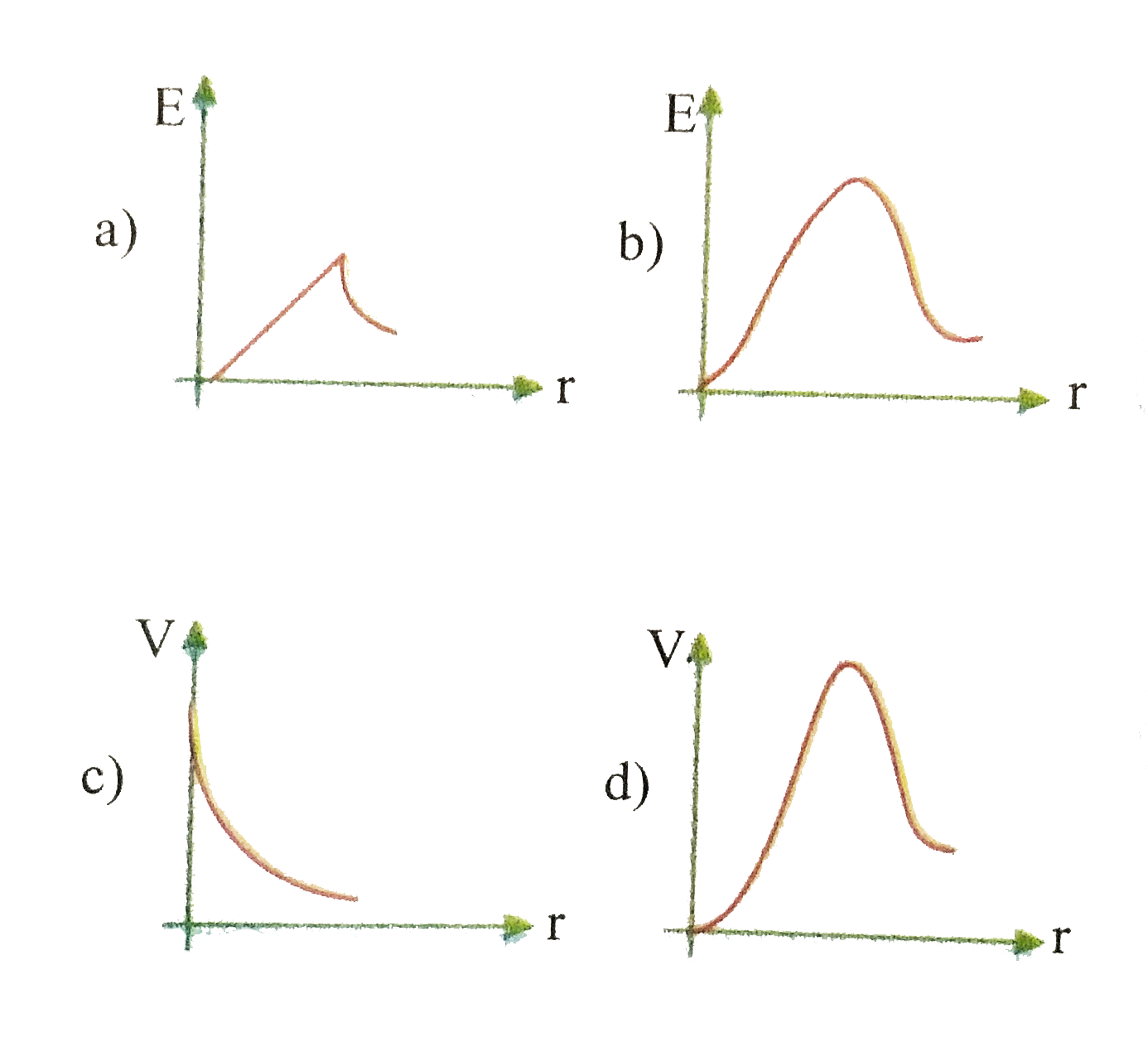
View SolutionFor a uniformly charged ring of radius R, the electric field on its exis has the largest magnitude at a distance h from its centre. Then value of h is :
View SolutionA charge +Q is uniformly distributed over a thin ring of the radius R. The velocity of an electron at the moment when it passes through the centre O of the ring, if the electron was initially at far away on the axis of the ring is (m=mass of electron, K=14πε0)
View SolutionAn electric dipole is kept on the axis of a uniformly charged ring at distance from the centre of the ring. The direction of the dipole moment is along the axis. The dipole moment is p, charge of the ring is Q & radius of the ring is R. The force on the dipole is
View SolutionA circular ring of mass M and radius 'a' is placed in a gravity free space. A small particle of mass m placed on the axis of the ring at a distance √3a from the center of the ring, is released from rest. The velocity with which the particle passes through the center of the ring is
View SolutionConsider a uniformly charged ring of radius R. Find the point on the axis where the electric field is maximum.
View Solution
