The sides PQ, PR of triangle PQR are equal, and S, T are points on PR, PQ such that ∠PSQand∠PTR are right angles
Prove that the triangles PTR and PSQ are congruent.
To prove that triangles PTR and PSQ are congruent, we will use the Angle-Side-Angle (ASA) congruence criterion. Here is a step-by-step solution:
Step 1: Identify the given information
We are given that:
- In triangle PQR, sides PQ and PR are equal (PQ = PR).
- Points S and T are on sides PR and PQ, respectively, such that ∠PSQ and ∠PTR are right angles (90 degrees).
Step 2: Draw the triangle and label the points
Draw triangle PQR with points P, Q, and R. Mark points S on PR and T on PQ. Indicate that ∠PSQ and ∠PTR are right angles.
Step 3: State the angles and sides
From the given information, we can state:
1. PQ = PR (given)
2. ∠PSQ = 90° (given)
3. ∠PTR = 90° (given)
4. ∠P is common to both triangles PTR and PSQ.
Step 4: Apply the ASA criterion
Now, we can apply the ASA criterion for triangle congruence:
- We have one angle (∠P) that is common to both triangles.
- We have two sides (PQ and PR) that are equal.
- We have two right angles (∠PSQ and ∠PTR) that are equal.
Step 5: Conclude the proof
Since we have two angles and the included side equal in both triangles, we can conclude that:
Triangle PTR is congruent to triangle PSQ by the Angle-Side-Angle (ASA) criterion.
Thus, we have proved that triangles PTR and PSQ are congruent.
We are given that:
- In triangle PQR, sides PQ and PR are equal (PQ = PR).
- Points S and T are on sides PR and PQ, respectively, such that ∠PSQ and ∠PTR are right angles (90 degrees).
Draw triangle PQR with points P, Q, and R. Mark points S on PR and T on PQ. Indicate that ∠PSQ and ∠PTR are right angles.
From the given information, we can state:
1. PQ = PR (given)
2. ∠PSQ = 90° (given)
3. ∠PTR = 90° (given)
4. ∠P is common to both triangles PTR and PSQ.
Now, we can apply the ASA criterion for triangle congruence:
- We have one angle (∠P) that is common to both triangles.
- We have two sides (PQ and PR) that are equal.
- We have two right angles (∠PSQ and ∠PTR) that are equal.
Since we have two angles and the included side equal in both triangles, we can conclude that:
Triangle PTR is congruent to triangle PSQ by the Angle-Side-Angle (ASA) criterion.
Topper's Solved these Questions
- CHAPTERWISE REVISION (STAGE 3) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 3) EXERCISE - ISOSCELES TRIANGLES
2 Videos
- CHAPTERWISE REVISION (STAGE 3) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 3) EXERCISE - INEQUALITIES
7 Videos
- CHAPTERWISE REVISION (STAGE 3) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 3) EXERCISE - LOGARITHM
9 Videos
- CHAPTERWISE REVISION (STAGE 1) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 1) EXERCISE - Graphical solution
10 Videos
- CIRCLEBOOK - ICSECHAPTER - CIRCLEEXERCISE - EXERCISE 17(D)
12 Videos
Similar Questions
The sides PQ, PR of triangle PQR are equal, and S, T are points on PR, PQ such that ∠PSQand∠PTR are right angles
If OS and RT intersect at M, prove that the triangles PTM and PSM are congruent.
View SolutionThe sides AB and BC and the median AD of triangle ABC are equal to the sides PQ and QR and the median PM of triangle PQR respectively. Prove that the triangles ABC and PQR are congruent.
View SolutionIn quadrilateral PQRS , PS = QR and ∠SPQ=∠RQP . Prove that :
∠QPR=∠PQS
View SolutionIn two right triangles, one side and an acute angle of one triangle are equal to one side and the corresponding acute angle of the other triangle. Prove that the two triangles are congruent.
View SolutionIn two right triangles, one side and an acute angle of one triangle are equal to one side and the corresponding acute angle of the other triangle. Prove that the two triangles are congruent.
View SolutionIn quadrilateral PQRS , PS = QR and ∠SPQ=∠RQP . Prove that :
PR=QS
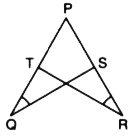
View SolutionThe given figure shows PQ=PRand∠Q=∠R.
Prove that ΔPQS=ΔPRT
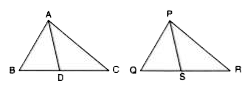
View SolutionIn the following figures, the sides AB and BC and the median AD of triangle ABC are respectively equal to the sides PQ and QR and median PS of the triangle PQR. Prove that ΔABCandΔPQR are congruent.
View SolutionIn a triangle PQR, QR = PR and ∠P=36∘. Which is the largest side of the triangle ?
View SolutionIn two right triangles one side an acute angle of one are equal to the corresponding side and angle of the other. Prove that the triangles are congruent.
View Solution
