Two cells of 1.5 V and 2 V , having internal resistances of 1Ω and 2Ω respectively, are connected in parallel so as to read the current in the same direction through an external resistance of 5Ω . The current in the external resistance will be
The correct Answer is:117A,917A,1017A
To solve the problem of finding the current through the external resistance when two cells are connected in parallel, we can follow these steps:
Step 1: Identify the components and their values
We have two cells:
- Cell 1: EMF (E1) = 1.5 V, Internal Resistance (R1) = 1 Ω
- Cell 2: EMF (E2) = 2 V, Internal Resistance (R2) = 2 Ω
- External Resistance (R3) = 5 Ω
Step 2: Calculate the equivalent EMF (V_AB) and equivalent internal resistance (R_eq)
When cells are connected in parallel, the equivalent EMF (V_AB) can be calculated using the formula:
VABReq=E1R1+E2R2
Where Req is the equivalent internal resistance given by:
1Req=1R1+1R2
Step 3: Calculate the equivalent internal resistance (R_eq)
Using the values:
1Req=11+12=1+0.5=1.5
Thus,
Req=11.5=23Ω
Step 4: Calculate the equivalent EMF (V_AB)
Now, substituting the values into the EMF equation:
VABReq=1.51+22
Calculating the right side:
VABReq=1.5+1=2.5
Now substituting Req:
VAB=2.5×Req=2.5×23=53V
Step 5: Calculate the total current (I) through the external resistance (R3)
Using Ohm's law, the current through the external resistance can be calculated as:
I=VABR3
Substituting the values:
I=535=515=13A
Final Answer
The current in the external resistance will be 13A.
---
We have two cells:
- Cell 1: EMF (E1) = 1.5 V, Internal Resistance (R1) = 1 Ω
- Cell 2: EMF (E2) = 2 V, Internal Resistance (R2) = 2 Ω
- External Resistance (R3) = 5 Ω
When cells are connected in parallel, the equivalent EMF (V_AB) can be calculated using the formula:
Using the values:
Now, substituting the values into the EMF equation:
Using Ohm's law, the current through the external resistance can be calculated as:
The current in the external resistance will be 13A.
Topper's Solved these Questions
- CURRENT ELECTRICITYBook:VMC MODULES ENGLISHChapter:CURRENT ELECTRICITYExercise:PRACTICE EXERCISE-6
Explore 5 Videos
- CURRENT ELECTRICITYBook:VMC MODULES ENGLISHChapter:CURRENT ELECTRICITYExercise:IN-CHAPTER EXERCISE-A
Explore 10 Videos
- CURRENT ELECTRICITYBook:VMC MODULES ENGLISHChapter:CURRENT ELECTRICITYExercise:PRACTICE EXERCISE-3
Explore 4 Videos
- CAPACITORSBook:VMC MODULES ENGLISHChapter:CAPACITORSExercise:JEE Advance ( Archive ) LEVEL 48
Explore 1 Video
- DC CIRCUITBook:VMC MODULES ENGLISHChapter:DC CIRCUITExercise:JEE ADVANCED ARCHIVE
Explore 68 Videos
Similar Questions
Two cells of emfs 1.5 V and 2.0v having internal resistance 0.2Σ and 0.3 Σ respectively are connected in parallel . Calculate the emf and internal resistance of the equivalent cell .
View SolutionTen cells each of emf 1V and internal resistance 1Ω are connected inseries. In this arrangement, polarity of two cells is reversed and the system is connected to an external resistance of 2Ω. Find the current in the circuit.
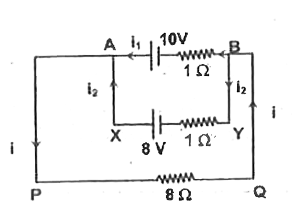
View SolutionTwo cells having emf's of 10 V and 8 V, and internal resistance of 1Ω(each) are connected as shown with an external resistance of 8Ω Find the current flowing through the circuit.
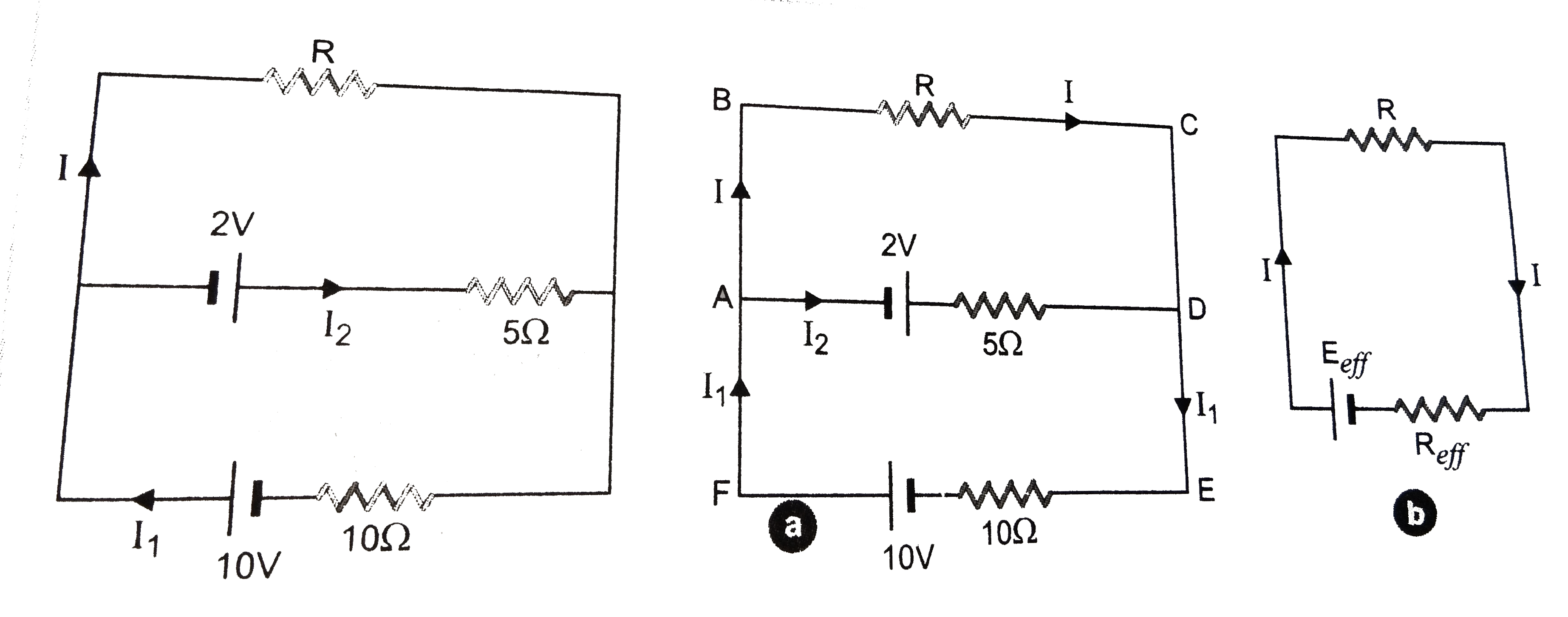
View SolutionTwo cells of voltage 10Vand 2V and internal resistance 10Ω and 5Ω respectively , are connected in parallel with the pesition and of 10V battery connected to negative pole of 2V battery Find the effected voltage and effected resistance of the combination
View Solution24 cells, each of emf 1.5V and internal resistance is 2Ω connected to 12Ω series external resistance. Then,
View SolutionTwo resistors of resistances 2Ω and 6Ω are connected in parallel. This combination is then connected to a battery of emf 2 V and internal resistance 0.5Ω. What is the current flowing through the battery ?
View Solutiontwo heading coils of resistances 10Ωand20Ω are connected in parallel and connected to a battery of emf 12 V and internal resistance 1Ω Thele power consumed by the n are in the ratio
View SolutionTwo batteries of emf 4 V and 8 V with internal resistances 1Ω and 2Ω are connected in a circuit with a resistance of 9Ω as shown in figure. The current and potential difference between the points P and Q are
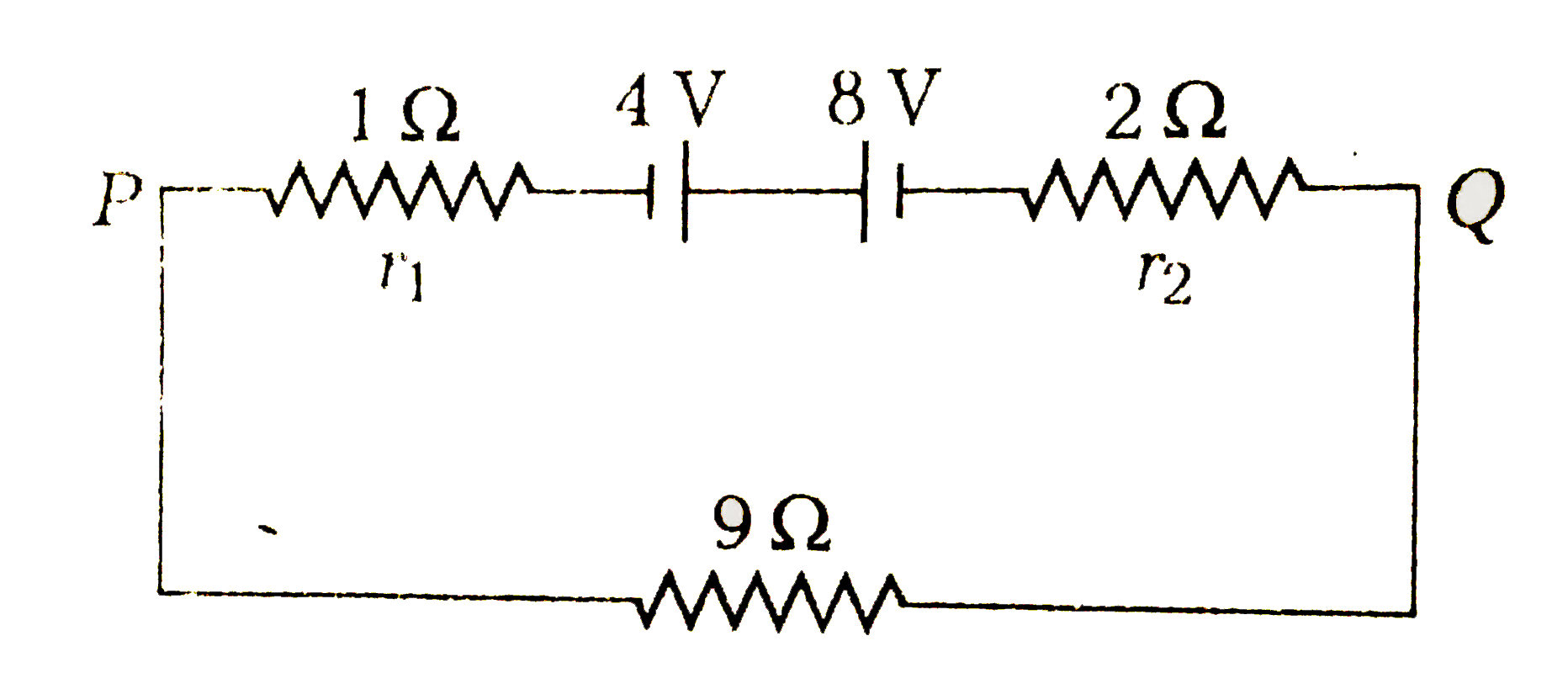
View Solution24 cells of emf 1.5V each having internal resistance of 1 ohm are connected to an external resistance of 1.5 ohms. To get maximum current,
View SolutionA battery of e.m.f. 15 V and internal resistance 3Ω is connected to two resistors 3 Ω and 6 Ω connected in parallel. Find :
the current in 6 Ω resistor.
View Solution