Complete Solutions to Circles of chapter CIRCLES of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | CIRCLES |
| NCERT EXEMPLAR | Circles |
| Number Of Questions | 44 |
| Academic Year | 2023-24 |
If radii of two concentric circles are 4 cm and 5 cm, then length of each chord of one circle which is tangent to the other circle, is
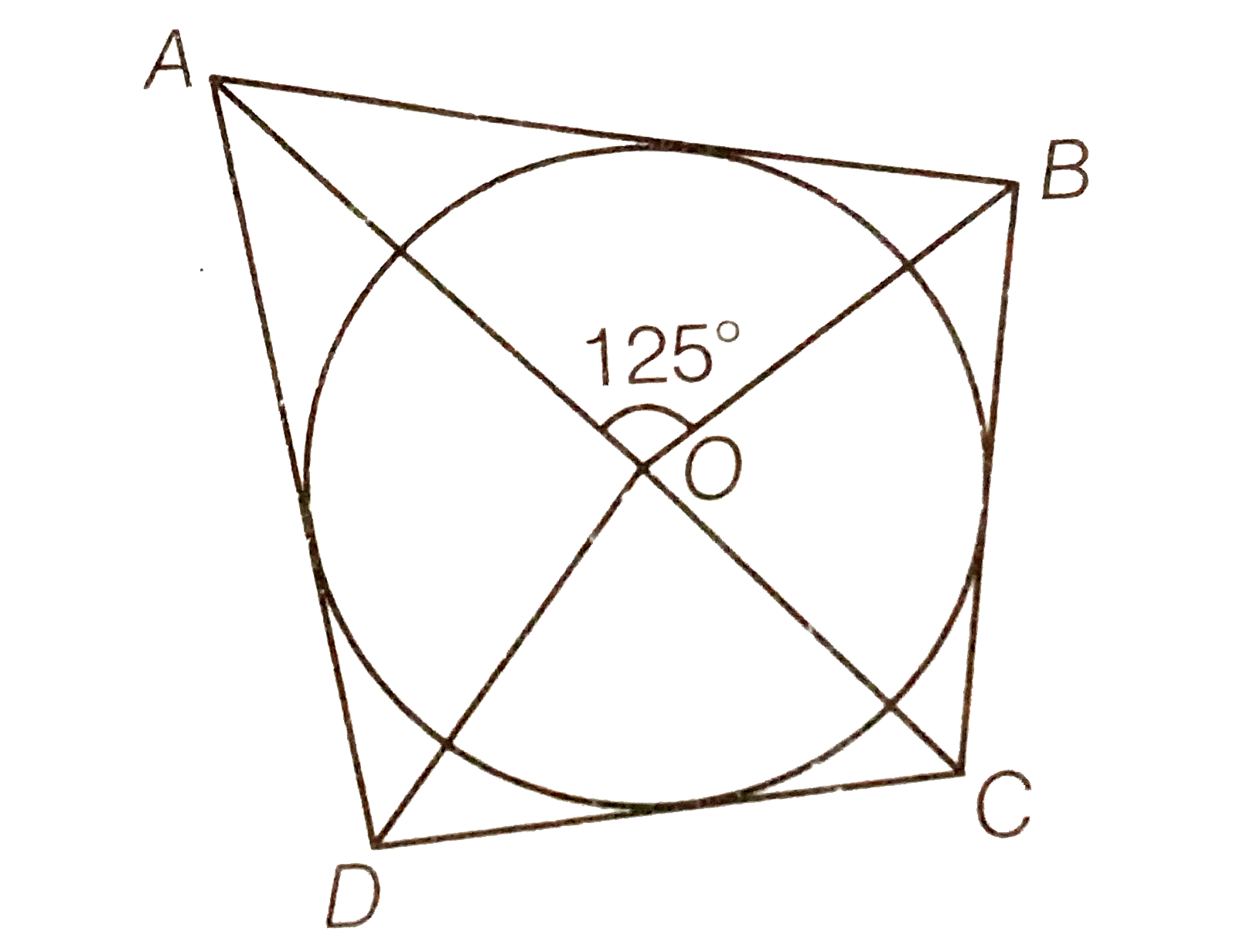
View SolutionIn figure, if `angleAOB=125^(@), then angleCOD` is equal to
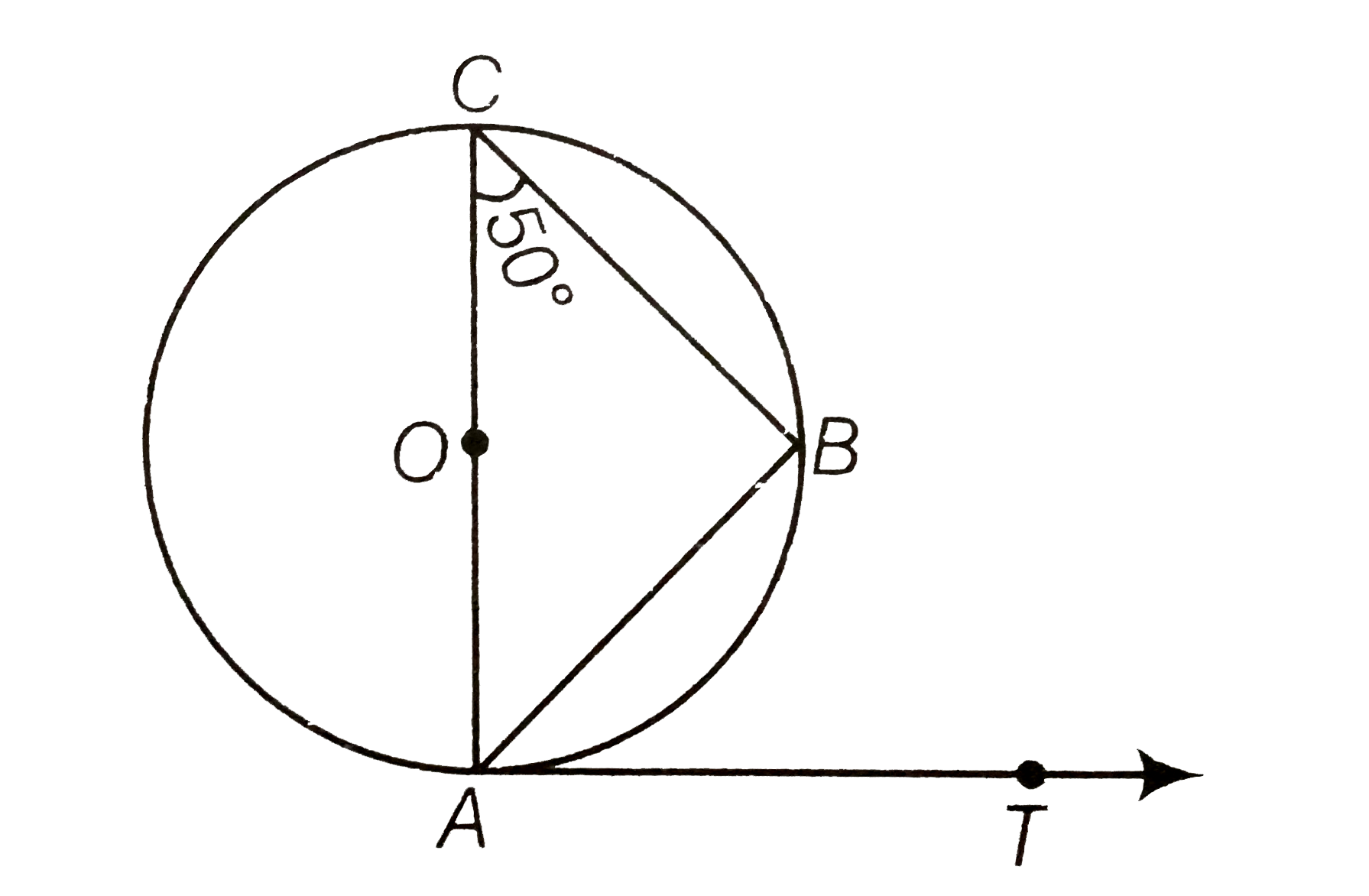
View SolutionIn figure, AB is a chord of the circle and AOC is its diameter such that `angleACB=50^(@)`. If AT is the tangent to the circle at the point A, then `angleBAT` is equal to
View SolutionFrom a point P which is at a distance of 13 cm from the center O of a circle of radius 5 cm, the pair of tangents PQ and PR to the circle is drawn. Then, the area of the quadrilateral POQR is
View SolutionAt one end A of a diameter AB of a circle of radius 5 cm, tangent `XAY` is drawn to the circle. Find the length of the chord `CD` parallel to XY and at a distance 8 cm from A .
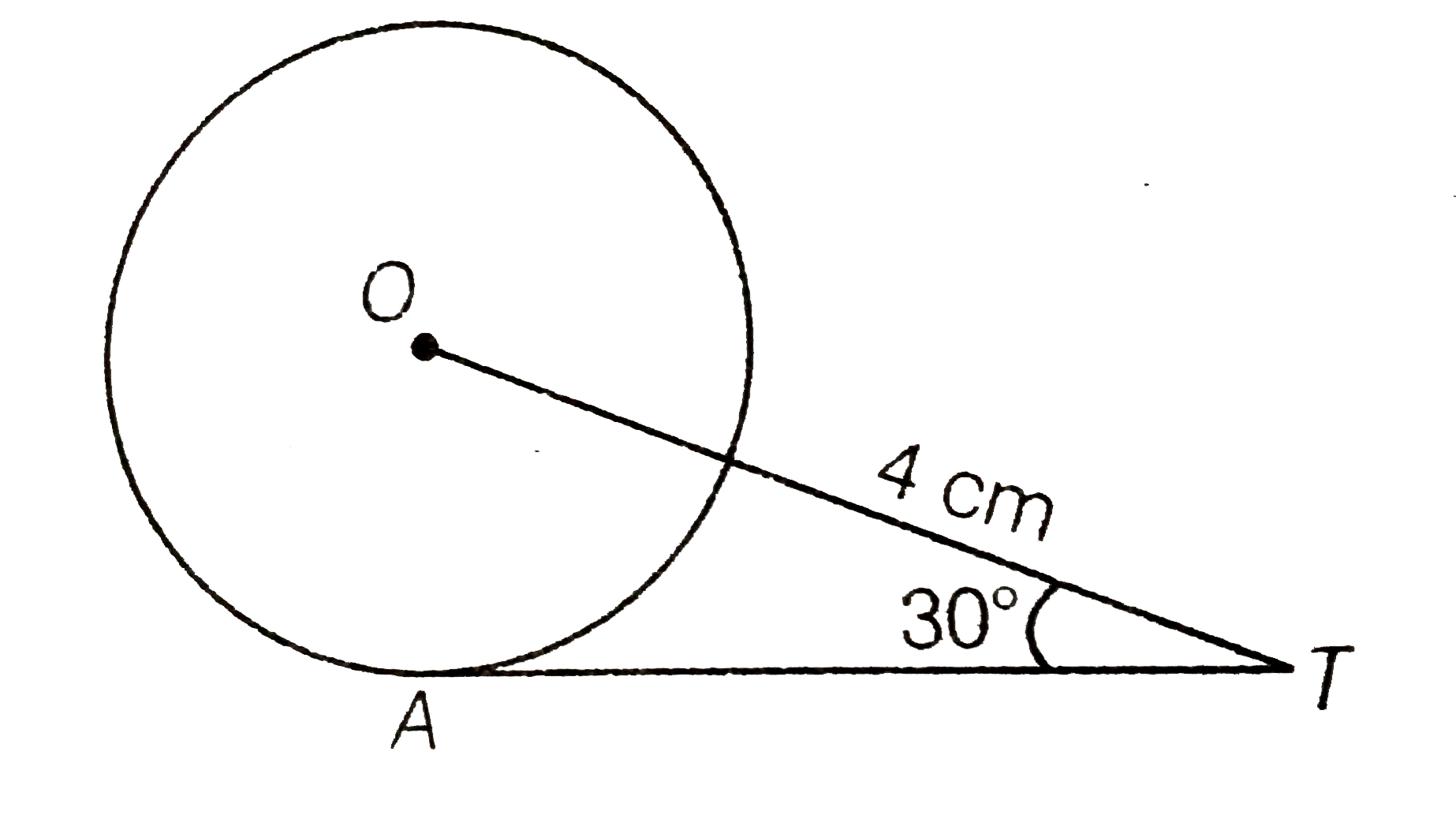
View SolutionIn figure, AT is a tangent to the circle with centre 0 such that OT = 4 cm and `angleOTA=30^(@)`. Then, AT is equal to
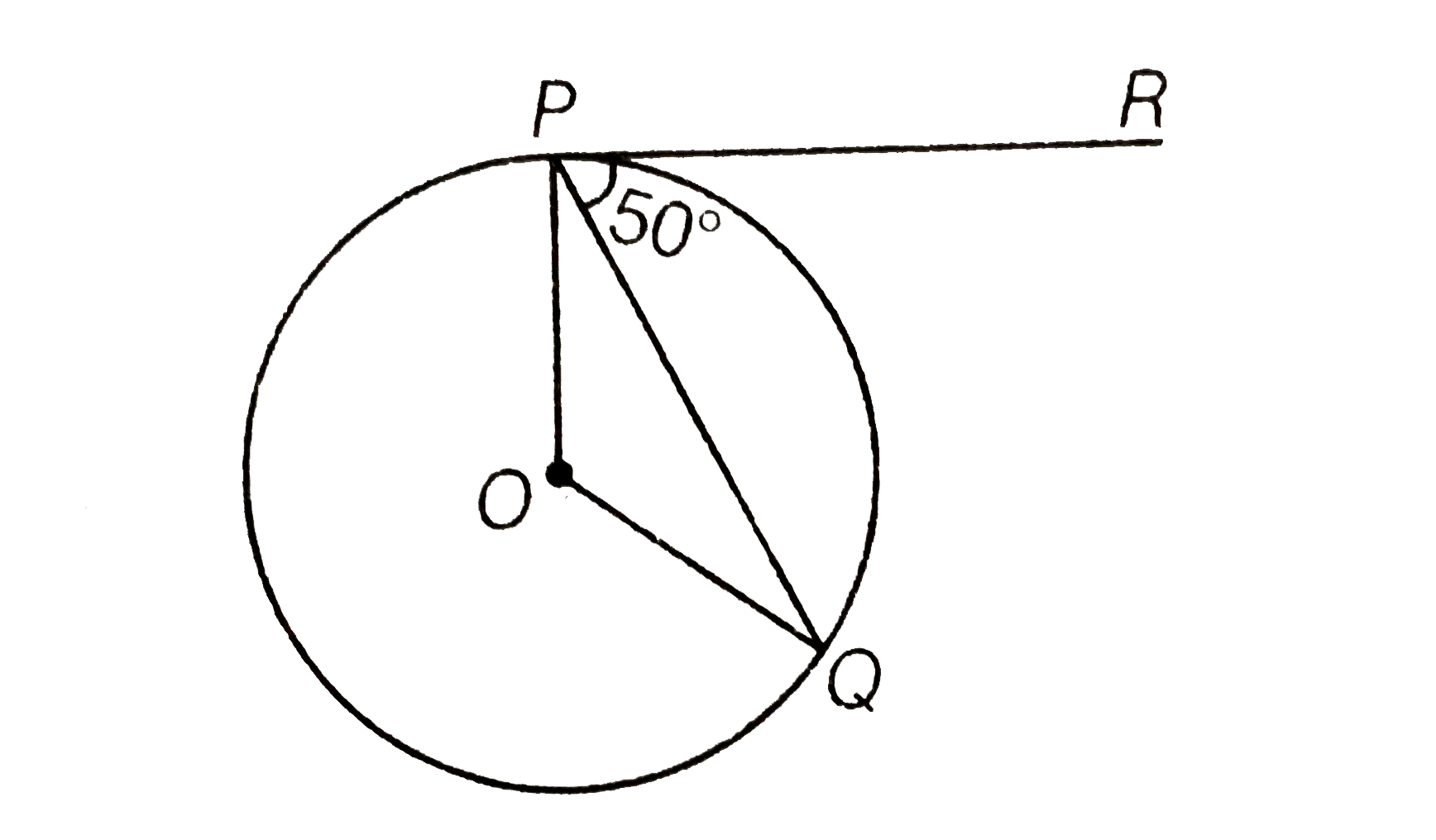
View SolutionIn figure, if `O` is the centre of a circle, PQ is a chord and the tangent PR at P makes an angle of `50^(@)` with PQ, then `anglePOQ` is equal to
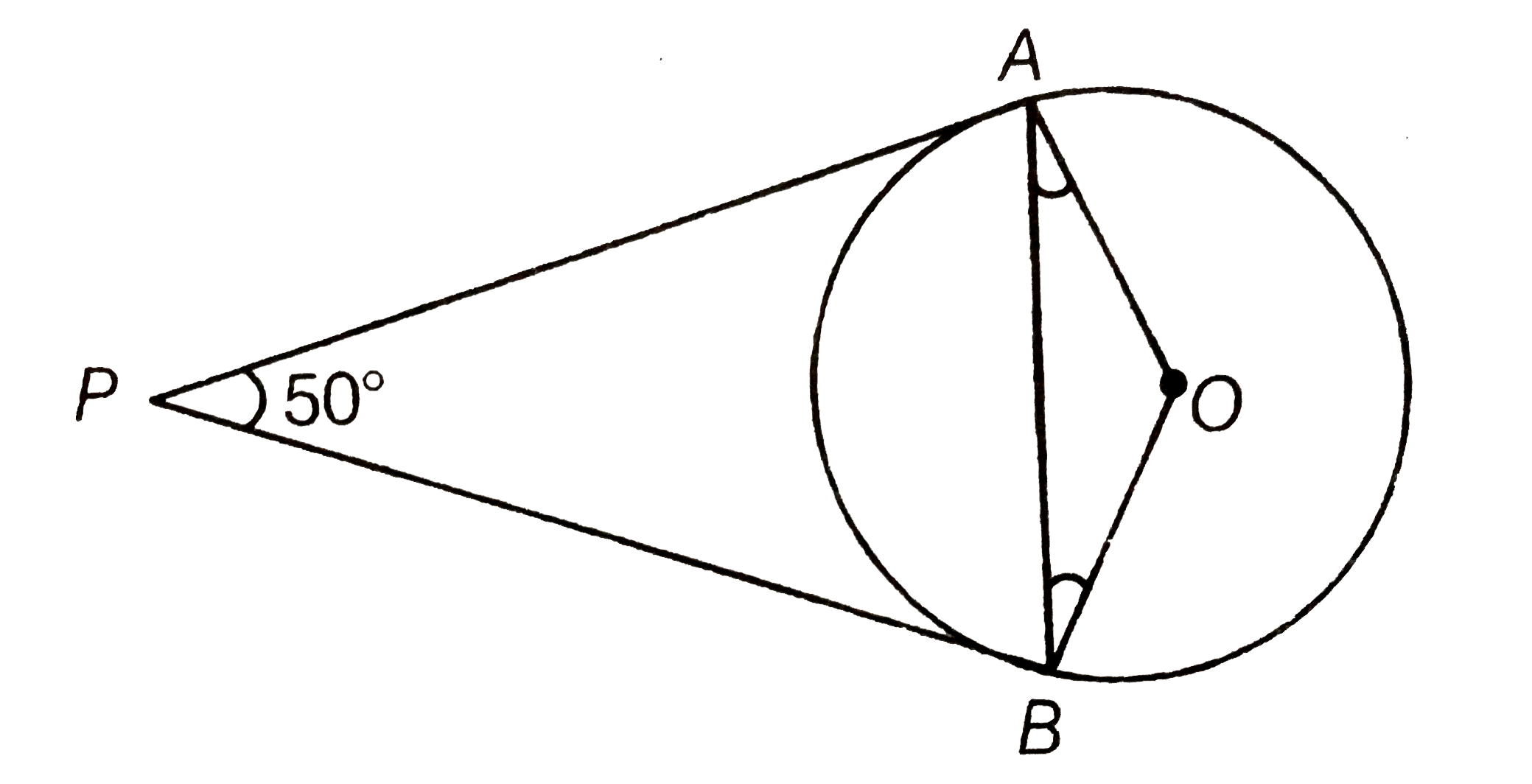
View SolutionIn figure, if `PA` and `PB` are tangents to the circle with centre `O` such that `angleAPB=50^(@)`, then `angleOAB` is equal to
View SolutionIf two tangents inclinced at an angle `60^@` are drawn to a circle of radius 3 cm, then find the length of each tangent.
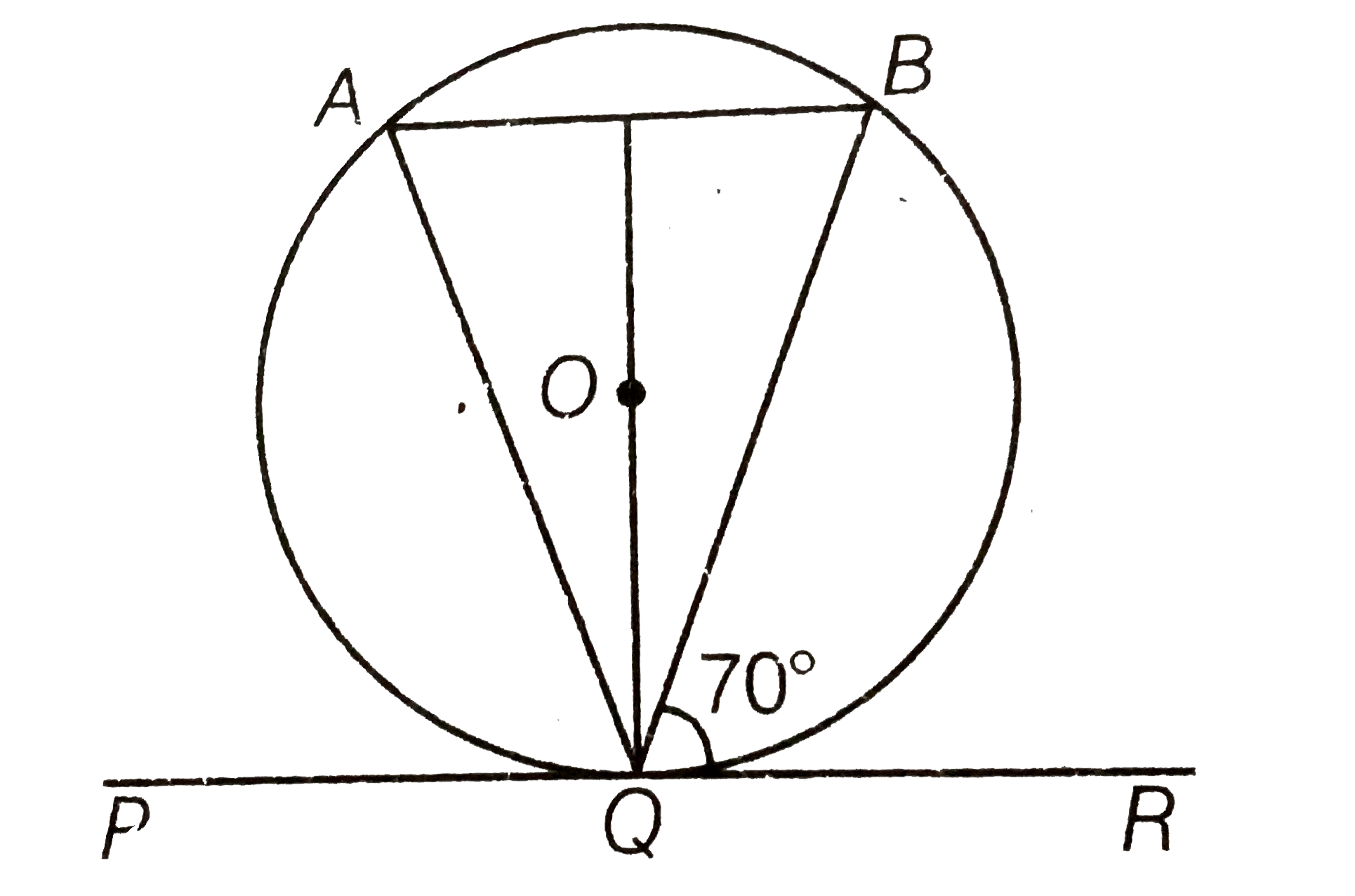
View SolutionIn figure, if PQR is the tangent to a circle at Q whose centre is O, AB is a chord parallel to PR and `angleBQR=70^(@)` then `angleAQB` is equal to
View SolutionIf a chord `AB` subtends and angle of `60^(@)` at the centre of a circle, then the angle between the tangents to the circle drawn from `A` and `B` is
View SolutionThe length of tangent from an external point on a circle is always greater than the radius of the circle.
View SolutionThe length of tangent from an external point P on a circle with centre 0 is always less than OP.
View SolutionThe angle between two tangents to a circle may be `0^(@)`.
View SolutionWrite true or false and state reason
If angle between two tangents drawn from a point P to a circle of radius a and centre 0 is `90^(@)` then `OP=asqrt2`.
View SolutionIf angle between two tangents drawn from a point P to a circle of radius a and centre 0 is `60^(@)` then `OP=asqrt3`.
View SolutionThe tangent to the circumcircle of an isosceles `DeltaABC` at A, in which AB= AC, is parallel to BC.
View SolutionIf a number of circles pass through the end points P and Q of a line segment PQ, then their centres lie on the perpendicular bisector of PQ.
View SolutionIf a number of circles pass through the end points P and Q of a line segment PQ, then their centres lie on the perpendicular bisector of PQ.
View SolutionAB is a diameter of a circle and AC is its chord such that `angleBAC=30^(@)`. If the tangent at C intersects AB extended at D, then BC=BD.
View SolutionOut of the 2 concentric circle the radius of the outer circle is 5 cm and the chord AC of the length 8 cm is a tangent to the inner circle find the radius of the inner circle
View SolutionTwo tangents PQ and PR are drawn from an external point to a circle with centre 0. Prove that QORP is cyclic quadrileral.
View SolutionProve that the centre of a circle touching two intersecting lines lies on the angle bisector of the lines.
View SolutionIf from an external point B of a circle with centre O, two tangents BC and BD are drawn such that `angleDBC=120^(@)`, prove that `BC+BD=BO` i.e., BO=2BC.
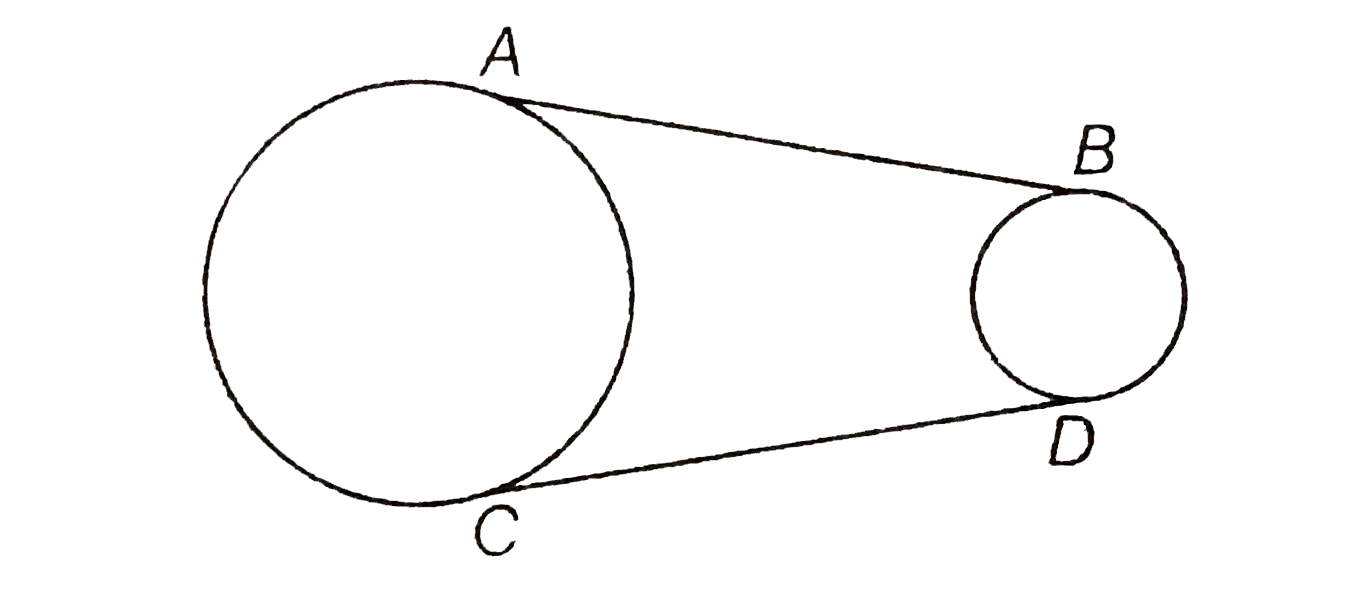
View SolutionIn figure, AB and CD are common tangents to two circles of unequal radii. Prove that AB=CD
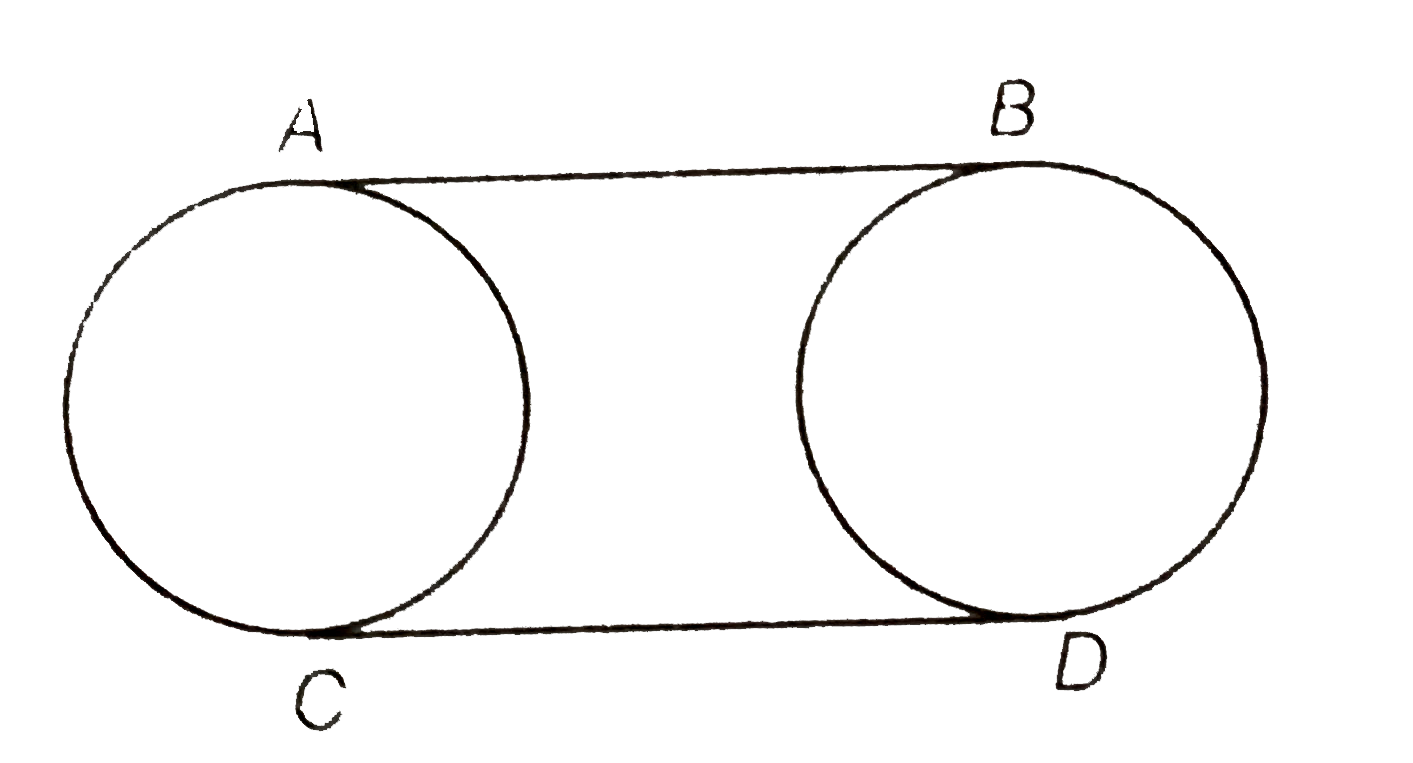
View SolutionIn figure, AB and CD are common tangents to two circles of equal radii. Prove that AB=CD.
View SolutionIn figure, common tangents AB and CD to two circles intersect at E. Prove that AB=CD.
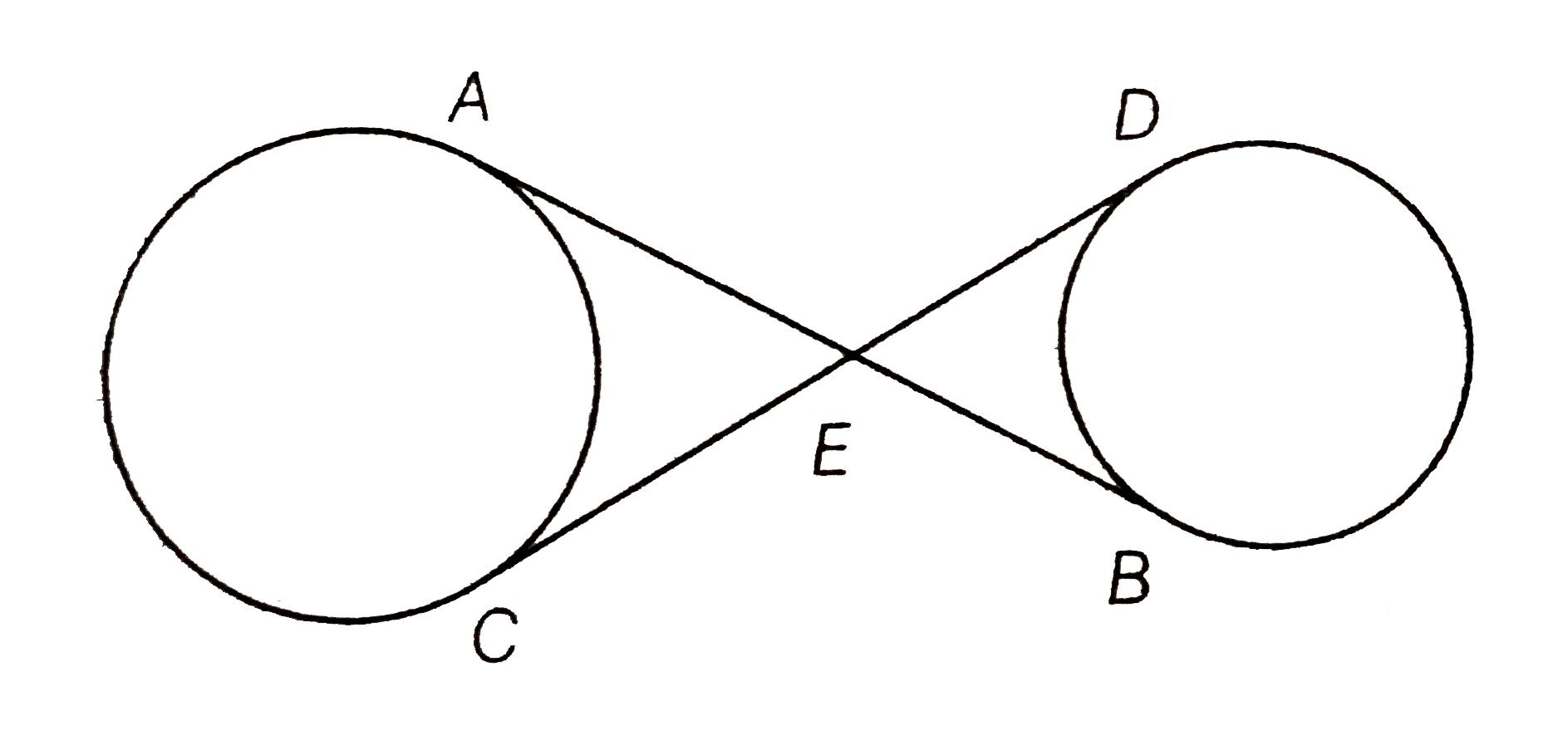
View SolutionA chord PQ of a circle is parallel to the tangent drawn at a point R of the circle,Prove that R bisects the arc `PRQ.`
View SolutionProve that the tangents drawn at the end points of a chord of a circle make equal angles with the chord.
View SolutionProve that a diameter AB of a circle bisects all those chords which are parallel to the tangent at the point A.
View SolutionIf a hexagon ABCDEF circumscribe a circle, prove that `AB + CD + EF=BC+DE+FA`
View SolutionLet's denotes the semi-perimeter of a `DeltaABC` in which BC=a, CA=b and AB=c. If a circle touches the sides BC, CA, AB, at D, E, F, respectively. Prove that BD=s-b.
View SolutionFrom an external point P, two tangents, PA and PB are drawn to a circle with centre 0. At one point E on the circle tangent is drawn which intersects PA and PB at C and D, respectively. If PA=10 cm, find the perimeter of the triangle PCD.
View SolutionIf AB is chord of a circle with centre O, AOC is a diameter and AT is the tangent at A as shown in figure. Prove that `angleBAT=angleACB.`
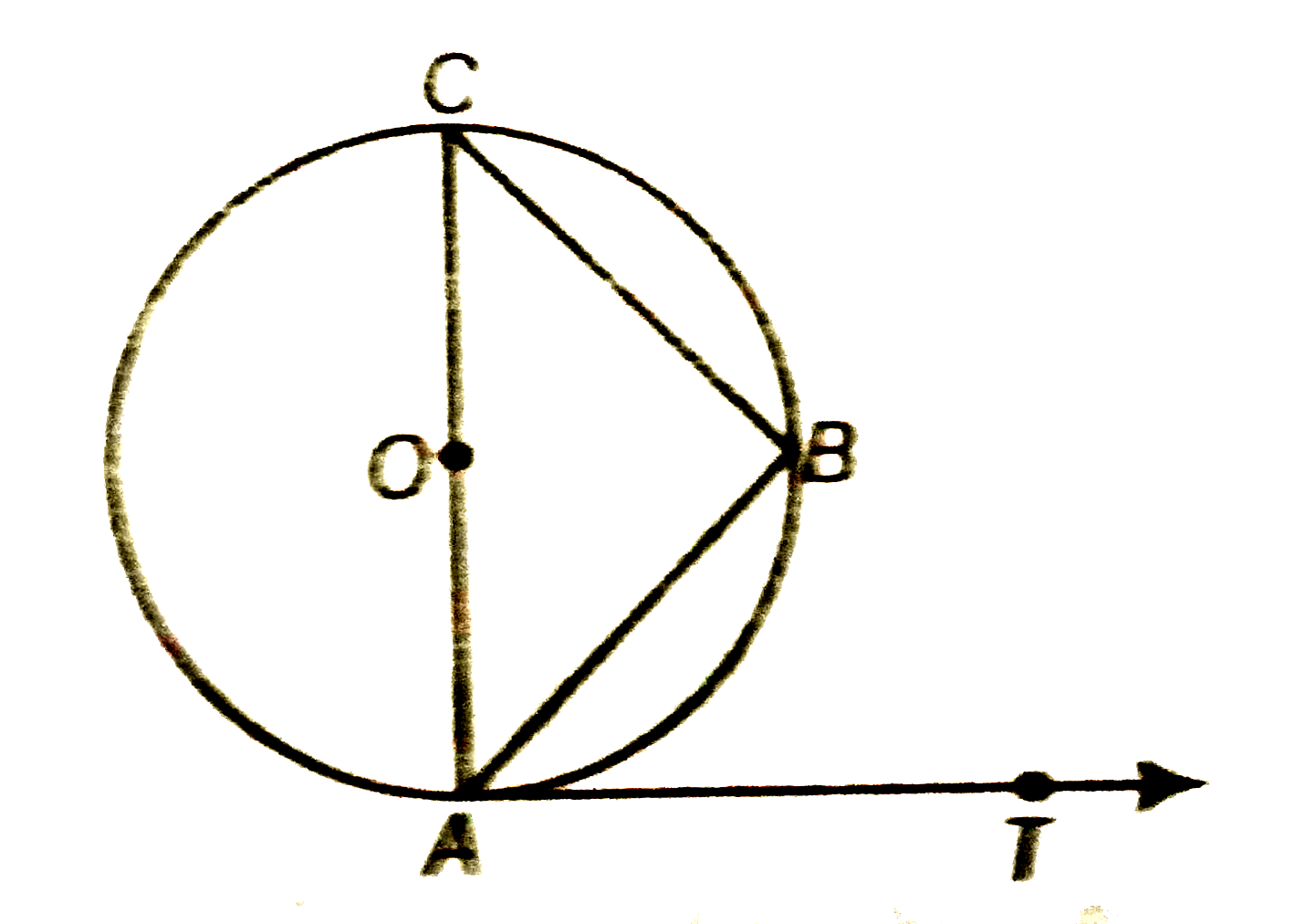
View SolutionTwo circles with centers O and O' of radii 6cm and 8 cm respectively intersect two points P and Q such that OP and O'P are tangents to the two circle. The length of the common chord PQ is
View SolutionIn a right angle triangle `Delta ABC` is which `/_ B = 90^@` a circle is drawn with AB diameter intersecting the hypotenuse AC at P.Prove that the tangent to the circle at PQ bisects BC.
View SolutionIn figure, tangents PQ and PR are drawn to a circle such that `angleRPQ=30^(@)`. A chord RS is drawn parallel to the tangent PQ. Find the `angleRQS`.
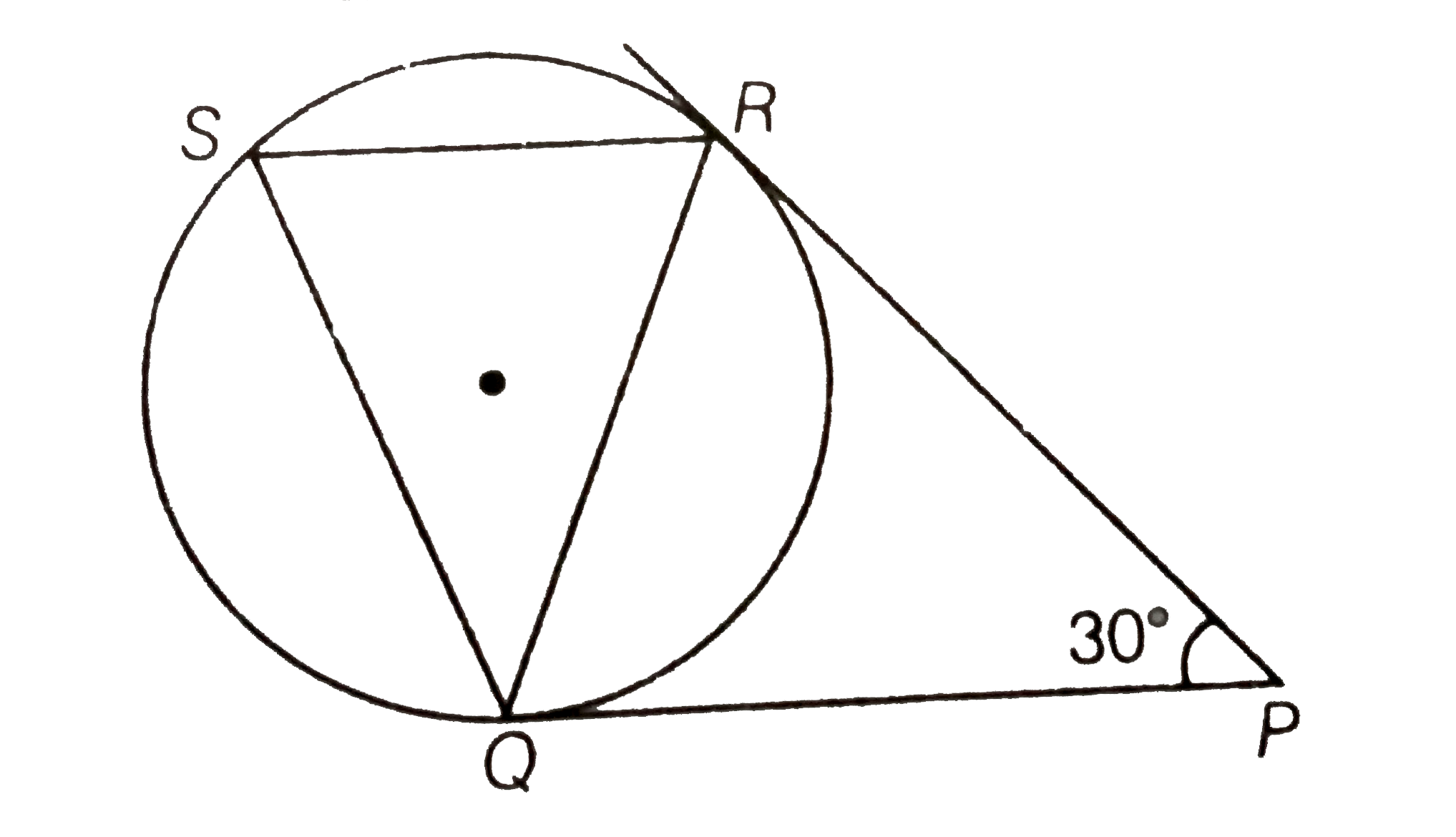
View SolutionAB is a diameter of a circle and AC is its chord such that `angleBAC=30^(@)`. If the tangent at C intersects AB extended at D, then BC=BD.
View Solution. Prove that the tangent drawn at the mid-point of an arc of a circle is parallel to the chord joining the end points of the arc.
View SolutionIn a figure the common tangents, AB and CD to two circles with centers O and O' intersect at E. Prove that the points O, E and O' are collinear.
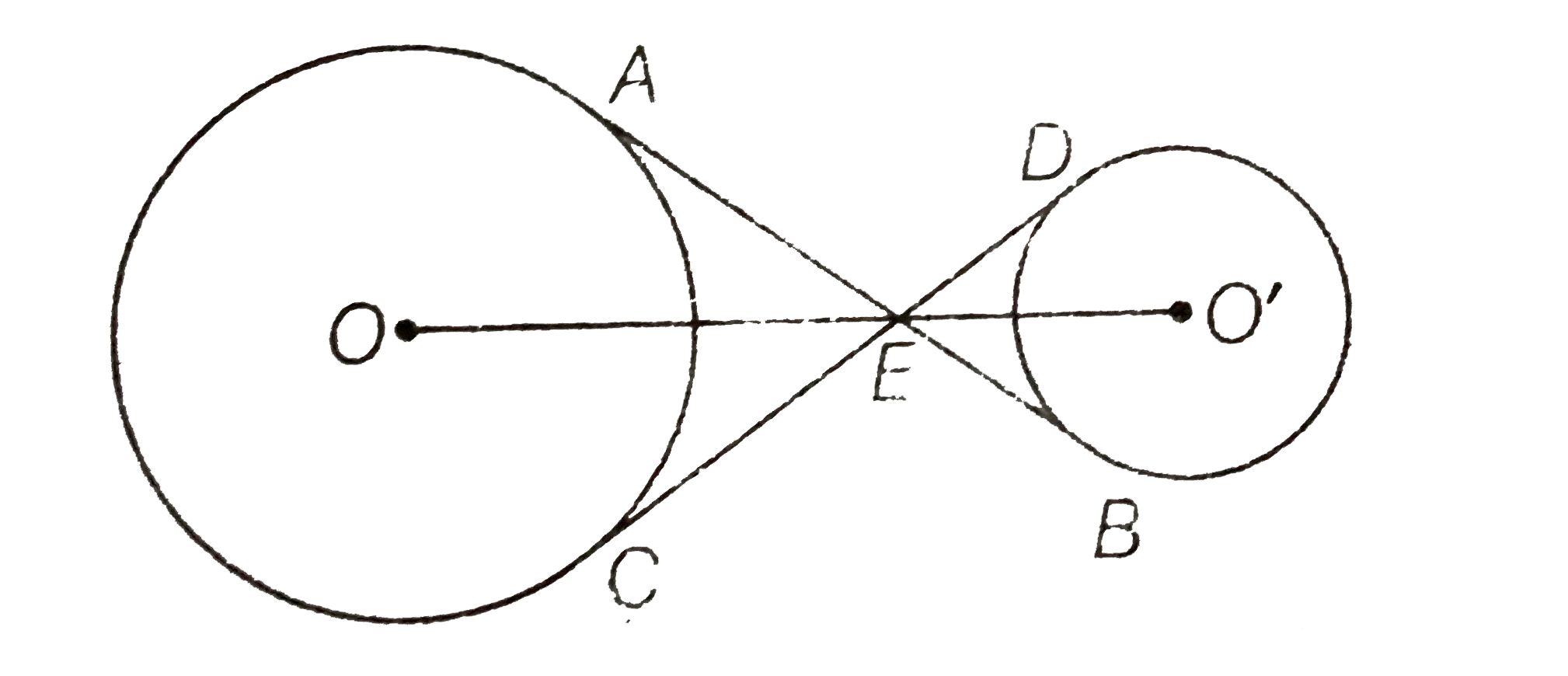
View SolutionType V: O is the center of the circle of radius 5cm. T is a point such that OT=13cm and OT intersects the circle at E . If AB is the tangent to the circle at E; find the length of AB.
View SolutionThe tangent at a point C of a circle and a diameter AB when extended intersect at P. If `anglePCA=110^(@) "find" angleCBA`.
View SolutionIf an isosceles triangle `A B C` in which `A B=A C=6c m` is inscribed in a circle of radius `9c m ,` find the area of the triangle.
View SolutionA is a point at a distance 13 cm from the centre O of a circle of radius 5 cm. AP and AQ are the tangents to the circle at P and Q. If a tangent BC is drawn at a point R lying on the minor arc PQ to intersect AP at B and AQ at C, find the perimeter of the `Delta ABC`
View Solution
Circles questions and complete solutions for chapter CIRCLES of NCERT EXEMPLAR MATHS (HINGLISH) of Class 10