Complete Solutions to Example of chapter ELECTROSTATIC POTENTIAL AND CAPACITORS of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | ELECTROSTATIC POTENTIAL AND CAPACITORS |
| DC PANDEY NEET | Example |
| Number Of Questions | 89 |
| Academic Year | 2023-24 |
How much work will be done in bringing a charge of `400 muC` from infinity to some point P in the region of electric field ? Given that the electric potential at point P is 20 V ?
View SolutionFind the work done by some external force in moving a charge `q=2muC` from infinity to a point where electric potential is `10^4` V.
View SolutionThe electric potential at point `A` is `20 V` and at `B` is `-40 V`. Find the work done by an external and electrostatic force in moving an electron slowly from B to A.
View SolutionDetermine the potential at a point 0.50 m (i) from `a+20 muC` potential charge (ii) from `a-20 muC` point charge.
View SolutionWhat is the electrostatic potential at the surface of a silver nucleus of diameter 12.4 fermi ? Atomic number silver is 47.
View SolutionThree point charge `q_1=1muC, q_2=-2muC` and `q_3=3muC` are placed at `(1m, 0,0), (0,2m,0)` and `(0,0,3m)` respectively. Find the electric potential at as origin.
View SolutionIn the given figure, there are four point charges placed at the vertices of a square of side, `a=1.4 m`. If `q_(1)=+18 nC,q_(2)=-24 nC,q_(3)+35nC and q_(4)=+16 nC`m then find the electric at the centre P of the square Assume the potential to be zero at infinity.

View SolutionSuppose that three point charges, `q_(a),q_(b) and q_(c)` are arranged at the vertices of a right-angled triangle, as shown. What is the absolute potential at the position of the third charge, if `q_(a)= -6.0 muC,q_(b) = +4.0 muC,q_(c) = +2.0 muC,a=4.0 m and b=3.0 m` ?

View Solution(a) The charges each `Q` are placed at `x - d , 2d , 4d ,…oo`. Find electric potential at origin `O`.
(b) A charge `+Q` is placed at each of the points `x = d , x = 3d ,x = 5d ,…..,oo` on the `x`-axis , and a charge `-Q` is placed at each of the points `x = 2d , x = 4d , x= 6d , ...,oo`.Find the electric potential at the origin `O`.
View SolutionFind out the points on the line joining two charges `+qand -3q`(kept at as distance of `1.0 m`) where electric potential is zero.
View SolutionTwo charges `3 xx 10^(-8) C` and `-2 xx 10^(-8)C` are located 15 cm apart. At what point on the line joining the two charges is the electric potential zero? Take the potential at infinity to be zero.
View SolutionAn electric dipole consists of two charges of equal magnitude and opposite sign separately by a distance 2a. The dipole is along the X-axis and is centred at the origin
(i) Calculate the electric potential at point P
(ii) Calculate V at a point far from the dipole.
View SolutionSketch the vairation of electric potential on the x-axis with respect to `x` for `x= - infty` to `x = + infty` in the following cases

View SolutionThe electric potential in a region is represented as
`V=2x+3y-z`
obtain expression for electric field strength.
View SolutionFind `V_(ab)` in an electric fiels `vec E = (2 hat I + 3 hat j + 4 hat k) NC^-1` where `vec r_a = (hat I - 2 hat j + hat k) m` and `vec r_b = (2 hat i + hat j - 2 hat k) m`.
View SolutionIn uniform electric field, `E = 10 NC^(-1)` as shown in figure, find
(i) `V_(A)-V_(B)`
(ii) `V_(B)-V_(C)`.
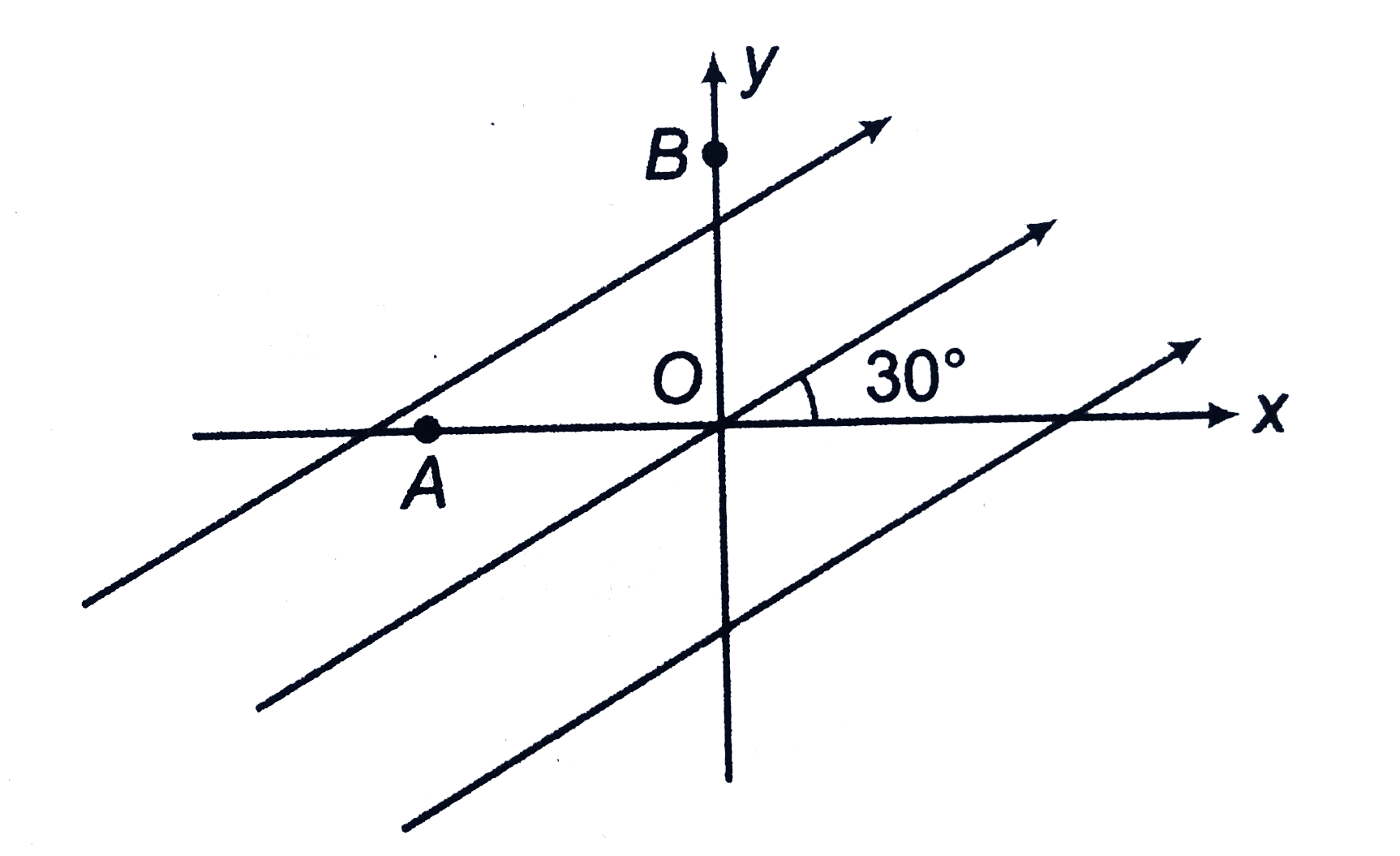
View SolutionA uniform electric field of `100 V//m` is directed at `30^@` with the positive x-axis as shown in figure. Find the potential difference `V_(BA)` if `OA=2`m and `OB=4 m.`
View SolutionTwo points A and B are 2 cm apart and a uniform electric field E acts along the straight line `AB` directed A to B with `E=200N//C`. A particle of charge `+10^-6C` is taken from A to B along AB, Calculate
a. the force on the charge
b. the potential difference `V_A -V_B` and
c.the work done on the charge by E
View SolutionIn the uniform electric field shown in figure, find

View SolutionAn electric field `E = (20hati + 30 hatj)` N/C exists in the space. If thepotential at the origin is taken be zero, find the potential at `(2 m, 2 m)`.
View SolutionEquipotential spheres are drawn round a point charge. As we move away from charge will the spacing between two spheres having a constant potential difference decrease, increase or remain constant.
View SolutionA charge `q=10muC` is distributed uniformly over the circumference of a ring of radius 3m placed on x-y placed with its centre art origin. Find the electric potential at a point `P(0,0,4m)`
View SolutionTwo identical thin ring, each of radius R meters, are coaxially placed a distance R metres apart. If `Q_1` coulomb, and `Q_2` coulomb, are repectively the charges uniformly spread on the two rings, the work done in moving a charge q from the centre of one ring to that of the other is
View SolutionA spherical drop of water carrying a charge of `3 xx 10^(-10) C` has potential of 500V at its surface. When two such drops having same charge and radius combine to from a single spherical drop, what is the potential at the surface of the new drop?
View SolutionA charge Q is distributed over two concentric hollow spheres of radii r and `R (gt r)` such that the surface charge densities are equal. Find the potential at the common centre.
View SolutionA bullet of mass `2 gm` is having a charge of `2 muc`. Through what potential difference must it be accelerated, starting from rest, to acquire a speed of `10 m//s`
View SolutionA ball of mass m having a charge q is released from rest in a region where a horizontal electric field E exists.
(i) Find the resultant force acting on the ball
(ii) find the trajectory followed by the ball.
View SolutionA block having mass `'m'` and charge `q` is resting on a frictionless plane at distance `L` from the wall as shown in fig.Discuss the motion of the block when a uniform electric field `E` is applied horizontally towards the wall assuming that collision of the block with the wall is perfectly elastic.
View SolutionTwo point charges each `50 mu C` are fixed on `y`-axis at `y = + 4 m` and `y = - 4m`. Another charged particle having charge `-50 mu C` and mass `20 g` is moving along the positive `x`-axis. When it is at `x = -3 m` , its speed is `20 m//sec`. Find the speed of charged particle when it reaches to origin. Also , find distance of charged particle from origin , when its kinetic energy becomes zero.
View SolutionA spherical oil drop, radius `10^(-4)` cm has on it at a certain a total charge of 40 electrons. Calculate the energy that would be required to place an additional electron on the drop.
View SolutionA uniform electric field `E_0` is directed along positive y-driection. Find the change in electric potential energy of a positive test charge `q_0` when it is displaced in this field from `y_i=a` to `y_f=2a` along the y-axis.
View SolutionFind the change in electric potential energy, `Delta U` as a charge of `2.20 xx 10^(-6)C`, mass from a point A to point B, given that the change in electric potential between these points is `DeltaV=V_(B)-V_(A)=24.0V`.
View SolutionA charge is moved in an electric field of a fixed charge distribution from point A to another point B slowly. The work done by external agent in doing so is `100 J`. What is the change in potential energy of the charge as it moves from A to B ? What is the work done by the electric field of the charge distribution as the charge moves from A to B ?
View SolutionThree charges ` 0.1` coulomb each are placed on the corners of an equilateral triangle of side `1 m`. If the energy is supplied to this system at the rate of ` 1 kW` how much time would be required to move one to the charges on to the midpoint of the line joining the two ?
View SolutionIn a hydrogen atom, the electron and proton are bound together at a distance of above 0.53 Å. Estimate the potential energy of the system in eV, assuming zero potential energy at infinite separation between the electron and the proton.
View SolutionA point charge `q_1 =-5.8muC` is held stationary at the origin. A second point charge `q_2=+4.3muC` moves from the pont `(0.26m 0,0)` to `(0.38m, 0, 0)`. How much work is done by the electric force on `q_2`?
View SolutionWhat minimum work must be done by an external force to bring a charge `q=3.00 muC` from a great distance away (take `t = infty`) to a point `0.500 m` from a charge ` = 20.0 muC` ?
View SolutionA point charge `q_1=9.1muC` is held fixe at origin. A second pont charge `q_2=-0.42muC` and `a` mass `3.2xx10^-4kg` is placed on the x-axis, `0.96m` from the origin. The second point charge is released at rest. What is its speed when it is `0.24m` from the origin?
View SolutionAn alpha particle with kinetic energy `10 Me V` is heading toward a stationary tin nucleus of atomic number 50. Calculate the distance of closest approach (Fig . 3.23).
 .
.
View SolutionA proton is fixed at origin. Another proton is released from rest, from a point at a distance `r` from origin. Taking charge of portion as e and mass as `m`, find the speed of the proton (i) at a distance `2r` from origin (ii) at large distance from origin.
View SolutionA uniformly charged thin ring has radius 10.0 cm and total charge `+12.0 nC`. An electron is placed on the ring's axis a distance `25.0 cm` from the centre of the ring and is constrained to stay on the axis of the ring. The electron is then released from rest, then
(i) describe the subsequent motion of the electron
(ii) find the speed of the electron when it reaches the centre of the ring.
View SolutionThree points charges of `1 C, 2C and 3C` are placed at the corners of an equilateral triangle of side 100 cm. Find the work done to move these charges to the corners of a similar equilateral triangle of side 50 cm.
View SolutionFind the electric potential energy of the system of charges
View SolutionFour charges `q_(1) = 1 muC,q_(2)=2 muC,q_(3)=-3 muC and q_(4)=4 muC` are kept on the vertices of a square of side `1 m`. Find the electric potential energy of this system of charges.
View SolutionTwo points charges are located on the x-axis, `q_1=-1muC` at `x=0 and q_2=+1muc` at `x=1m`.
a. Find the work that must be done by an external force to bring a third point charge `q_3=+1muC` from infinity to `x=2m`.
b. Find the total potential energy of the system of three charges.
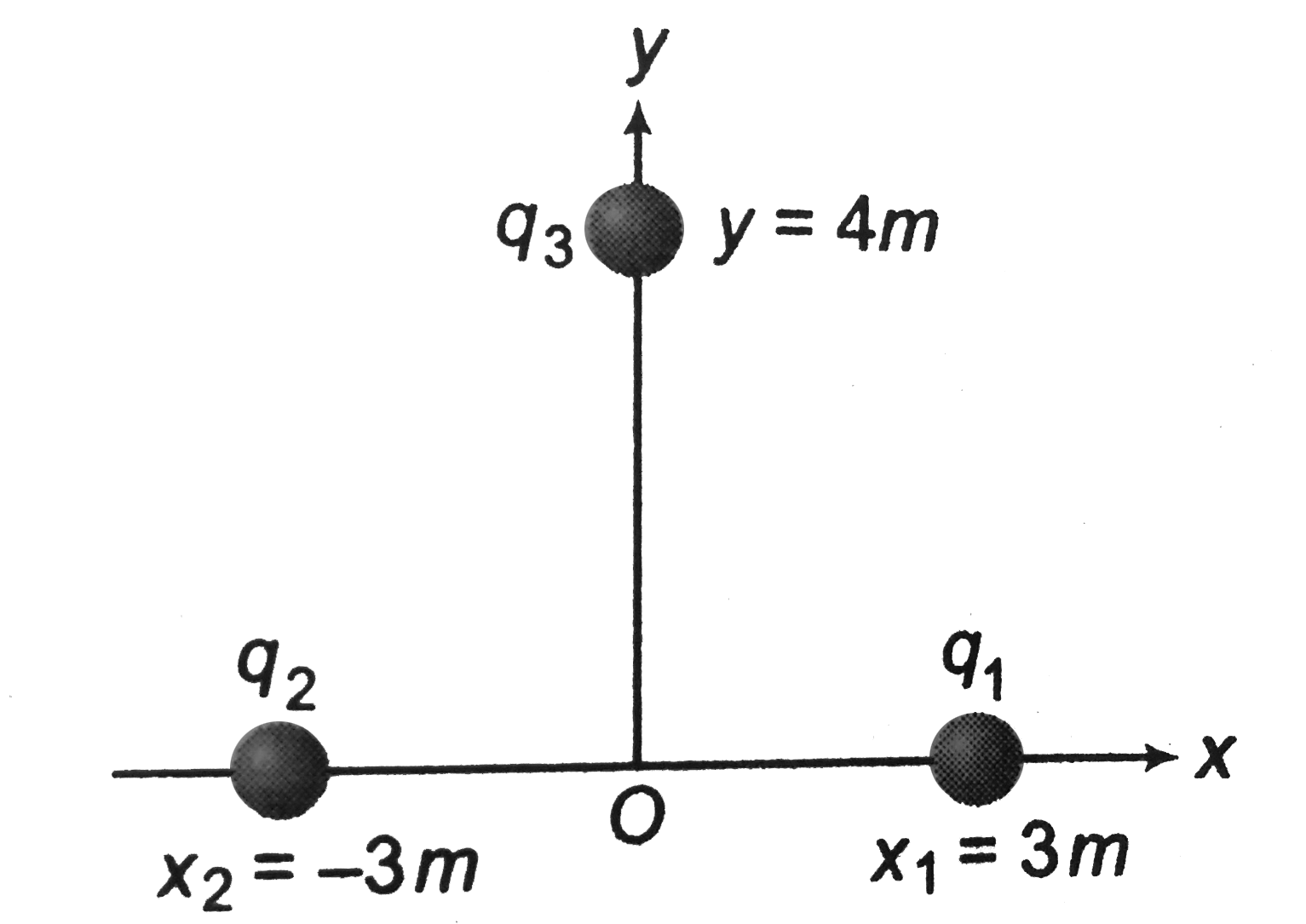
View SolutionTwo point charges `q_1=q_2=2muC` are fixed at `x_1=+3 m` and `x_2=-3m` as shown in figure. A third particle of mass 1 g and charge `q_3=-4muC` are released from rest at `y=4.0m`.Find the speed of the particle as it reaches the origin.
View SolutionWhen an electric dipole is placed in a uniform electric field making angle `theta` with electric field, it experiences a torque `tau`. Calculate the minimum work donein changing the orientation to `2 theta`.
View SolutionTwo point-charges `+2e and -2 e` are situated at a distance of `2.4 Å` from each other and constitude an electric dipole. This dipole is placed in a uniform electric field of `4.0 xx 10^(5) "Vm"^(-1)`. Calculate
(i) electric dipole moment,
(ii) potential energy of the dipole in equilibrium position,
(iii) work done in rotating the dipole through `180^(@)` from the equilibrium position.
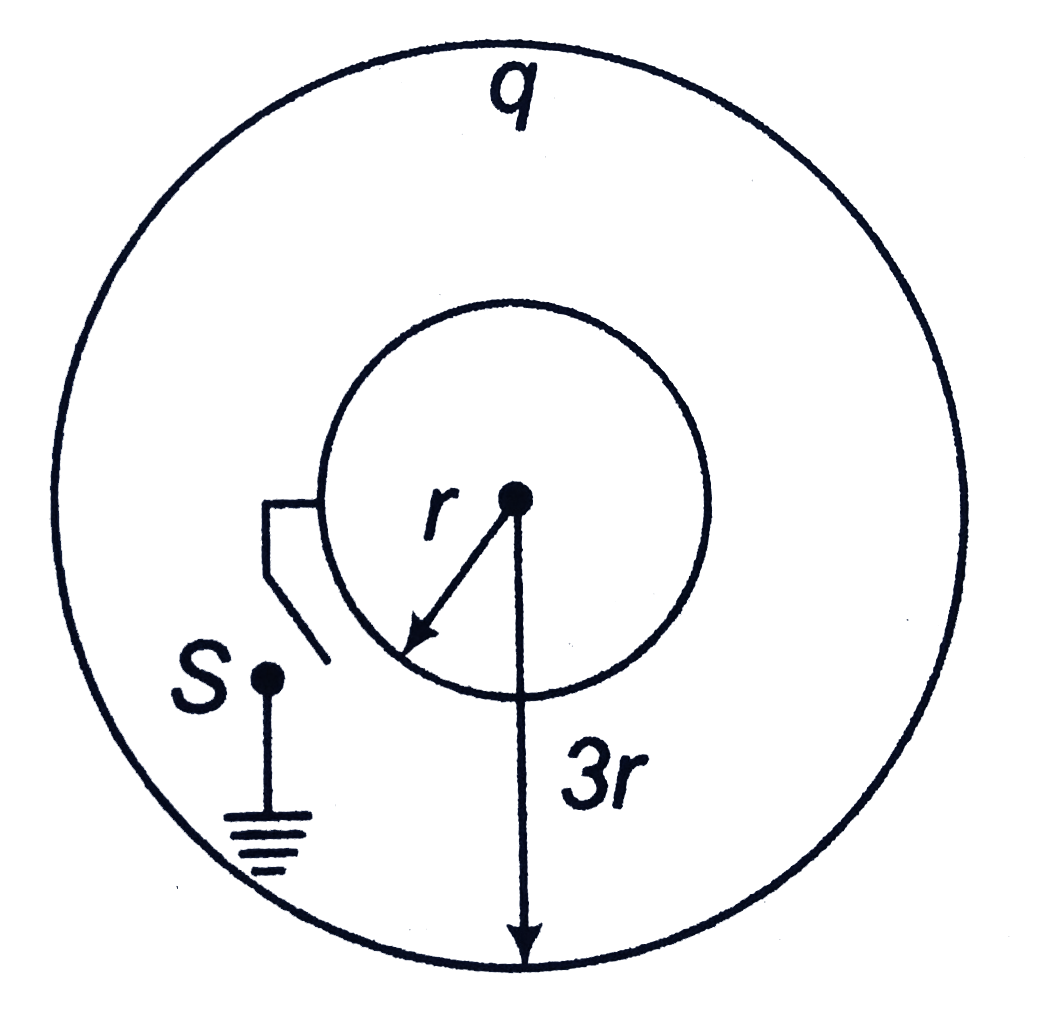
View SolutionFigure shows two conducting thin concentric shells of radi `r` and `3r`. The outer shell carries a charge q. Inner shell in neutral. Find the charge that wilL flow from inner shell to earth the switch S is closed.
View SolutionA sphere of `4 cm` radius is suspended within a hollow sphere of `6 cm` radius. The inner sphere is charged to potential `3` e.s.u. and the outer sphere is earthed. The charge on the inner sphere is
View SolutionA capacitor of `0.75 muF` is charged to a voltage of 16 V. What is the magnitude of the charge on each plate of the capacitor ?
View SolutionA sphere of radius `0.03m` is suspended within a hollow sphere of radius `0.05m`. If the inner sphere is charged to a potential of `1500` volt and outer sphere is earthed. Find the capacitance and the charge of the inner sphere.
View SolutionThe thickness of air layer between two coating of a spherical capacitor is 2cm. The capacitor has same capacitance as the sphere of 1.2m diameter. Find the radii of its surfaces.
View SolutionA parallel plate capacitor is constructed with plates of area `0.0280 m^(2)` and separation `0.550 mm`. Find the magnitude of the charge on each plate of this capacitor when the potential between the plates is `20.1 V`

View SolutionA dielectric slab of thickness 1.0 cm and dielectric constant 5 is placed between the plates of a parallel plate capacitor of plate area `0.01 m^(2)` and separation 2.0 cm. Calculate the change in capacity on introduction of dielectric. What would be on the change, if the dielectric slab were conducting?
View SolutionAn air-cored capacitor of plate area A and separation d has a capacity C. Two dielectric slabs are inserted between its plates in two different manners as shown. Calculate the capacitance in each case.

View SolutionA parallel plate capacitor has plate `A` and separation `d` between the plates. The capacitor is connected to a battery of emf `V`. (a) find the charge on the capacitor. (b) the plate separation is decreases to `d//2`. Find the extra charge given by the battery to the positive plate. (c) work done in reducing separation between plates.
View SolutionThe distance between the plates of a parallel plate capacitor is `0.04m` A field of `5000V//m` is established between the plates and an unchanged metal plate of thickness `0.01m` is inserted intothe condenser parallel to its plate. Find the `p.d`. between the plates (a) before the introducing of the metal plate and (b) after its introduction. what would be the `p.d.` if plate of dielectric constant `K=2` is introduced in place of metal plate?
View SolutionAn isolated `16 muF` parallel plate air capacitor has a potential difference of 100 V. dielectric slab having relative permittivity (i.e., dielectric constant) = 5 is introduced to fill the space between the two plates completely. Calculate.
(i) the new capacitance of the capacitor
(ii) the new potential differece between the two plates of capacitor.
View SolutionA capacitor `(C=50 muF)` is charged to a potential difference of `20V`. The charging battery is disconnected and the capacitor is connected to another cell of emf `10V` with the positive plate of capacitor joined with the positive terminal of cell.
(a) find charge flown through `10V` cell.
(b) is work done by the cell ot is it done on the cell? find its magnitude.
(c) find the heat developed in connecting wires.
View SolutionA parallel plate capacitor `(C=50 muF. d=4mm)` is charged to `200V` and then charging battery is removed. Now a dielectric slab `(K=4)` of thickness `2mm` is placed between the plates. Find new potential difference across capacitor.
View SolutionIn the circuit shown in figure find
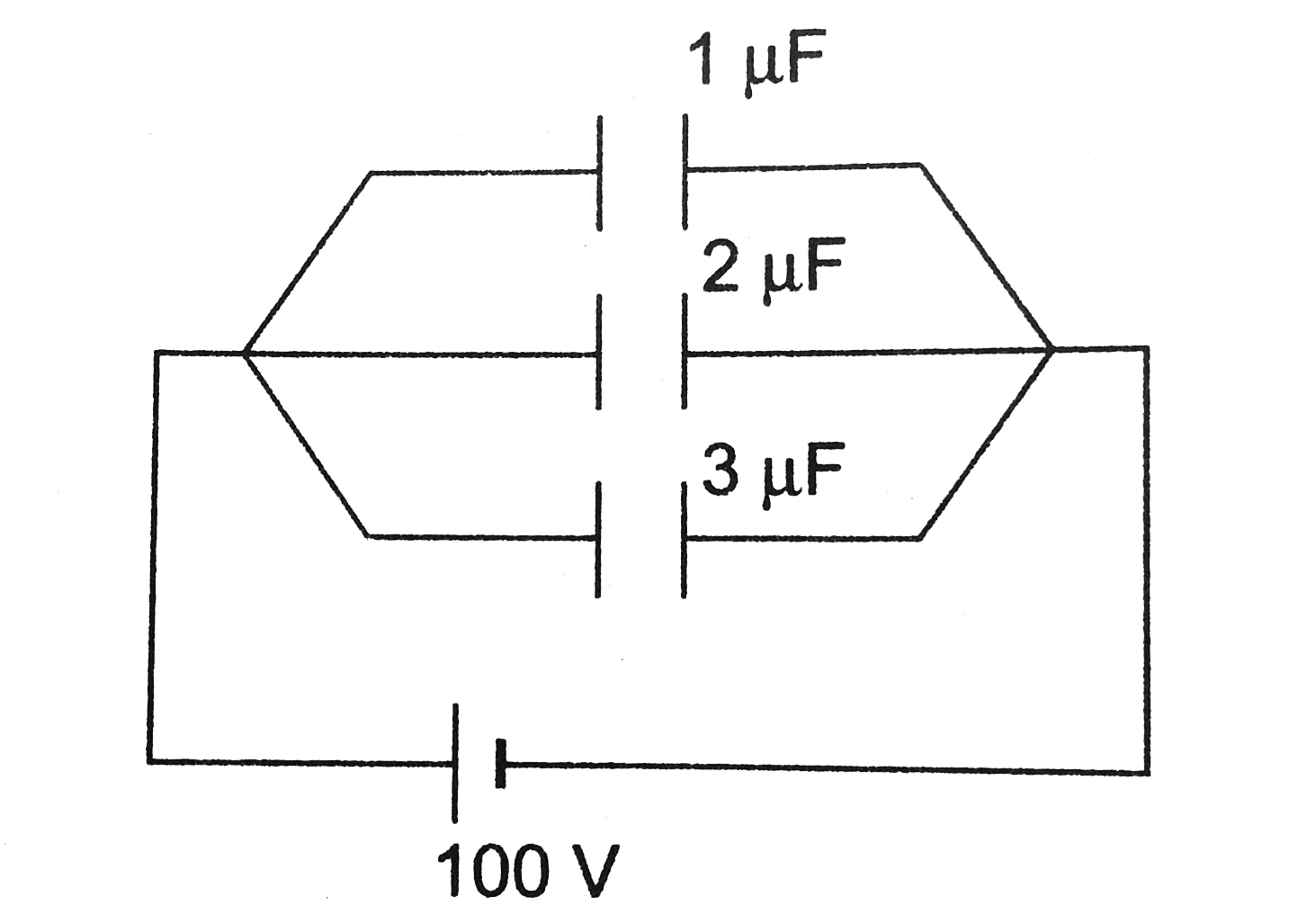
a. the equivalent capacitance and
b. the charge stored in each capacitor.
View SolutionFind the potential difference and charge on each capacitor.
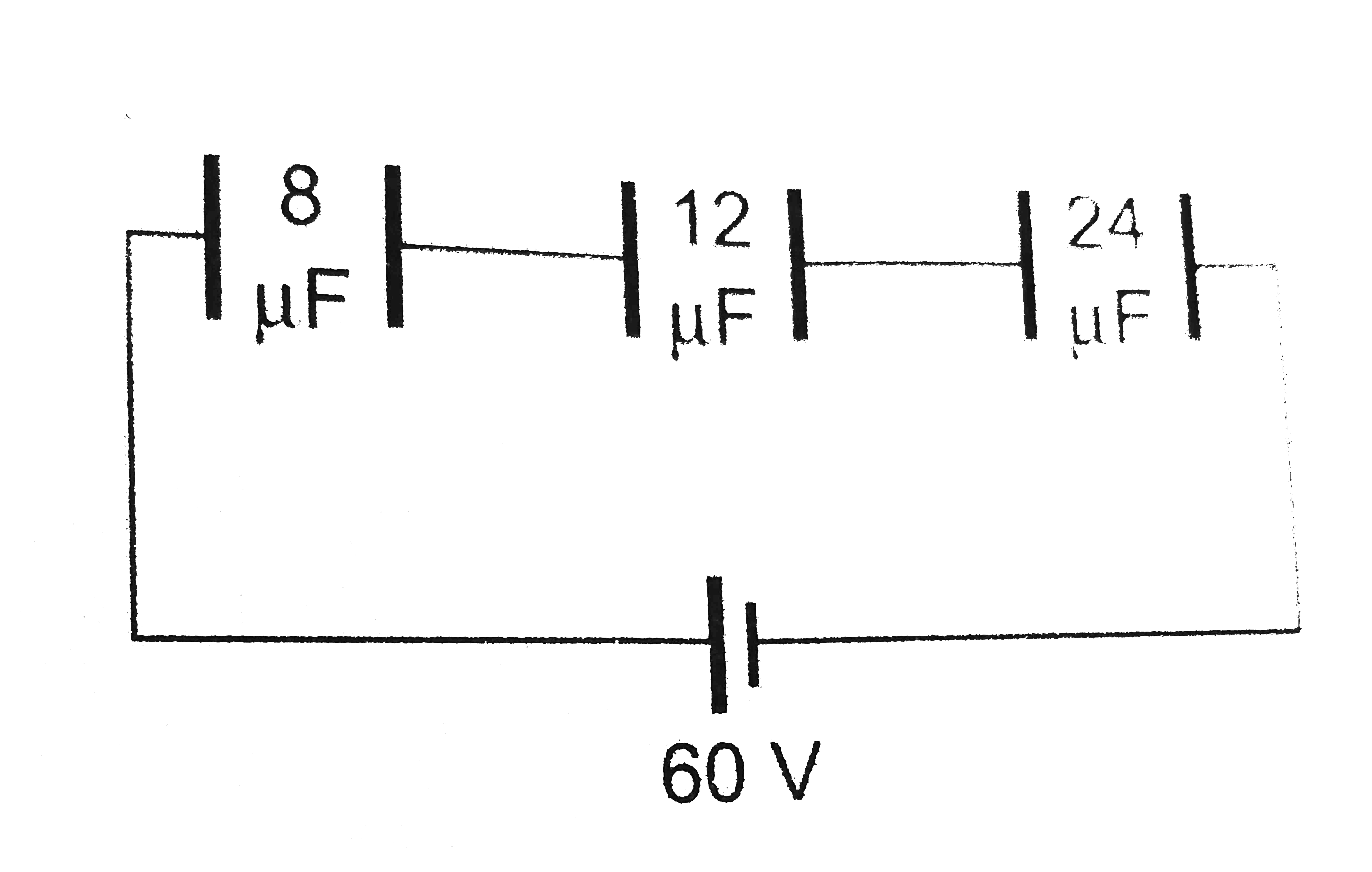
View SolutionFind the equivalent capacitance between A and B
(i)
Three conducting concentric shells of radii `r,2r,4r`
(ii)
An isolated ball-shaped conductor of radius `r` surrounded by an adjacent concentrc layer of dielectric `(K)` and outer radius 2r.
View SolutionIn the circuit shown in figure find

a. the equivalent capacitance and
b. the charge stored in each capacitor.
View Solution
(i) Find the equivalent capacitance of the combination shown in the figure (a), when `C_(1)=2.0 muF,C_(2)=4.0 muF and C_(3) = 3.0 muF`
(ii) The input terminals A and B in Fig. (a) are connected to a battery of 12 V. Find the potential and the charge of each capacitor.
View SolutionFor the following arrangement, find the equivalent capacitance between A and B
 .
.
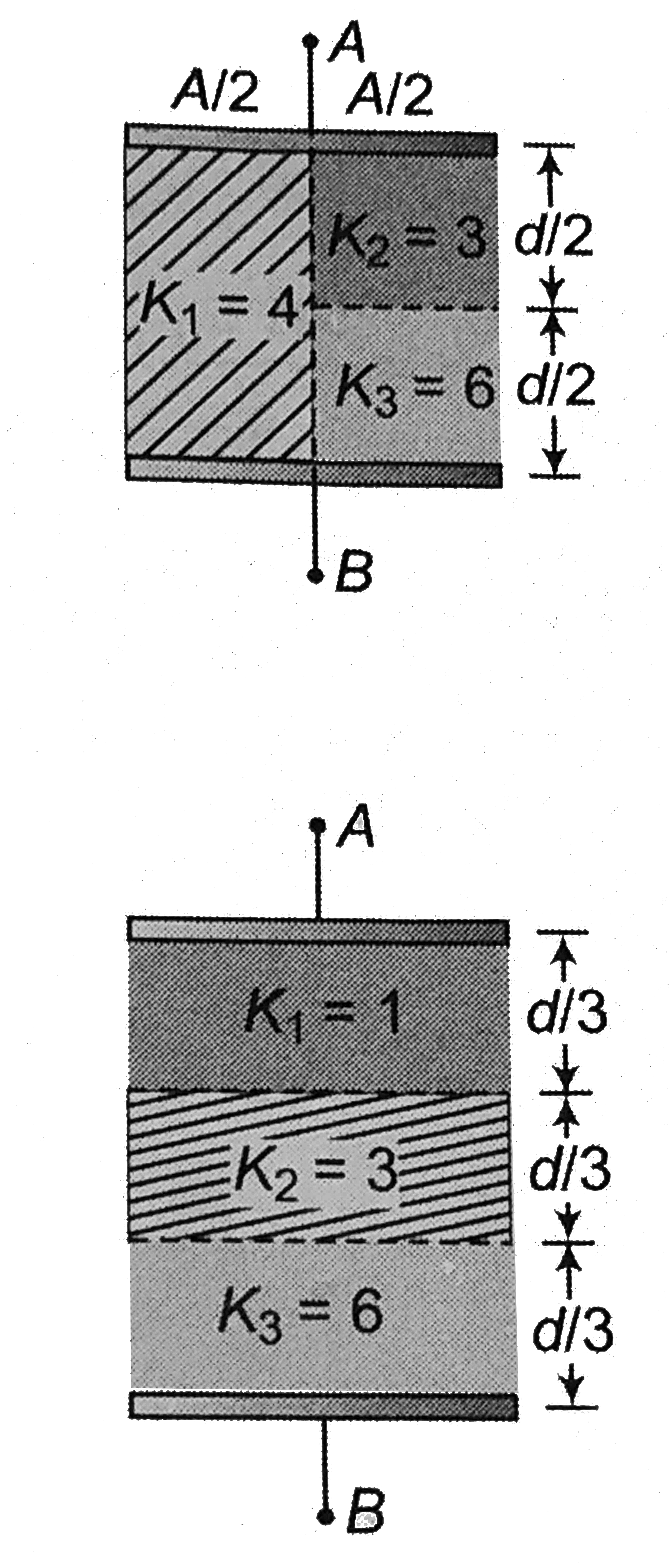
View SolutionFind the equivalent capacitance between `A` and `B`.`A`: area of each plate, `d`: separation between the plates.
View SolutionFind the potential difference and charge on each capacitance. All capacitances are in `muF`.
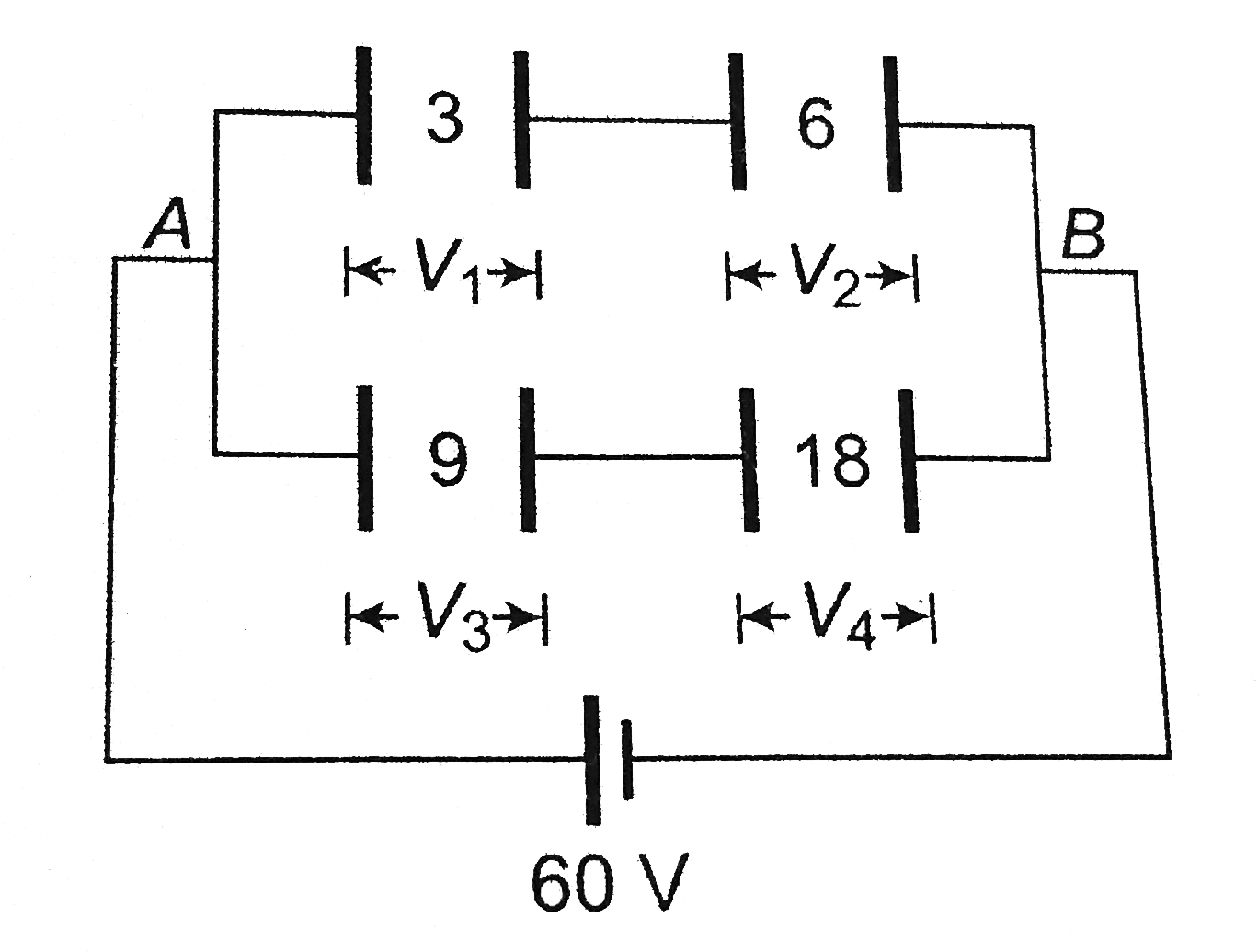
View SolutionIf charge on `5 muF` capacitor is `50 muC`, then find the potential difference on `4 muF` and emf of battery.

View SolutionIn the following figures, area of each plate is `A` and `d` is separation between adjacent plates. Find the capacitance of system between points `A` and `B`.

View SolutionFive identical capacitor plates each of area. A are arranged such that adjacent plates are at a distance d apart. The plates are connected to a square of emf V as shown. What is the magnitude and nature of charge on plates 1 and 3, respectively ?

View SolutionFind equivalent capacitance between points A and B shown in figure

View SolutionFind equivalent capacitance between points A and B

View SolutionFind the equivalent capacitance between A and B. All the capacitors have capacitance C.

View SolutionFigure shows a combination of twelve capacitors, each pf capacitance C, forming a cube. Find the equivalent capacitance of the combination (i) between the diagonally opposite corners A and B of the cube (ii) between the diagonally opposite corners A and D of a face of the cube.

View SolutionFind the equivalent capacitance between A and B. All the capacitors have capacitance C.

View SolutionFind the equivalent capacitance of the infinite ladder shown in the figure (a) between the points A and B

View SolutionIn the network of capacitors given ahead. Find the effective capacitance between point P and Q
If `C_(1)=C_(2)=C_(3)=C_(4)=4 muF `
Value is not given `C_(1) and C_(2)`

View SolutionFind the equivalent capacitance between `A` and `B`.
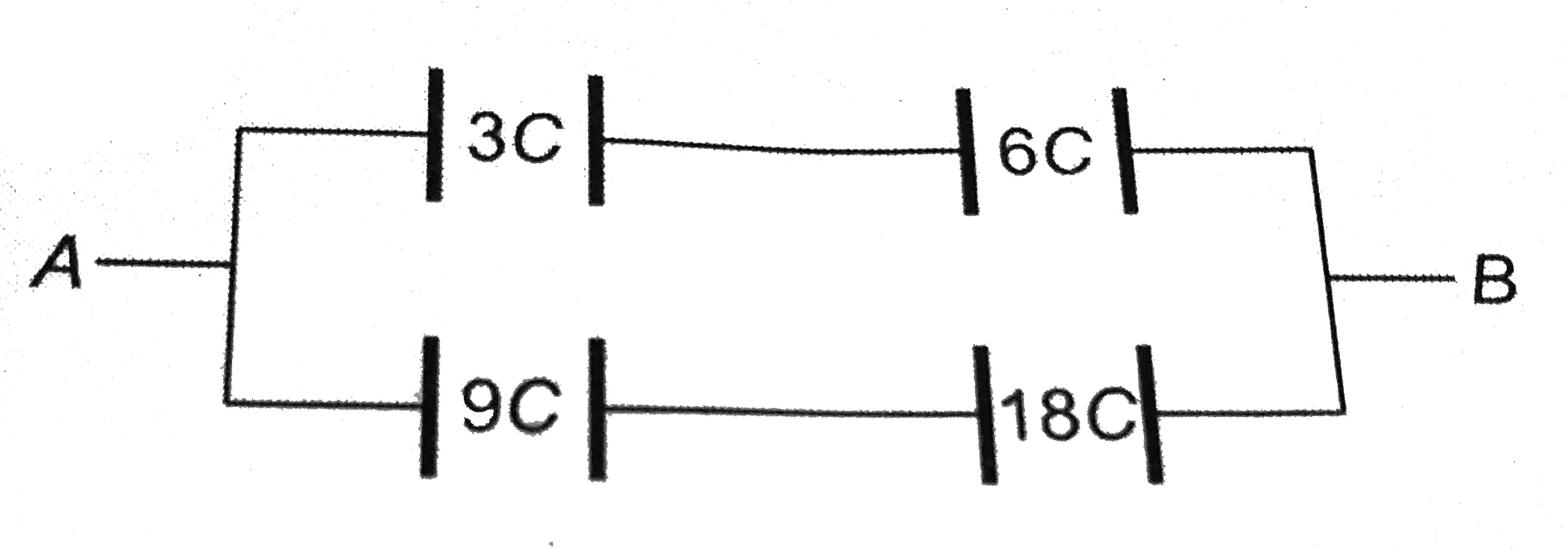
View SolutionFind the charges on the three capacitors shown in figure
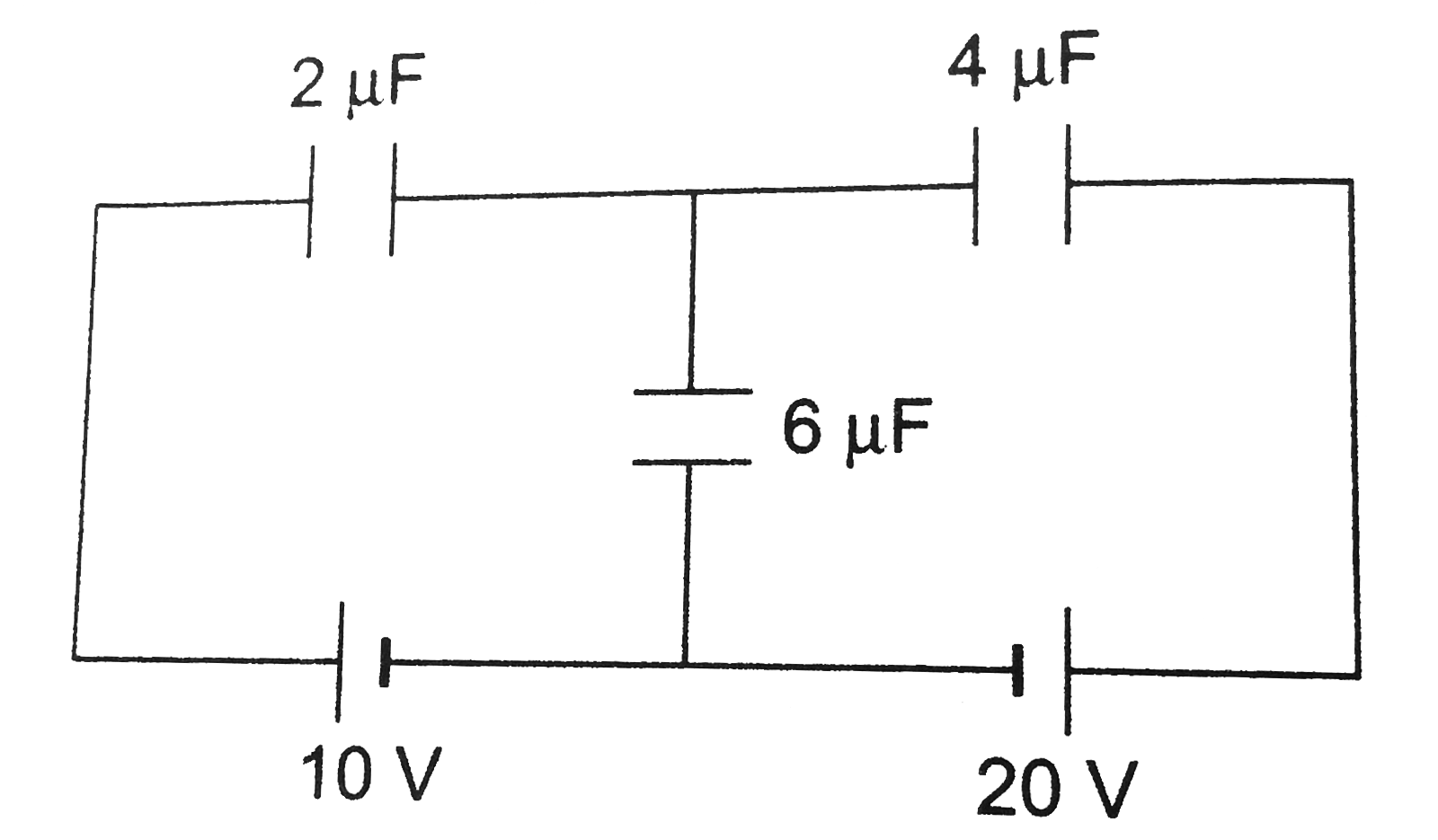
View SolutionCalculate energy stored in a capacitor of `5 mu F` when it is charged to a potential of 250 volt.
View SolutionThe plates of a parallel plate capacitor have on area of `90 cm^(2)` each and are separated by `2.5 mm`. The capacitor is charged to 400 V. How much electrostatic energy is stored in it ? How much when it is filled with a dielectric medium `K = 3` and then charged ? If it is first charged as an air capacitor and then filled with th dielectric, then ?
View SolutionTwo capacitors of capacitances `C_(1)=2muF` and `C_(2)=8muF` are connected in series and the resulting combination is connected across a `300V` battery. Calculate the charge, potential difference and energy stored in the capacitor separately.
View SolutionThe capacitance of a variable radio capacitor can be changed from `50pF` to `200pF` by turning the dial from `0^(@)` to `180^(@)`. With the dial set at `180^(@)`, the capacitor is connected to a `400V` battery. After charging, the capacitor is disconnected from the battery and dial is tuned at `0^(@)`?
(a) what is the `p.d.` across the capacitor when dial reads `0^(@)`?
(b) how much work is required to turn the dial, if friction is neglected?
View SolutionTwo isolated sphereical conductors have radii `5 cm` and `0 cm`, espctively They have charges of `12muC` and `-3muC`. Find the charges after they are connected by a conductng wire. Also find the common potential after resistribution.
View SolutionTwo parallel plates capacitors A and B having capacitance of `1 muF and 5 muF` are charged separately to the same potential of 100 V. Now, the positive plate of A is connected to the negative plate of B and the negative plate of A to the positive plate of B. Find the final charges on each capacitors.
View SolutionA capacitor of capacitance `5muF` is charged to potential `20V` and isolated. Now, an uncharged capacitor is connected in parallel to it. If the charge distributes equally on these capacitors, find total energy stored in capacitors.
View SolutionA capacitor `A` of capacitance `4muF` is charged to `30V` and another capacitor `B` of capacitance `2muF` is charged to `15V`. Now, the positive plate of `A` is connected to the negative plate of `B` and negative plate of `A` to the positive plate of `B`. find the final charge of each capacitor and loss of electrostatic energy in the process.
View Solution
Example questions and complete solutions for chapter ELECTROSTATIC POTENTIAL AND CAPACITORS of DC PANDEY ENGLISH of Class 12
