Level 2 from ARIHANT PHYSICS (HINGLISH) KINEMATICS for Class 11
| Class 11 | PHYSICS |
| Chapter | KINEMATICS |
| ARIHANT PHYSICS (HINGLISH) | Level 2 |
| Medium | English |
| Academic Year | 2023-2024 |
Question 1
A particle is travelling on a curved path. In an interval Δt its speed changed from v to 2v. However, the change in magnitude of its velocity was found to be ∣∣∣−−→ΔV∣∣∣=√5v. What can you say about the direction of velocity at the beginning and at the end of the interval (Δt)?View SolutionQuestion 2
Two tourist A and B who are at a distance of 40 km from their camp must reach it together in the shortest possible time. They have one bicycle and they decide to use it in turn. ‘A’ started walking at a speed of 5kmhr1 and B moved on the bicycle at a speed of 15kmhr1. After moving certain distance B left the bicycle and walked the remaining distance. A, on reaching near the bicycle, picks it up and covers the remaining distance riding it. Both reached the camp together. (a) Find the average speed of each tourist. (b) How long was the bicycle left unused?View SolutionQuestion 3
The position time graph for a particle travelling along x axis has been shown in the figure. State whether following statements are true of false.
(a) Particle starts from rest at t=0.
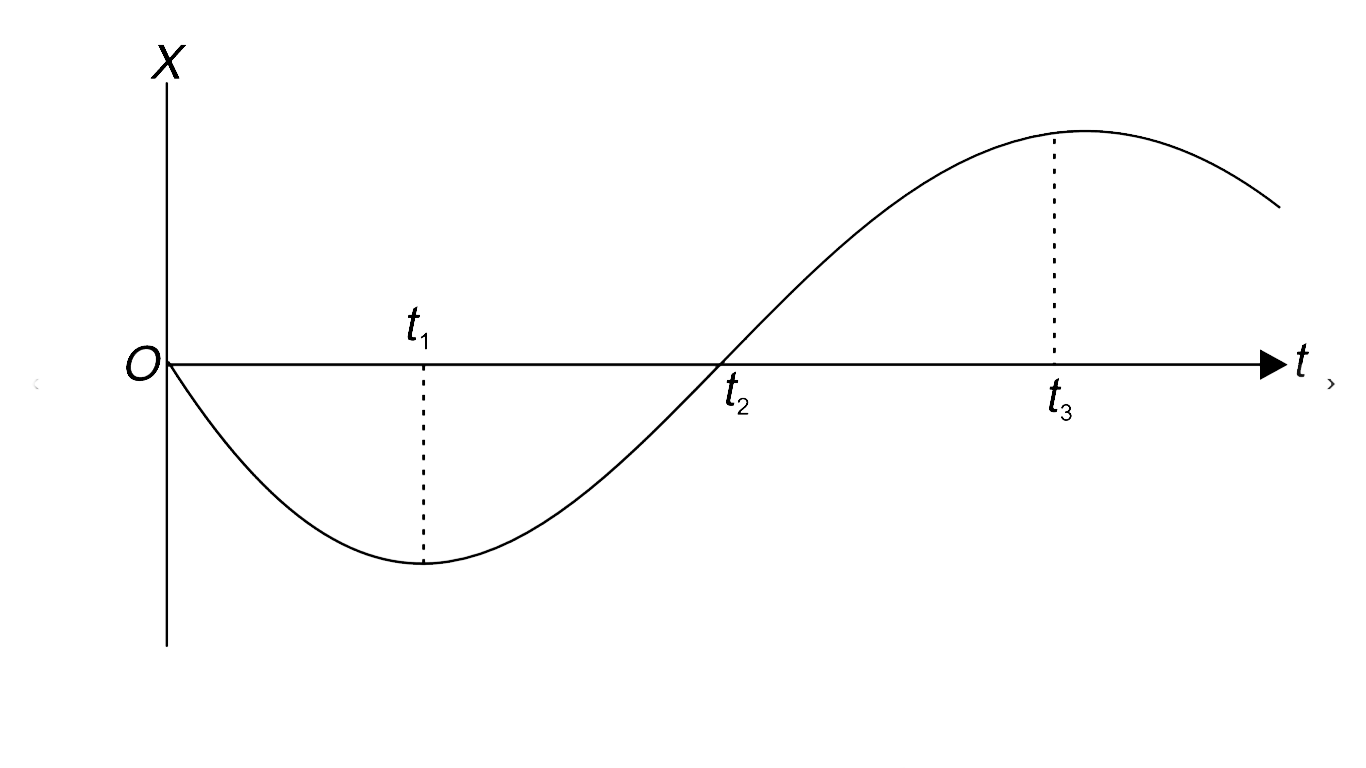
(b) Particle is retarding in the interval 0 to t1 and accelerating in the interval t1 to t2.
(c) The direction of acceleration has changed once during the interval 0 to t3View SolutionQuestion 4
The position time graph for a particle moving along X axis has been shown in the fig. At which of the indicated points the particle has
(i) negative velocity but acceleration in positive X direction.
(ii) positive velocity but acceleration in negative X direction.
(iii) received a sharp blow (a large force for negligible interval of time)?
 View Solution
View SolutionQuestion 5
A particle is moving along positive X direction and is retarding uniformly. The particle crosses the origin at time t=0 and crosses the point x=4.0m at t=2s.
(a) Find the maximum speed that the particle can possess at x=0.
(b) Find the maximum value of retardation that the particle can have.View SolutionQuestion 6
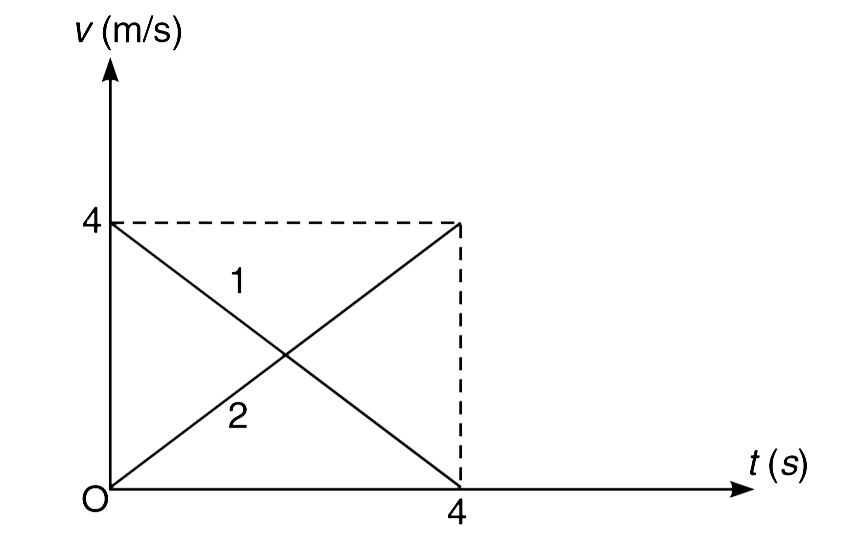
The velocity time graph for two particles (1 and 2) moving along X axis is shown in fig. At time t=0, both were at origin.
(a) During first 4 second of motion what is maximum separation between the particles? At what time the separation is maximum?
(b) Draw position (x) vs time (t) graph for the particles for the given interval.
 View Solution
View SolutionQuestion 7
A ball travelling in positive X direction with speed V0 hits a wall perpendicularly and rebounds with speed V0. During the short interaction time (Δt) the force applied by the wall on the ball varies as shown in figure.
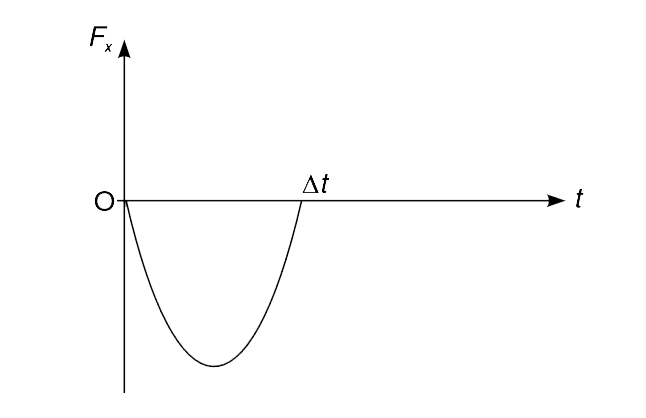
Draw the velocity-time graph for the ball during the interval 0 to ΔtView SolutionQuestion 8
For a particle moving along a straight line consider following graphs A, B, C and D. Here x, v and t are position, velocity and time respectively.
(i) In which of the graphs the magnitude of acceleration is decreasing with time?
(ii) In which of the graphs the magnitude of acceleration is increasing with time?
(iii) If the body is definitely going away from the starting point with time, which of the given graphs represent this condition.View SolutionQuestion 12
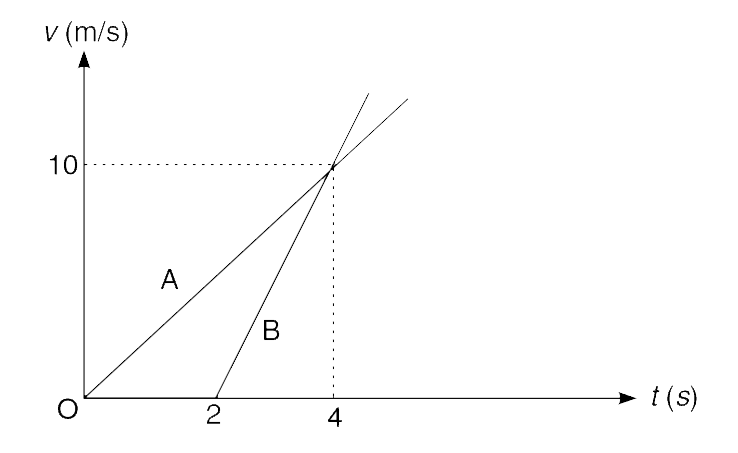
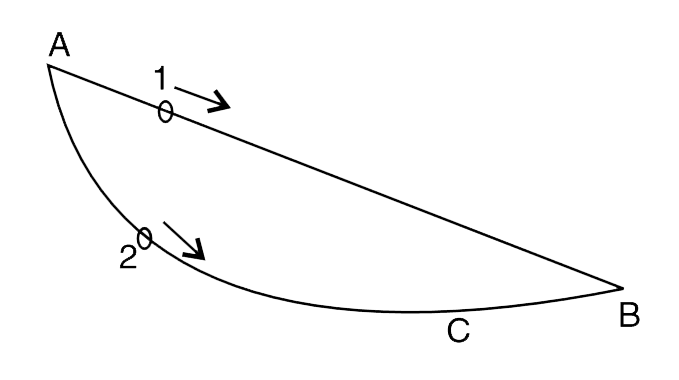
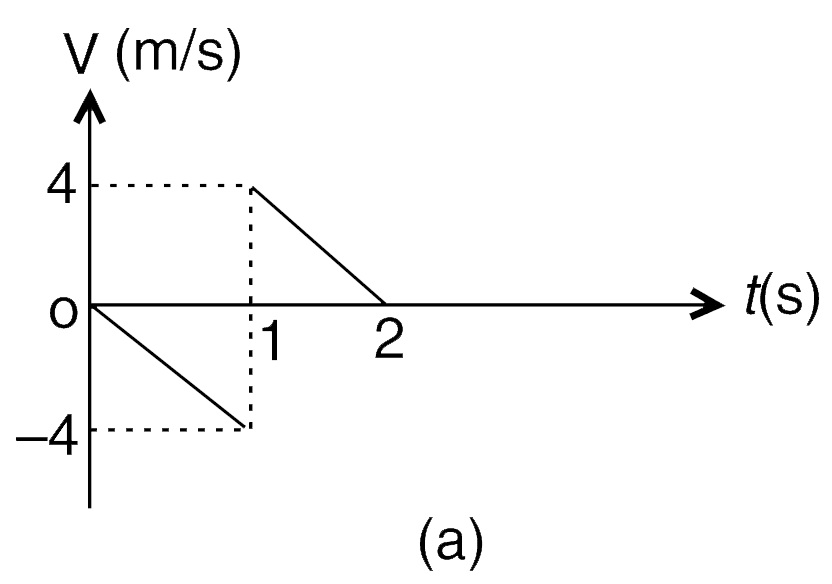
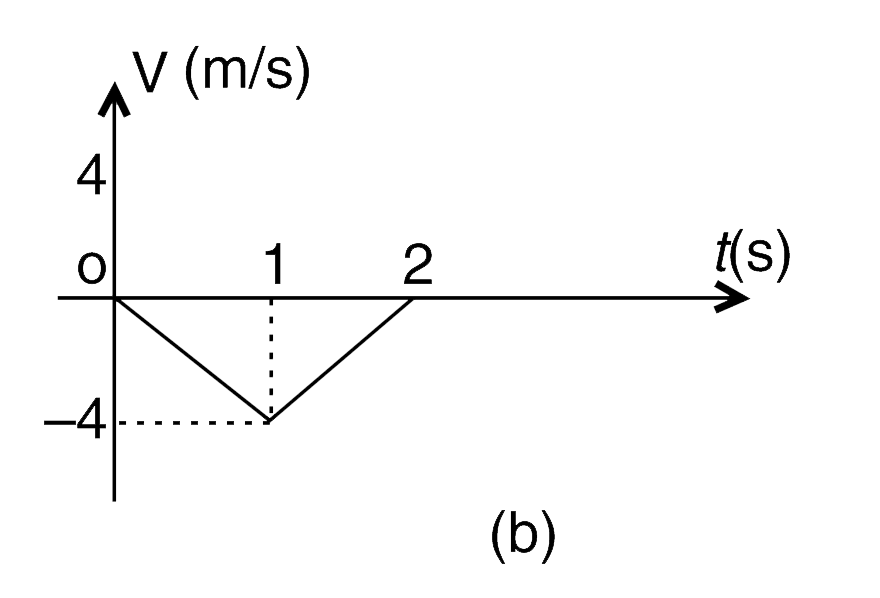
The velocity (V) – time (t) graphs for two particles A and B moving rectilinearly have been shown in the figure for an interval of 2 second.
(a) At t=1s, which of the two particles (A or B) has received a severe blow?
(b) Draw displacement (X) – time (t) graph for both of them.
 View Solution
View SolutionQuestion 13
A particle starts moving rectilinearly at time t=0 such that its velocity(v) changes with time (t) as per equation –
v=(t2–2t)m/s for 0<t<2s
=(–t2+6t–8)m/s for 2≤te4s
(a) Find the interval of time between t=0 and t=4s when particle is retarding.
(b) Find the maximum speed of the particle in the interval 0≤t≤4s. View SolutionQuestion 14
Our universe is always expanding. The rate at which galaxies are receding from each other is given by Hubble’s law (discovered in 1929 by E. Hubble). The law states that the rate of separation of two galaxies is directly proportional to their separation. It means relative speed of separation of two galaxies, presently at separation r is given by v = Hr
H is a constant known as Hubble’s parameter. Currently accepted value of H is 2.32×x10–18s–1
(a) Express the value of H in unit of
Km.s−1Mega light year
(b) Find time required for separation between two galaxies to change from r to 2r. View SolutionQuestion 16
A ball is dropped from a height H above the ground. It hits the ground and bounces up vertically to a height H2 where it is caught. Taking origin at the point from where the ball was dropped, plot the variation of its displacement vs velocity. Take vertically downward direction as positive.View SolutionQuestion 19
Two nearly identical balls are released simultaneously from the top of a tower. One of the balls fall with a constant acceleration of g1=9.80ms–2 while the other falls with a constant acceleration that is 0.1% greater than g1. [This difference may be attributed to variety of reasons. You may point out few of them]. What is the displacement of the first ball by the time the second one has fallen 1.0 mm farther than the first ball? View SolutionQuestion 21
(a) A particle starts moving at t=0 in x−y plane such that its coordinates (in cm) with time (in sec) change as x=3t and y=4sin(3t). Draw the path of the particle.
(b) If position vector of a particle is given by →r=(4t2−16t)ˆi+(3t2−12t)ˆj, then find distance travelled in first 4 sec. View SolutionQuestion 23
A ball is projected from the floor of a long hall having a roof height of H=10m. The ball is projected with a velocity of u=25ms–1 making an angle of θ=37∘ to the horizontal. On hitting the roof the ball loses its entire vertical component of velocity but there is no change in the horizontal component of its velocity. The ball was projected from point A and its hits the floor at B. Find distance AB.
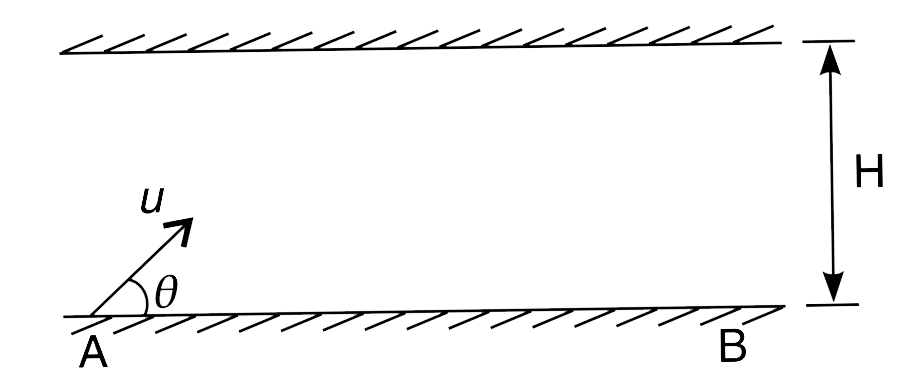 View Solution
View SolutionQuestion 24
In a tennis match Maria Sharapova returns an incoming ball at an angle that is 4° below the horizontal at a speed of 15m/s. The ball was hit at a height of 1.6 m above the ground. The opponent, Sania Mirza, reacts 0.2 s after the ball is hit and runs to the ball and manages to return it just before it hits the ground. Sania runs at a speed of 7.5m/s and she had to reach 0.8 m forward, from where she stands, to hit the ball.
(a) At what distance Sania was standing from Maria at the time the ball was returned by Maria? Assume that Maria returned the ball directly towards Sania.
(b) With what speed did the ball hit the racket of Sania?
[g=9.8m/s2]View SolutionQuestion 25
A player initially at rest throws a ball with an initial speed u=19.5m/s at an angle θ=sin−1(1213) to the horizontal. Immediately after throwing the ball he starts running to catch it. He runs with constant acceleration (a) for first 2 s and thereafter runs with constant velocity. He just manages to catch the ball at exactly the same height at which he threw the ball. Find ‘a’. Take g=10m/s2. Do you think anybody can run at a speed at which the player ran? View SolutionQuestion 26
In a cricket match, a batsman hits the ball in air. A fielder, originally standing at a distance of 12 m due east of the batsman, starts running 0.6 s after the ball is hits. He runs towards north at a constant speed of 5m/s and just manages to catch the ball 2.4 s after he starts running. Assume that the ball was hit and caught at the same height and take g=10m/s2g=10m/s2 Find the speed at which the ball left the bat and the angle that its velocity made with the vertical.View SolutionQuestion 28
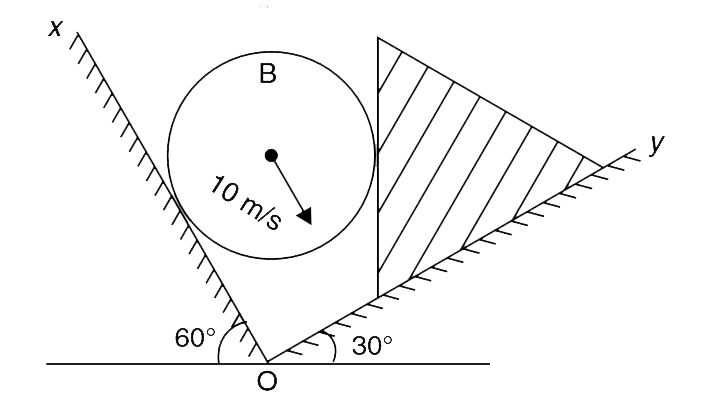
A boy ‘A’ is running on a circular track of radius R. His friend, standing at a point O on the circumference of the track is throwing balls at speed u=√gR. Balls are being thrown randomly in all possible directions. Find the length of the circumference of the circle on which the boy is completely safe from being hit by a ball.
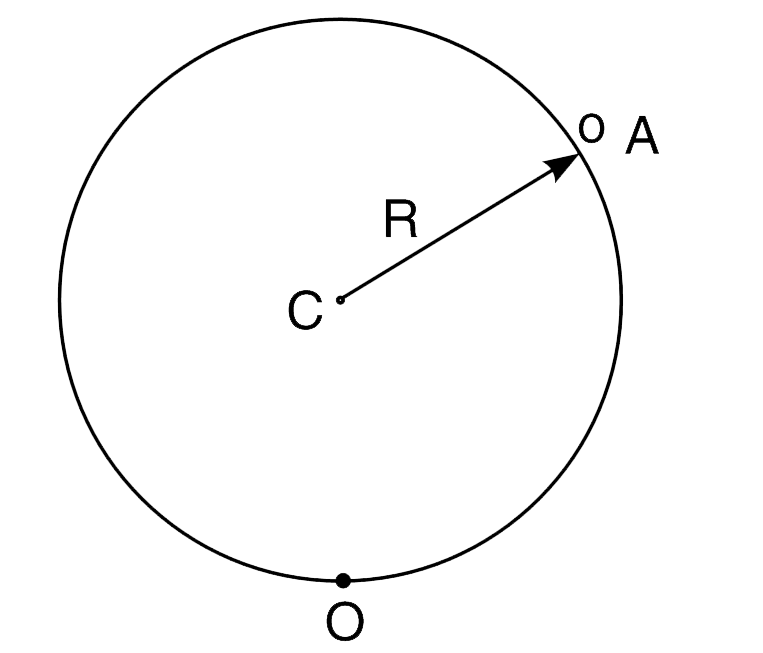 View Solution
View SolutionQuestion 29
A rectangular cardboard ABCD has dimensions of 40cm×x30cm. It is moving in a direction perpendicular to its shorter side at a constant speed of 2cm/s. A small insect starts at corner A and moves to diagonally opposite corner C. On reaching C it immediately turns back and moves to A. Throughout the motion the insect maintains a constant speed relative to the board. It takes 10 s for the insect to reach C starting from A. Find displacement and distance travelled by the insect in reference frame attached to the ground in the interval the insect starts from A and comes back to A.
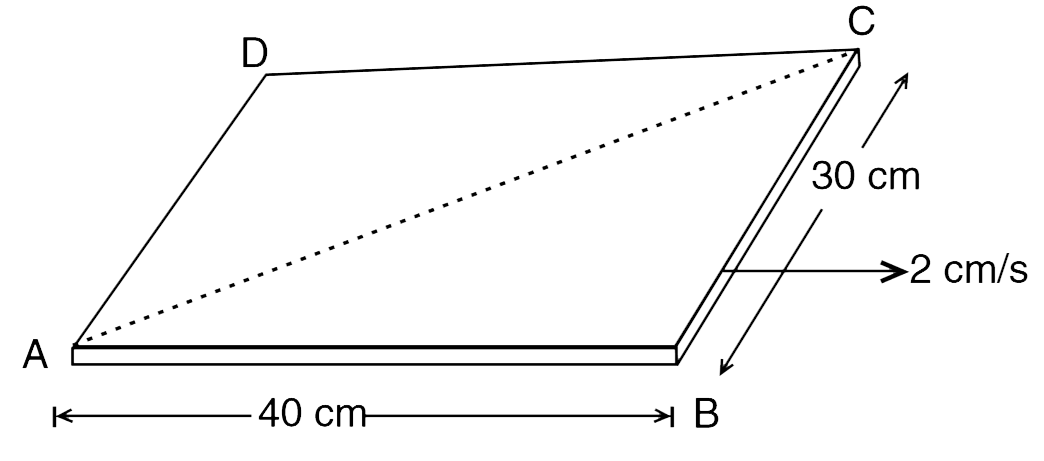 View Solution
View SolutionQuestion 30
Two particles A and B separated by 10 m at time t=0 are moving uniformly. A is moving along line AB at a constant velocity of 4m/s and B is moving perpendicular to the velocity of A at a constant velocity of 5m/s. After what time the two particles will be nearest to each other?
 View Solution
View SolutionQuestion 31
Four cars are moving along a straight road in the same direction. Velocity of car 1 is 10m/s. It was found that distance between car 1 and 2 is decreasing at a rate of 2m/s, whereas driver in car 4 observed that he was nearing car 2 at a speed of 8m/s. The gap between car 2 and 3 is decreasing at a rate of 3m/s.
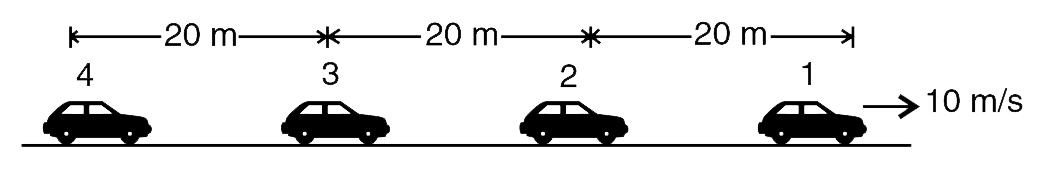
(a) t=0, after how much time t0 will the driver of car 2 see for the first time, that another car overtakes him?
(b) Which car will be first to overtake car 1? View SolutionQuestion 33
A physics professor was driving a Maruti car which has its rear wind screen inclined at θ=37∘ to the horizontal. Suddenly it started raining with rain drops falling vertically. After some time the rain stopped and the professor found that the rear wind shield was absolutely dry. He knew that, during the period it was raining, his car was moving at a constant speed of Vc=20km/hr.[tan37∘=0.75]
(a) The professor calculated the maximum speed of vertically falling raindrops as Vmax. What is value of Vmax that he obtained.
(b) Plot the minimum driving speed of the car vs. angle of rear wind screen with horizontal (θ) so as to keep rain off the rear glass. Assume that rain drops fall at constant speed VrView SolutionQuestion 34
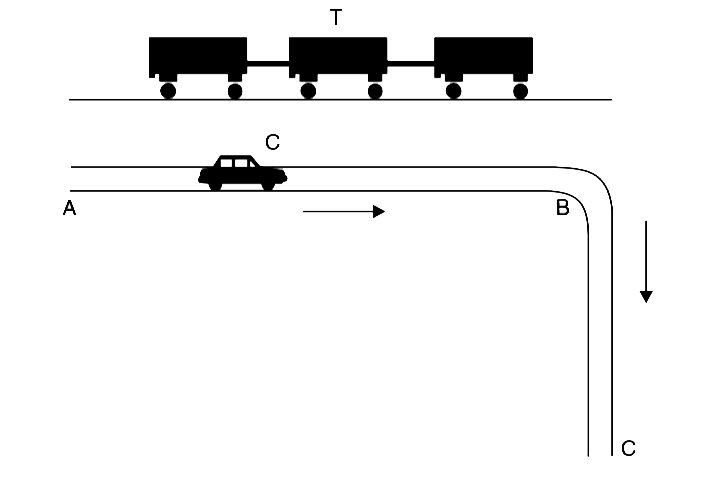 A train(T) is running uniformly on a straight track. A car is travelling with constant speed along section AB of the road which is parallel to the rails. The driver of the car notices that the train is having a speed of 7m/s with respect to him. The car maintains the speed but takes a right turn at B and travels along BC. Now the driver of the car finds that the speed of train relative of him is 13m/s. Find the possible speeds of the car.View Solution
A train(T) is running uniformly on a straight track. A car is travelling with constant speed along section AB of the road which is parallel to the rails. The driver of the car notices that the train is having a speed of 7m/s with respect to him. The car maintains the speed but takes a right turn at B and travels along BC. Now the driver of the car finds that the speed of train relative of him is 13m/s. Find the possible speeds of the car.View SolutionQuestion 35
 A police car B is chasing a culprit’s car A. Car A and B are moving at constant speed V1=108km/hr and V2=90km/hr respectively along a straight line. The police decides to open fire and a policeman starts firing with his machine gun directly aiming at car A. The bullets have a velocity u=305m/s relative to the gun. The policeman keeps firing for an interval of T0=20s. The Culprit experiences that the time gap between the first and the last bullet hitting his car is Δt. Find Δt. View Solution
A police car B is chasing a culprit’s car A. Car A and B are moving at constant speed V1=108km/hr and V2=90km/hr respectively along a straight line. The police decides to open fire and a policeman starts firing with his machine gun directly aiming at car A. The bullets have a velocity u=305m/s relative to the gun. The policeman keeps firing for an interval of T0=20s. The Culprit experiences that the time gap between the first and the last bullet hitting his car is Δt. Find Δt. View SolutionQuestion 36
A chain of length L is supported at one end and is hanging vertically when it is released. All of the chain falls freely with acceleration g. The moment, the chain is released a ball is projected up with speed u from a point 2 L below the point of support. Find the interval of time in which the ball will cross through the entire chain.View SolutionQuestion 38
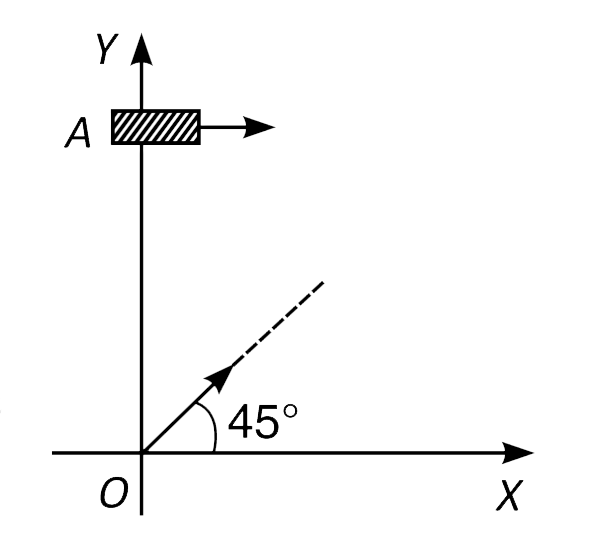
A small cart A starts moving on a horizontal surface, assumed to be x-y plane along a straight line parallel to x-axis (see figure) with a constant acceleration of 4m/s2. Initially it is located on the positive y-axis at a distance 9 m from origin. At the instant the cart starts moving, a ball is rolled along the surface from the origin in a direction making an angle 45° with the x-axis. The ball moves without friction at a constant velocity and hits the cart.
(a) Describe the path of the ball in a reference frame attached to the cart.
(b) Find the speed of the ball.
 View Solution
View SolutionQuestion 39
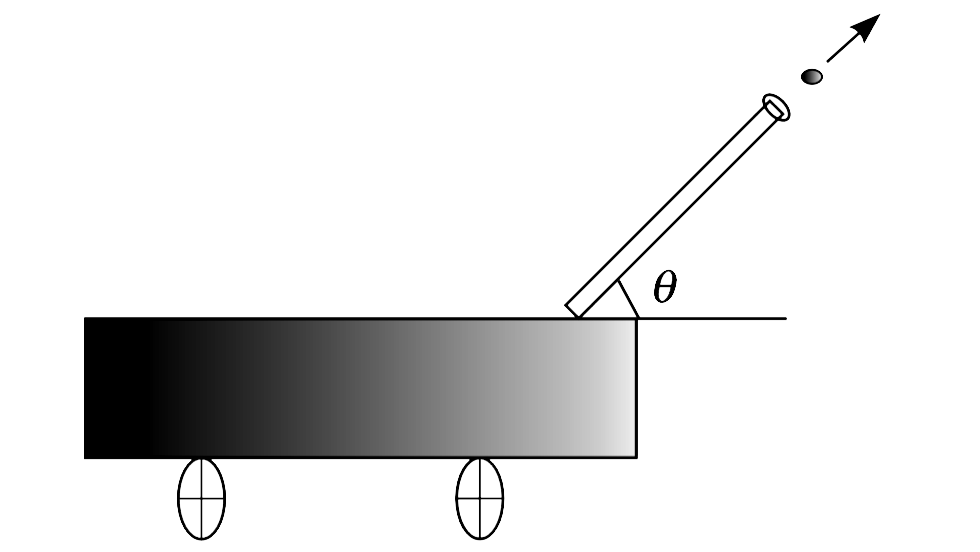
(a) A boy on a skateboard is sliding down on a smooth incline having inclination angle q. He throws a ball such that he catches it back after time T. With what velocity was the ball thrown by the boy relative to himself ?

(b) Barrel of an anti aircraft gun is rotating in vertical plane (it is rotating up from the horizontal position towards vertical orientation in the plane of the fig). The length of the barrel is L=√2m and barrel is rotating with angular velocity ω=2rad/s. At the instant angle θ is 45° a shell is fired with a velocity 2√2m/s with respect to the exit point of the barrel. The tank recoils with speed 4m/s. What is the launch speed of the shell as seen from the ground?
 View Solution
View SolutionQuestion 40
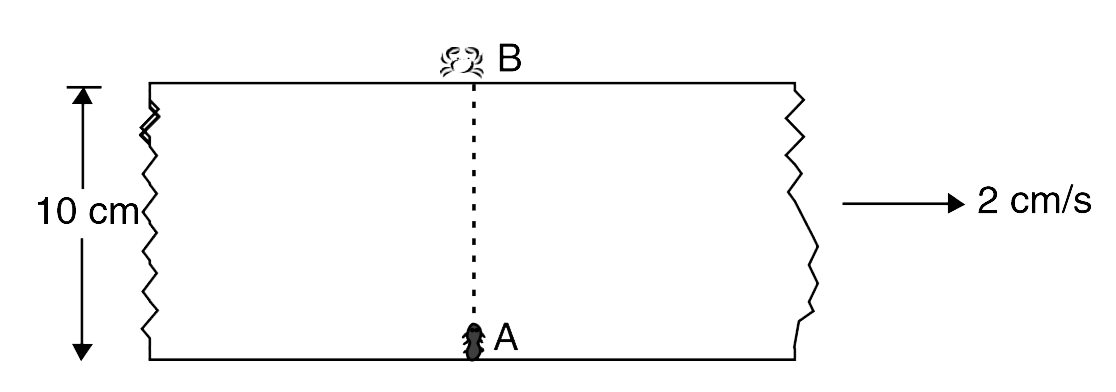
long piece of paper is10 cm wide and is moving uniformly along its length with a velocity of 2cm/s. An ant starts moving on the paper from point A and moves uniformly with respect to the paper. A spider was located exactly opposite to the ant just outside the paper at point B at the instant the ant started to move on the paper. The spider, without moving itself, was able to grab the ant 5 second after it (the ant) started to move. Find the speed of ant relative to the paper.
 View Solution
View SolutionQuestion 41
Two particles A and B are moving uniformly in a plane in two concentric circles. The time period of rotation is TA=8 minute and TB=11 minute respectively for the two particles. At timet=0, the two particles are on a straight line passing through the centre of the circles. The particles are rotating in same sense. Find the minimum time when the two particles will again fall on a straight line passing through the centre. View SolutionQuestion 43
Two paper discs are mounted on a rotating vertical shaft. The shaft rotates with a constant angular speed ω and the separation between the discs is H. A bullet is fired vertically up so that it pierces through the two discs. It creates holes H1 and H2 in the lower and the upper discs. The angular separation between the two holes (measured with respect to the shaft axis) is θ. Find the speed (v) of the bullet. Assume that the speed of the bullet does not change while travelling through distance H and that the discs do not complete even one revolution in the interval the bullet pierces through them.
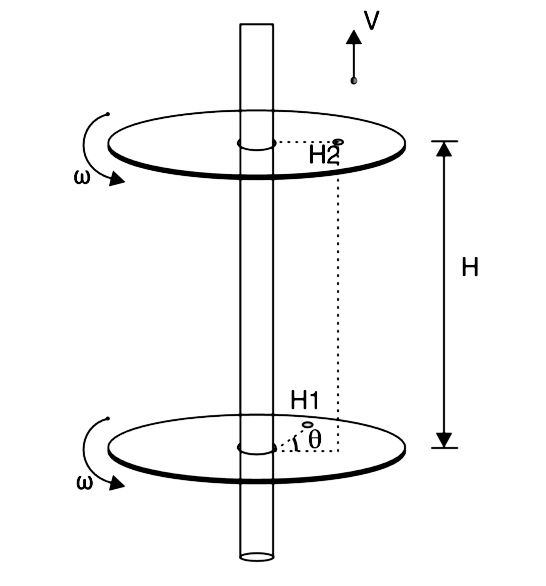 View Solution
View SolutionQuestion 44
(a) A car moves around a circular arc subtending an angle of 60° at the centre. The car moves at a constant speed u0 and magnitude of its instantaneous acceleration is a0. Find the average acceleration of the car over the 60° arc.
(b) The speed of an object undergoing uniform circular motion is 4m/s. The magnitude of the change in the velocity during 0.5 sec is also 4m/s. Find the minimum possible centripetal acceleration (in m/s2) of the object.View SolutionQuestion 45
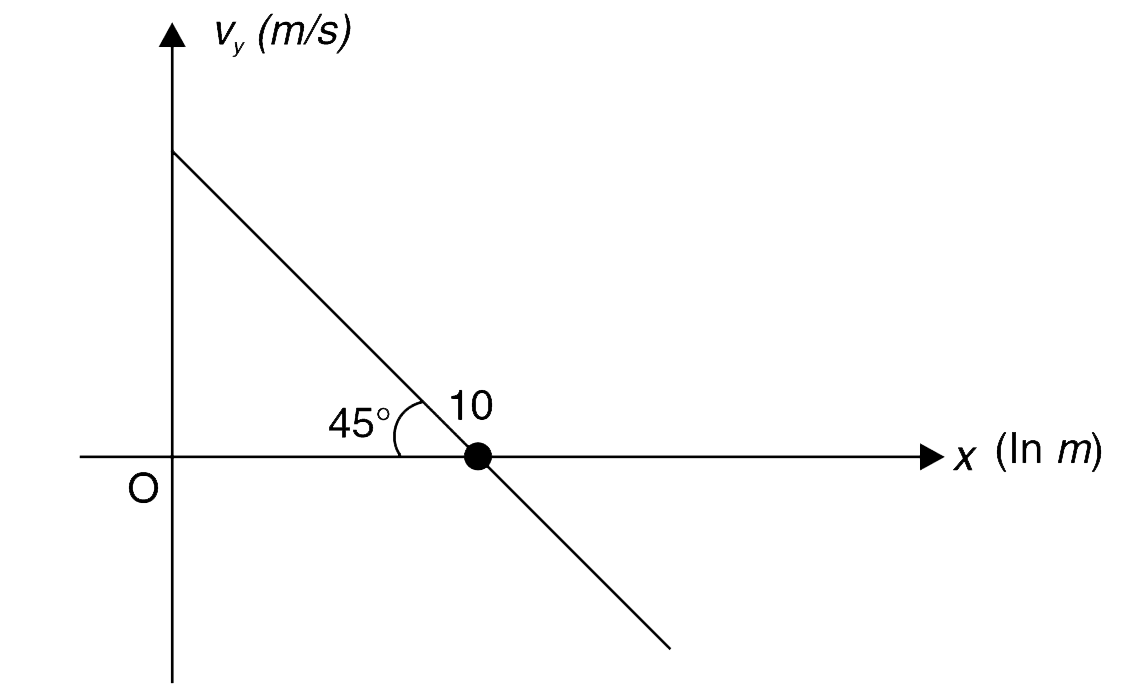
A particle is fixed to the edge of a disk that is rotating uniformly in anticlockwise direction about its central axis. At time t=0 the particle is on the X axis at the position shown in figure and it has velocity v
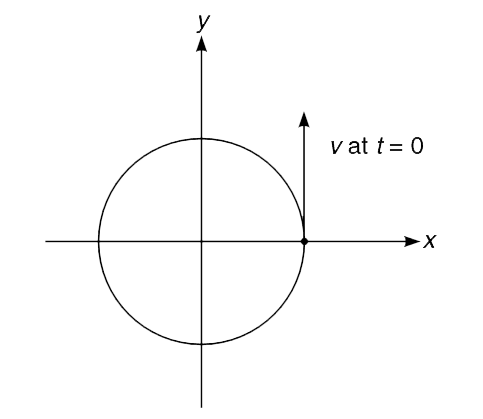
(a) Draw a graph representing the variation of the x component of the velocity of the particle as a function of time.
(b) Draw the y-component of the acceleration of the particle as a function of time. View SolutionQuestion 46
A disc is rotating with constant angular velocity ω in anticlockwise direction. An insect sitting at the centre (which is origin of our co-ordinate system) begins to crawl along a radius at time t=0 with a constant speed V relative to the disc. At time t=0 the velocity of the insect is along the X direction.
(a) Write the position vector (→r) of the insect at time ‘t’.
(b) Write the velocity vector (→v) of the insect at time ‘t’.
(c) Show that the X component of the velocity of the insect become zero when the disc has rotated through an angle θ given by tanθ=1θ.
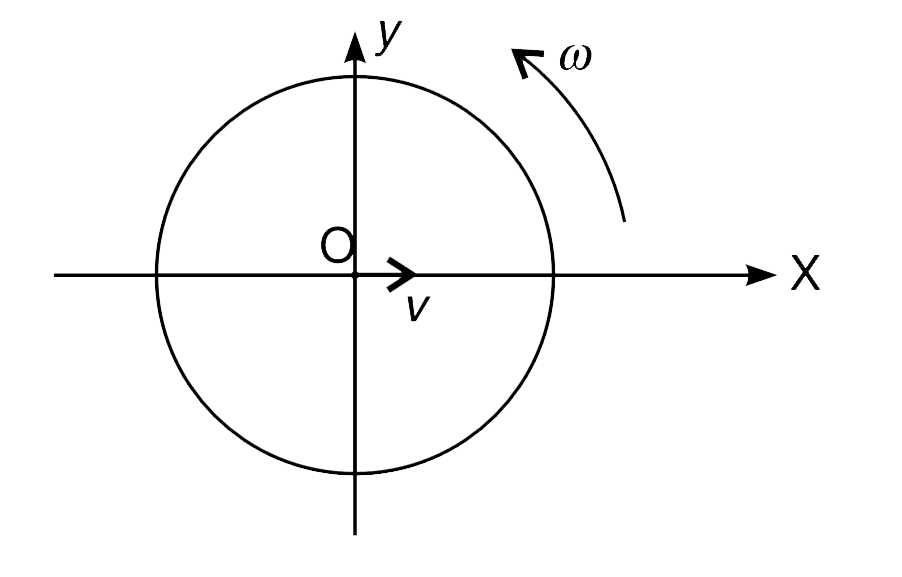 View Solution
View SolutionQuestion 47
(a) A point moving in a circle of radius R has a tangential component of acceleration that is always n times the normal component of acceleration (radial acceleration). At a certain instant speed of particle is v0. What is its speed after completing one revolution?
(b) The tangential acceleration of a particle moving in xy plane is given by at=a0cosθ. Where a0 is a positive constant and q is the angle that the velocity vector makes with the positive direction of X axis. Assuming the speed of the particle to be zero at x=0, find the dependence of its speed on its x co-ordinate. View SolutionQuestion 48
A particle is rotating in a circle. When it is at point A its speed is V. The speed increases to 2 V by the time the particle moves to B. Find the magnitude of change in velocity of the particle as it travels from A to B. Also, find −→VA−−→ΔV, where −→VA is its velocity at point A and −−→ΔV is change in velocity as it moves from A to B. View SolutionQuestion 52
Two friends A and B are running on a circular track of perimeter equal to 40 m. At time t=0 they are at same location running in the same direction. A is running slowly at a uniform speed of 4.5km/hr whereas B is running swiftly at a speed of 18km/hr.
(a) At what time t0 the two friends will meet again?
(b) What is average velocity of A and B for the interval t=0 to t=t0? View SolutionQuestion 53
A particle is moving along x axis. Its position as a function of time is given by x=x(t). Say whether following statements are true or false.
(a) The particle is definitely slowing down if d2xdt2>0 and dxdt<0
(b) The particle is definitely moving towards the origin if d(x2)dt<0View SolutionQuestion 55
Harshit and Akanksha both can run at speed v and walk at speed u(u<v). They together start on a journey to a place that is at a distance equal to L. Akanksha walks half of the distance and runs the second half. Harshit walks for half of his travel time and runs in the other half. View SolutionQuestion 56
There are two cars on a straight road, marked as x axis. Car A is travelling at a constant speed of VA=9m/s. Let the position of the Car A, at time t=0, be the origin. Another car B is L=40m ahead of car A at t=0 and starts moving at a constant acceleration of a=1m/s2 (at t = 0). Consider the length of the two cars to be negligible and treat them as point objects.
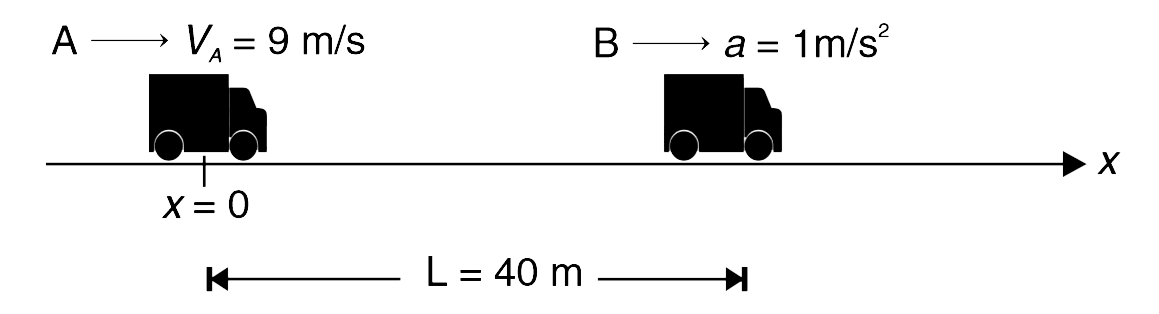
(a) Plot the position–time (x–t) graph for the two cars on the same graph. The two graphs intersect at two points. Draw conclusion from this.
(b) Determine the maximum lead that car A can have. View SolutionQuestion 57
Particle A is moving with a constant velocity of VA=50ms–1 in positive x direction. It crossed the origin at time t=10s. Another particle B started at t=0 from the origin and moved with a uniform acceleration of aB=2ms–2 in positive x direction.
(a) For how long was A ahead of B during the subsequent journey?
(b) Draw the position (x) time (t) graph for the two particles and mark the interval for which A was ahead of B. View SolutionQuestion 58
(a) A particle is moving along the x axis and its velocity vs position graph is as shown. Is the acceleration of the particle increasing, decreasing or remains constant?
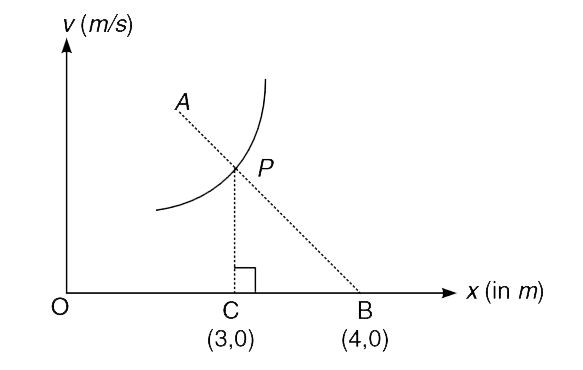
(b) A particle is moving along x axis and its velocity (v) vs position (x) graph is a curve as shown in the figure. Line APB is normal to the curve at point P. Find the instantaneous acceleration of the particle at x=3.0m.
 View Solution
View SolutionQuestion 59
A particle has co-ordinates (x, y). Its position vector makes on angle θ with positive x direction. In an infinitesimally small interval of time the particle moves such that length of its position vector does not change but angle θ increases by dθ. Express the change in position vector of the particle in terms of x, y, dθ and unit vectors ˆi and ˆj.
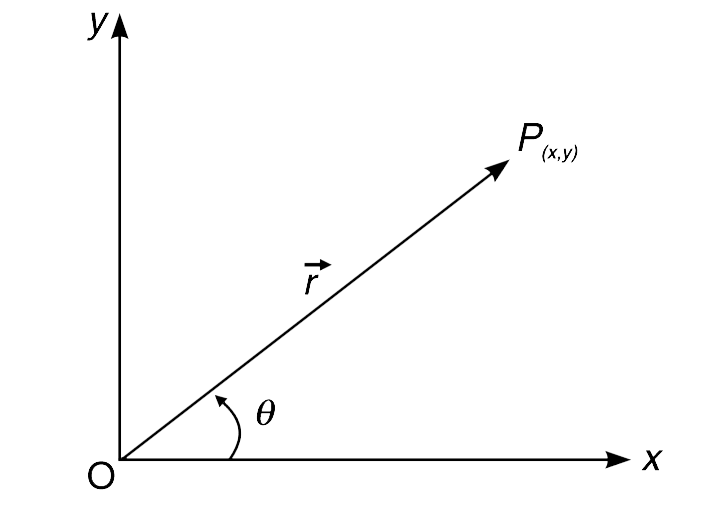 View Solution
View SolutionQuestion 60
A rope is lying on a table with one of its end at point O on the table. This end of the rope is pulled to the right with a constant acceleration starting from rest. It was observed that last 2 m length of the rope took 5 s in crossing the point O and the last 1m took 2 s in crossing the point O.
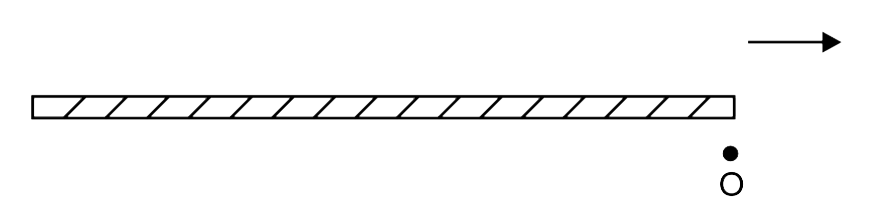
(a) Find the time required by the complete rope to travel past point O.
(b) Find length of the rope. View SolutionQuestion 61
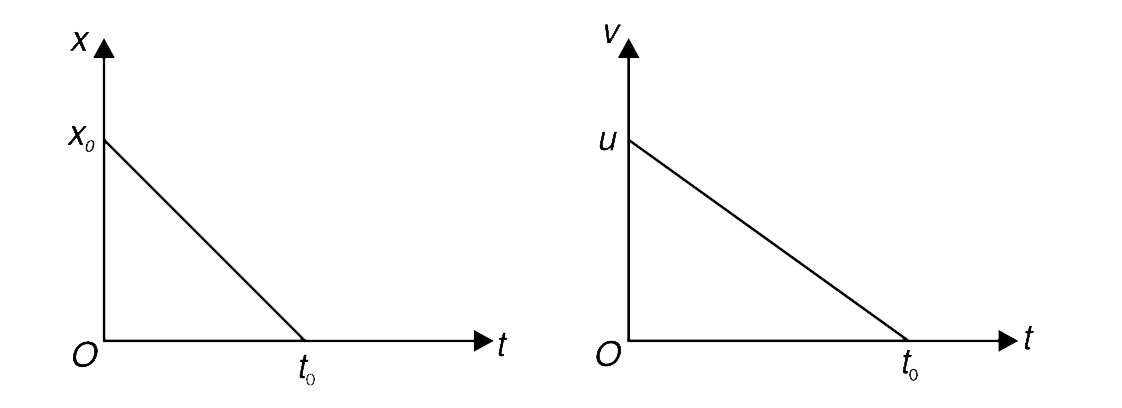
Two particles 1 and 2 move along the x axis. The position (x) - time (t) graph for particle 1 and velocity (v) - time (t) graph for particle 2 has been shown in the figure. Find the time when the two particles collide. Also find the position (x) where they collide. It is given that x0=ut0, and that the particle 2 was at origin at t=0. View SolutionQuestion 62
Two stations A and B are 100km apart. A passenger train crosses station A travelling at a speed of 50km/hr. The train maintains constant speed for 1 hour 48 minute and then the driven applies brakes to stop the train at station B in next 6 minute. Another express train starts from station B at the time the passenger train was crossing station A. The driver of the express train runs the train with uniform acceleration to attain a peak speed v0. Immediately after the train attains the peak speed v0, he applies breaks which cause the train to stop at station A at the same time the passenger train stops at B. Brakes in both the trains cause uniform retardation of same magnitude. Find the travel time of two trains and v0. View SolutionQuestion 63
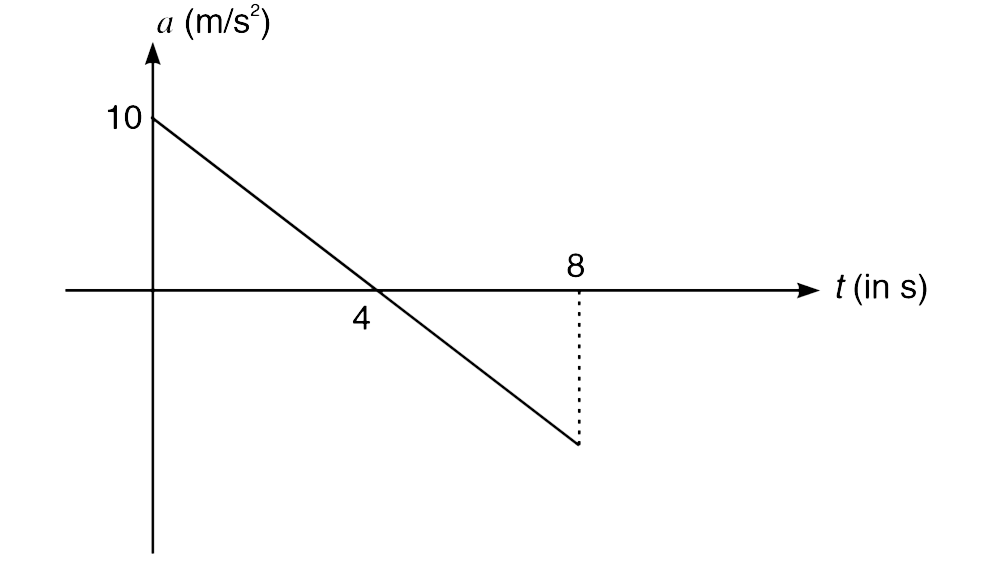
Particle A starts from rest and moves along a straight line. Acceleration of the particle varies with time as shown in the graph. In 10 s the velocity of the particle becomes 60m/s and the acceleration drops to zero. Another particle B starts from the same location at time t=1.1s and has acceleration – time relationship identical to A with a delay of 1.1s. Find distance between the particles at time t=15s.
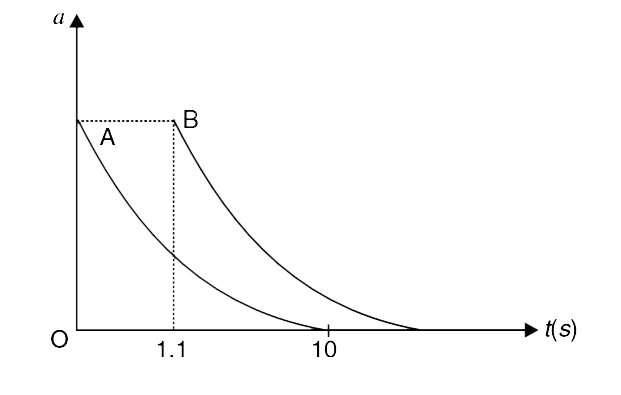 View Solution
View SolutionQuestion 64
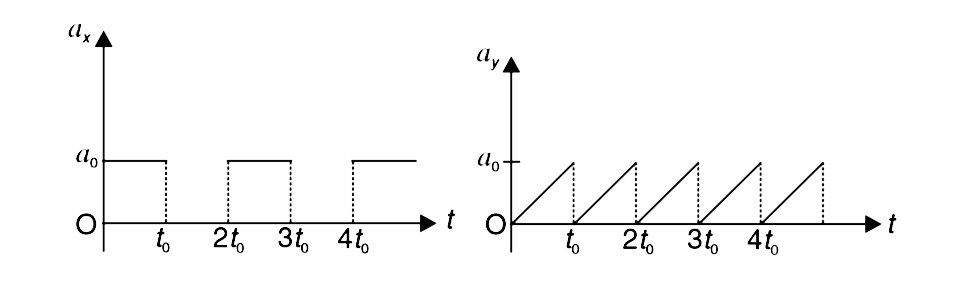
A particle is moving in x–y plane. The x and y components of its acceleration change with time according to the graphs given in figure. At time t=0, its velocity is v0 directed along positive y direction. if a0=v0t0, find the angle that the velocity of the particle makes with x axis at time t=4t0. View SolutionQuestion 65
A particle is moving along positive x direction and experiences a constant acceleration of 4m/s2 in negative x direction. At time t=3 second its velocity was observed to be 10m/s in positive x direction.
(a) Find the distance travelled by the particle in the interval t=0 to t=3s. Also find distance travelled in the interval t=0 to t=7.5s..
(b) Plot the displacement – time graph for the interval t=0 to 7.5s. View SolutionQuestion 66
A bead moves along a straight horizontal wire of length L, starting from the left end with velocity v0. Its retardation is proportional to the distance that remains to the right end of the wire. Find the initial retardation (at left end of the wire) if the bead reaches the right end of the wire with a velocity v02.View SolutionQuestion 67
A ball is projected vertically up from the ground surface with an initial velocity of u=20m/s. O is a fixed point on the line of motion of the ball at a height of H=15m from the ground. Plot a graph showing variation of distance (s) of the ball from the fixed point O, with time (t). [Take g=10m/s2]. Plot the graph for the entire time of flight of the ball.
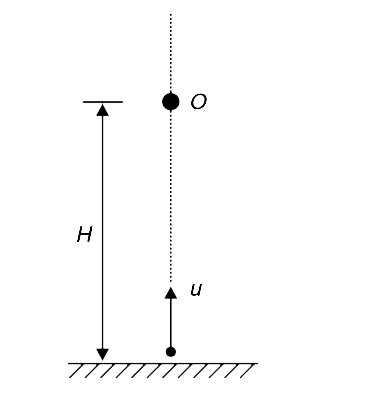 View Solution
View SolutionQuestion 68
Two bodies 1 and 2 of different shapes are released on the surface of a deep pond. The mass of the two bodies are m1=1kg and m2=1.2kg respectively. While moving through water, the bodies experience resistive force given as R=bv, where v is speed of the body and b is a positive constant dependent on shape of the body. For bodies 1 and 2 value of b is 2.5kg/s and 3.0kg/s respectively. Neglect all other forces apart from gravity and the resistive force, while answering following questions : [Hint : acceleration =force/mass]
(i) With what speed v10 and v20 will the two bodies hit the bed of the pond. [Take g=10m/s2]
(ii) Which body will acquire speed equal to half the terminal speed in less time. View SolutionQuestion 69
A prototype of a rocket is fired from the ground. The rocket rises vertically up with a uniform acceleration of 54m/s2. 8 second after the start a small nut gets detached from the rocket. Assume that the rocket keeps rising with the constant acceleration.
(a) What is the height of the rocket at the instant the nut lands on the ground
(b) Plot the velocity – time graph for the motion of the nut after it separates from the rocket till it hits the ground. Plot the same velocity– time graph in the reference frame of the rocket. Take vertically upward direction as positive and g=10m/s2 View SolutionQuestion 70
An elevator starts moving upward with constant acceleration. The position time graph for the floor of the elevator is as shown in the figure. The ceiling to floor distance of the elevator is 1.5 m. At t=2.0s, a bolt breaks loose and drops from the ceiling.
(a) At what time t0 does the bolt hit the floor?
(b) Draw the position time graph for the bolt starting from time t=0. [take g=10m/s2]
 View Solution
View SolutionQuestion 71
At t=0 a projectile is projected vertically up with a speed u from the surface of a peculiar planet. The acceleration due to gravity on the planet changes linearly with time as per equation g=αt where α is a constant.
(a) Find the time required by the projectile to attain maximum height.
(b) Find maximum height attained.
(c) Find the total time of flight. View SolutionQuestion 72
A wet ball is projected horizontally at a speed of u=10m/s from the top of a tower h=31.25m high. Water drops detach from the ball at regular intervals of Δt=1.0s after the throw.
(a) How many drops will detach from the ball before it hits the ground.
(b) How far away the drops strike the ground from the point where the ball hits the ground? View SolutionQuestion 73
Two stones of mass m and M(M>m) are dropped Δt time apart from the top of a tower. Take time t=0 at the instant the second stone is released. Let Δv and Δs be the difference in their speed and their mutual separation respectively. Plot the variation of Δv and Δs with time for the interval both the stones are in flight. [g=10m/s2]View SolutionQuestion 74
A particle is moving in the xy plane on a sinusoidal course determined by y = A sin kx, where k and A are constants. The X component of the velocity of the particle is constant and is equal to v0 and the particle was at origin at time t=0. Find the magnitude of the acceleration of the particle when it is at point having x co ordinate x=π2k.View SolutionQuestion 75
A ball is projected from a cliff of height h=19.2 m at an angle a to the horizontal. It hits an incline passing through the foot of the cliff, inclined at an angle θ to the horizontal. Time of flight of the ball is T=2.4s. Foot of the cliff is the origin of the co-ordinate system, horizontal is x direction and vertical is y direction (see figure). Plot of y co-ordinate vs time and y component of velocity of the ball (vy) vs its x co-ordinate (x) is as shown. x and y are in m and time is in s in the graph. [g=10m/s2]
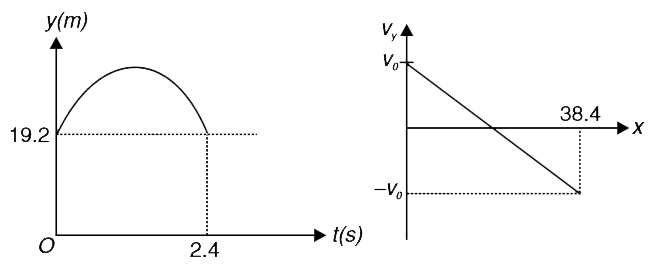
(a) Find the angle of projection a
(b) Find the inclination (θ) of the incline.
(c) If the ball is projected with same speed but at an angle θ(= inclination of incline) to the horizontal, will it hit the incline above or below the point where it struck the incline earlier? View SolutionQuestion 76
A canon can fire shells at speed u. Inclination of its barrel to the horizontal can be changed in steps of Δθ=1∘ ranging from θ1=15∘ to θ2=85∘. Let Rn be the horizontal range for projection angle θ=n∘.
ΔRn=|Rn−Rn+1|
For what value of n the value of ΔRn is maximum? Neglect air resistance.
(ii) A small water sprinkler is in the shape of a hemisphere with large number of uniformly spread holes on its surface. It is placed on ground and water comes out of each hole with speed u. Assume that we mentally divide the ground into many small identical patches – each having area ΔS. What is the distance of a patch from the sprinkler which receives maximum amount of water ?View SolutionQuestion 77
A gun fires a large number of bullets upward. Due to shaking of hands some bullets deviate as much as 1° from the vertical. The muzzle speed of the gun is 150m/s and the height of gun above the ground is negligible. The radius of the head of the person firing the gun is 10cm. You can assume that acceleration due to gravity is nearly constant for heights involved and its value is g=10m/s2. The gun fires 1000 bullets and they fall uniformly over a circle of radius r. Neglect air resistance. You can use the fact sinθ≅θ when θ is small.
(a) Find the approximate value of r. (b) What is the probability that a bullet will fall on the person’s head who is firing? View SolutionQuestion 78
Three stones are projected simultaneously with same speed u from the top of a tower. Stone 1 is projected horizontally and stone 2 and stone 3 are projected making an angle q with the horizontal as shown in fig. Before stone 3 hits the ground, the distance between 1 and 2 was found to increase at a constant rate u.
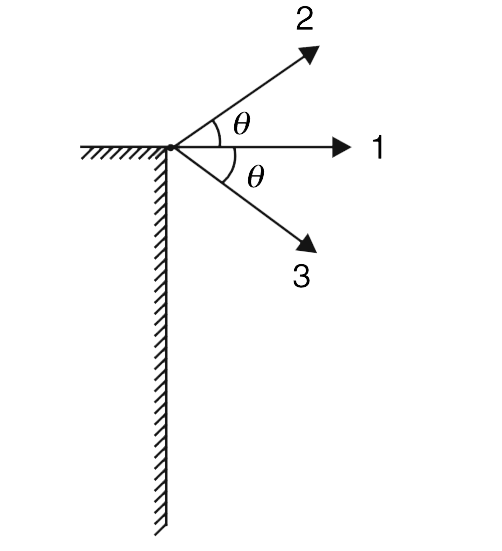
(a) Find θ
(b) Find the rate at which the distance between 2 and 3 increases. View SolutionQuestion 80
A wall OP is inclined to the horizontal ground at an angle α. Two particles are projected from points A and B on the ground with same speed (u) in directions making an angle θ to the horizontal (see figure). Distance between points A and B is x0=24m. Both particles hit the wall elastically and fall back on the ground. Time of flight (time required to hit the wall and then fall back on to the ground) for particles projected from A and B are 4 s and 2 s respectively. Both the particles strike the wall perpendicularly and at the same location. [In elastic collision, the velocity component of the particle that is perpendicular to the wall gets reversed without change in magnitude]
(a) Calculate maximum height attained by the particle projected from A.
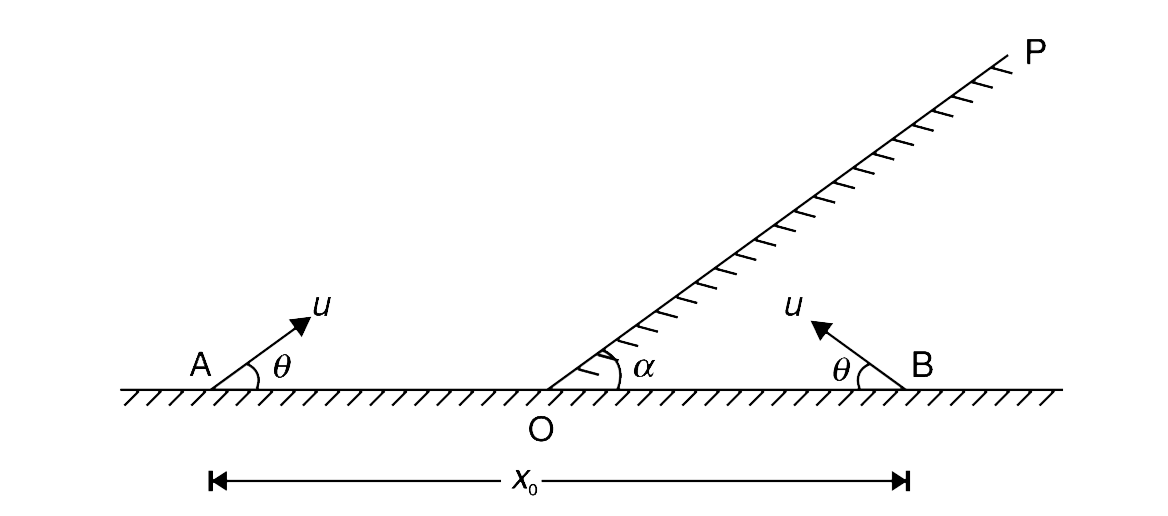
(b) Calculate the inclination of the wall to the horizontal (α)[g=10m/s2] View SolutionQuestion 81
AB is a pipe fixed to the ground at an inclination of 37°. A ball is projected from point O at a speed of u=20m/s at an angle of 53° to the horizontal and it smoothly enters into the pipe with its velocity parallel to the axis of the pipe. [Takeg=10ms–2]
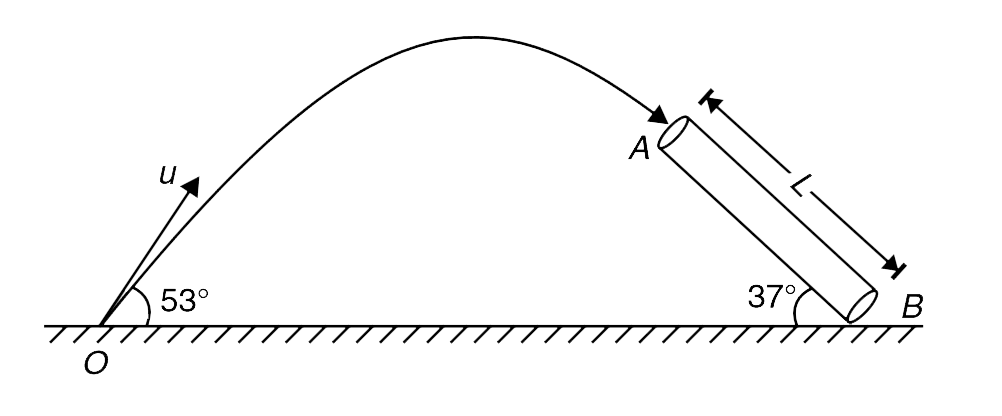
(a) Find the length L of the pipe
(b) Find the distance of end B of the pipe from point O. View SolutionQuestion 82
(a) A boy throws several balls out of the window of his house at different angles to the horizontal. All balls are thrown at speed u=10m/s and it was found that all of them hit the ground making an angle of 45° or larger than that with the horizontal. Find the height of the window above the ground [take g=10m/s2]
(b) A gun is mounted on an elevated platform AB. The distance of the gun at A from the edge B is AB=960m. Height of platform is OB=960m. The gun can fire shells with a velocity of u=100m/s at any angle. What is the minimum distance (OP) from the foot of the platform where the shell of gun can reach ?
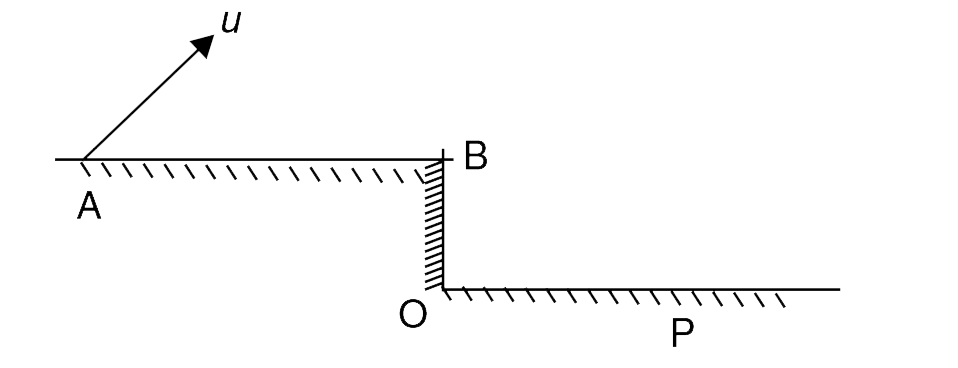 View Solution
View SolutionQuestion 83
An object A is kept fixed at the point x=3m and y=1.25m on a plank p raised above the ground . At time t=0 the plank starts moving along the +x direction with an acceleration 1.5m/s2. At the same instant a stone is projected from the origin with a velocity →u as shown . A stationary person on the ground observes the stone hitting the object during its downward motion at an angle 45(∘) to the horizontal . All the motions are in the X−Yplane . Find →u and the time after which the stone hits the object . Take g=10m/s
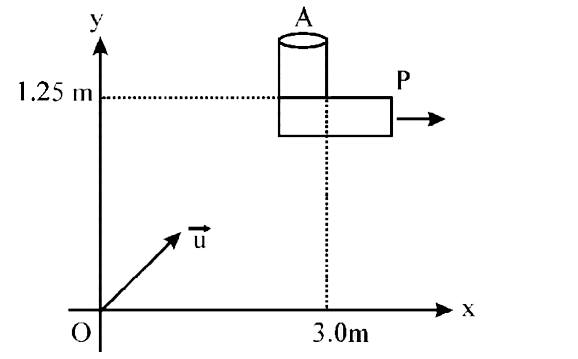 View Solution
View SolutionQuestion 84
(a) A particle is thrown from a height h horizontally towards a vertical wall with a speed v as shown in the figure. If the particle returns to the point of projection after suffering two elastic collisions, one with the wall and another with the ground, find the total time of flight. [Elastic collision means the velocity component perpendicular to the surface gets reversed during collision.]
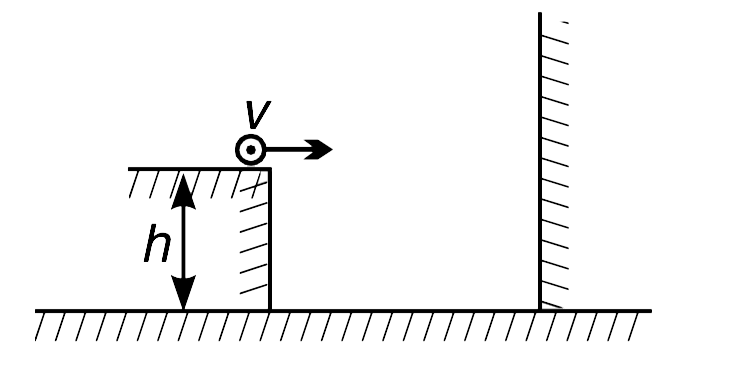
(b) Touching a hemispherical dome of radius R there is a vertical tower of height H=4R. A boy projects a ball horizontally at speed u from the top of the tower. The ball strikes the dome at a height R2 from ground and rebounds. After rebounding the ball retraces back its path into the hands of the body. Find u.
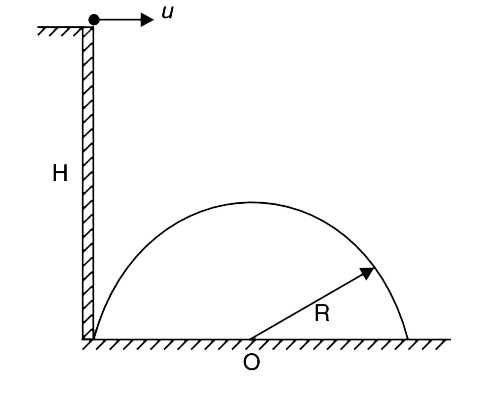 View Solution
View SolutionQuestion 85
A city bus has a horizontal rectangular roof and a rectangular vertical windscreen. One day it was raining steadily and there was no wind.
(a) Will the quantity of water falling on the roof in unit time be different for the two cases (i) the bus is still (ii) the bus is moving with speed v on a horizontal road ?
(b) Draw a graph showing the variation of quantity of water striking the windscreen in unit time with speed of the bus (v)View SolutionQuestion 86
A truck is travelling due north descending a hill of slope angle θ=tan–1(0.1) at a constant speed of 90km/hr. At the base of the hill there is a gentle curve and beyond that the road is level and heads 30∘ east of north. A south bound police car is travelling at 80km/hr along the level road at the base of the hill approaching the truck. Find the velocity of the truck relative to police car in terms of unit vectors ˆi,ˆj and ˆk . Take x axis towards east, y axis towards north and z axis vertically upwards. View SolutionQuestion 87
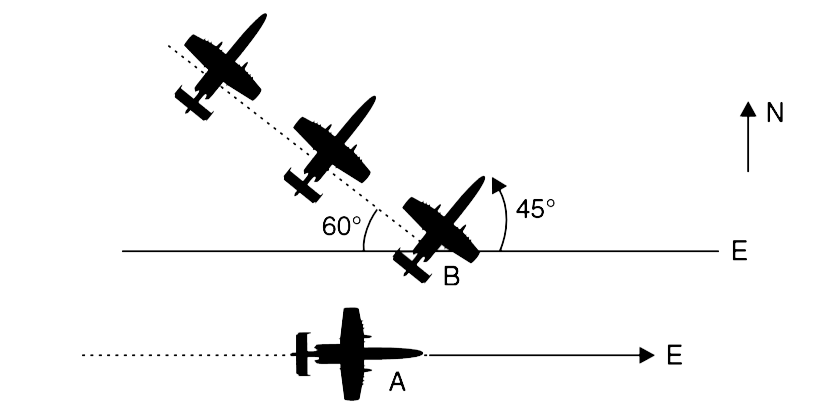
Two persons A and B travelling at 60km/hr–1 in their cars moving in opposite directions on a straight road observe an airplane. To the person A, the airplane appears to be moving perpendicular to the road while to the observe B the plane appears to cross the road making an angle of 45°.
(a) At what angle does the plane actually cross the road (relative to the ground).
(b) Find the speed of the plane relative to the ground.View SolutionQuestion 88
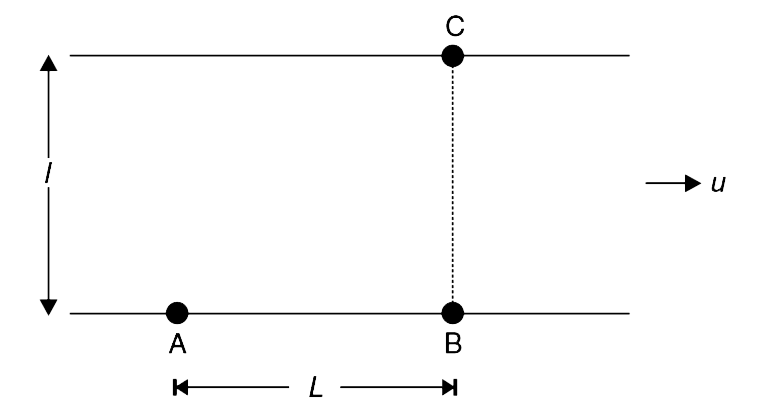
Two friends A and B are standing on a river bank L distance apart. They have decided to meet at a point C on the other bank exactly opposite to B. Both of them start rowing simultaneously on boats which can travel with velocity V=5km/hr in still water. It was found that both reached at C at the same time. Assume that path of both the boats are straight lines. Width of the river is l=3.0km and water is flowing at a uniform speed of u=3.0km/hr.
(a) In how much time the two friends crossed the river.
(b) Find L. View SolutionQuestion 89
On a frictionless horizontal surface , assumed to be the x−y plane , a small trolley A is moving along a straight line parallel to the y−aξs( see figure) with a constant velocity of (√3−1)m/s . At a particular instant , when the line OA makes an angle of 45(∘) with the x−aξs , a ball is thrown along the surface from the origin O. Its velocity makes an angle ϕ with the x−aξsandithitsthetrol≤y.<br>(a)Themotionoftheballisobservedomtheameofthetrol≤y.Calca––tethe∠thetamadebythevelocity→−−→roftheballwiththe x-axis in this frame .
(b) Find the speed of the ball with respect to the surface , if ϕ=(4θ)/(4).
 View Solution
View SolutionQuestion 90
A large , heavy box is sliding without friction down a smooth plane of inclination θ . From a point P on the bottom of the box , a particle is projected inside the box . The initial speed of the particle with respect to the box is u , and the direction of projection makes an angle α with the bottom as shown in Figure .
(a) Find the distance along the bottom of the box between the point of projection p and the point Q where the particle lands . ( Assume that the particle does not hit any other surface of the box . Neglect air resistance .)
(b) If the horizontal displacement of the particle as seen by an observer on the ground is zero , find the speed of the box with respect to the ground at the instant when particle was projected .
 View Solution
View SolutionQuestion 91
A ball is projected in vertical x–y plane from a car moving along horizontal x direction. The car is speeding up with constant acceleration. Which one of the following trajectory of the ball is not possible in the reference frame attached to the car? Give reason for your answer. Explain the condition in which other trajectories are possible. Consider origin at the point of projection.
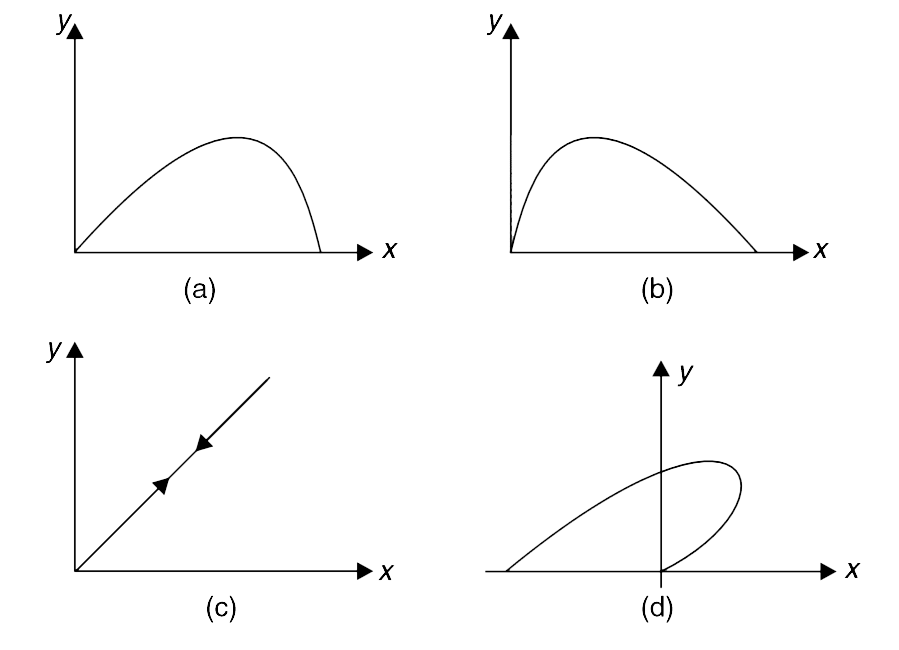 View Solution
View SolutionQuestion 92
A boy standing on a cliff 50 m high throws a ball with speed 40m/s directly aiming towards a man standing on ground at B. At the same time the man at B throws a stone with a speed of 10m/s directly aiming towards the boy.
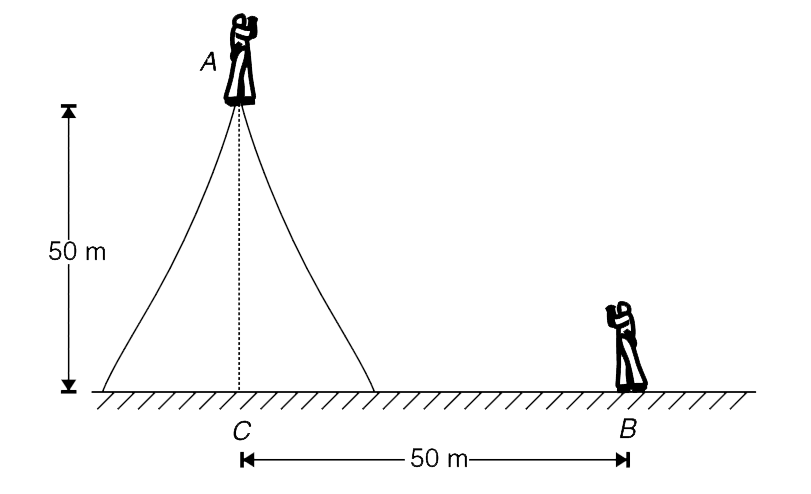
(a) Will the ball and the stone collide? If yes, at what time after projection?
(b) At what height above the ground the two objects collide?
(c) Draw the path of ball in the reference frame of the stone. View SolutionQuestion 93
A man walking downhill with velocity V0 finds that his umbrella gives him maximum protection from rain when he holds it such that the stick is perpendicular to the hill surface. When the man turns back and climbs the hill with velocity V0, he finds that it is most appropriate to hold the umbrella stick vertical. then the actual speed of raindrops in terms of V0 is : (the inclination of the hill is θ=37∘)
 View Solution
View SolutionQuestion 94
There are two hills A and B and a car is travelling towards hill A along the line joining the two hills. Car is travelling at a constant speed u. There is a wind blowing at speed u in the direction of motion of the car (i.e., from hill B to A). When the car is at a distance x1 from A and x2 from B it sounds horn (for very short interval). Driver hears the echo of horn from both the hills at the same time.
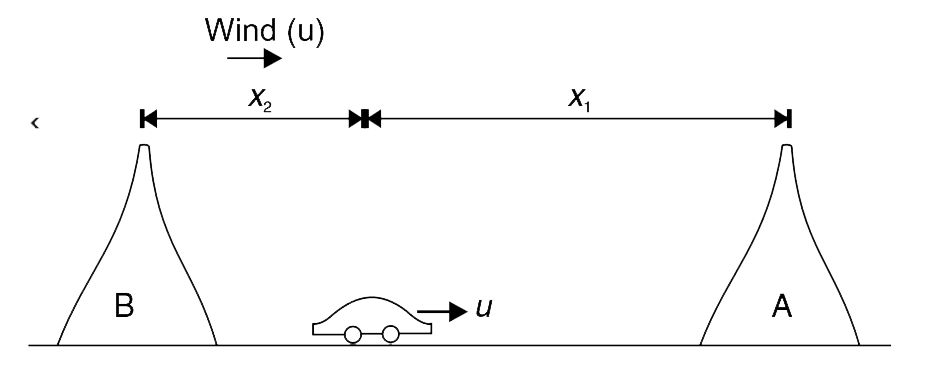
Find the ratio x1x2 taking speed of sound in still air to ve V. View SolutionQuestion 95
The figure shows a square train wagon ABCD which has a smooth floor and side length of 2 L. The train is mov- ing with uniform acceleration (a) in a direction parallel to DA. A 'ball is rolled along the floor with a veloci- ty u, parallel to AB, with respect to the wagon. The ball passes through the centre of the wagon floor. At the instant it is at the centre, brakes are CD AB u 2 L 2 Lapplied and the train begins to retard at a uniform rate that is equal to its previous acceleration (a)
(a) Will the ball hit the wall BC or wall CD or the corner C?
(b) What is speed of the ball, relative to the wagon at the instant it hits a wall ?
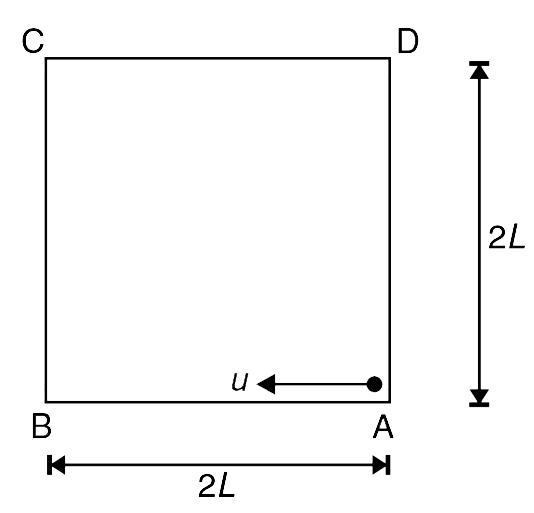 View Solution
View SolutionQuestion 96
Five particles are projected simultaneously from the top of a tower that is h=32m high. The initial velocities of projection are as shown in figure. Velocity of 2 and 5 are horizontal.
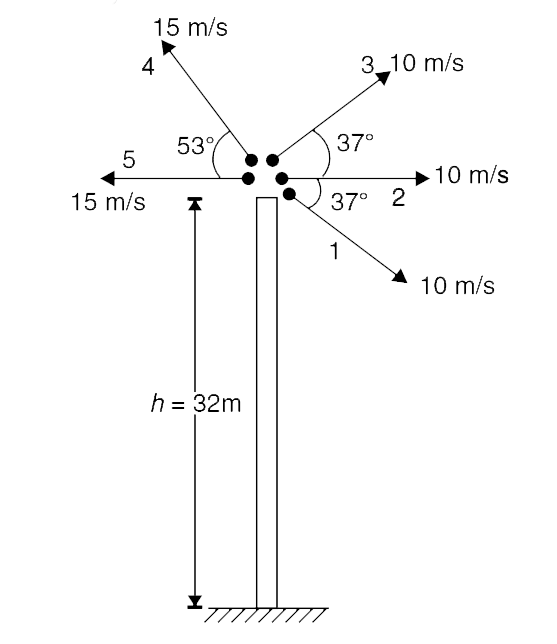
(a) Which particle will hit the ground first?
(b) Separation between which two particles is maximum at the instant the first particle hits the ground?
(c) Which two particles are last and last but one to hit the ground? Calculate the distance between these two particles (still in air), at a time 0.3s after the third particle lands on ground.
[g=10m/s2,tan37∘=34] View SolutionQuestion 97
From the top of a long smooth incline a small body A is projected along the surface with speed u. Simultaneously, another small object B is thrown horizontally with velocity v=10m/s, from the same point. The two bodies travel in the same vertical plane and body B hits body A on the incline. If the inclination angle of the incline is θ=cos−1(45) find
(a) the speed u with which A was projected.
(b) the distance from the point of projection, where the two bodies collide.
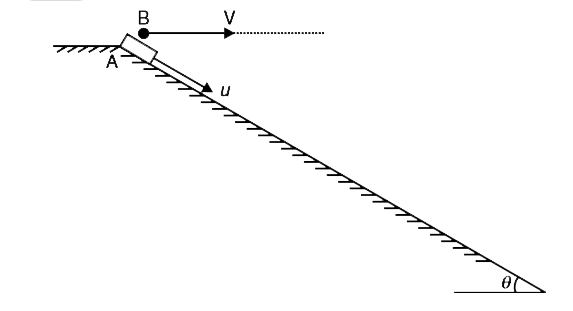 View Solution
View SolutionQuestion 98
A man is on straight road AC, standing at A. He wants to get to a point P which is in field at a distance ‘d’ off the road (see figure). Distance AB is l=50. The man can run on the road at a speed v1=5m/s and his speed in the field is v2=3m/s.
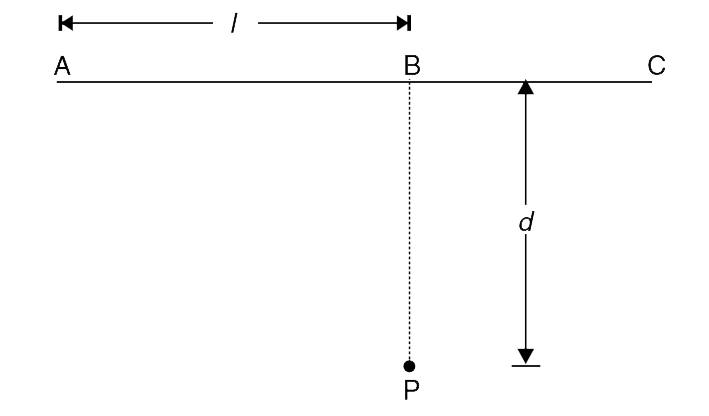
(a) Find the minimum value of ‘d’ for which man can reach point P in least possible time by travelling only in the field along the straight line AP.
(b) If value of ‘d’ is half the value found in (a), what length the man must run on the road before entering the field, in order to reach ‘P’ in least possible time. View SolutionQuestion 99
Two particles, A and B are moving in concentric circles in anticlockwise sense in the same plane with radii of the circles being γA=1.0m and γB=2.0m respectively. The particles move with same angular speed of ω=4rad/s. Find the angular velocity of B as observed by A if
(a) Particles lie on a line passing through the centre of the circle.
(b) Particles lie on two perpendicular lines passing through the centre. View SolutionQuestion 100
(a) An unpowered rocket is in flight in air. At a moment the tracking radar gives following data regarding the rocket. r = distance of the rocket from the radar = 4000m,drdt=0,dθdt=1.8deg/sec, where θ is the angle made by position vector of the rocket with respect to the vertical.
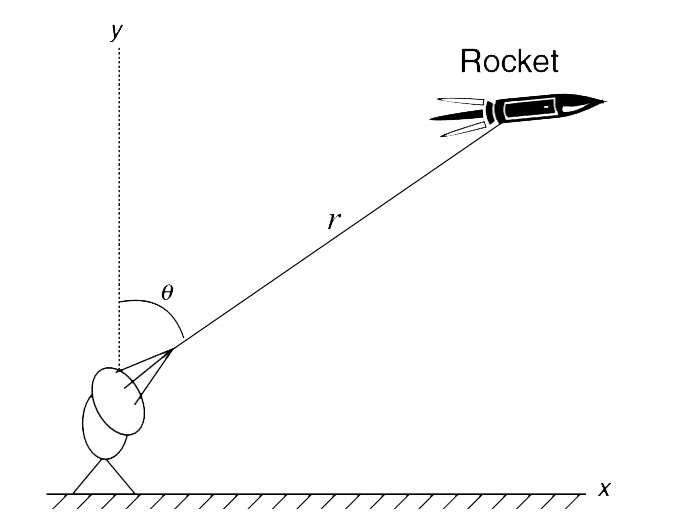
(a) Neglect atmospheric resistance and take g=9.8m/s2 at the concerned height. Neglect height of radar. Calculate the height of the rocket above the ground.
(b) Two points A and B are moving in X - Y plane with constant velocity of VA=(6ˆi−9ˆj)m/s and VB=(ˆi+ˆj)m/s respectively. At time t=0 they are 15 m apart and both of them lie on y axis with A lying away on positive Y axis with respect to B. What is the angular velocity of A with respect to B at t=1s? View SolutionQuestion 101
A stone is projected horizontally with speed u from the top of a tower of height h.
(a) Calculate the radius of curvature of the path of the stone at the point where its tangential and radial accelerations are equal.
(b) What shall be the height (h) of the tower so that radius of curvature of the path is always less than the value obtained in (a) above. View SolutionQuestion 102
A stick of length L=2.0m is leaned against a wall as shown. It is released from a position when θ=60∘. The end A of the stick remains in contact with the wall and its other end B remains in contact with the floor as the stick slides down. Find the distance travelled by the centre of the stick by the time it hits the floor.
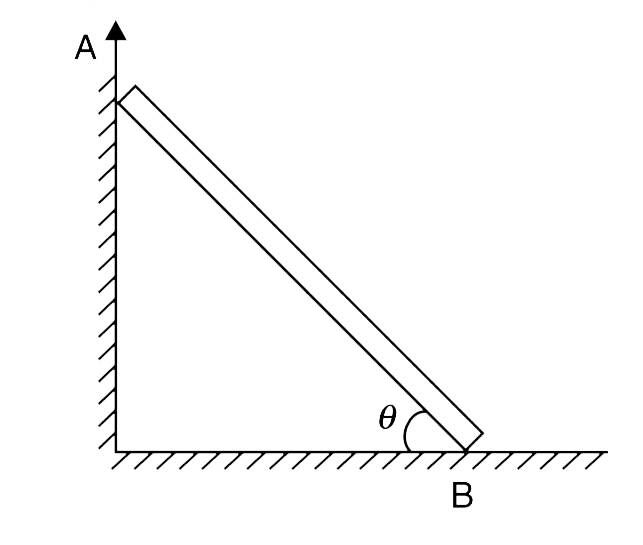 View Solution
View SolutionQuestion 103
(a) A line PQ is moving on a fixed circle of radius R. The line has a constant velocity v perpendicular to itself. Find the speed of point of intersection (A) of the line with the circle at the moment the line is at a distance d=R/2 from the centre of the circle.
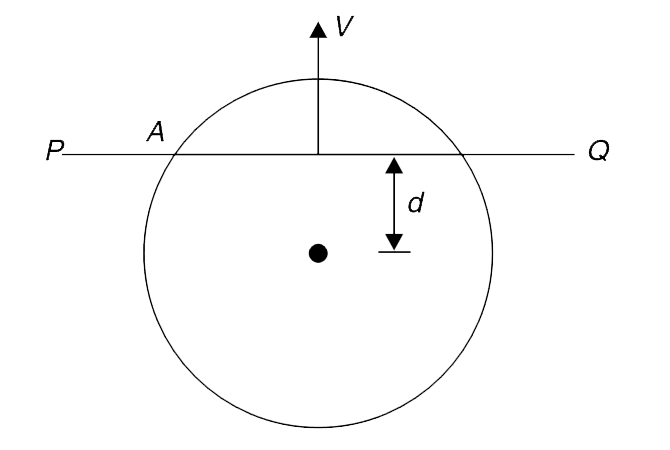
(b) In the figure shown a pin P is confined to move in a fixed circular slot of radius R. The pin is also constrained to remains inside the slot in a straight arm O'A. The arm moves with a constant angular speed w about the hinge O'. What is the acceleration of point P ?
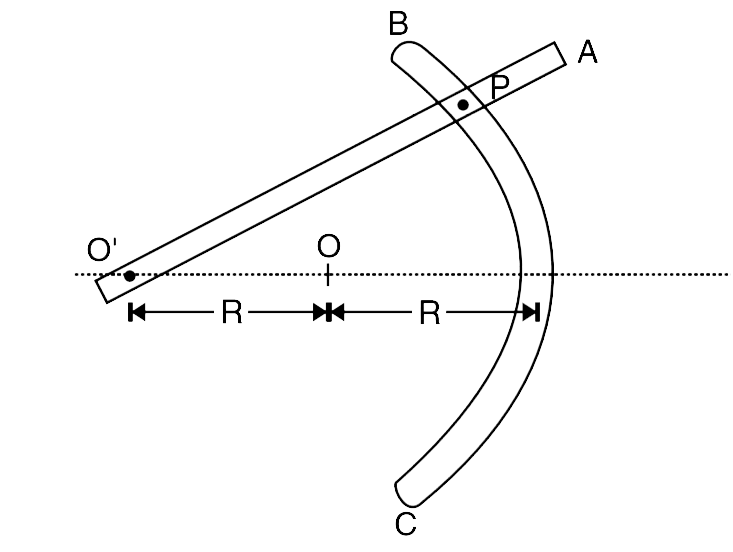 View Solution
View SolutionQuestion 104
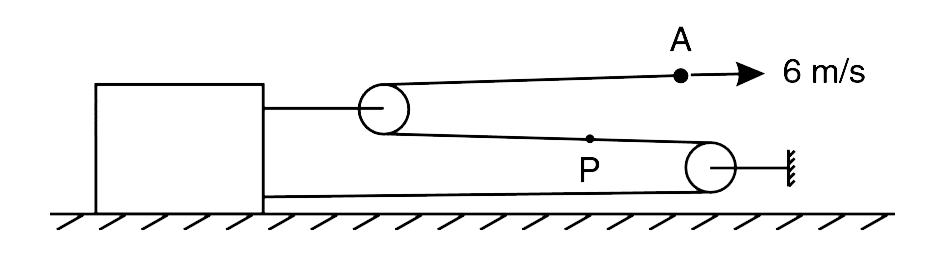
A flexible inextensible cord supports a mass M as shown in figure. A1,A2 and B are small pulleys in contact with the cord. At time t=0 cord PQ is horizontal and A1,A2 start moving vertically down at a constant speed of v1, whereas B moves up at a constant speed of v2. Find the velocity of mass M as a function of time.
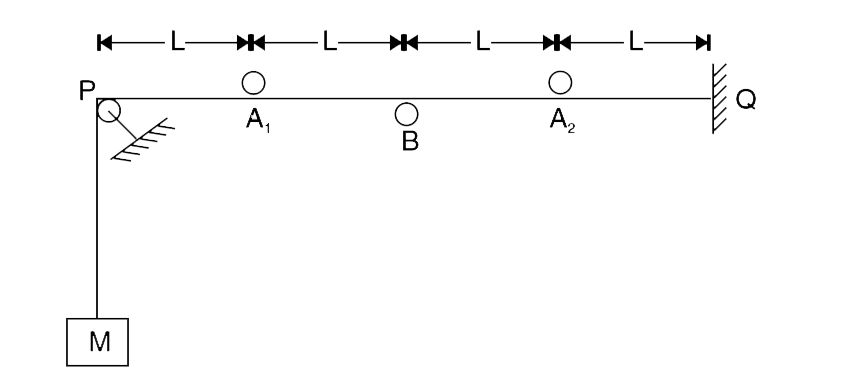 View Solution
View SolutionQuestion 106
A meter stick AB is lying on a horizontal table. Its end A is pulled up so as to move it with a constant velocity VA=4ms–1 along a vertical line. End B slides along the floor.
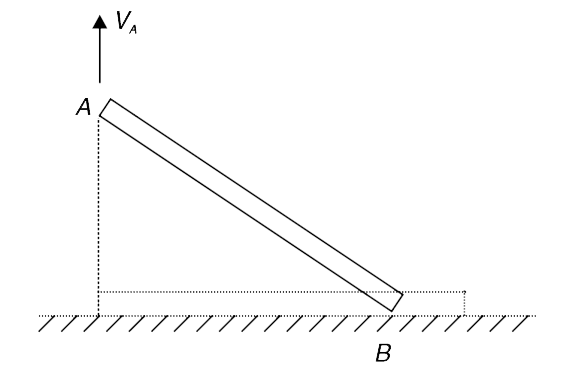
(a) After how much time (t0) speed (VB) of end B becomes equal to the speed (VA) of end A ?
(b) Find distance travelled by the end B in time t0.View SolutionQuestion 107
One end of a rope is fixed at a point on the ceiling the other end is held close to the first end so that the rope is folded. The second end is released from this position. Find the speed at which the fold at F is descending at the instant the free end of the rope is going down at speed V.
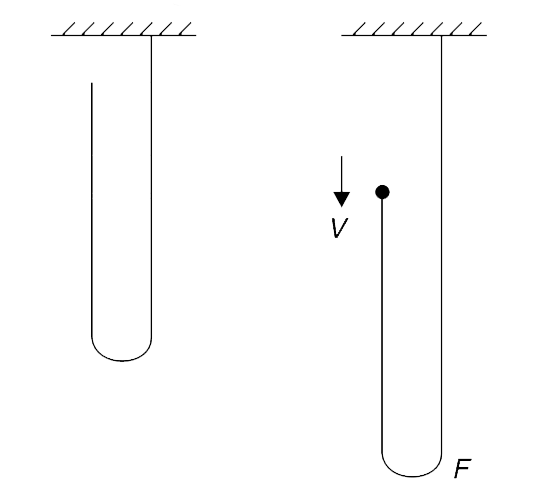 View Solution
View SolutionQuestion 108
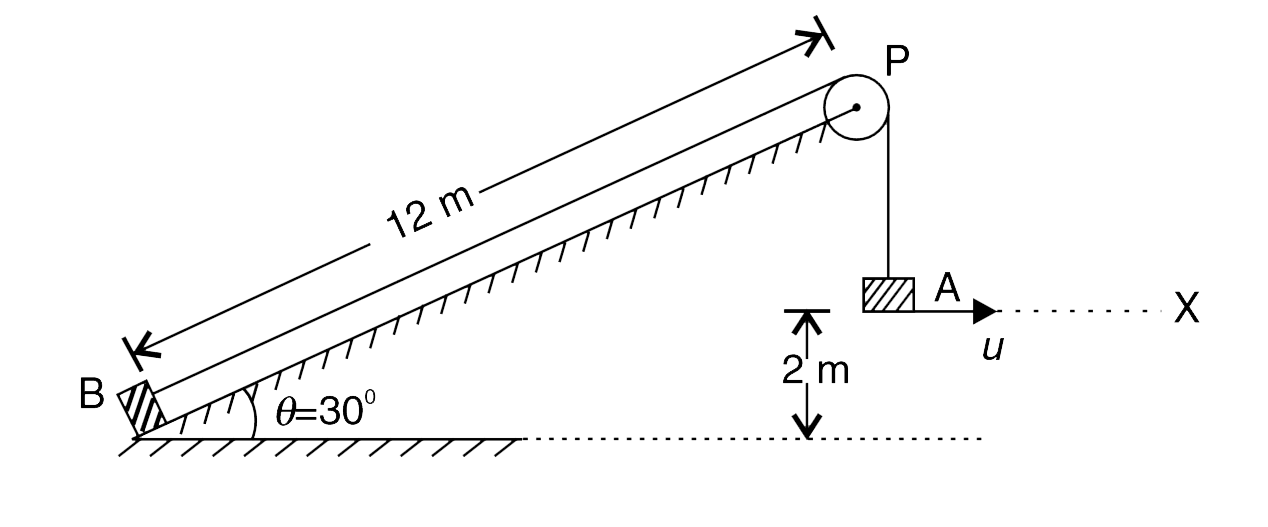
Block A rests on inclined surface of wedge B which rests on a horizontal surface. The block A is connected to a string, which passes over a pulley P (fixed rigidly to the wedge B) and its other end is securely fixed to a wall at Q. Segment PQ of the string is horizontal and Q is at a large distance from P. The system is let go from rest and the wedge slides to right as A moves on its inclined face. Find the distance travelled by A by the time it reaches the bottom of the inclined surface.
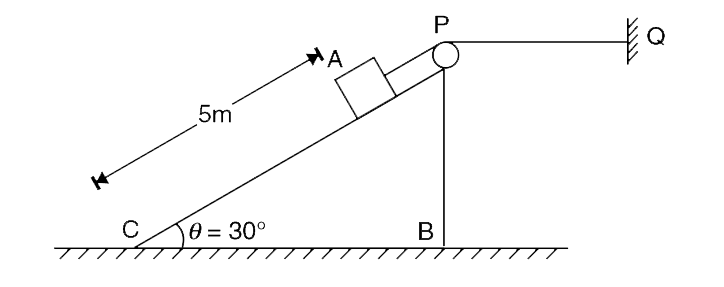 View Solution
View SolutionQuestion 110
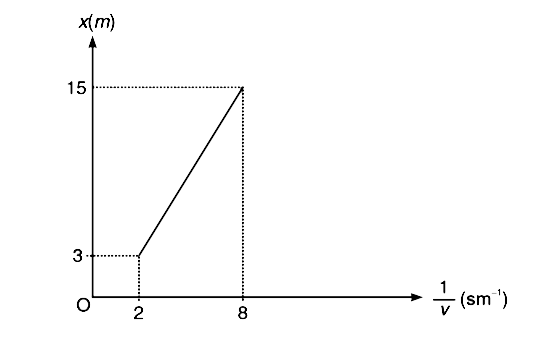
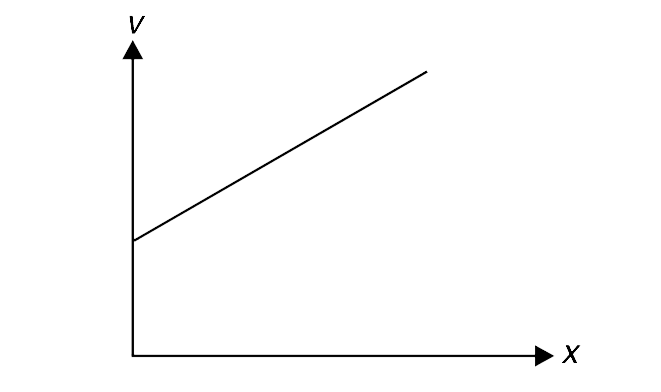
A car manufacturer usually tells a optimum speed (V0) at which the car should be driven to get maximum mileage. In order to find the optimum speed for a new model, an engineer of the car company experimented a lot and finally plotted a graph between the extreme time t (defined as number of hours a tank full of petrol lasts) vs the constant speed V at which car was run.
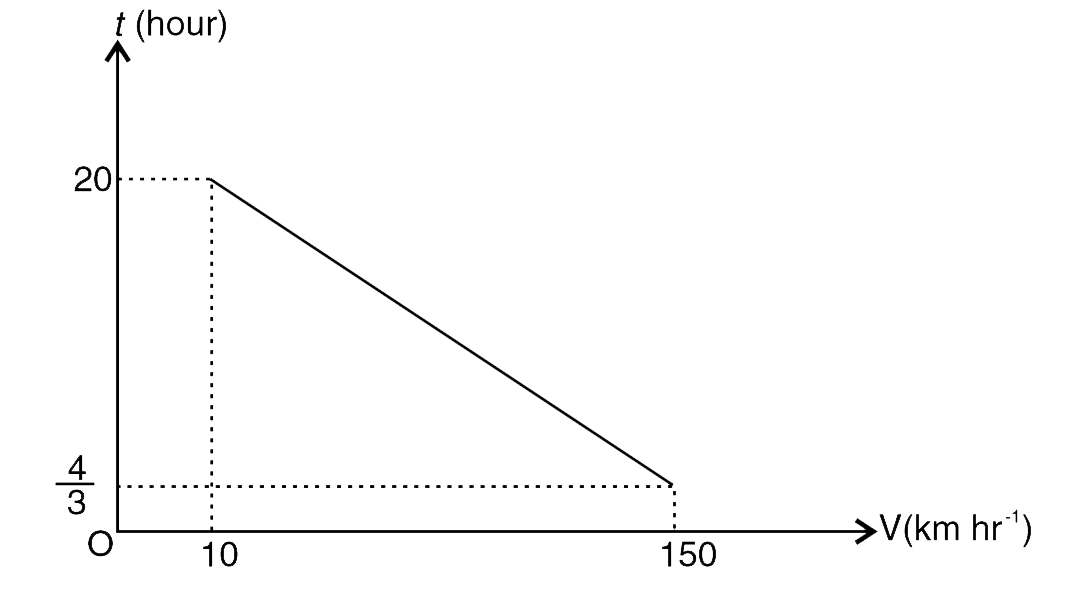
(a) Calculate the optimum speed V0 for this new model.
(b) If the fuel tank capacity of this car is 50 litre, what maximum mileage can be obtained from this car?View SolutionQuestion 111
While starting from a station, a train driver was instructed to stop his train after time T and to cover maximum possible distance in that time.
(a) If the maximum acceleration and retardation for the train are both equal to ‘a’, find the maximum distance it can cover.
(b) Will the train travel more distance if maximum acceleration is ‘a’ but the maximum retardation caused by the brakes is ‘2a’? Find this distance. View SolutionQuestion 112
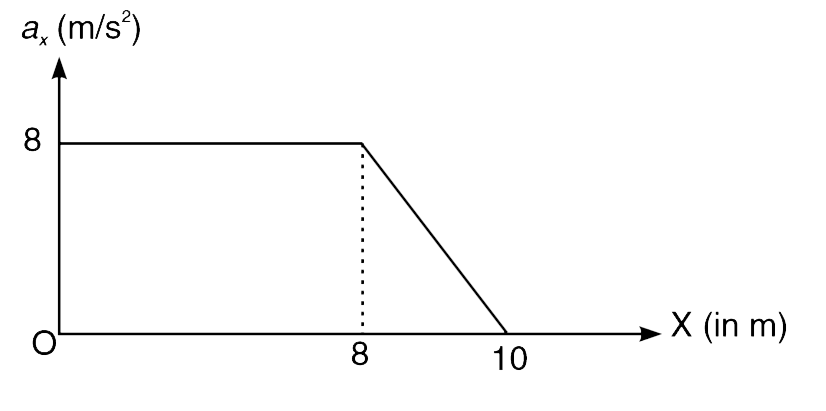
Two particles 1 and 2 start simultaneously from origin and move along the positive X direction. Initial velocity of both particles is zero. The acceleration of the two particles depends on their displacement (x) as shown in fig.
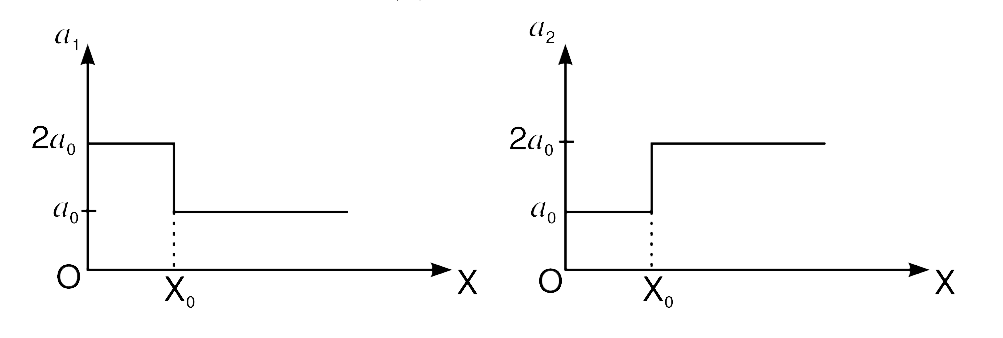
(a) Particles 1 and 2 take t1 and t2 time respectively for their displacement to become x0. Find t2t1.
(b) Which particle will cover 2x0 distance in least time? Which particle will cross the point x=2x0 with greater speed?
(c) The two particles have same speed at a certain time after the start. Calculate this common speed in terms of a0 and x0.View SolutionQuestion 113
A cat is following a rat. The rat is running with a constant velocity u. The cat moves with constant speed v with her velocity always directed towards the rat. Consider time to be t=0 at an instant when both are moving perpendicular to each other and separation between them is L. View SolutionQuestion 114
(a) Prove that bodies starting at the same time t=0 from the same point, and following frictionless slopes in different directions in the same vertical plane, all lie in a circle at any subsequent time.
(b) Using the above result do the following problem. A point P lies above an inclined plane of inclination angle α. P is joined to the plane at number of points by smooth wires, running in all possible directions. Small bodies (in shape of beads) are released from P along all the wires simultaneously. Which body will take least time to reach the plane.
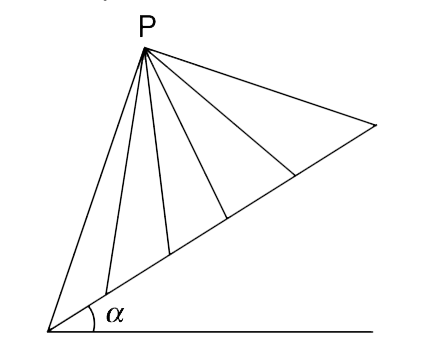 View Solution
View SolutionQuestion 115
The acceleration due to gravity near the surface of the earth is →g . A ball is projected with velocity →u from the ground.
(a) Express the time of flight of the ball.
(b) Write the expression of average velocity of the ball for its entire duration of flight. Express both answers in terms of →u and →g. View SolutionQuestion 116
A ball is projected from point O on the ground. It hits a smooth vertical wall AB at a height h and rebounds elastically. The ball finally lands at a point C on the ground. During the course of motion, the maximum height attained by the ball is H.
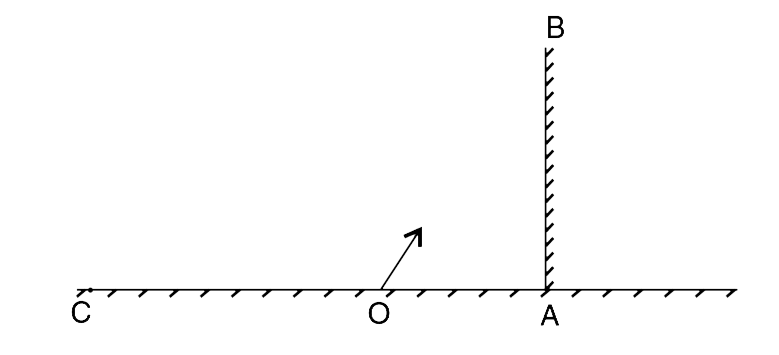
(a) Find the ratio hH if OAOC=13
(b) Find the magnitude of average acceleration of the projectile for its entire course of flight if it was projected at an angle of 45∘ to the horizontal. View SolutionQuestion 117
A boy can throw a ball up to a speed of u=30m/s . He throws the ball many a times, ensuring that maximum height attained by the ball in each throw is h=20m. Calculate the maximum horizontal distance at which a ball might have landed from the point of projection. Neglect the height of the boy. [g=10m/s2]View SolutionQuestion 118
A valley has two walls inclined at 37° and 53° to the horizontal. A particle is projected from point P with a velocity of u=20m/s along a direction perpendicular to the incline wall OA. The Particle hits the incline surface RB perpendicularly at Q. Take g=10m/s2 and find:
(a) The time of flight of the particle.
(b) Vertical height h of the point P from horizontal surface OR.
[tan37∘=34]
 View Solution
View SolutionQuestion 119
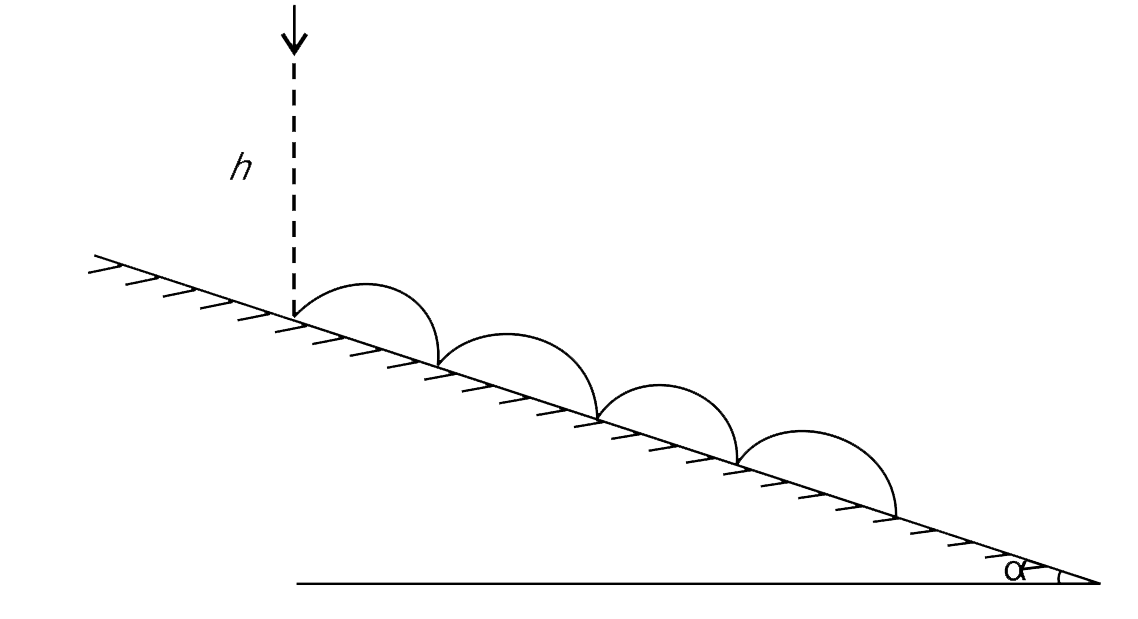
A ball is released in air above an incline plane inclined at an angle α to the horizontal. After falling vertically through a distance h it hits the incline and rebounds. The ball flies in air and then again makes an impact with the incline. This way the ball rebounds multiple times. Assume that collisions are elastic, i.e., the ball rebound without any loss in speed and in accordance to the law of reflection.
(a) Distance between the points on the incline where the ball makes first and second impact is l1 and distance between points where the ball makes second and third impact is l2. Which is large l1 or l2?
(b) Calculate the distance between the points on the incline where the ball makes second and fifth impact. View SolutionQuestion 120
A terrorist ‘A’ is walking at a constant speed of 7.5km/hr due West. At time t=0, he was exactly South of an army camp at a distance of 1 km. At this instant a large number of army men scattered in every possible direction from their camp in search of the terrorist. Each army person walked in a straight line at a constant speed of 6km/hr.
(a) What will be the closest distance of an army person from the terrorist in this search operation?
(b) At what time will the terrorist get nearest to an army person? View SolutionQuestion 121
A large wedge BCD, having its inclined surface at an angle θ=45∘ to the horizontal, is travelling horizontally leftwards with uniform velocity u=10m/s
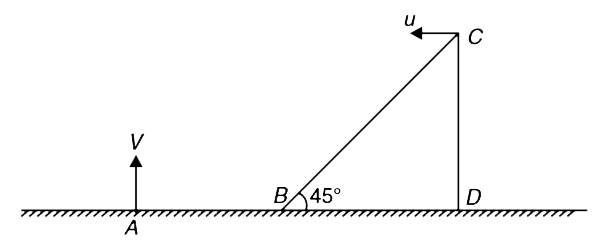
At some instant a particle is projected vertically up with speed V=20m/s from point A on ground lying at some distance right to the lower edge B of the wedge. The particle strikes the incline BC normally, while it was falling. [g=10m/s2]
(a) Find the distance AB at the instant the particle was projected from A.
(b) Find the distance of lower edge B of the wedge from point A at the instant the particle strikes the incline.
(c) Trace the path of the particle in the reference frame attached to the wedge. View SolutionQuestion 122
The speed of river current close to banks is nearly zero. The current speed increases linearly from the banks to become maximum (=V0) in the middle of the river. A boat has speed ‘u’ in still water. It starts from one bank and crosses the river. Its velocity relative to water is always kept perpendicular to the current. Find the distance through which the boat will get carried away by the current (along the direction of flow) while it crosses the river. Width of the river is l. View SolutionQuestion 123
A water sprinkler is positioned at O on horizontal ground. It issues water drops in every possible direction with fixed speed u. This way the sprinkler is able to completely wet a circular area of the ground (see fig). A horizontal wind starts blowing at a speed of u2√2.Mark the area on the ground that the sprinkler will now be able to wet.
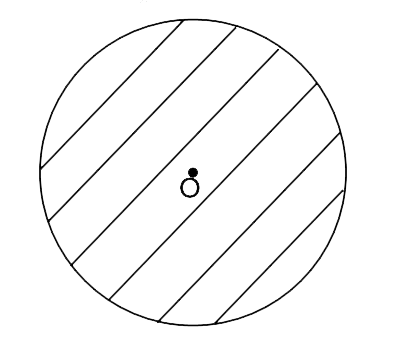 View Solution
View SolutionQuestion 124
A cylinder of radius R has been placed in a corner as shown in the fig. A wedge is pressed against the cylinder such that its inclined surfaces touches the cylinder at a height of 2R5 from thw ground. Now the wedge is pushed to the left at a constant speed V=15m/s. With what speed will the cylinder move?
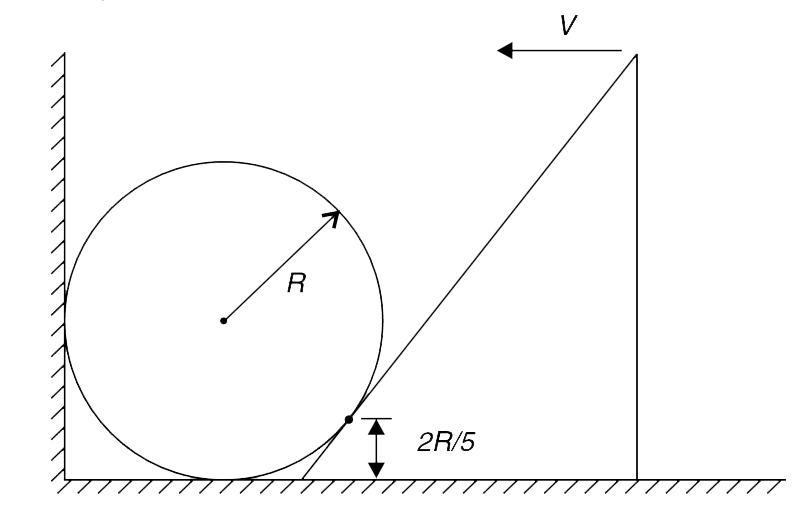 View Solution
View SolutionQuestion 125
The entrance to a harbour consists of 50m gap between two points A and B such that B is due east of A. Outside the harbour there is a 8km/hr current flowing due east. A motor boat is located 300 m due south of A. Neglect size of the boat for answering following questions-
(a) Calculate the least speed (Vmin) that the motor boat must maintain to enter the harbour.
(b) Show that the course it must steer when moving at Vmin does not depend on the speed of the current. View SolutionQuestion 126
Two small pegs (A and B) are at horizontal and vertical separation b and h respectively. A small block of mass M is suspended with the help of two light strings passing over A and B as shown in fig. The two string are always kept at right angles (i.e., <APB=90∘). Find the minimum possible gravitation potential energy of the mass assuming the reference level at location of peg A. [Hint: the potential energy is minimum when the block is at its lowest position]
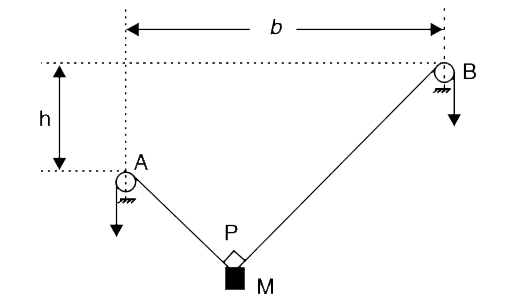 View Solution
View SolutionQuestion 127
A ball of mass m is projected from ground making an angle q to the horizontal. There is a horizontal wind blowing in the direction of motion of the ball. Due to wind the ball experiences a constant horizontal force of mg√3 in direction of its motion. Find θ for which the horizontal range of the ball will be maximum. View SolutionQuestion 129
In the arrangement shown in the figure, the block C begins to move down at a constant speed of 7.5cm/s at time t=0. At the same instant block A is made to start moving down at constant acceleration. It starts at M and its speed is 30cm/s when it reaches N(MN=20cm). Assuming that B started from rest, find its position, velocity and acceleration when block A reaches N.
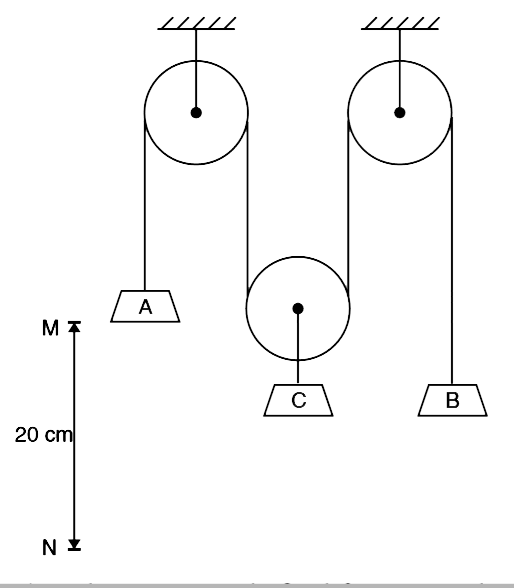 View Solution
View SolutionQuestion 130
A rocket prototype is fired from ground at time t=0 and it goes straight up. Take the launch point as origin and vertically upward direction as positive x direction. The acceleration of the rocket is given by
a=g2−kt2,0<t≤t0
=−g,t>t0
Where t0=√3g2k
(a) Find maximum velocity of the rocket during the up journey.
(b) Find maximum height attained by the rocket.
(c) Find total time of flight.View SolutionQuestion 131
A man standing inside a room of length L rolls a ball along the floor at time t=0. The ball travels at constant speed v relative to the floor, hits the front wall (B) and rebounds back with same speed v. The man catches the ball back at the wall A at time t0. The ball travelled along a straight line relative to the man inside the room. Another observer standing outside the room found that the entire room was travelling horizontally at constant velocity v in a direction parallel to the two walls A and B.

(a) Find the average speed of the ball in the time interval t=0 to t=t0 as observed by the observer outside the room.
(b) If the room has acceleration in the direction of its velocity draw a sketch of the path of the ball as observed by the observer standing outside. Assume that velocity of room was v at the instant the ball was released. View SolutionQuestion 132
There is a tall cylindrical building standing in a field. Radius of the cylinder is R=8m. A boy standing at A (at a distance of 10 m from the centre of the cylindrical base of the building) knows that his friend is standing at B behind the building. The line joining A and B passes through the centre of the base of the building. Distance between A and B is 50 m. A wants to throw a ball to B but he realizes that the building is too tall and he cannot throw the ball over it. He throws the ball at a speed of 20m/s such that his friend at B has to move minimum distance to catch it.
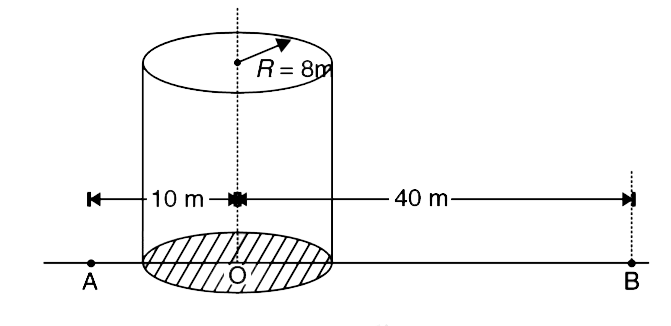
(a) What is the minimum distance that boy at B will have to move to catch the ball?
(b) At what angle to the horizontal does the boy at A throws the ball? Assume that the ball is released and caught at same height above the ground.
[Take g=10m/s2 and sin−1(0.75)≅48.6∘View SolutionQuestion 133
A wet umbrella is held upright (see figure). The man holding it is rotating it about its vertical shaft at an angular speed of ω=5 rad s–1. The rim of the umbrella has a radius of r=0.5 m and it is at a height of H=1.8 m from the floor. The man holding the umbrella gradually increases the angular speed to make it 2ω. Calculate the area of the floor that will get wet due to water drops spun off the rim and hitting the floor. [g=10m/s2].
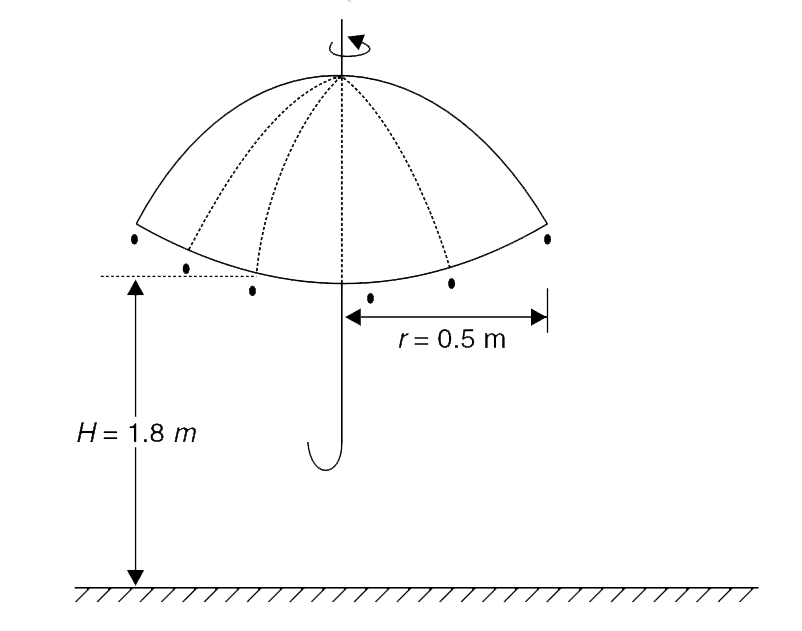 View Solution
View SolutionQuestion 134
A ball is projected vertically up from ground. Boy A standing at the window of first floor of a nearby building observes that the time interval between the ball crossing him while going up and the ball crossing him while going down is t1. Another boy B standing on the second floor notices that time interval between the ball passing him twice (during up motion and down motion) is t2.
(a) Calculate the height difference (h) between the boy B and A.
(b) Assume that the height of boy A from the point of projection of the ball is also equal to h and calculate the speed with which the ball was projected. View SolutionQuestion 135
A stick of length L is dropped from a high tower. An ant sitting at the lower end of the stick begins to crawl up at the instant the stick is released. Velocity of the ant relative to the stick remains constant and is equal to u. Assume that the stick remains vertical during its fall, and length of the stick is sufficiently long.

(a) Calculate the maximum height attained by the ant measured from its initial position.
(b) What time after the start the ant will be at the same height from where it started?View SolutionQuestion 136
Two balls are projected simultaneously from the top of a tall building. The first ball is projected horizontally at speed u1=10m/s and the other one is projected at an angle θ=tan−1(43) to the horizontal with a velocity u2.[g=10m/s2]
(a) Find minimum value of u2(=u0) so that the velocity vector of the two balls can get perpendicular to each other at some point of time during their course of flight. ltbr? (b) Find the time after which velocities of the two balls become perpendicular if the second one was projected with speed u0. View SolutionQuestion 137
There is a large wedge placed on a horizontal surface with its incline face making an angle of 37∘ to the horizontal. A particle is projected in vertically upward direction with a velocity of u=6.5m/s from a point O on the inclined surface. At the instant the particle is projected, the wedge begins to move horizontally with a constant acceleration of a=4m/s2. At what distance from point O will the particle hit the incline surface if
(i) direction of a is along BC?
(ii) direction of a is along AB?
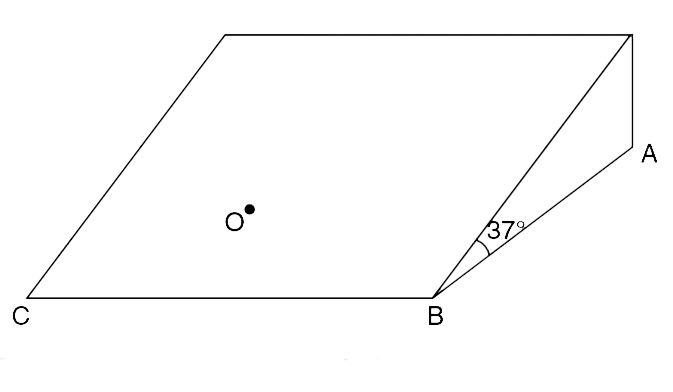 View Solution
View SolutionQuestion 138
The windshield of a truck is inclined at 37∘ to the horizontal. The truck is moving horizontally with a constant acceleration of a=5m/s2. At the instant the velocity of the truck is v0=0.77m/s, an insect jumps from point A on the windshield, with a velocity u=2.64m/s (relative to ground) in vertically upward direction. It falls back at point B on the windshield. Calculate distance AB. Assume that the insect moves freely under gravity and g=10m/s2.
 View Solution
View SolutionQuestion 139
Two persons are pulling a heavy block with the help of horizontal inextensible strings. At the instant shown, the velocities of the two persons are v1 and v2 directed along the respective strings with the strings making an angle of 60∘ between them.
(a) Find the speed of the block at the instant shown.
(b) For what ratio of v1 and v2 the instantaneous velocity of the block will be along the direction of v1.
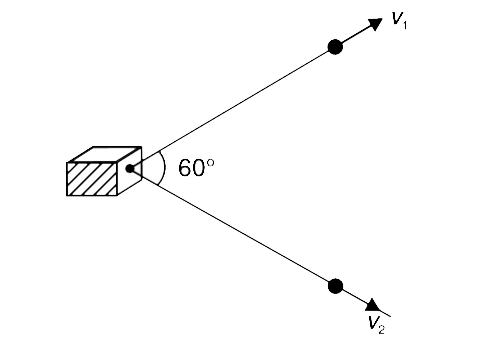 View Solution
View SolutionQuestion 140
A heavy block 'B' is sliding with constant velocity u on a horizontal table. The width of the block is L. There is an insect A at a distance d from the block as shown in the figure. The insect wants to cross to the opposite side of the table. It begins to crawl at a constant velocity v at the instant shown in the figure. Find the least value of v for which the insect can cross to the other side without getting hit by the block.
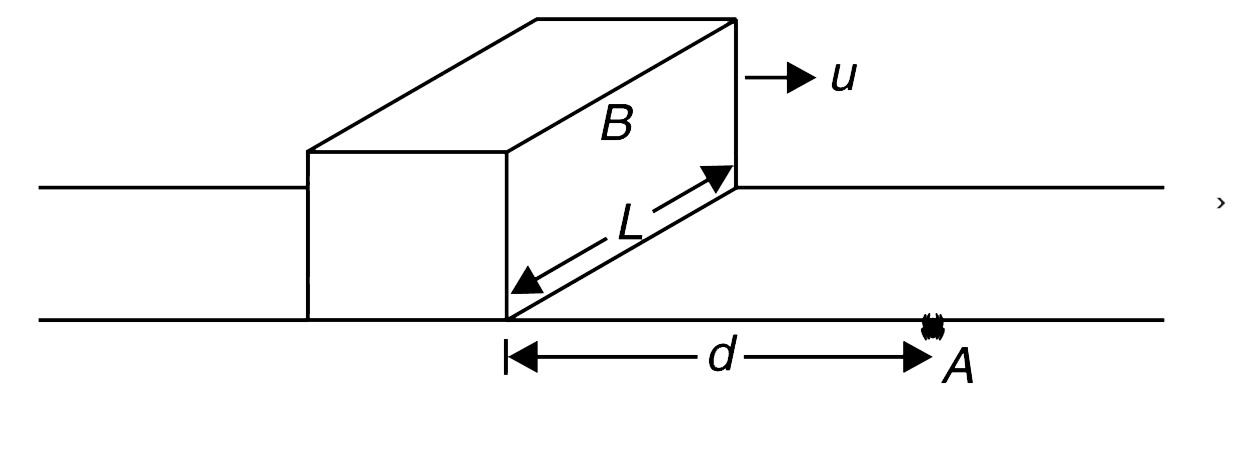 View Solution
View SolutionQuestion 141
A projectile is thrown from ground at a speed v0 at an angle α to the horizontal. Consider point of projection as origin, horizontal direction as X axis and vertically upward as Y axis. Let t be the time when the velocity vector of the projectile becomes perpendicular to its position vector.
(a) Write a quadratic equation in t.
(b) What is the maximum angle a for which the distance of projectile from the point of projection always keeps on increasing? [Hint: Start from the equation you obtained in part (a)]View Solution