Complete Solutions to EXERCISE of chapter INTRODUCTION TO GRAPHS of Class 8 book with complete answers and questions
| Class 8 | MATHS |
| Chapter | INTRODUCTION TO GRAPHS |
| NCERT EXEMPLAR | EXERCISE |
| Number Of Questions | 86 |
| Academic Year | 2023-24 |
Comparison of parts of a whole may be done by a
View SolutionA graph that displays data that changes continuously over periods of time is
View SolutionIn the given graph the coordinates of point x are
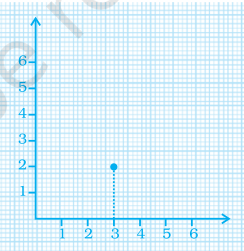
View SolutionIn the given graph the letter that indicates the point (0, 3) is
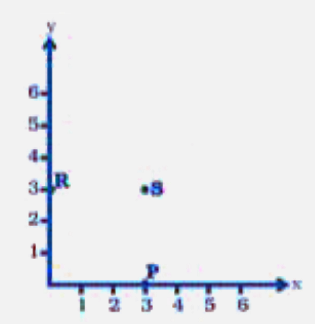
View SolutionThe point (3, 4) is at a distance of
View SolutionA point which lies on both the axis is __________
View SolutionThe coordinates of a point at a distance of 3 units from the x axis and 6 units from the y axis is
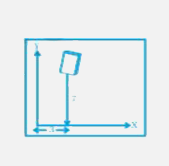
View SolutionIn the given figure the position of the book on the table may be given by
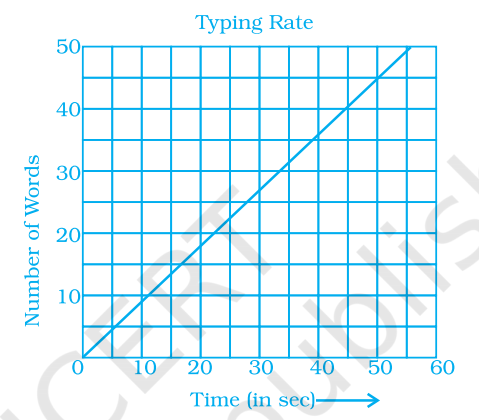
View SolutionData was collected on a student’s typing rate and graph was drawn as shown below. Approximately how many words had this student typed in 30 seconds?
View SolutionWhich graphs of the following represent the table below?
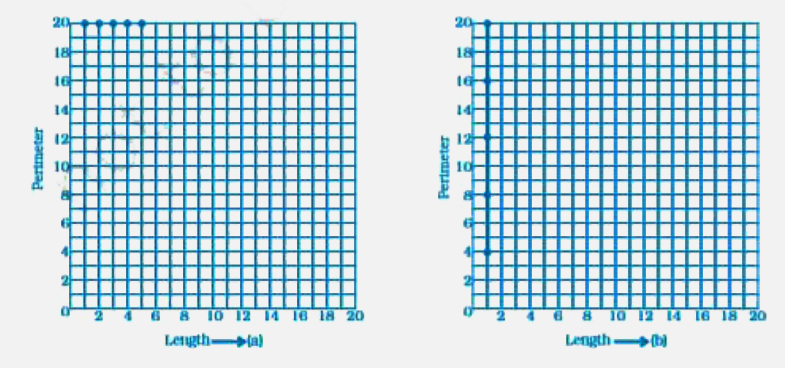
View Solution__________ displays data that changes continuously over periods of time.
View SolutionThe relation between dependent and independent variables is shown through a __________.
View SolutionWe need __________ coordinates for representing a point on the graph sheet.
View SolutionA point in which the x-coordinate is zero and y-coordinate is non zero will lie on the _________
View SolutionThe horizontal and vertical line in a line graph are usually called __________ and __________.
View SolutionThe process of fixing a point with the help of the coordinates is known as __________ of the point.
View SolutionThe distance of any point from the y-axis is the __________ coordinate.
View SolutionAll points with y-coordinate as zero lie on the __________.
View SolutionFor the point (5, 2), the distance from the x-axis is __________ units.
View SolutionThe x-coordinate of any point lying on the y-axis will be __________.
View SolutionThe y-coordinate of the point (2, 4) is __________.
View SolutionIn the point (4, 7), 4 denotes the __________.
View SolutionA point has 5 as its x–coordinate and 4 as its y–coordinate. Then the coordinates of the point are given by __________.
View SolutionIn the coordinates of a point, the second number denotes the __________.
View SolutionThe point where the two axes intersect is called the __________.
View SolutionFor fixing a point on the graph sheet we need two coordinates.
View SolutionState True or False
A line graph can also be a whole unbroken line.
View SolutionThe distance of any point from the x-axis is called the x-coordinate.
View SolutionThe distance of the point (3, 5) from the y-axis is 5.
View SolutionState True or False
The ordinate of a point is its distance from the y-axis.
View SolutionIn the point (2, 3), 3 denotes the y-coordinate.
View SolutionThe coordinates of the origin are (0, 0).
View SolutionThe points (3, 5) and (5, 3) represent the same point.
View SolutionThe y-coordinate of any point lying on the x-axis will be zero.
View SolutionMatch the coordinates given in Column A with the items mentioned in Column B.

View SolutionMatch the ordinates of the points given in Column A with the items mentioned in Column B.

View SolutionFrom the given graph, choose the letters that indicate the location of the points given below.
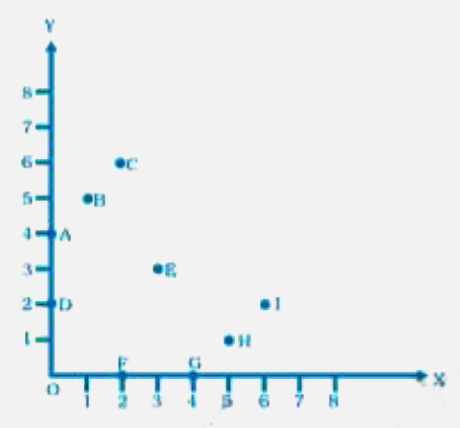
View SolutionFind the coordinates of all letters in the graph given below.
View SolutionPlot the given points on a graph sheet.
(i) (5,4)
(ii) (2,0)
(iii) (3,1)
(iv) (0,4)
(v) (4,5)
View SolutionStudy the given map of a zoo and answer the following questions.
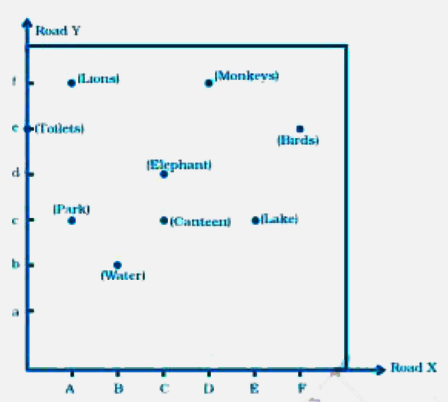
View SolutionWrite the x-coordinate (abscissa) of each of the given points.
(a) (7,3)
(b) (5,7)
(c ) (0,5)
View SolutionWrite the y-coordinate (ordinate) of each of the given points.
(a) (3,5)
(b) (4,0)
(c ) (2,7)
View SolutionPlot the given points on a graph sheet and check if the points lie on a straight line. If not, name the shape they form when joined in the given order.
(a) (1, 2), (2, 4), (3, 6), (4, 8).
(b) (1, 1), (1, 2), (2, 1), (2, 2).
(c ) (4, 2), (2, 4), (3, 3), (5, 4).
View SolutionIf y–coordinate is 3 times x-coordinate, form a table for it and draw a graph.
View SolutionMake a line graph for the area of a square as per the given table.
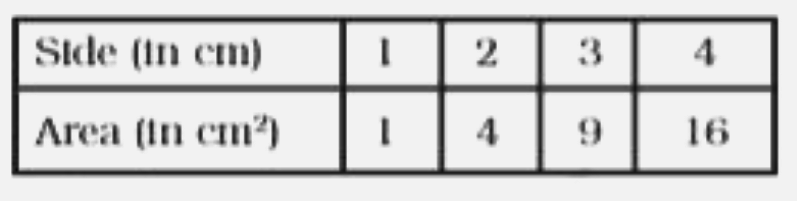
Is it a linear graph?
View SolutionThe cost of a note book is Rs 10. Draw a graph after making a table showing cost of 2, 3, 4, .... note books. Use it to find
(a) the cost of 7 notebooks.
(b) The number of note books that can be purchased with Rs 50.
View SolutionExplain the situations represented by the following distance-time graphs.
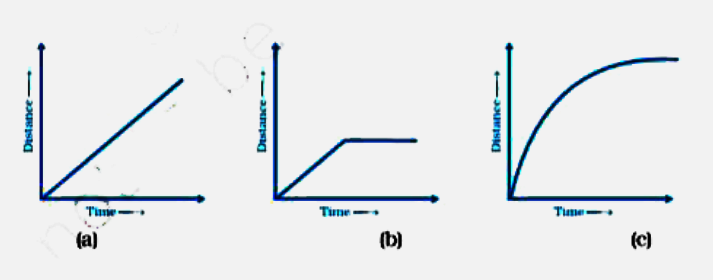
View SolutionComplete the given tables and draw a graph for each.
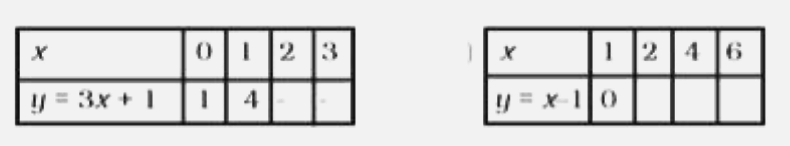
View SolutionStudy the given graphs (a) and (b) and complete the corresponding tables below.
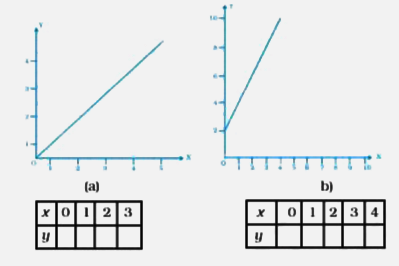
View SolutionDraw a graph for the radius and circumference of circle using a suitable scale.
(Hint : Take radius = 7, 14, 21 units and so on)
From the graph,
a) Find the circumference of the circle when radius is 42 units.
(b) At what radius will the circumference of the circle be 220 units?
View SolutionThe graph shows the maximum temperatures recorded for two consecutive weeks of a town. Study the graph and answer the questions that follow.
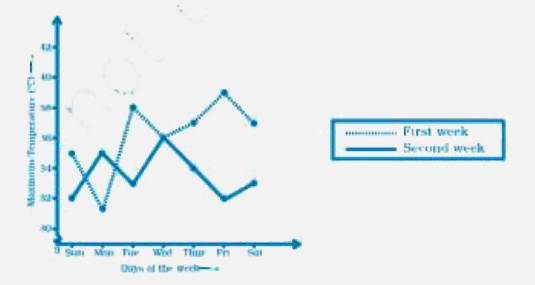
(a) What information is given by the two axes?
(b) In which week was the temperature higher on most of the days?
(c) On which day was the temperature same in both the weeks?
(d) On which day was the difference in temperatures the maximum for both the weeks?
(e) What were the temperatures for both the weeks on Thursday?
(f) On which day was the temperature 35°C for the first week?
(g) On which day was the temperature highest for the second week?
View SolutionThe graph given below gives the actual and expected sales of cars of a company for 6 months. Study the graph and answer the questions that follow.
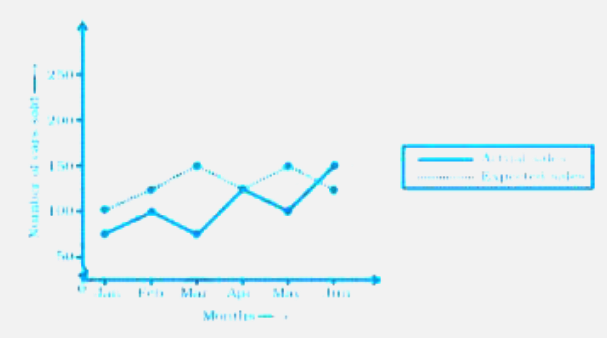
(a) In which month was the actual sales same as the expected sales?
(b) For which month(s) was (were) the difference in actual and expected sales the maximum?
(c) For which month(s) was (were) the difference in actual and expected sales the least?
(d) What was the total sales of cars in the months–Jan, Feb. and March?
(e) What is the average sales of cars in the last three months?
(f) Find the ratio of sales in the first three months to the last three months.
View SolutionThe graph given below shows the marks obtained out of 10 by Sonia in two different tests. Study the graph and answer the questions that follow.
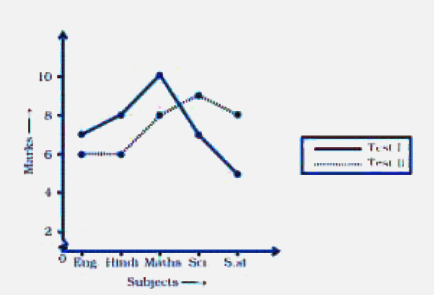
(a) What information is represented by the axes?
(b) In which subject did she score the highest in Test I?
(c) In which subject did she score the least in Test II?
(d) In which subject did she score the same marks in both the Tests?
(e) What are the marks scored by her in English in Test II?
(f) In which test was the performance better?
(g) In which subject and which test did she score full marks?
View SolutionFind the coordinates of the vertices of the given figures.
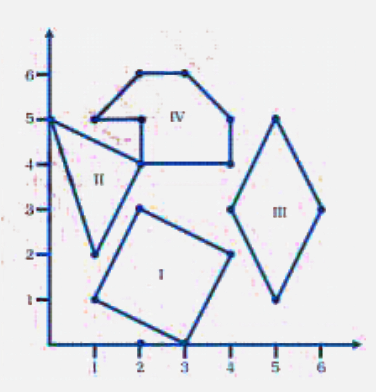
View SolutionStudy the graph given below of a person who started from his home and returned at the end of the day. Answer the questions that follow.
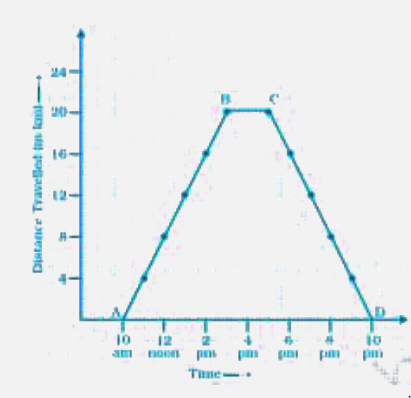
(a) At what time did the person start from his home?
(b) How much distance did he travel in the first four hours of his journey ?
(c) What was he doing from 3 pm to 5 pm?
(d) What was the total distance travelled by him throughout the day ?
(e) Calculate the distance covered by him in the first 8 hours of his journey.
(f) At what time did he cover 16 km of his journey?
(g) Calculate the average speed of the man from (a) A to B (b) B to C (c) (h)At what time did he return home?
View SolutionPlot a line graph for the variables p and q where p is two times q i.e, the equation is p = 2q.
View SolutionStudy the graph and answer the questions that follow.
(a) What information does the graph give?
(b) On which day was the temperature the least?
(c) On which day was the temperature `31^(@)C?`
(d) Which was the hottest day?
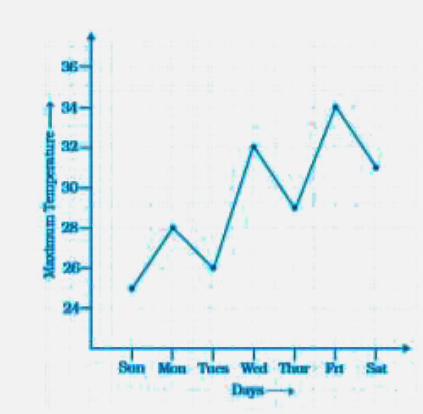
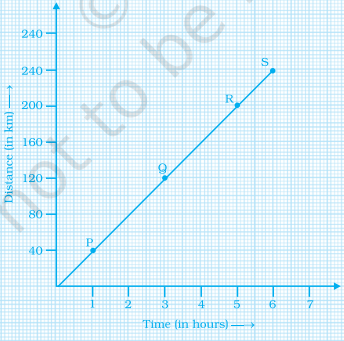
View SolutionStudy the distance-time graph given below for a car to travel to certain places and answer the questions that follow.
(a) How far does the car travel in 2 hours?
(b) How much time does the car take to reach R?
(c) How long does the car take to cover 80 km?
(d) How far is Q from the starting point?
(e) When does the car reach the place S after starting?
View SolutionLocate the points A (1,2), B (4,2) and C (1,4) on a graph sheet taking suitable axes. Write the coordinates of the fourth point D to complete the rectangle ABCD.
View SolutionLocate the points A(1,2), B (3,4) and C (5,2) on a graph sheet taking suitable axes. Write the coordinates of the fourth point D to complete the rhombus ABCD. Measure the diagonals of this rhombus and find whether they are equal or not.
View SolutionLocate the points P (3,4), Q (1,0), R (0,4), S (4,1) on a graph sheet.
View SolutionThe graph given below compares the sales of ice creams of two vendors for a week.
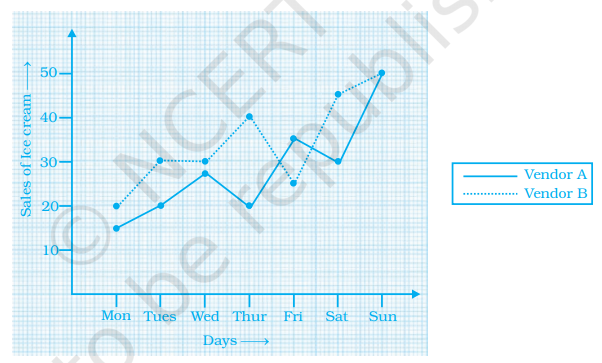
Observe the graph and answer the following questions.
(a) Which vendor has sold more icecreams on Friday?
(b) For which day was the sales same for both the vendors?
(c) On which day did the sale of vendor A increase the most as compared to the previous day?
(d) On which day was the difference in sales the maximum?
(e) On which two days was the sales same for vendor B?
View SolutionThe table given below shows the temperatures recorded on a day at different times.
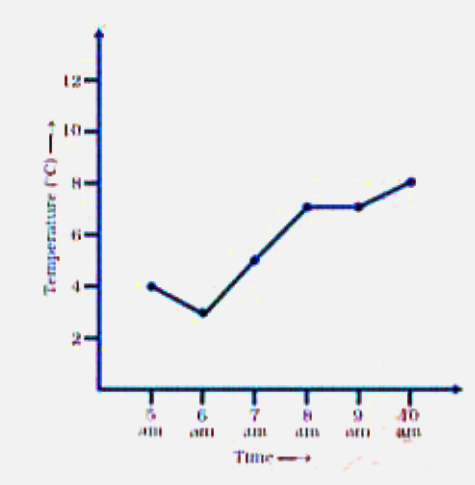
Observe the table and answer the following questions.
(a) What is the temperature at 8 am?
(b) At what time is the temperature `3^(@)C?`
(c) During which hour did the temperature fall?
(d) What is the change in temperature between 7 am and 10 am?
(e) During which hour was there a constant temperature?
View SolutionThe following table gives the growth chart of a child.
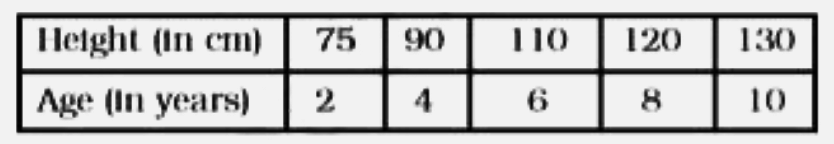
Draw a line graph for the table and answer the questions that follow.
(a) What is the height at the age of 5 years?
(b) How much taller was the child at the age of 10 than at the age of 6 ?
(c) Between which two consecutive periods did the child grow more faster ?
View SolutionThe following is the time-distance graph of Sneha’s walking.
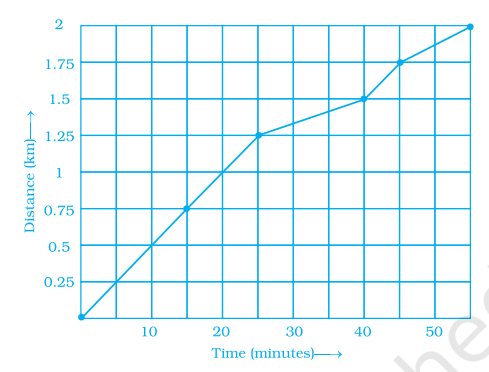
(a) When does Sneha make the least progress? Explain your reasoning.
(b) Find her average speed in km/hour.
View SolutionDraw a parallelogram ABCD on a graph paper with the coordinates given in Table I. Use this table to complete Tables II and III to get the coordinates of E, F, G, H and J, K, L, M.
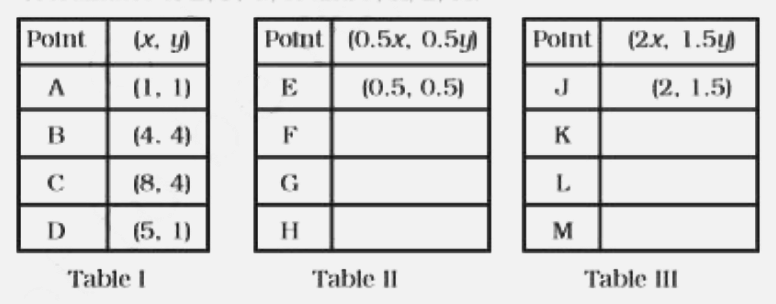
Draw parallelograms EFGH and JKLM on the same graph paper. Plot the points (2, 4) and (4, 2) on a graph paper, then draw a line segment joining these two points.
View SolutionDraw the line passing through (2, 3) and (3, 2). Find the coordinates of the points at which this line meets the x-axis and y-axis.
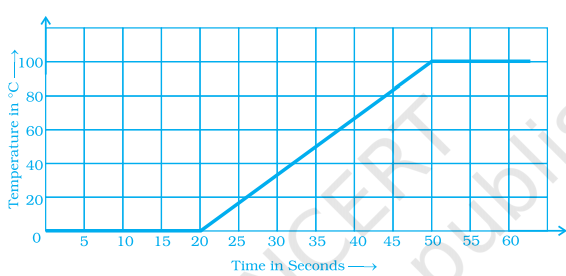
View SolutionThe following graph shows the change in temperature of a block of ice when heated. Use the graph to answer the following questions:
(a) For how many seconds did the ice block have no change in temperature?
(b) For how long was there a change in temperature?
(c) After how many seconds of heating did the temperature become constant at `0^(@)C`?
(d) What was the temperature after 25 seconds?
(e) What will be the temperature after 1.5 minutes? Justify your answer.
View SolutionThe following graph shows the number of people present at a certain shop at different times. Observe the graph and answer the following questions.
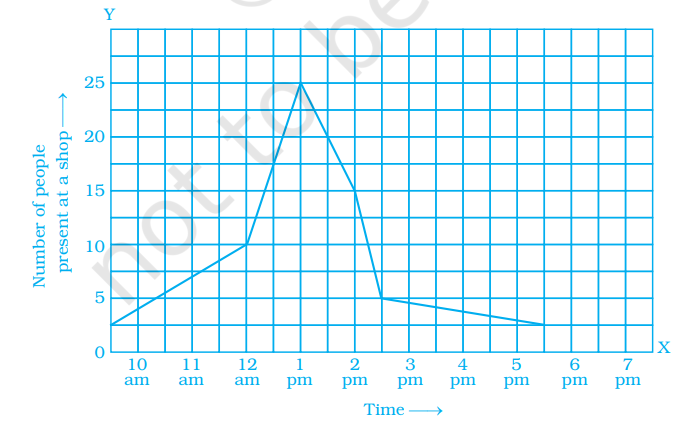
(a) What type of a graph is this?
(b) What information does the graph give?
(c) What is the busiest time of day at the shop?
(d) How many people enter the shop when it opens?
(e) About how many people are there in the shop at 1:30 pm?
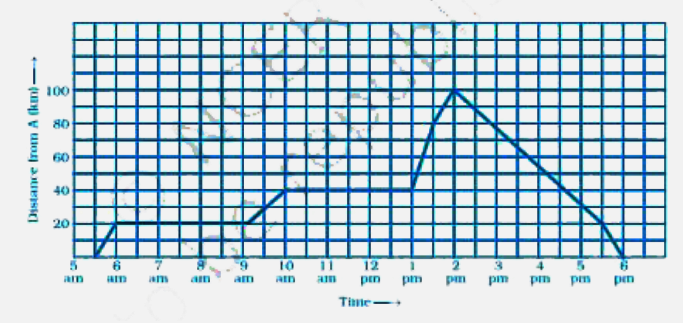
View SolutionA man started his journey on his car from location A and came back. The given graph shows his position at different times during the whole journey.
(a) At what time did he start and end his journey?
(b) What was the total duration of journey?
(c) Which journey, forward or return, was of longer duration?
(d) For how many hours did he not move?
(e) At what time did he have the fastest speed?
View SolutionThe following graph shows the journey made by two cyclists, one from town A to B and the other from town B to A.
(a) At what time did cyclist II rest? How long did the cyclist rest?
(b) Was cyclist II cycling faster or slower after the rest?
(c) At what time did the two cyclists meet?
(d) How far had cyclist II travelled when he met cyclist I?
(e) When cyclist II reached town A, how far was cyclist I from town B?
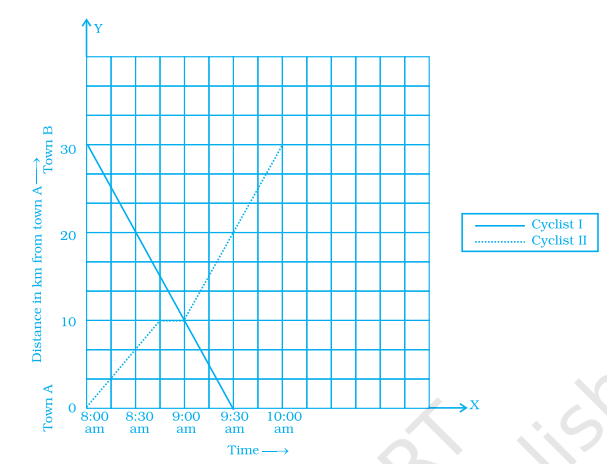
View SolutionAjita starts off from home at 07.00 hours with her father on a scooter that goes at a uniform speed of 30 km/h and drops her at her school after half an hour. She stays in the school till 13.30 hours and takes an auto rickshaw to return home. The rickshaw has a uniform speed of 10 km/h. Draw the graph for the above situation and also determine the distance of Ajita’s school from her house.
View SolutionDraw the line graph using suitable scale to show the annual gross profit of a company for a period of five years.
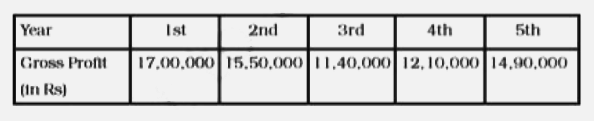
View SolutionThe following chart gives the growth in height in terms of percentage of full height of boys and girls with their respective ages.
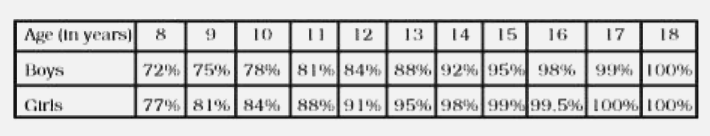
Draw the line graph of above data on the same sheet and answer the following questions.
(a) In which year both the boys and the girls achieve their maximum height?
(b) Who grows faster at puberty (14 years to 16 years of age)?
View SolutionThe table shows the data collected for Dhruv’s walking on a road.
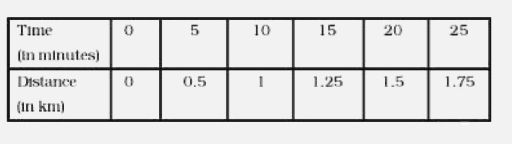
(a) Plot a line graph for the given data using a suitable scale.
(b) In what time periods did Dhruv make the most progress?
View SolutionObserve the given graph carefully and complete the table given below.
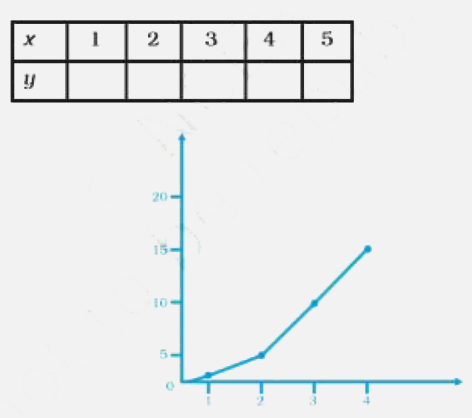
View SolutionThis graph shows the per cent of students who dropped out of school after completing High School. The point labelled A shows that, in 1996, about 4.7% of students dropped out.
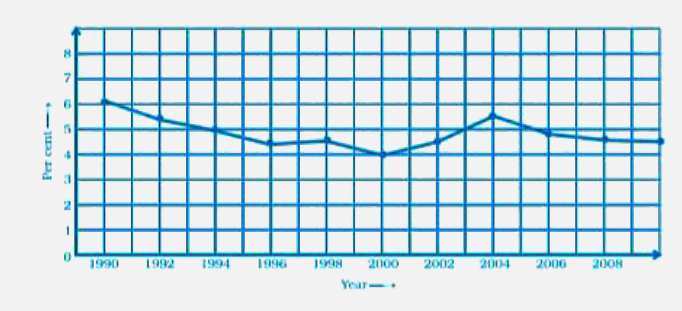
(a) In which year was the dropout the rate highest? In which year was it the lowest?
(b) When did the per cent of students who dropped out of high school first fall below 5%?
(c) About what per cent of students dropped out of high school in 2007? About what per cent of students stayed in high school in 2008?
View SolutionObserve the toothpick pattern given below:
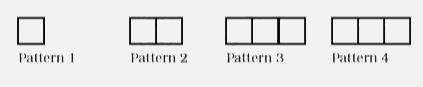
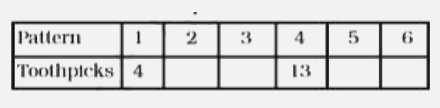
(a) Imagine that this pattern continues. Complete the table to show the number of toothpicks in the first six terms.
(b) Make a graph by taking the pattern numbers on the horizontal axis and the number of toothpicks on the vertical axis. Make the horizontal axis from 0 to 10 and the vertical axis from 0 to 30.
(c) Use your graph to predict the number of toothpicks in patterns 7 and 8. Check your answers by actually drawing them.
(d) Would it make sense to join the points on this graph? Explain.
View SolutionConsider this input/output table.

(a) Graph the values from the table by taking Input along horizontal axis from 0 to 8 and Output along vertical axis from 0 to 24.
(b) Use your graph to predict the outputs for inputs of 3 and 8.
View SolutionThis graph shows a map of an island just off the coast of a continent. The point labelled B represents a major city on the coast. The distance between grid lines represents 1 km.
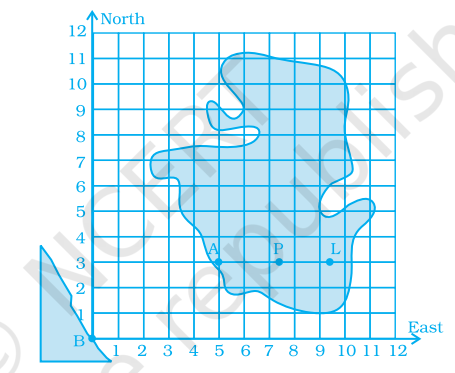
Point A represents a resort that is located 5 km East and 3 km North of Point B. The values 5 and 3 are the coordinates of Point A. The coordinates can be given as the ordered pair (5, 3), where 5 is the horizontal coordinate and 3 is the vertical coordinate.
(i) On a copy of the map, mark the point that is 3 km East and 5 km North of Point B and label it S. Is Point S in the water or on the island? Is Point S in the same place as Point A?
(ii) Mark the point that is 7 km east and 5 km north of Point B and label it C. Then mark the point that is 5 km east and 7 km north of Point B and label it D. Are Points C and D in the same place? Give the coordinates of Points C and D.
(iii) Which point is in the water, (2, 7) or (7, 2)? Mark the point which is in water on your map and label it E.
(iv) Give the coordinates of two points on the island that are exactly 2 km from Point A.
(v) Give the coordinates of the point that is halfway between Points L and P.
(vi) List three points on the island with their x-coordinates greater than 8.
(vii) List three points on the island with a y-coordinate less than 4.
View SolutionAs part of his science project, Prithvi was supposed to record the temperature every hour one Saturday from 6 am to midnight. At noon, he was taking lunch and forgot to record the temperature. At 8:00 pm, his favourite show came on and so forgot again. He recorded the data so collected on a graph sheet as shown below.
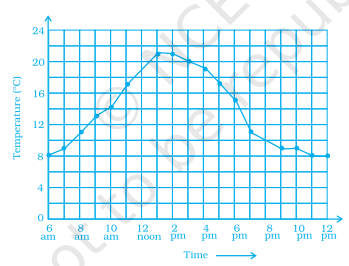
(a) Why does it make sense to connect the points in this situation?
(b) Describe the overall trend, or pattern, in the way the temperature changes over the time period shown on the graph.
(c) Estimate the temperature at noon and 8 pm.
View SolutionThe graph given below compares the price (in Rs) and weight of 6 bags (in kg) of sugar of different brands A, B, C, D, E, F.
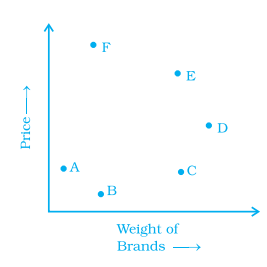
(a) Which brand(s) costs/cost more than Brand D?
(b) Bag of which brand of sugar is the heaviest?
(c) Which brands weigh the same?
(d) Which brands are heavier than brand B?
(e) Which bag is the lightest?
(f) Which bags are of the same price?
View SolutionThe points on the graph below represent the height and weight of the donkey, dog, crocodile, and ostrich shown in the drawing.
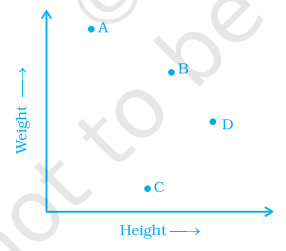
(a) What are the two variables represented in the graph?
(b) Which point represents each animals? Explain.
View SolutionThe two graphs below compare Car A and Car B. The left graph shows the relationship between age and value. The right graph shows the relationship between size and maximum speed.
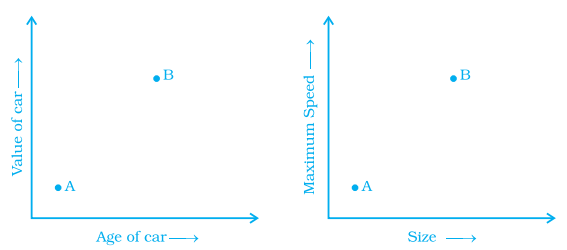
Use the graphs to determine whether each statement is true or false, and explain your answer.
(a) The older car is less valuable.
(b) The faster car is larger.
(c) The larger car is older.
(d) The faster car is older.
(e) The more valuable car is slower.
View SolutionSonal and Anmol made a sequence of tile designs from square white tiles surrounding one square purple tile. The purple tiles come in many sizes. Three of the designs are shown below.
(a) Copy and complete the table
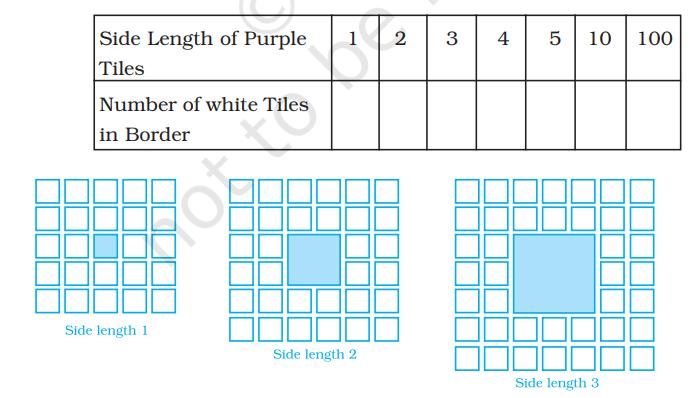
(b) Draw a graph using the first five pairs of numbers in your table.
(c) Do the points lie on a line?
View SolutionSonal and Anmol then made another sequence of the designs. Three of the designs are shown below.
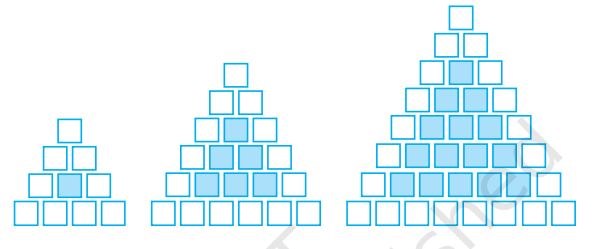
(a) Complete the table.
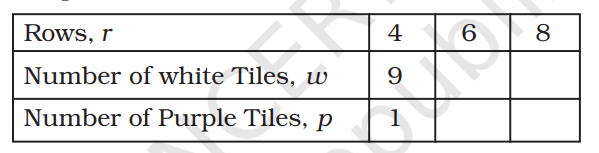
(b) Draw a graph of rows and number of white tiles. Draw another graph of the number of rows and the number of purple tiles. Put the number of rows on the horizontal axis.
(c) Which graph is linear?
View Solution
EXERCISE questions and complete solutions for chapter INTRODUCTION TO GRAPHS of NCERT EXEMPLAR of Class 8