Complete Solutions to Examples of chapter AREA of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | AREA |
| CENGAGE NEW | Examples |
| Number Of Questions | 46 |
| Academic Year | 2023-24 |
Find the area of the closed figure bounded by the curves `y=sqrt(x),y=sqrt(4-3x)a n dy=0`
05:30View SolutionFind the area, lying above the x=axis and included between the circle `x^2+y^2=8x` and the parabola `y^2=4xdot`
07:58View SolutionFind the area bounded by
(i) y`=log""_(e)|x|andy=0`
(ii) `y=|log""_(e)|x||andy=0`03:27View SolutionFind the area bounded by `y=1/(x^2-2x+2)` and x-axis.
03:15View SolutionThe area of the region for which `0>0` is
05:08View SolutionIf the area of bounded between the x-axis and the graph of `y=6x-3x^2` between the ordinates `x=1a n dx=a` is `19` units, then `a` can take the value `4or-2` two value are in (2,3) and one in `(-1,0)` two value are in (3,4) and one in `(-2,-1)` none of these
08:15View SolutionProve that area common to ellipse `(x^2)/(a^2)+(y^2)/(b^2)=1` and its auxiliary circle `x^2+y^2=a^2` is equal to the area of another ellipse of semi-axis `aa n da-bdot`
03:00View SolutionLet `f(x)=` maximum `{x^2, (1-x)^2, 2x(1 - x)}` where `x in [0, 1].` Determine the area of the region bounded by the curve `y=f(x)` and the lines `y = 0,x=0, x=1.`
06:20View SolutionConsider the region formed by the lines `x=0,y=0,x=2,y=2.` If the area enclosed by the curves `y=e^x a n dy=1nx ,` within this region, is being removed, then find the area of the remaining region.
06:53View SolutionDraw a rough sketch of the curve `y=(x^2+3x+2)/(x^2-3x+2)` and find the area of the bounded region between the curve and the x-axis.
07:04View SolutionFind the area bounded by the curve `y=(x-1)(x-2)(x-3)` lying between the ordinates `x=0a n dx=3.`
06:16View SolutionFind the area bounded by the curve `x={{:(-2-y",",ylt-1),(y^(3)",",-1leyle1),(2-y",",ygt1):}` and x=0 is
04:18View SolutionFind the area enclosed by the graph of `y=log_(e)(x+1),`y-axis, and the line y=1
03:09View SolutionFind the area bounded by the curve `y=sin^(-1)x` and the line `x=0,|y|=pi/2dot`
03:54View SolutionFind the area of the region bounded by the curves `y=sqrt(x+2) and y=(1)/(x+1)` between the lines x=0 and x=2.
04:04View SolutionThe area bounded by `y = sin^(-1)x, y= cos^(-1)x` and the x-axis, is given by
03:25View SolutionFind the area bounded by the parabola `y=x^2+1` and the straight line `x+y=3.`
04:52View SolutionFind the area bounded by the curves `y=s in xa n dy=cosx` between two consecutive points of the intersection.
04:53View SolutionFind the ratio in which the area bounded by the curves `y^2=12 xa n dx^2=12 y` is divided by the line `x=3.`
14:10View SolutionFind the area of the figure bounded by the parabolas `x=-2y^2, x=1-3y^2dot`
05:05View SolutionThe area common to regions `x^2+y^2-2xlt=0` and `ygeqsin(pix)/2dot`
06:37View SolutionFind the area of the region enclosed by the curves `y=xlogxa n dy=2x-2x^2dot`
07:50View SolutionFind the area bounded by `y^2lt=4x ,x^2+y^2geq2x ,a n dxlt=y+2` in the first quadrant.
16:27View SolutionSketch the region bounded by the curves `y=x^2a n dy=2/(1+x^2)` . Find the area.
06:10View SolutionFind the area bounded by the curves `y=x^(3)-x and y=x^(2)+x.`
05:53View SolutionFind the area bounded by `y=-x^(3)+x^(2)+16x and y=4x`
06:15View SolutionFind the area of the region enclosed by `y=-5x-x^(2) and y=x` on interval `[-1,5]`
04:45View SolutionIf the area enclosed by curve `y=f(x)a n dy=x^2+2` between the abscissa `x=2a n dx=alpha,alpha>2,` is `(alpha^3-4alpha^2+8)s qdot` unit. It is known that curve `y=f(x)` lies below the parabola `y=x^2+2.`
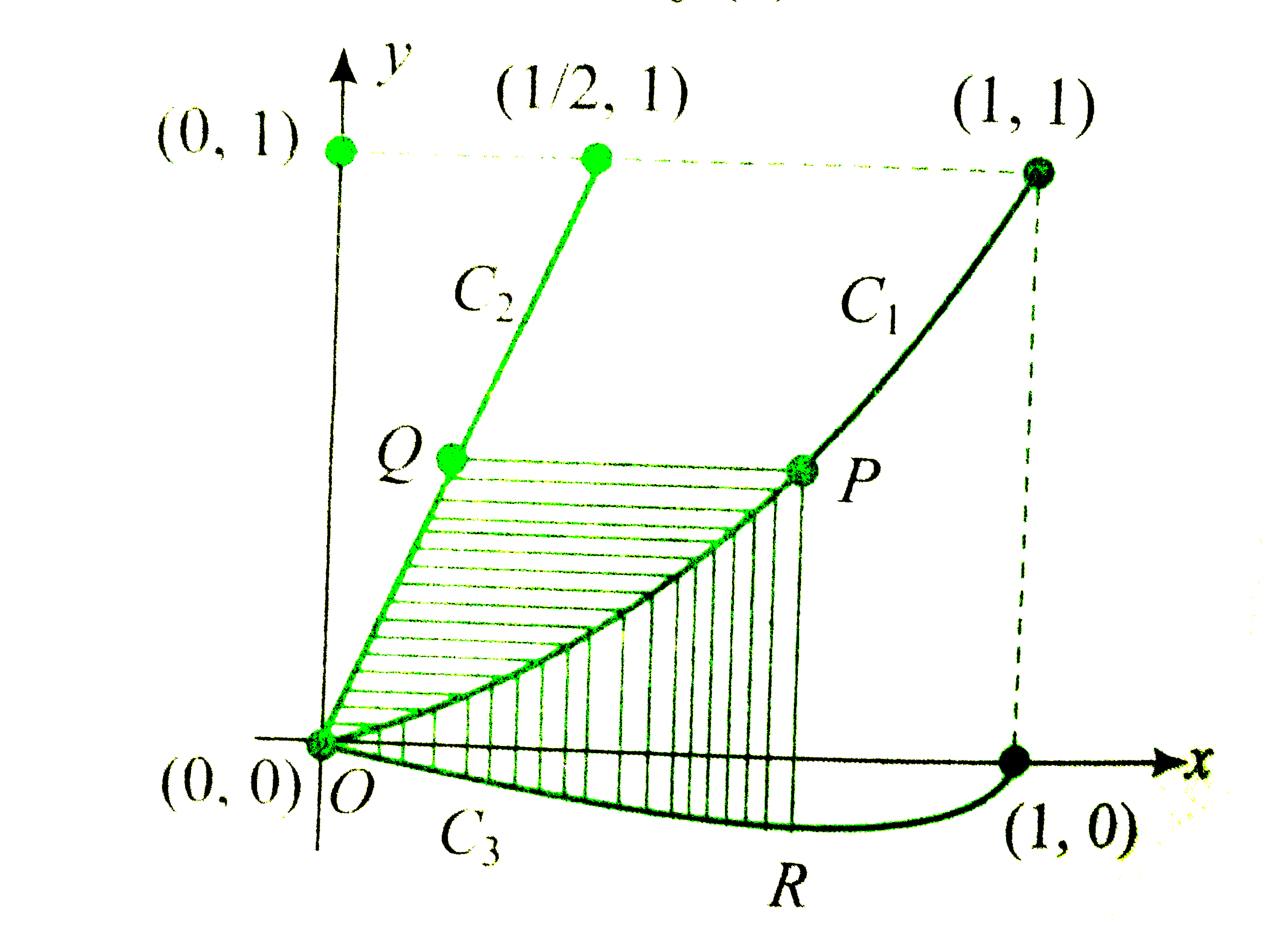
03:58View SolutionLet `C_(1) and C_(2)` be the graphs of the functions `y=x^(2) and y=2x,` respectively, where `0le x le 1." Let "C_(3)` be the graph of a function y=f(x), where `0lexle1, f(0)=0.` For a point P on `C_(1),` let the lines through P, parallel to the axes, meet `C_(2) and C_(3)` at Q and R, respectively (see figure). If for every position of `P(on C_(1)),` the areas of the shaded regions OPQ and ORP are equal, determine the function f(x).
 View Solution
View SolutionIf the area bounded by `f(x)=sqrt(tan x), y=f(c), x=0 and x=a, 0ltcltalt(pi)/(2)` is minimum then find the value of c.
06:57View SolutionFind the area of the region `R` which is enclosed by the curve `ygeqsqrt(1-x^2)` and max `{|x|,|y|}lt=4.`
05:08View SolutionPlot the region in the first quadrant in which points are nearer to the origin than to the line `x=3.`
08:33View SolutionConsider a square with vertices at `(1,1),(-1,1),(-1,-1),a n d(1,-1)dot` Set `S` be the region consisting of all points inside the square which are nearer to the origin than to any edge. Sketch the region `S` and find its area.
12:21View SolutionLet `O(0,0),A(2,0),a n dB(1 1/(sqrt(3)))` be the vertices of a triangle. Let `R` be the region consisting of all those points `P` inside ` O A B` which satisfy `d(P , O A)lt=min[d(p ,O B),d(P ,A B)]` , where `d` denotes the distance from the point to the corresponding line. Sketch the region `R` and find its area.
06:03View SolutionFind the area enclosed by `y=g(x),` x-axis, x=1 and x=37, where g(x) is inverse of `f(x)=x^(3)+3x+1`.
04:39View SolutionFind the area bounded by the curve `f(x)=x+ sin x and ` its inverse function between the ordinates `x=0" to "x=2pi`.
02:22View SolutionFind the area bounded by the curve `x^2=y ,x^2=-ya n dy^2=4x-3`
06:10View SolutionFind the area of the region enclosed by the curve `y=|x-(1)/(x)|(xgt0)` and the line y=2
06:53View SolutionFind the area of the region bounded by the curves `y=x^2,y=|2-x^2|,a n dy l=2,` which lies to the right of the line `x=1.`
06:15View SolutionThe ratio in which the line `x-1=0` divides the area bounded by the curves `2x+1=sqrt(4y+1),y=x and y=2 ` is
04:32View SolutionIf `S_(0),S_(1),S_(2),…` are areas bounded by the x-axis and half-wave of the curve `y=sin pi sqrt(x)," then prove that "S_(0),S_(1),S_(2),…` are in A.P…
View SolutionFind the area of the figure enclosed by the curve `5x^2+6x y+2y^2+7x+6y+6=0.`
06:33View SolutionFind the area bounded by the curves `x^2+y^2=4, x^2=-sqrt2 y` and `x=y`
08:38View SolutionFind the area of the region bounded by the curve `C : y=tanx ,t a nge n td r a w ntoC` at `x=pi/4,` and the x-axis.
06:26View SolutionCompute the area of the region bounded by the curves `y-e x(log)_e xa n dy=(logx)/(e x)`
11:44View SolutionLet `A_n` be the area bounded by the curve `y=(tanx)^n` and the lines `x=0,y=0,` and `x=pi/4dot` Prove that for `n >2,A_n+A_(n-2)=1/(n-1)` and deduce `1/(2n+2)
07:01View Solution
Examples questions and complete solutions for chapter AREA of CENGAGE MATHS (HINGLISH) of Class 12
