Linked Comprehension from CENGAGE PHYSICS (HINGLISH) WAVE OPTICS for Class 12
| Class 12 | PHYSICS |
| Chapter | WAVE OPTICS |
| CENGAGE PHYSICS (HINGLISH) | Linked Comprehension |
| Medium | English |
| Academic Year | 2023-2024 |
Question 6
By an anodizing process, a transparent film of aluminium oxide of thickness t=250 nm and index of refraction n2=1.80 is deposited on a sheet of polished aluminium What is the color of utenslis made from this sheet with observer in white light? Assume normal incidence of the light.View SolutionQuestion 10
An oil film (n=1.45) floating on water is illuminated by white light at normal incidence. The film is 280 nm thick. Find (a) the color or the light in the visible specturm most strongly reflected and (b) the color of the light in the specturm most strongly transmitted. Explain your reasoning.View SolutionQuestion 12
In solar cells, a silicon solar cell (μ=3.5) is coated with a thin film
of silicon monoxide SiO(μ=1.45) to minimize reflective losses from the surface.
Determine the minimum thickness of SiO that produces the least reflection at a
wavelength of 550nm, near the centre of the visible spectrum. Assume
approximately normal incidence . View SolutionQuestion 19
Interference fringes are produced by a double slit arrangement
and a piece of plane parallel glass of refractive index 1.5 is interposed in one of
the interfering beam. If the fringes are displaced through 30 fringe widths for
light of wavelength 6×10−5cm, find the thickness of the plate. View SolutionQuestion 21
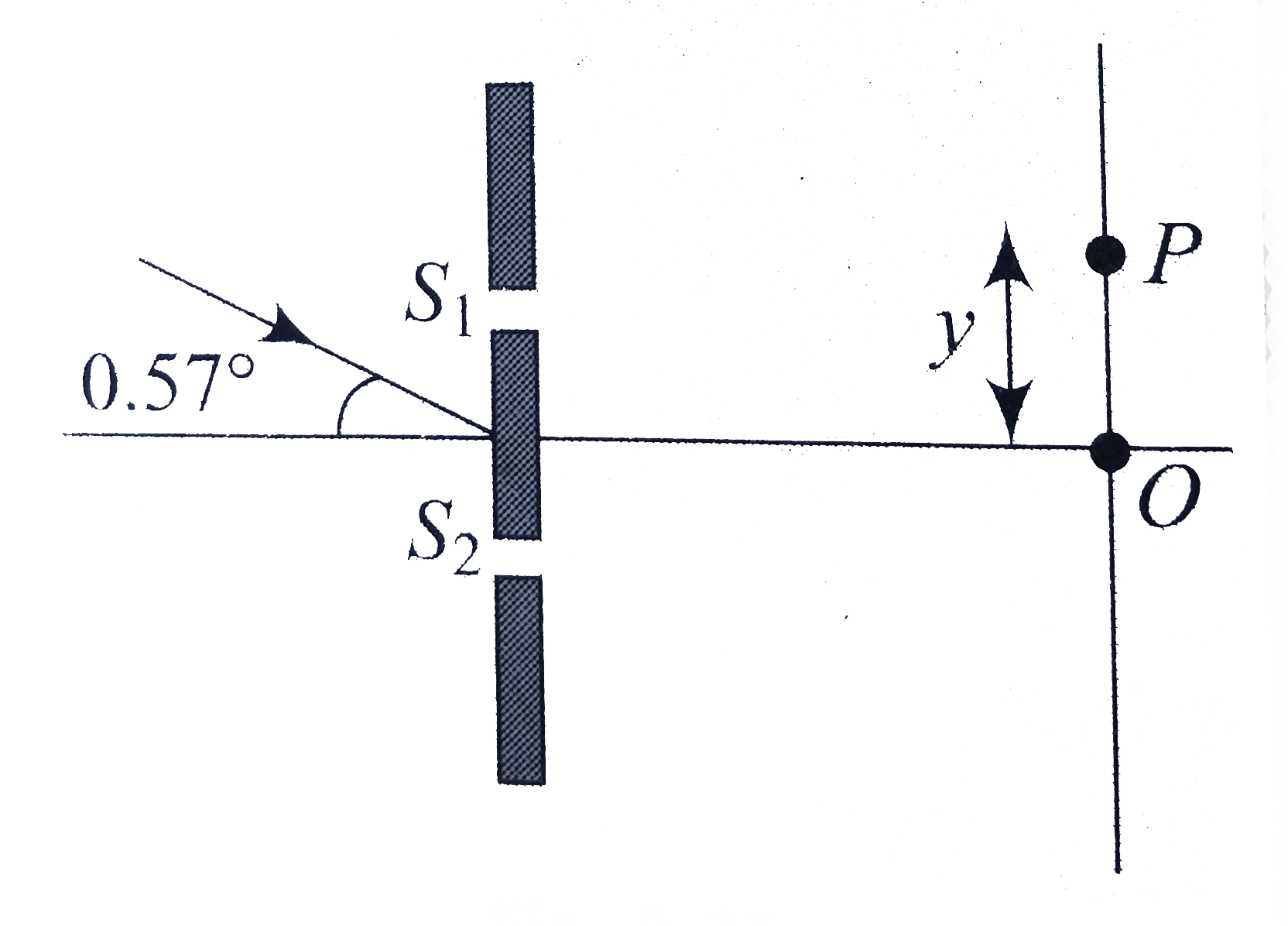
In YDSE with D=1m,d=1mm, light of wavelength 500nm is incident at an angle of 0.57∘ w.r.t. the axis of symmetry of the experimental set-up. If center of symmetry of screen is O as shown figure.
a. find the position of centrla maxima,
b. find intensity at point O in terms of intensity of central maxima I0, and
c. find number of maixma lying central maxima.
 .View Solution
.View SolutionQuestion 23
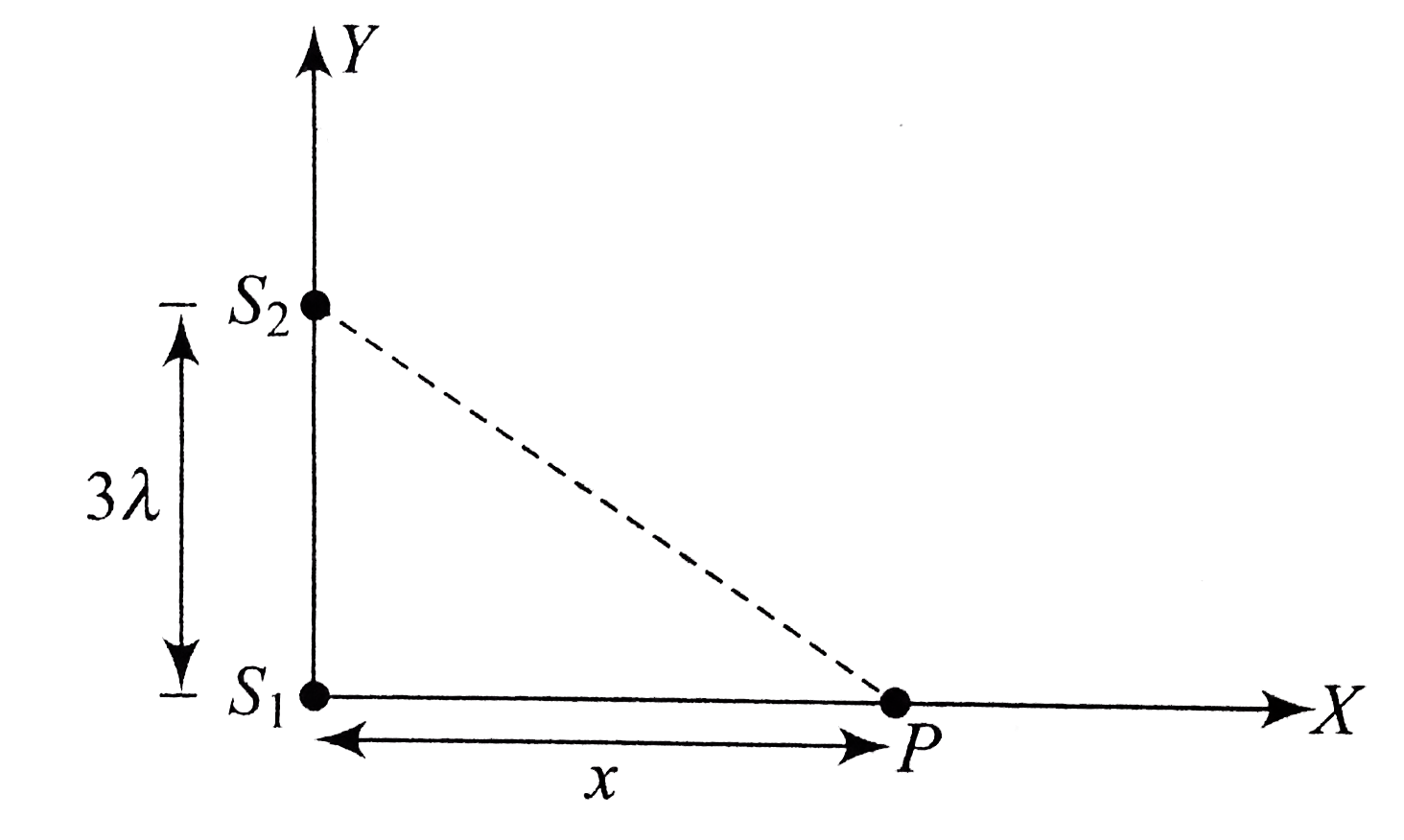
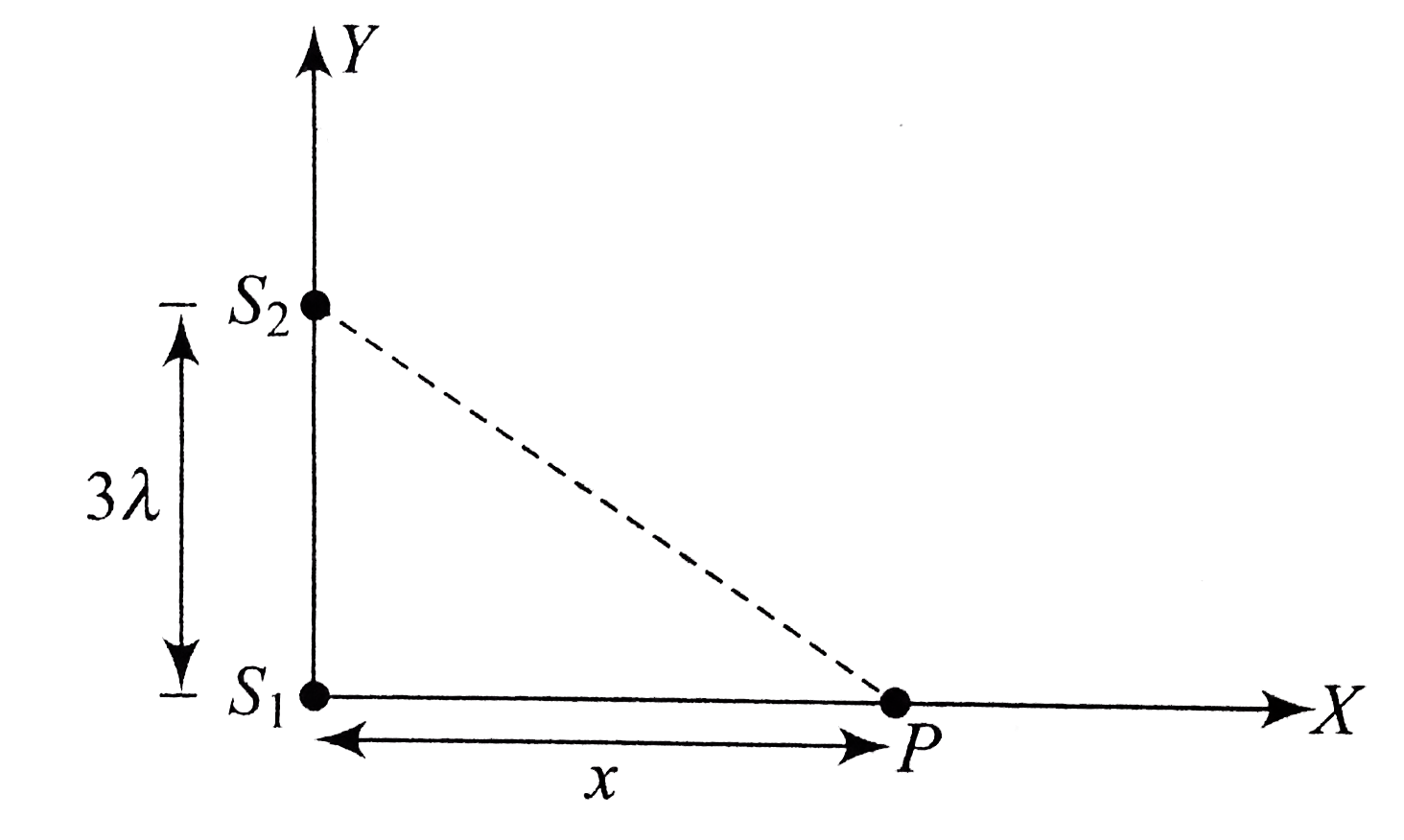
In an interference arrangement similar to double-slit experiment, S1 and S2 are illuminated with coherent source microwave source each of frequency 1 MHz. the sources are synchronized to have zero phase difference . This slits are separated by distance d=150.0m The intensity I(θ) is measured as a funciton of θ, where θ is defined as shown in figure. If I0 is maximum intensity, calculate I(θ)
For :
a. θ=0 b. θ=30∘
c. θ=90∘
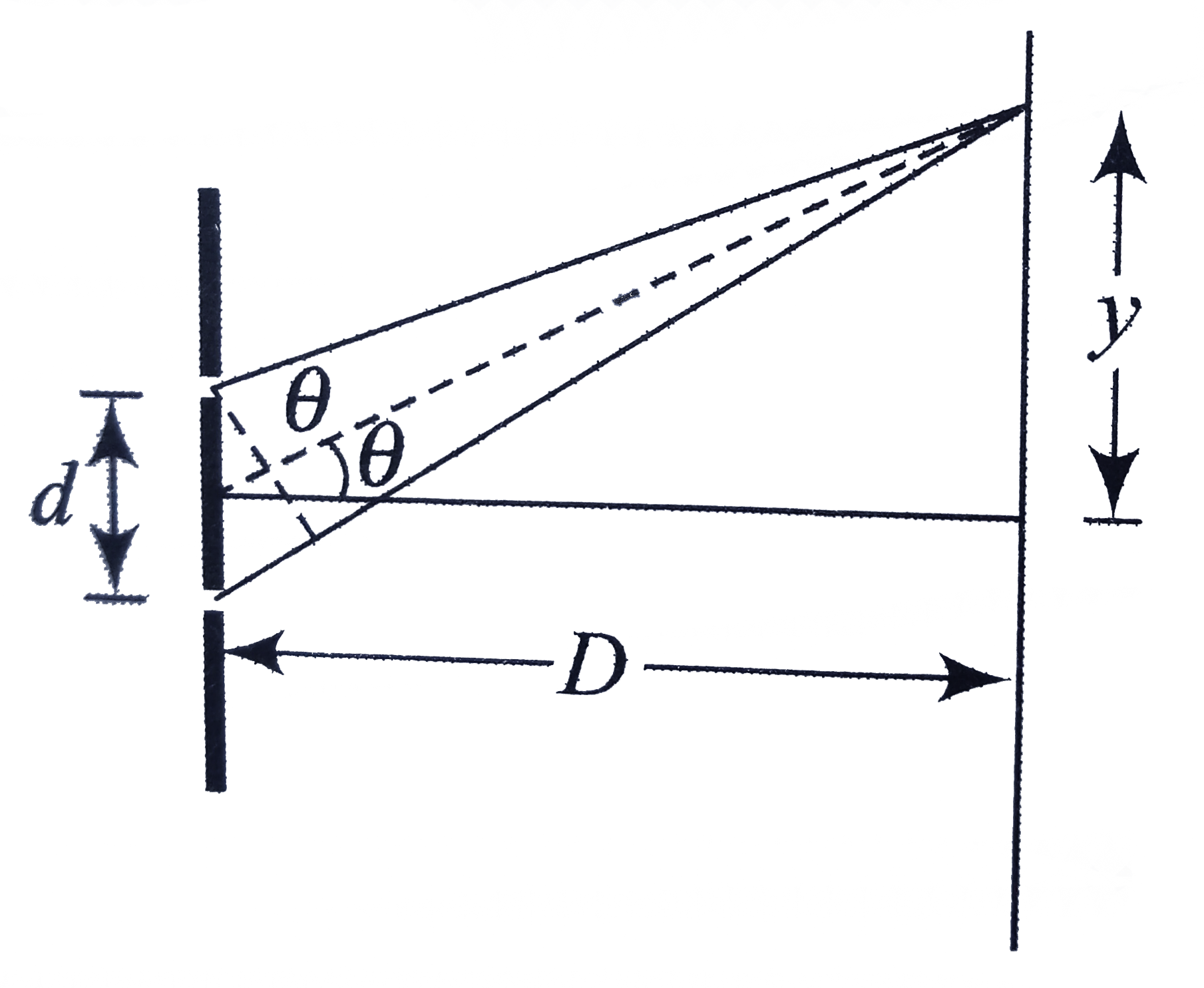 View Solution
View SolutionQuestion 27
Two radio antennas separated by 300 m as shown in figure simultaneously broadcast indentical singals at the same wavelength. A radio in a car traveling due north receives the signals. (a) If the car is at the position of the second maximum, what is the wavelength of the signals? (b) How much farther must the car travel to encounter the next mininum in reception? (Note: Do not use the small- angle approximation in this problem.)
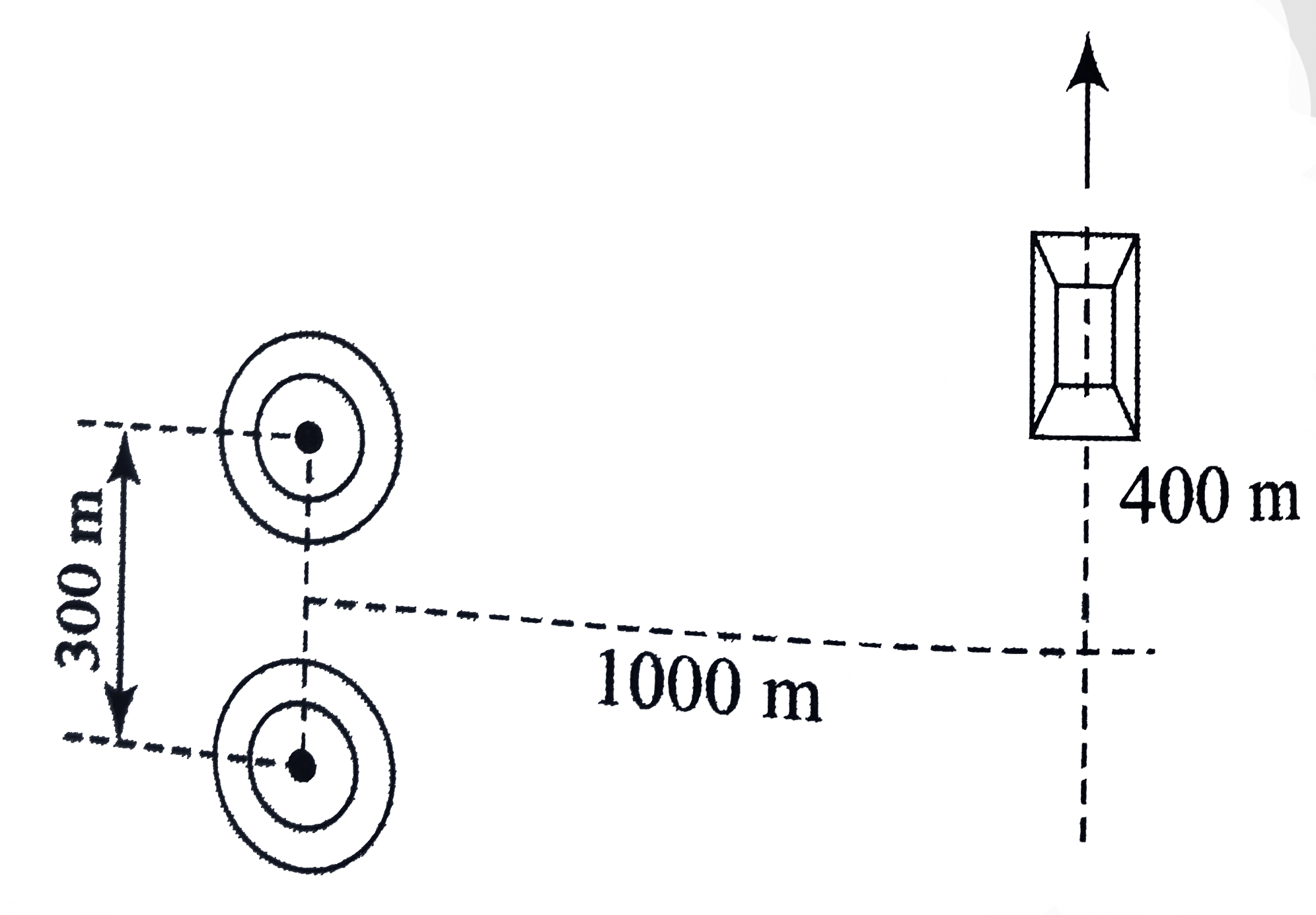 View Solution
View SolutionQuestion 30
The intensity on the screen at a certain point in a double-slit interference patteren is 25.0% of the maximum value. (a) What minimum phase difference (in radians) between the sources produces this result? (b) Express this phase difference as a path difference for 486.1 nm light.View SolutionQuestion 33
The width of one of the two slits in a Young's double slit experiment is double of the other slit. Assuming that the amplitude of the light coming from a slit is proportional to the slit width, find the ratio of the maximum to the minimum intensity in the interference pattern.View SolutionQuestion 34
Suppose that one of the slit in Young's experiment is wider than the order, so the amplitude of the light reaching the central part of the screen from one slit, acting alone, is twice that from the other slit, acting alone. Obtain an expression for the light intensity I at the screen as a function or θ the angular position.View SolutionQuestion 35
A monochromatic beam of light of 6000 Å is used in YDSE set-up. The two slits are covered with two thin films of equal thickness t but of different refractive indices as shown in figure. Considering the intensity of the incident beam on the slits to be I0, find the point on the screen at which intensity is I0 and is just above the central maxima. (Assume that there is no change in intensity of the light after passing through the films.) Consider t=6μm,d=1mm, and D=1m, where d and D have their usual meaning. Give your answer in mm.
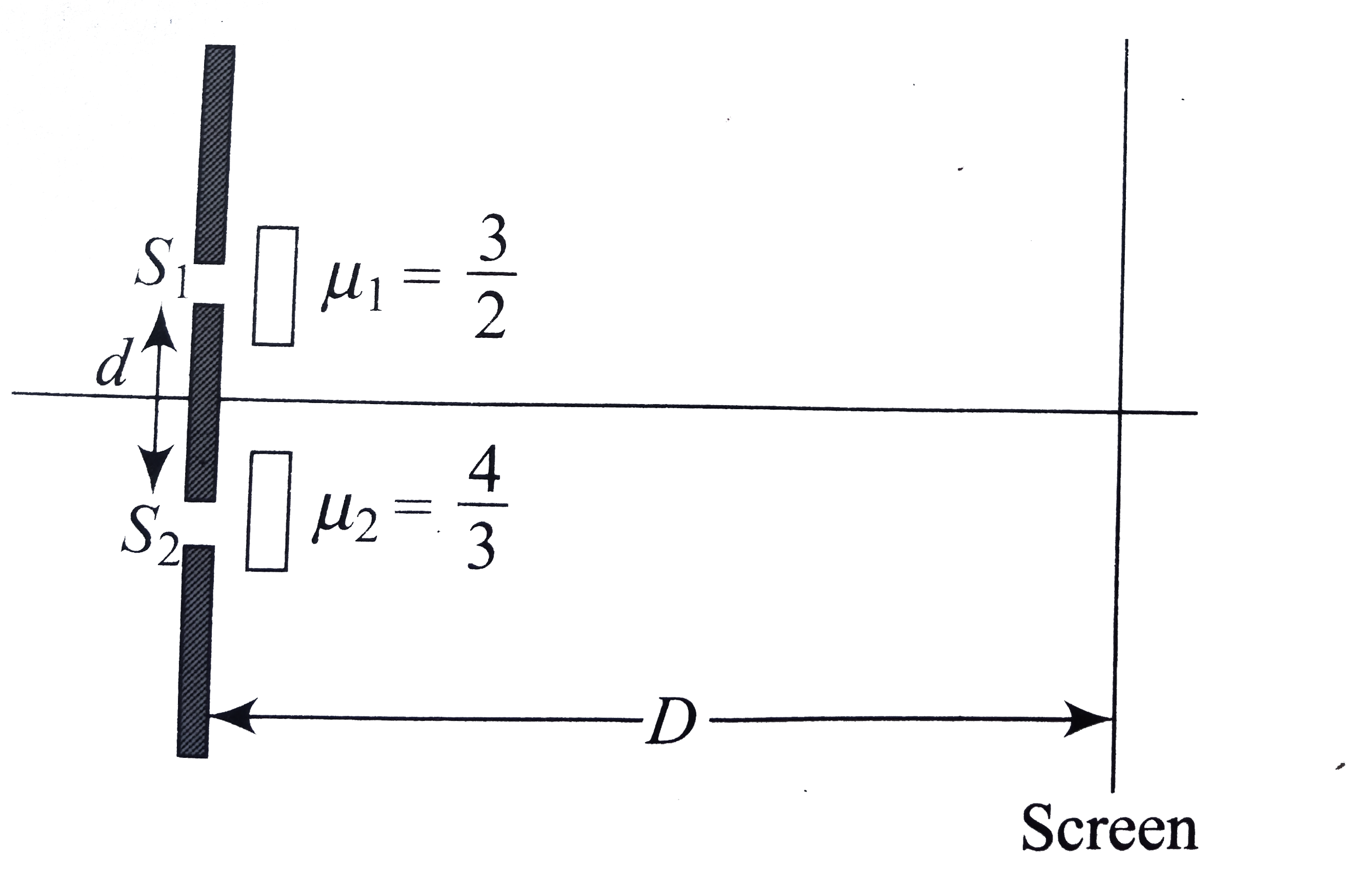 View Solution
View SolutionQuestion 36
A double slit of separation 1.5 mm is illuminated by white light (between 4000 and 8000 Å). On a screen 120 cm away colored interference pattern is formed. If a pinhole is made on this screen at a distance of 3.0 mm from the central white fringe, some wavelengths will be absent in the transimitted light. Find the second longest wavelength (in Å) which will be absent in the transmitted light.View SolutionQuestion 37
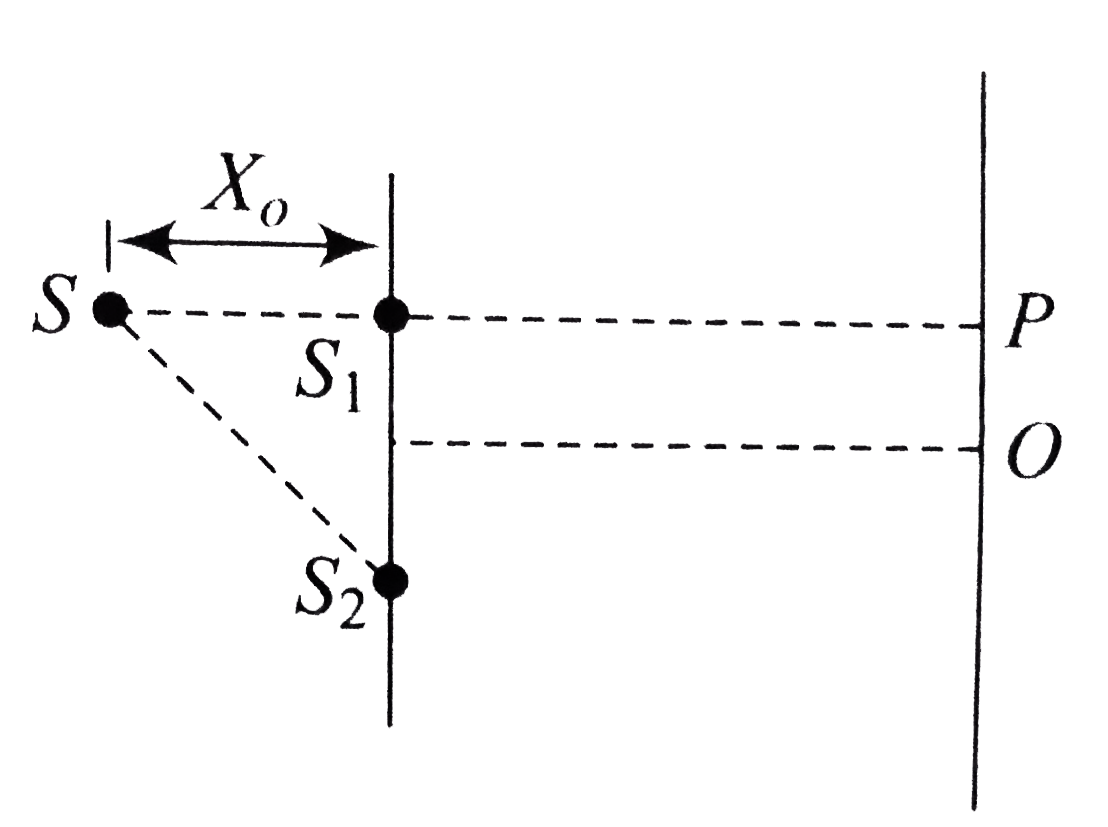
In figure S is a monochromatic source of light emitting light of wavelength of wavelength λ (in air). Light on slits S1 from S and then reaches in the slit S2 and S3 through a medium of refractive index μ1. Light from slit S2 and S3 reaches the screen through a medium of refractive index μ3. A thin transparent film of refractive index μ2 and thickness t is used placed in front of S2. Point P is symmetrical w.r.t. S2 and S3. Using the values d=1mm,D=1m,μ1=4/3,
μ2=3/2,μ3=9/5, and t=49×10−5m,
a. find distance of central maxima from P,
b. If the film in front of S2 is removed, then by what distance and in which direction will be central maxima shift ?
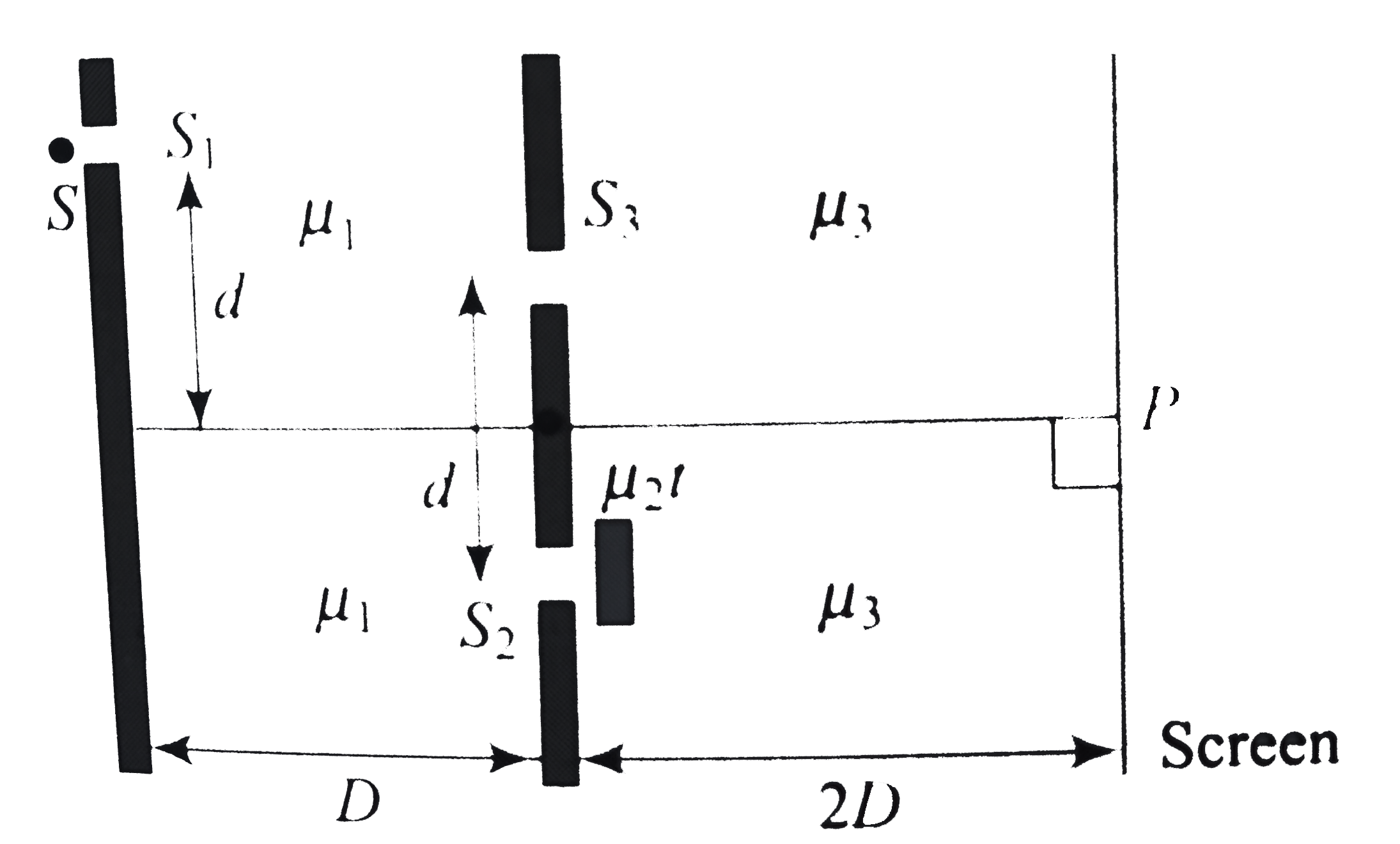 .View Solution
.View SolutionQuestion 38
In the Young's double-slit experiment, the amplitude os source S1 is three times the amplitude of the source S2. These sources are covered by perfectly transparent thin plates of same thickness but different refractive indices 1.6 and 1.5, respectively. Now, if the plates are interchanged and the amplitude of source S1 is made same as that of S2, then find the amout by which the intensity is changed at a point where previous central maxima was formed. Take thickness of the plate equal to (110/3)λ, where λ is the wavelength of light used.
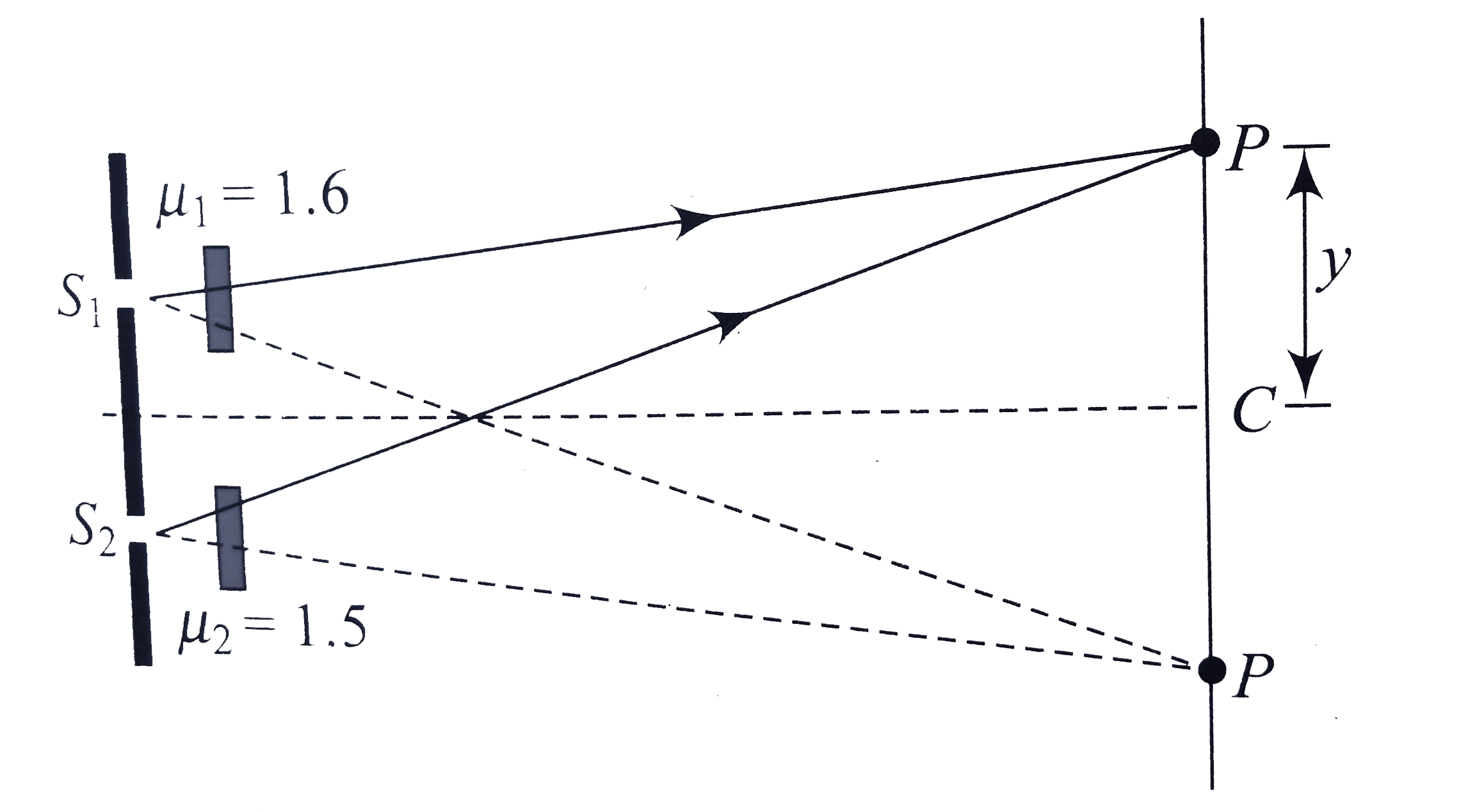 View Solution
View SolutionQuestion 39
Figure shows a narrow slit S illuminated b a monochromatic light of wavelength λ in a double-slit experiment. In the path of the rays reaching the upper slit S1, a tube to length L is interposed in which the index of reflection of the medium varies linearly as shown in figure . The position of the central maximum in the interference pattern on the screen was displaceed by N fringes. Find the value of N in terms of μ0, L and λ.
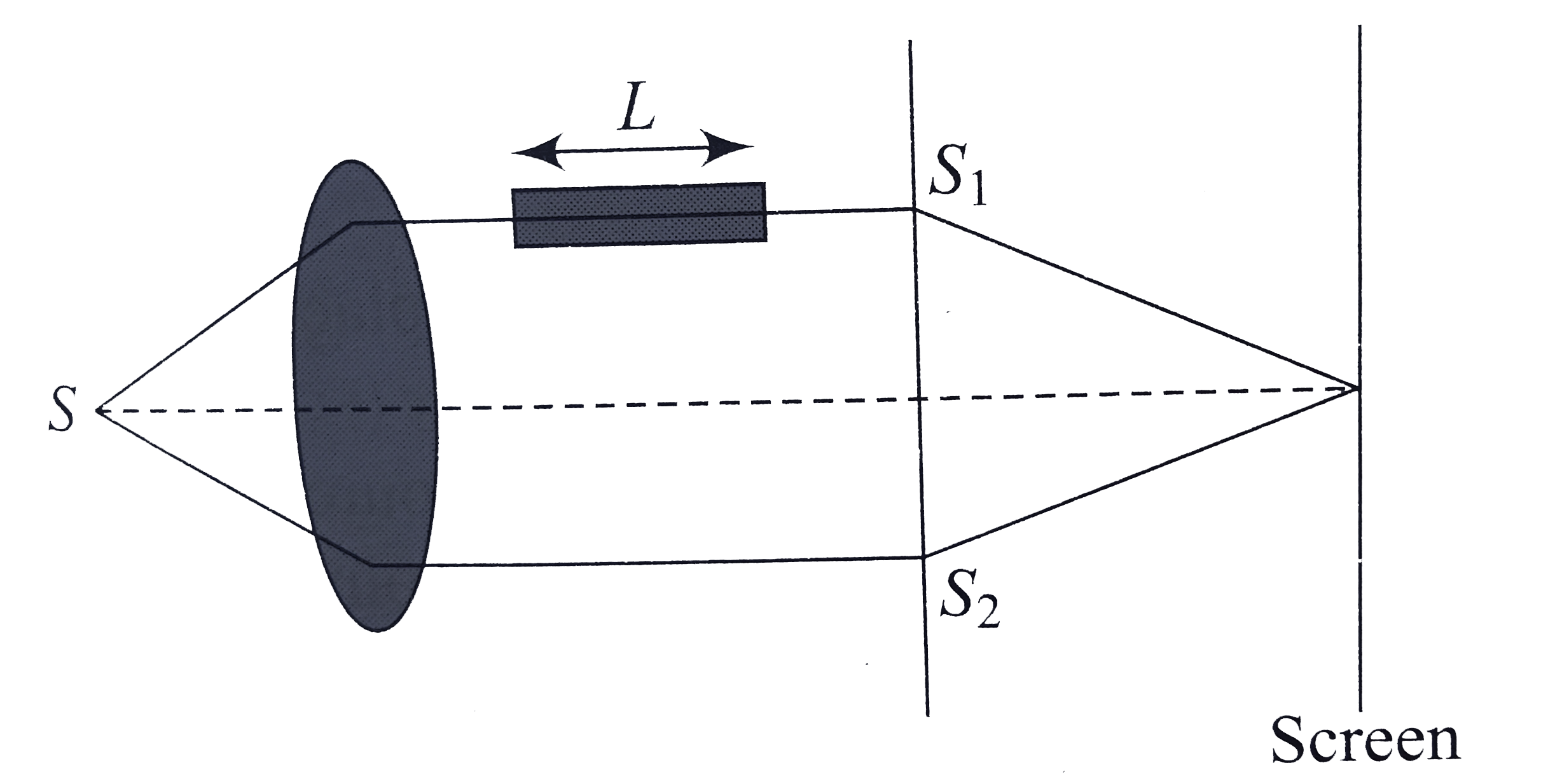
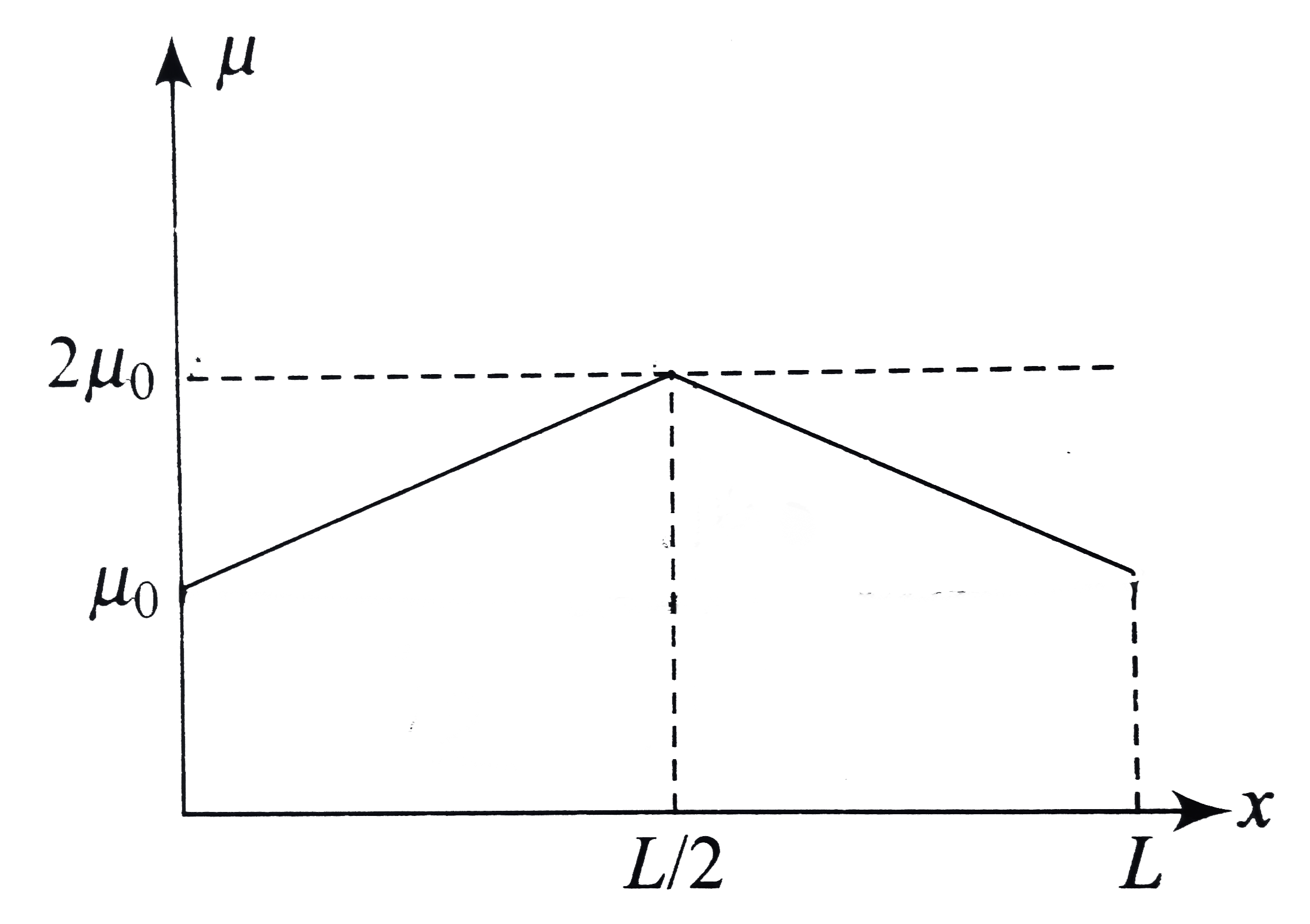 View Solution
View SolutionQuestion 40
In Young's double-slit experiment, a point source is placed on a solid slab of refractive index 6/5 at a distance of 2 mm from two slits spaced 3 mm apart as shown and at equal distance from both the slits. The screen is at a distance of 1 m from the slits. Wavelength of light used is 500 nm.
a. Find the position of the central maximum.
b. Find the order of the fringe formed at O.
c. A film of refractive index 1.8 is to be placed in front of S1 so that central maxima is formed where 200th maxima was formed. Find the thickness of film.
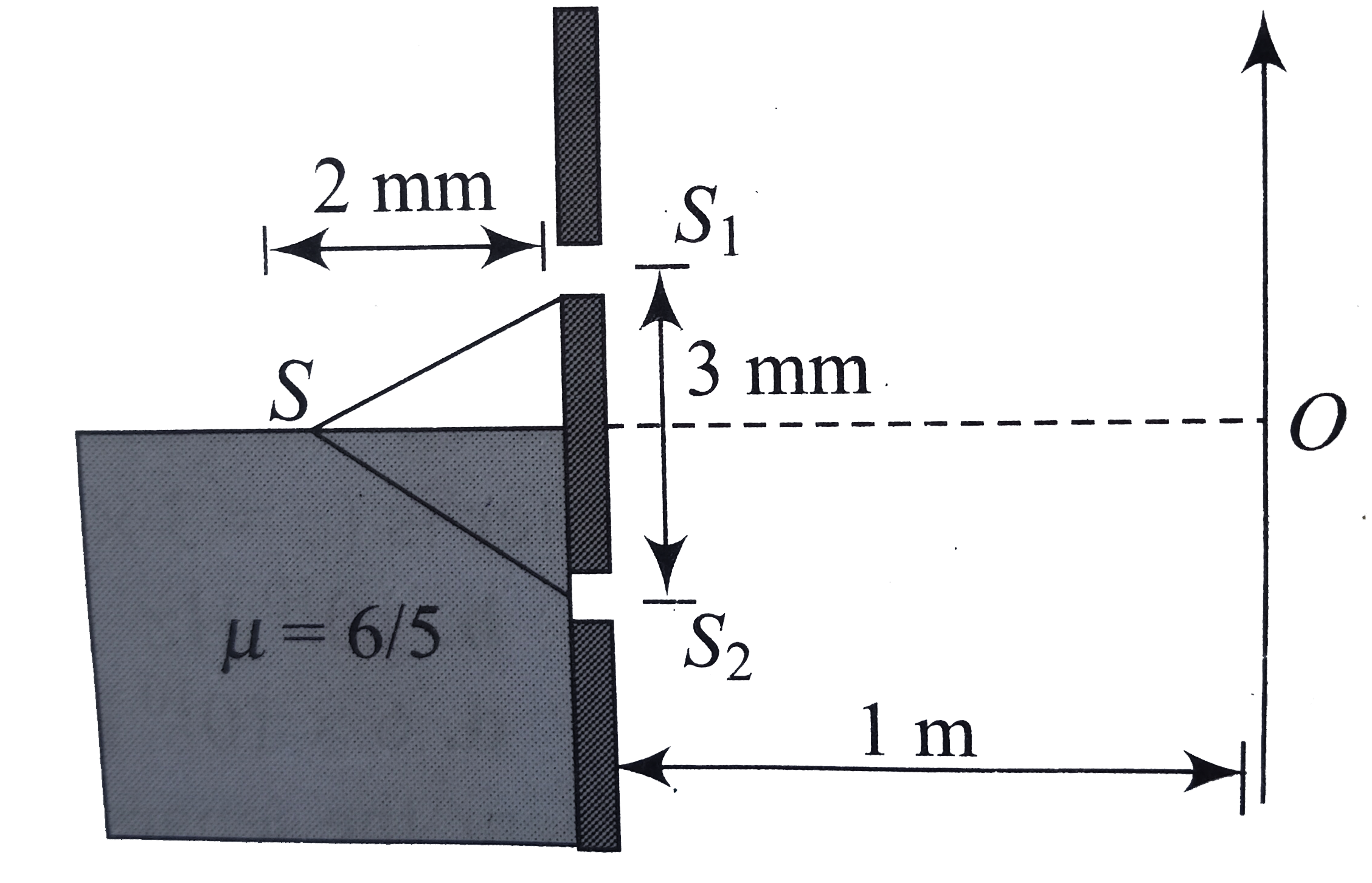 View Solution
View SolutionQuestion 41
In YDSE, the sources is red ligth of wavelength 7×10−7m. When a thin glass plate of refractive index 1.5 is put in the path of one of the interfering beams, the central bright fringe shifts by 10−3 m to the position previously occupied by the 5th bright fringe.
If the source is now changed to green light of wavelength 10−7m, the central fringe shifts to a position initially occupied by the sixth bright fringe due to red ligth. What will be refractive index of glass plate for the second ligth for changed source of ligth?View SolutionQuestion 43
Interference fringes are produced by a double slit arrangement
and a piece of plane parallel glass of refractive index 1.5 is interposed in one of
the interfering beam. If the fringes are displaced through 30 fringe widths for
light of wavelength 6×10−5cm, find the thickness of the plate. View SolutionQuestion 48
In a double-slit experiment, two parallel slits are illuminated first bylight of wavelength 400 nm and then by light of unknown wavelength. The fourth-order dark fringe resulting from the known wavelength of light falls in the same place on the screen as the second-order bright fringe from the unknown wavelength. The value of unknown wavelength of light isView SolutionQuestion 51
Blue light of wavelength 480 nm is most strongly reflected off a thin film of oil on a glass slab when viewed near normal incidence. Assuming that the index of refraction of the oil is 1.2 and that of the glass is 1.6, what is the minimum thickness of the oil film (other then zero)?View SolutionQuestion 52
The slits in a double-slit interference experiment are illuminated by orange light (λ=60nm). A thin transparent plastic of thickness t is placed in front of one of the slits. The nunber of fringes shifting on screen is plotted versus the refractive index μ of the plastic in graph shown in figure. The value of t is
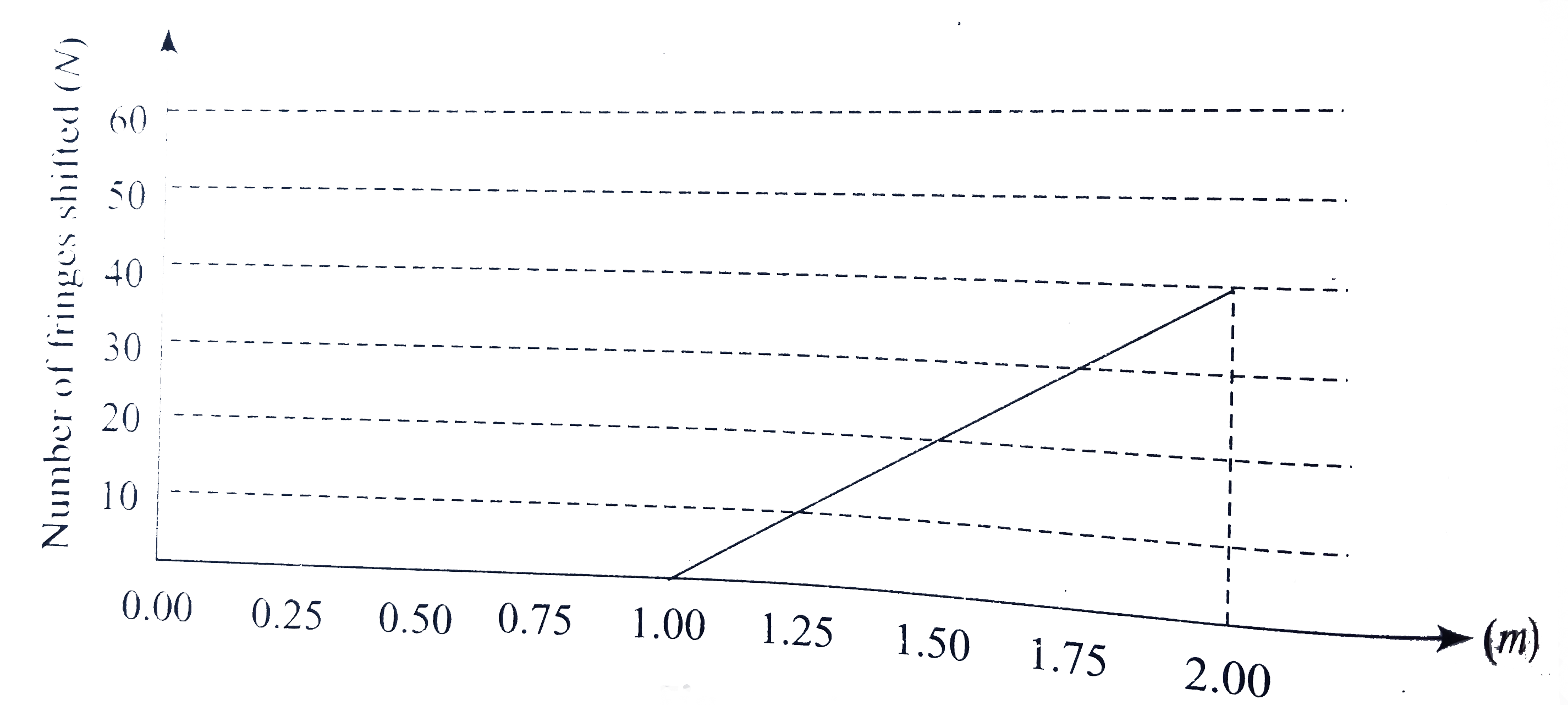 View Solution
View SolutionQuestion 56
In Young's double-slit experiment d/D=10−4 (d = distance between slits, D = distance of screen from the slits) At point P on the screen, resulting intensity is equal to the intensity due to the individual slit I0. Then, the distance of point P from the central maximum is (λ=6000Å)View SolutionQuestion 59
A plane wave of monochromatic light falls normally on a uniform thin or oil which covers a glass plate. The wavelength of source can be varied continuously. Complete destructive is observed for λ=5000Å and λ=1000Å and for no other wavelength in between. If μ of oil is 1.3 and that of glass is 1.5, the thickness of the film will be View SolutionQuestion 62
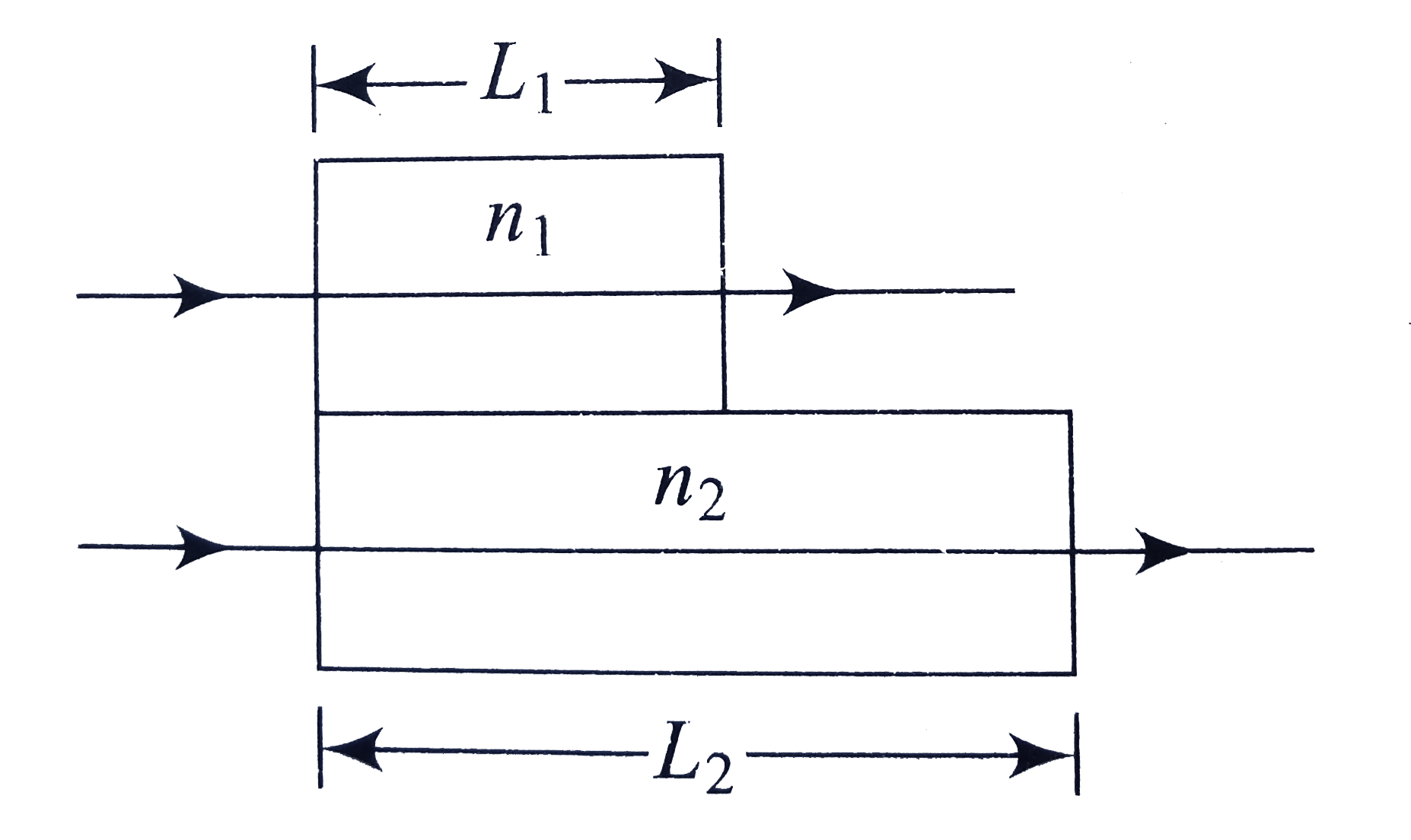
Two light waves having the same wavelength λ in vacuum are in phase initially. Then the first ray travels a path of length L1 through a medium of refractive index μ1. Then second ray travels a path of length L2 throug a medium of refractive index μ2. The two waves are then combined to observed interference effects. The phase difference between the two, when they interfere, is View SolutionQuestion 64
In Young's double-slit experiment, the two slits act as coherent sources of equal amplitude A and of wavelength λ. In another experiment with the same set-up the two slits are sources of equal amplitude A and wavelength λ, but are incoherent. The ratio of the intensity of light at the midpoint of the screen in the first case to that in the second case is....View SolutionQuestion 73
A plate of thickness t made of a material of refractive index μ is placed in front of one of the slits in a double slit experiment. (a) Find the changes in he optical path due to introduction of the plate. (b) Wht should be the minimum thickness t which will make the intensity at the centre of the fringe pattern zero ? Wavelength of the light used is λ. Neglect any absorption of light in the plate.View SolutionQuestion 78
In a standard Young's double-slit experiment with coherent light of wavelength 600 nm, the fringe width of the fringes in the central region (near the central fringe, P0) is observed it to be 3 mm. An extremely thin glass plate is introduced to be displaced by 11 mm. Another thin plate is placed before the second slit and it is observed that the fringe are now displaced by an additional 12 mm. If the additional optical path lengths introduced are Δ1 and Δ2 then
 View Solution
View SolutionQuestion 79
In Young's doulbe-slit experiment the separation between two coherent sources S1 and S2 is d and the distance between the source and screen is D. In the interference pattern, it is found that exactly in front of one slit, there occurs a minimum. Then the possible wavelengths used in the experiment areView SolutionQuestion 89
In the ideal double-slit experiment, when a glass-plate(refractive index 1.5) of thickness t is introduced in the path of one of the interfering beams (wave-length λ), the intensity at the position where the central maximum occurred previously remains unchanged. The minimum thickness of the glass-plate isView SolutionQuestion 93
In YDSE, light of wavelength λ=5000Å is used, which emerges in phase from two a slits distance d=3×10−7m apart. A transparent sheet of thickness t=1.5×106(−7)m, refractive indes n=1.17, is placed over one of the slits. Where does the central maxima of the interference now appear from the center of the screen? (Find the value of y?)
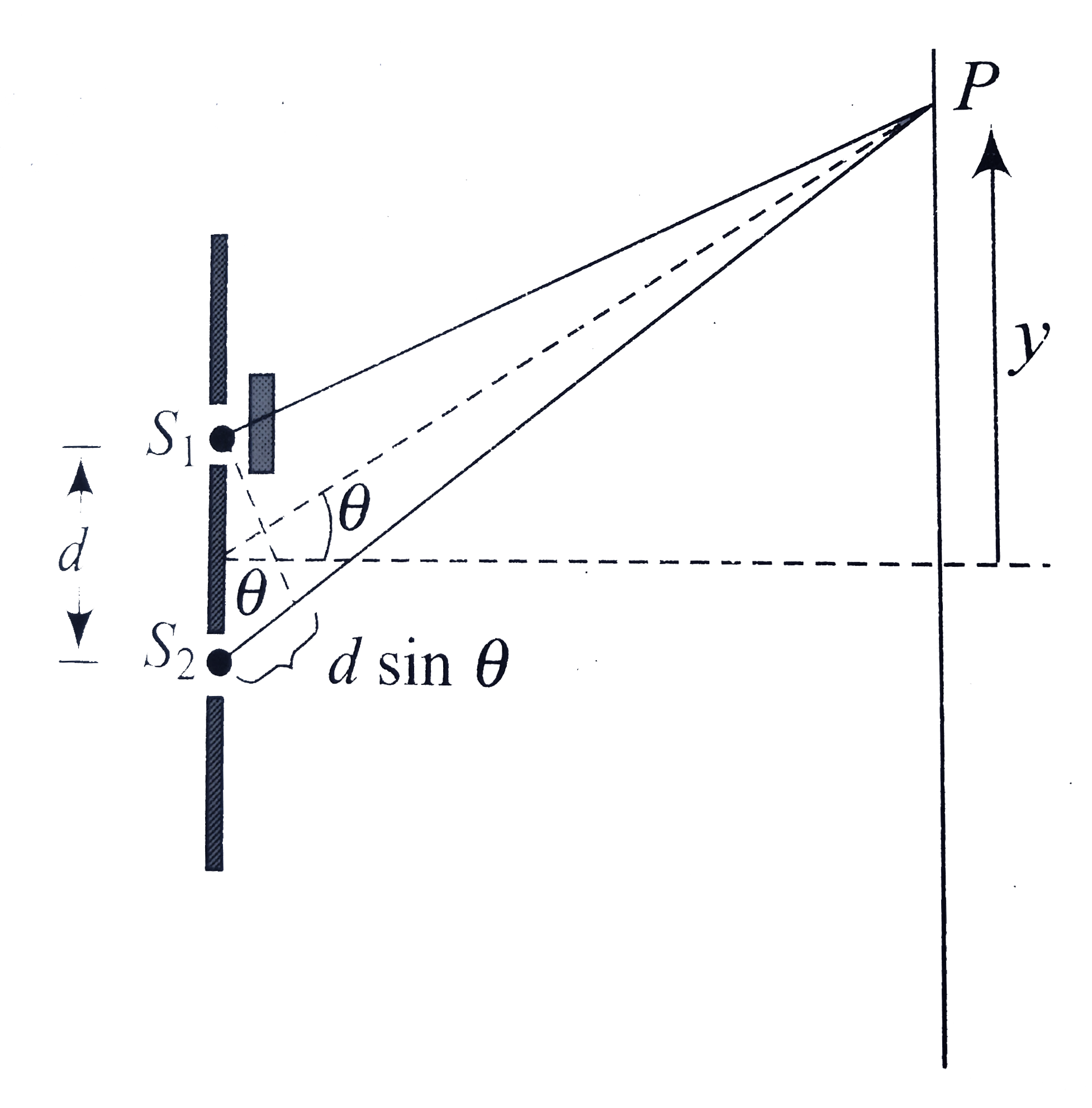 View Solution
View SolutionQuestion 94
A double-slit experiment is immersed in a liquid of refractive index 1.33. It has slit separation of 1 mm and distance between the plane of slits and screen is 1.33 m. The slits are illuminated by a parallel bam of light whose wavelength in air is 6830Å. Then the fringe width isView SolutionQuestion 96
A light of wavelength 6000Å shines on two narrow slits separeted by a distance 1.0 mm and illuminates a screen at a distance 1.5 m away. When one slit is covered by a thin glass plate of refractive index 1.8 and other slit by a thin glass plate of refractive index μ, the central maxima shifts by 0.1 rad. Both plates have the same thickness of 0.5 mm. The value of refractive index μ of the glass isView SolutionQuestion 99
Calculate the wavelength of light used in an interference experiment from the following data: Fringe width = 0.03 cm. Distance between slits and eyepiece through which the interference pattern is observed is 1 m. Distance between the images of the virtural when a convex lens of focal length 16 cm is used at a distance of 80 cm from the eyepiece is 0.8 cm.View SolutionQuestion 102
In a Young's double slit experiment using monochromatic light, the fringe pattern shifts by a certain distance on the screen when a mica sheet of refractive index 1.6 and thickness 1.964 microns is introduced in the path of one of the interfering waves. The mica sheet is then removed and the distance between the slits and screen is doubled. It is found that the distance between successive maxima now is the same as observed fringe shift upon the introduced of the mica sheet . Calculate the wavelength of the monochromatic light used in the experiment .View SolutionQuestion 104
In Young's double-slit experiment, the slit are 0.5 mm apart and the interference is observed on a screen at a distance of 100 cm from the slits, It is found that the ninth bright fringe is at a distance of 7.5 mm from the second dark fringe from the center of the fringe pattern. The wavelength of the light used is View SolutionQuestion 106
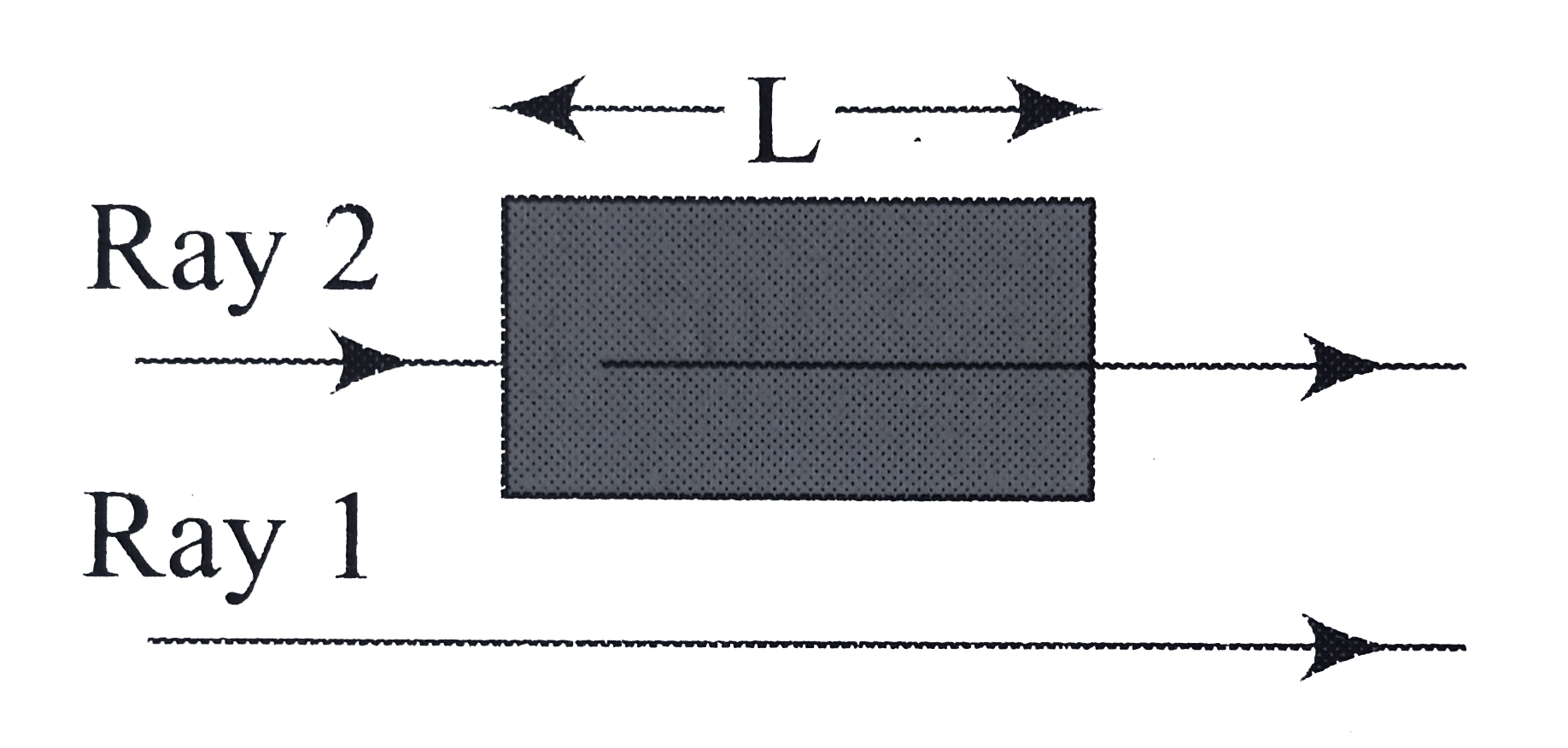
Two waves of light in air have the same wavelength and are intially in phase. They then travel through plastic layers with thickness of L1=3.5mm and L2=5.0mm and indices of refraction n1=1.7 and n2=1.25 as shown in figure. The rays later arrive at a common point. The longest wavelength of light for which constructive interference occurs at the point is
 View Solution
View SolutionQuestion 107
Light from a sources emitting two wavelengths λ1 and λ2 is allowed to fall on Young's double-slit apparatus after filtering one of the wavelengths. The position of interference maxima is noted. When the filter is removed both the wavelengths are incident and it is found that maximum intensity is produced where the fourth maxima occured previously. If the other wavelength if filtered, at the same location the third maxima is found. What is the raito of wavelength?View SolutionQuestion 109
As shown in figure waves with identical wavelengths and amplitudes and which are intially in phase travel through difference media. Ray 1 travels through air and Ray 2 through a transparent medium for equal length L, in four different situations. Rays reach a common point on the screen. The number of wavelengths in length L is N2 for Ray 2 and N1 for Ray 1. In the following table, values of N1 and N2 are given for all four situations. The order or the situations according to the intensity of the light at the common point in descending order is Situations
1 2 3 4
N1 2.75 1.80 3.00 3.25
N2 2.75 2.80 3.25 4.00
 View Solution
View SolutionQuestion 115
In YDSE shown in figure a parallel beam of light is incident on the slits from a medium of refractive index n1. The wavelength of light in this medium is λ1. A transparent of thickness t and refractive index n3 is put in front of one slit. The medium between the screen and the plane of the slits is n2. The phase difference between the light waves reaching point O (symmetrical, relative to the slits) is
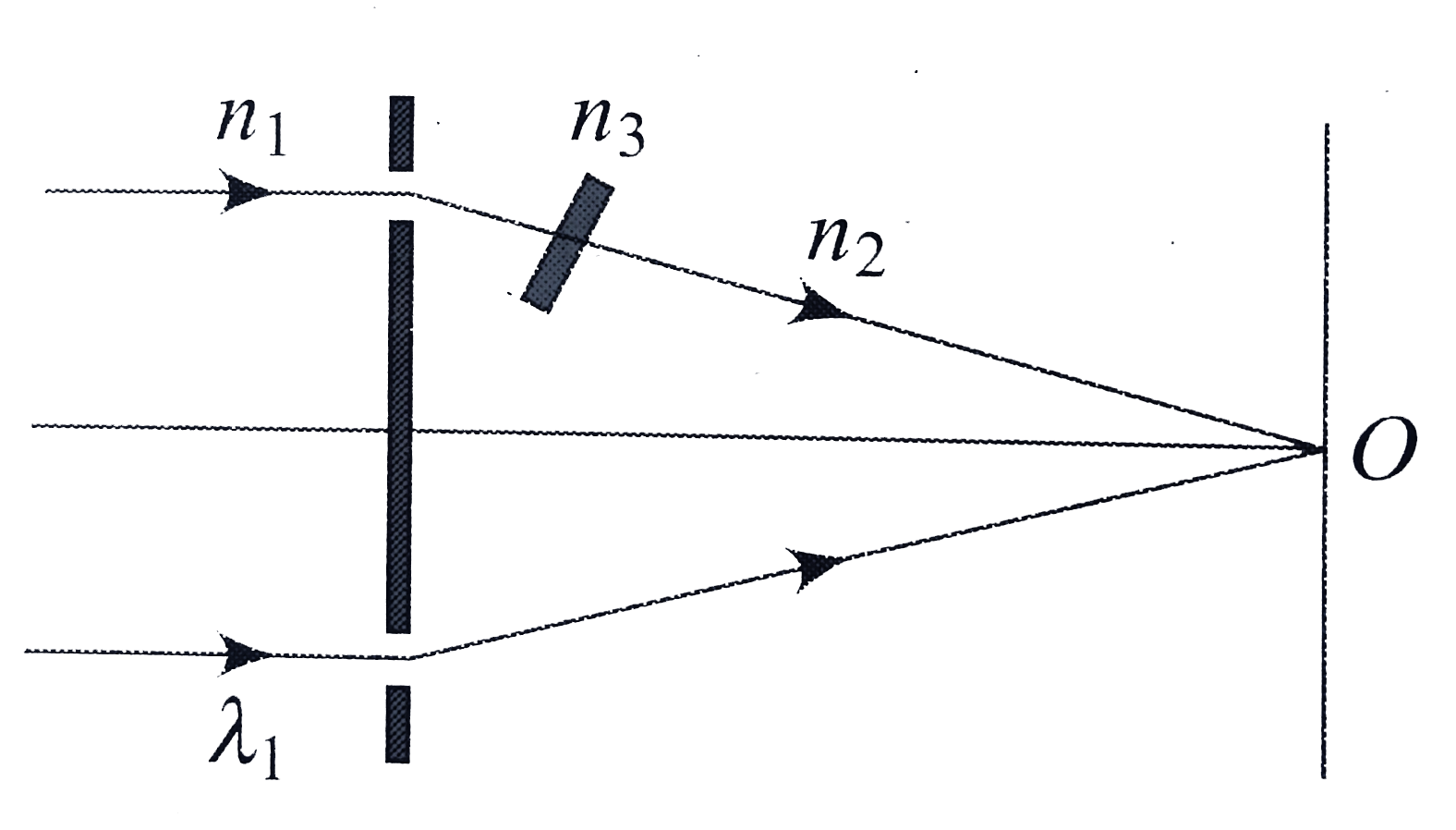 View Solution
View SolutionQuestion 117
In figure, parallel beam of light is incident on the plane of the slits of a Young's double-slit experiment. Light incident on the slit S1 passes through a medium of variable refractive index μ=1+ax (where 'x' is the distance from the plane of slits as shown), up to distance 'I' before falling on S1. Rest of the space is filled with air. If at 'O' a minima is formed. then the minimum value of the positive constant a (in terms of l and wavelength λ in air) is
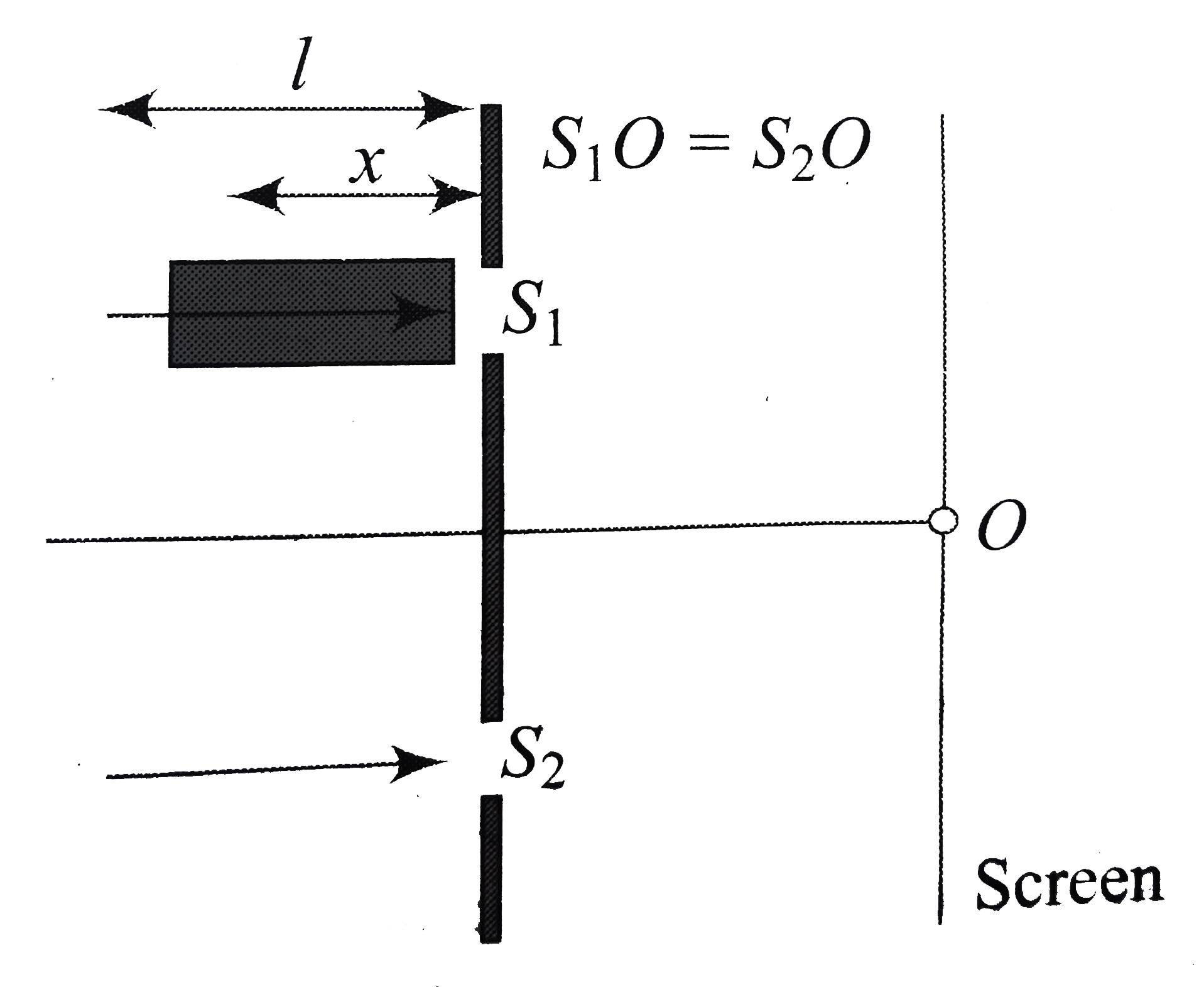 View Solution
View SolutionQuestion 118
Interference fringes were produced using light in a doulbe-slit experiment. When a mica sheet of uniform thickness and refractive index 1.6 (relative to air) is placed in the path of light from one of the slits, the central fringe moves through some distance. This distance is equal to the width of 30 interference bands if light of wavelength 4800 is used. The thickness (in μm) of mica isView SolutionQuestion 137
Two transparent slabs have the same thickness as shown in figure. One in made of material A of refractive index 1.5. The other is made of two materilas B and C with thickness in the ratio 1:2. The refractive index of C is 1.6. If a monochromatic parallel beam passing through the slabs has the same number of wavelengths inside both, the refractive index of B is
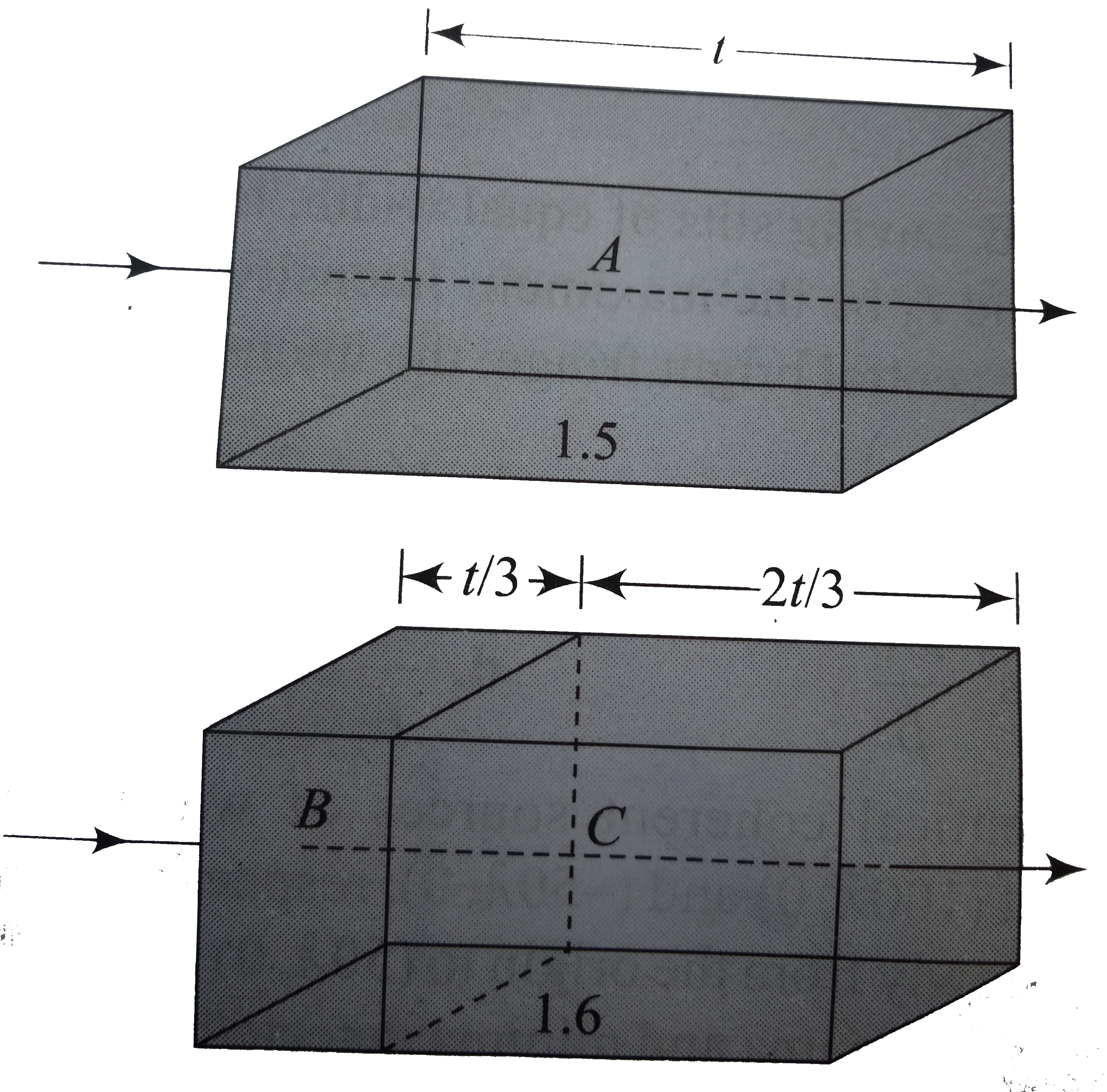 View Solution
View SolutionQuestion 139
In a Young's double slit experiment, the slits are 2mm apart and are illuminated with a mixture of two wavelength λ0=750nm and λ=900nm. The minimum distance from the common central bright fringe on a screen 2m from the slits where a bright fringe from one interference pattern coincides with a bright fringe from the other is View SolutionQuestion 143
A flake of glass (refractive index 1.5) is placed over one of the opening of a double-slit apparatus. The interference pattern displaced itself through seven successive maxima toward the side where the flake is placed. If wavelength of the light is λ=600nm, then the thickness of the flake is View SolutionQuestion 144
Two identical sources each of intensity I0 have a separation d=λ/8, where λ is the wavelength of the waves emitted by either source. The phase difference of the sources is π/4 The intensity distribution I(θ) in the radiation field as a function of θ Which specifies the direction from the sources to the distant observation point P is given byView SolutionQuestion 148
In a Young's double slit experiment using monochromatic light, the fringe pattern shifts by a certain distance on the screen when a mica sheet of refractive index 1.6 and thickness 1.964 microns is introduced in the path of one of the interfering waves. The mica sheet is then removed and the distance between the slits and screen is doubled. It is found that the distance between successive maxima now is the same as observed fringe shift upon the introduced of the mica sheet . Calculate the wavelength of the monochromatic light used in the experiment .View SolutionQuestion 151
YDSE is carried with two thin sheets of thickness 10.4μ m each and refractive index μ1=1.52andμ2=1.40 covering the slits S1andS2 respectively. If white light of range 400nm to 780 nm is used, then which wavelength will form maxima exactly at point O, the centre of the screen

View SolutionQuestion 152
High-quality camera lenses are often coated to prevent reflection. A lens has an optical index of refraction of 1.72 and a coating with an optical index os refraction 1.31. For near normal incidence, the minimum thickness of the coating to prevent reflection for wavelength of 5.3×10−7m isView SolutionQuestion 153
A long horizontal slit is placed 1 mm above a horizontal plane mirror. The interference between the light coming directly from the slit and the after reflection is seen on a screen 1 m away from the slit. If the mirror reflects only 64% of the light falling on it, the ratio of the maximum to the minimum intensity in the interference pattern observed on the screen is View SolutionQuestion 155
In young's double-slit experiment set up, sources S of wavelength 50 nm illumiantes two slits S1 and S2 which act as two coherent sources. The sources S oscillates about its own position according to the equation y=0.5sinπt, where y is in nm and t in seconds. The minimum value of time t for which the intensity at point P on the screen exaclty in front of the upper slit becomes minimum is
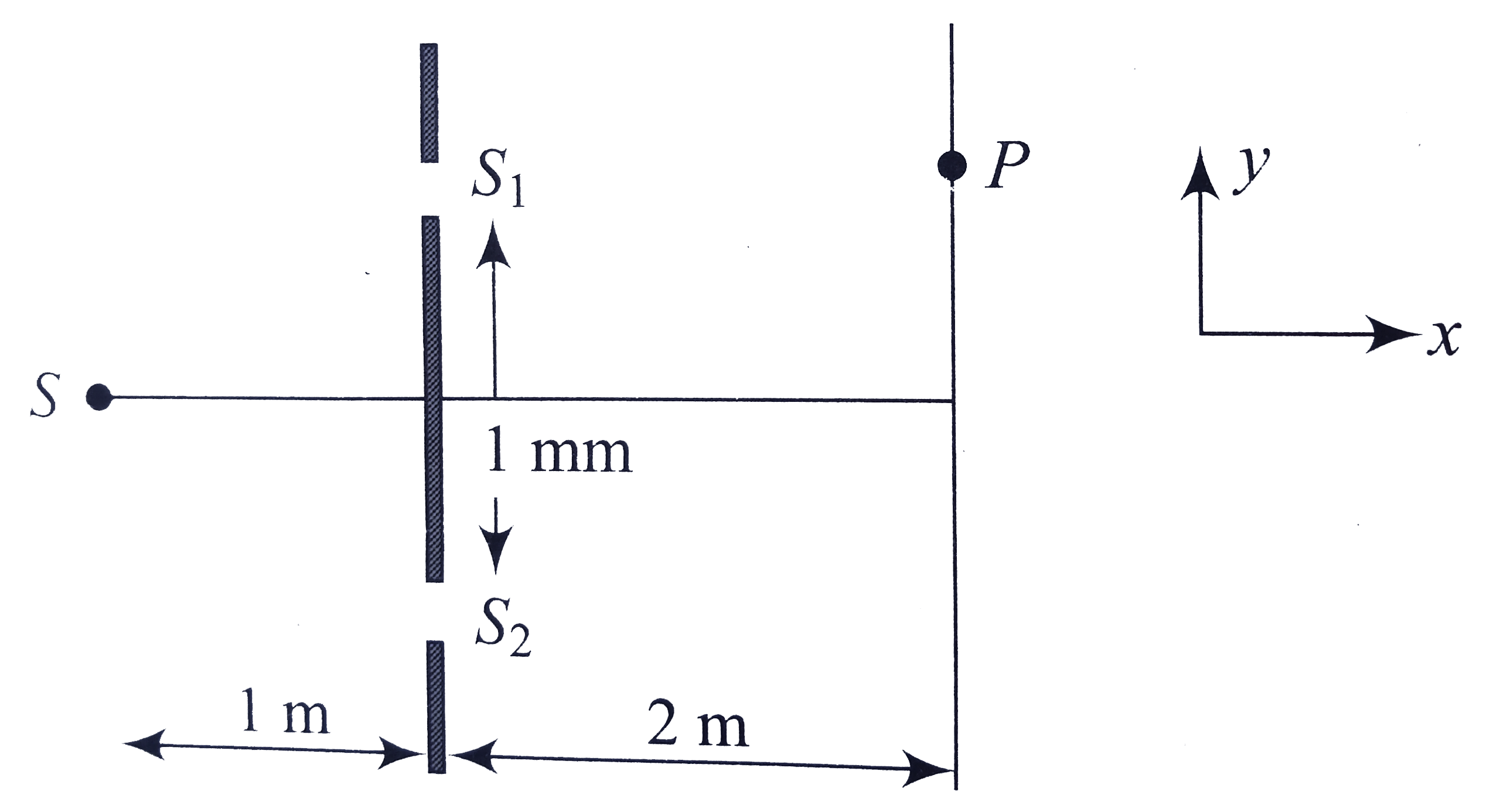 View Solution
View SolutionQuestion 157
A thin uniform film of refractive index 1.75 is placed on a sheet of glass of refractive index 1.5. At room temperature (20∘C) this film is just thick enoungh for light with wavelength 600 nm reflected off the top of the film to be canceled by light reflected from the top the glass. After the glass is placed in on oven and slowly heated to 170∘C, the film conceals reflected light with wavelength 606 nm. The coefficient of linear expansion of the film is (ignore any changes in the refractive index of the film due to the temperature change)View SolutionQuestion 158
Two slits speet 0.25 mm apart are placed 0.75 m from a screen and illuminated by coherent light with a wavelength of 650 nm. The intensity at the center of the central maximum (θ=0∘) is I0. The distance on the screen from the center of the central maximum to the point where the intensity has fallen to I0/2 is nearlyView SolutionQuestion 160
The index of refraction of a glass plate is 1.48 at θ1=30.∘C and varies linearly with temperature with a coefficient of 2.5×10−5.∘C−1. At 30.∘C, the length of the glass plate is 3 cm. This plate is placed in front of one of the slit n Young's double-slit experiment. If the plate is being heated so that it temperature increases at a rate of 5∘C−1 min, the light sources has wavelength λ=589nm and the glass plate initially ia at θ=30∘C. The number of fringes that shift on the screen in each minute is nearly (use approximation)View SolutionQuestion 161
Two thin parallel slits are made in on opaque sheet of film when a monochromatic beam of light is shonw through then at normal incidence. The first bright fringes in the transmitted light occur at ±45∘ with the original direction of the ligth beam on a distant screen when the apparatus is in air. When the apparatus is immersed in a liquid, the same bright fringe now occur at ±30∘. The index of refraction of the liquid isView SolutionQuestion 166
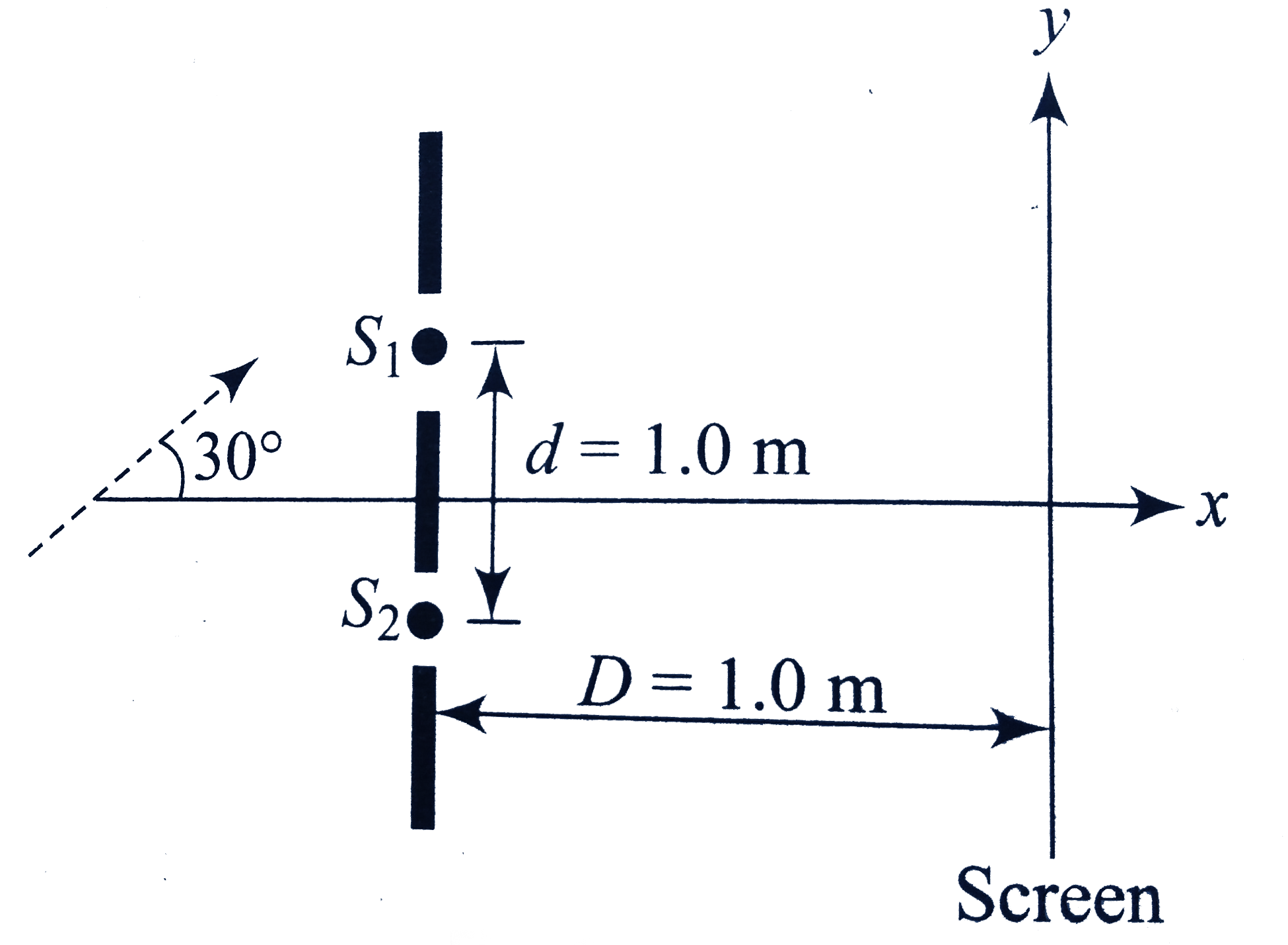
A parallel beam of light (λ=500) is incident at an angle α=30∘ with the normal to the slit plane in Young's double-slit experiment. Assume that the intensity due to each slit at any point on the screen is I0. Point O is equidistant from S1 and S2. The distance between slits is 1 mm. Then
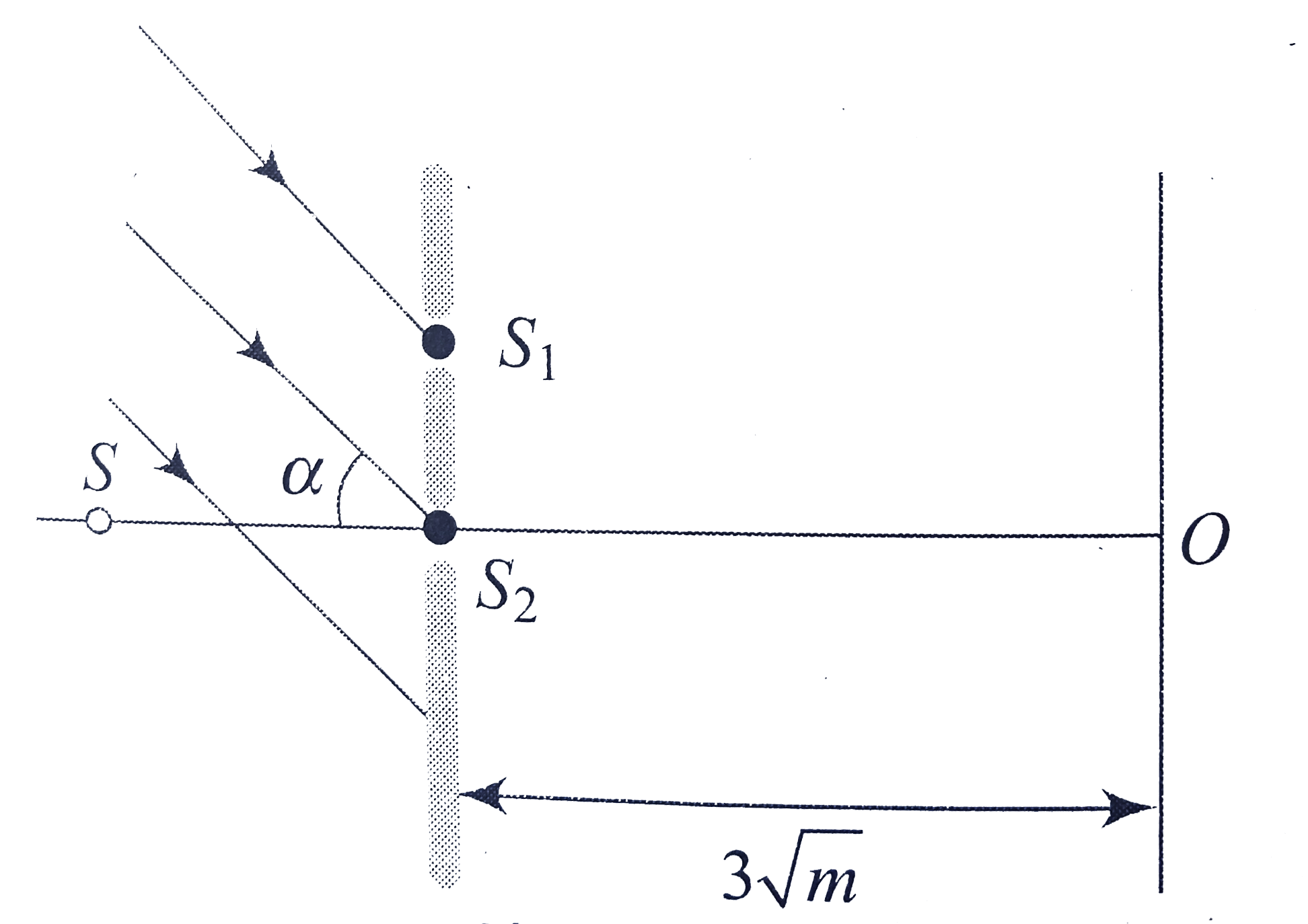 View Solution
View SolutionQuestion 171
A light wave of wavelength λ0 propagates from point A to point B. We introduce in its path a glass plate of refractive index n and thickness l. Then introduction of the plate alters the phase of the plate at B by an angle ϕ. If λ is the wavelength of light on emeriging from the plate, thenView SolutionQuestion 177
A radio transmitting station operating at a frequency of 120 MHz has two identical antennas that radiate in phase. Antenna B is 9 m to the right of antenna A. consider point P at a horizontal distance x to the right of antenna A as shown in figure. The value of x and order for which the constructive interference will occur at point P are
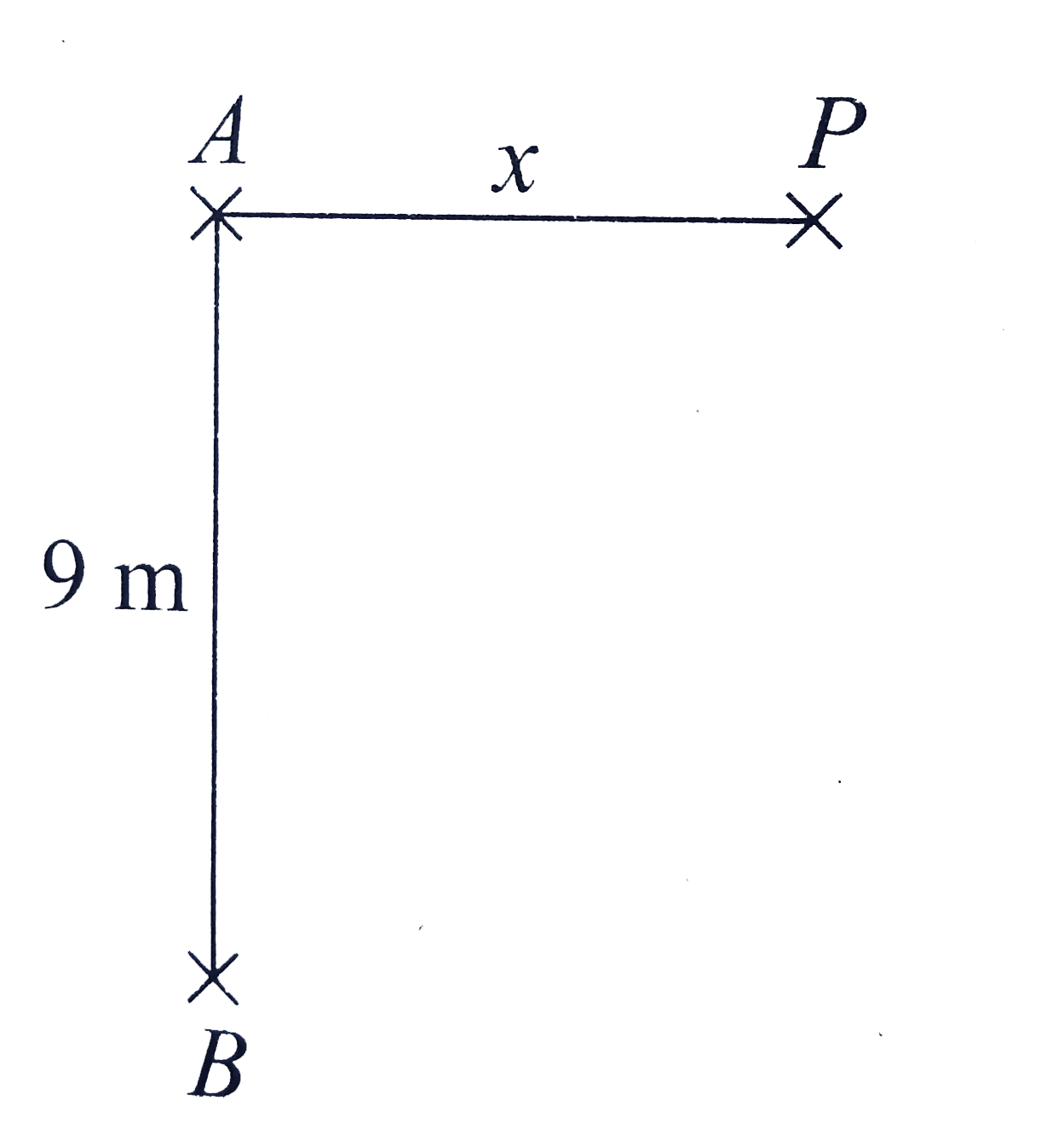 View Solution
View SolutionQuestion 178
A two-slit inteference, experiment uses coherent light of wavelength 5×10−7m. Intensity in the interference pattern for the following points are I1,I2,I3, and I4, respectively
1. A point that is close to one slit than the other by 5×10−7m.
2. A point where the light waves received from the two slits are out of phase by 4π3 rad.
3. A point that is closer to one slit than the other by 7.5×10−7m.
4. A point where the light waves received by the two slits are out of phase by π36 rad.
Then which of following statements is/are correct?View SolutionQuestion 181
Statement I: In Young's double-slit experiment, the two slits are at distance d apart. Interference pattern is observed on a screen at distance D from the slits. At a point on the screen which is directly opposite to one of the slits, a dark fringe is observed. Then, the wavelength of wave is proportional to the squar of distance between two slits.
Statement II: For a dark fringe, intensity is zeroView SolutionQuestion 187
Statement I: Two point coherent sources of light S1 and S2 are placed on a line as shown in figure. P and Q are two points on that line. If at point P maximum intensity is observed, then maximum intensity should also be observed at Q.

Statement II: In figure the distance |S1P−S2P| is equal to distance |S1Q−S2Q|. View SolutionQuestion 188
Statement I: Two coherent point sources of light having no-zero phase difference are separated by a small distance. Then, on the perpendicular bisector of line segment joining both the point sources, constructive inteference cannot be obtained.
Statement II: For two waves from coherent point sources to interfere constructively at a point, the magnitude of their phase difference at that point must be 2mπ (where m is non-negative integer).View SolutionQuestion 190
Statement I: For the situation shown in figure two identecal coherent light sources produce interference pattern on the screen. The intensity of minima nearest to S1 is not exactly zero.

Statement II: Minimum intensity is zero, when interfering waves have same intensity at the location of superposition.View SolutionQuestion 193
Statement I: An electron beam is used to obtain interference in a simple Young's double-slit experiment arrangement with appropriate distance between the slits. If the speed of electrons in increased, the width decreases.
Statement II: de Broglie wavelength of electron is inversely proportional to the speed of the electrons.View SolutionQuestion 194
A narrow tube is bent in the form of a circle of radius R, as shown in figure. Two small holes S and D are made in the tube at the position at right angle to each other. A source placed at S generates a wave of intensity I0 which two parts: one part travels along the longer path, while the other travels along the shorter path. Both the waves meet at point D where a detector is placed.
1. If a maxima if formed at a detector, then the magnitude of wavelength λ of the, wave produced is given byView SolutionQuestion 195
A narrow tube is bent in the form of a circle of radius R, as shown in figure. Two small holes S and D are made in the tube at the position at right angle to each other. A source placed at S generates a wave of intensity I0 which two parts: one part travels along the longer path, while the other travels along the shorter path. Both the waves meet at point D where a detector is placed.
2. If a minima is formed at the detector, then the magnitude of wavelength λ of the wave produced is given byView SolutionQuestion 196
A narrow tube is bent in the form of a circle of radius R, as shown in figure. Two small holes S and D are made in the tube at the position at right angle to each other. A source placed at S generates a wave of intensity I0 which two parts: one part travels along the longer path, while the other travels along the shorter path. Both the waves meet at point D where a detector is placed.
The maximum intensity produced at D is given byView SolutionQuestion 197
A thin film of a specific meterial can be used to decrease the intensity of reflected light. There is destrucive inteference of wave reflected from upper and lower surface of the film. These films are called non-reflecting or anti-reflecting coatings. The process of coating the lens or surface with non-reflecting film is called blooming as shown in figure The refracting index of coating (n1) is less than that of the glass (n2).

4. If a light of wavelength λ is incident normally and the thickness of film is t, then optical path difference between waves reflected from upper and lower surface of the film isView SolutionQuestion 198
A thin film of a specific meterial can be used to decrease the intensity of reflected light. There is destrucive inteference of wave reflected from upper and lower surface of the film. These films are called non-reflecting or anti-reflecting coatings. The process of coating the lens or surface with non-reflecting film is called blooming as shown in figure The refracting index of coating (n1) is less than that of the glass (n2).

5. magnesium fluoride (MgF2) is generally use as anti-reflection coating. If refractive index of MgF2 is 1.25, then minimum thickness of film required is (Take λ=500nm)View SolutionQuestion 199
A thin film of a specific meterial can be used to decrease the intensity of reflected light. There is destrucive inteference of wave reflected from upper and lower surface of the film. These films are called non-reflecting or anti-reflecting coatings. The process of coating the lens or surface with non-reflecting film is called blooming as shown in figure The refracting index of coating (n1) is less than that of the glass (n2).

6. If the thickness of film in above question in not technologically possible to manufacture, then next thickness of film required is (approximately)View SolutionQuestion 200
In a Young's double-slit experiment set up, source S of wavelength 6000Å illuminates two slits S1 and S2 which act two coherent sources. The sources S oscillates about its shown position according to the eqation y=1+cosπt, where y is in millimeter and t in second.
At t=0, fringe width is β1, and at t=2 s, width of figure is β2. ThenView SolutionQuestion 201
In a Young's double-slit experiment set up, source S of wavelength 6000Å illuminates two slits S1 and S2 which act two coherent sources. The sources S oscillates about its shown position according to the eqation y=1+cosπt, where y is in millimeter and t in second.
At t=2 s, the position of central maxima is View SolutionQuestion 202
In a Young's double-slit experiment set up, source S of wavelength 6000Å illuminates two slits S1 and S2 which act two coherent sources. The sources S oscillates about its shown position according to the eqation y=1+cosπt, where y is in millimeter and t in second.
At t=1 s, a slab of thickness 2×10−3mm an refractive index 1.5 is placed just in front of S1. The central maximum is formed atView SolutionQuestion 205
In YDSE, the sources is red ligth of wavelength 7×10−7m. When a thin glass plate of refractive index 1.5 is put in the path of one of the interfering beams, the central bright fringe shifts by 10−3 m to the position previously occupied by the 5th bright fringe.
What is the thickness of the plate?View SolutionQuestion 206
In YDSE, the sources is red ligth of wavelength 7×10−7m. When a thin glass plate of refractive index 1.5 is put in the path of one of the interfering beams, the central bright fringe shifts by 10−3 m to the position previously occupied by the 5th bright fringe.
If the source is now changed to green light of wavelength 10−7m, the central fringe shifts to a position initially occupied by the sixth bright fringe due to red ligth. What will be refractive index of glass plate for the second ligth for changed source of ligth?View SolutionQuestion 207
In YDSE, the sources is red ligth of wavelength 7×10−7m. When a thin glass plate of refractive index 1.5 is put in the path of one of the interfering beams, the central bright fringe shifts by 10−3 m to the position previously occupied by the 5th bright fringe.
Change is fringe width produced due to chanbe in wavelength isView SolutionQuestion 208
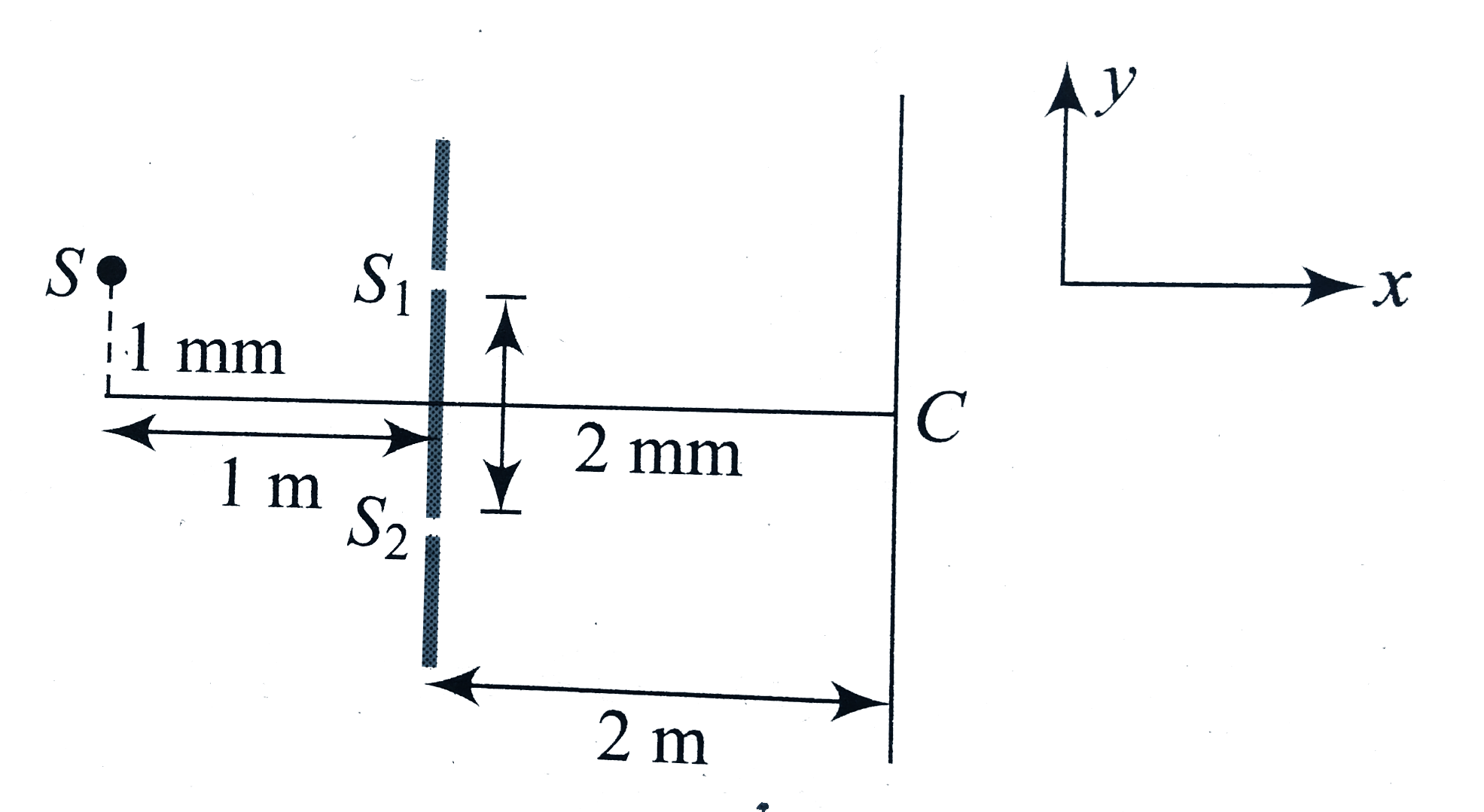
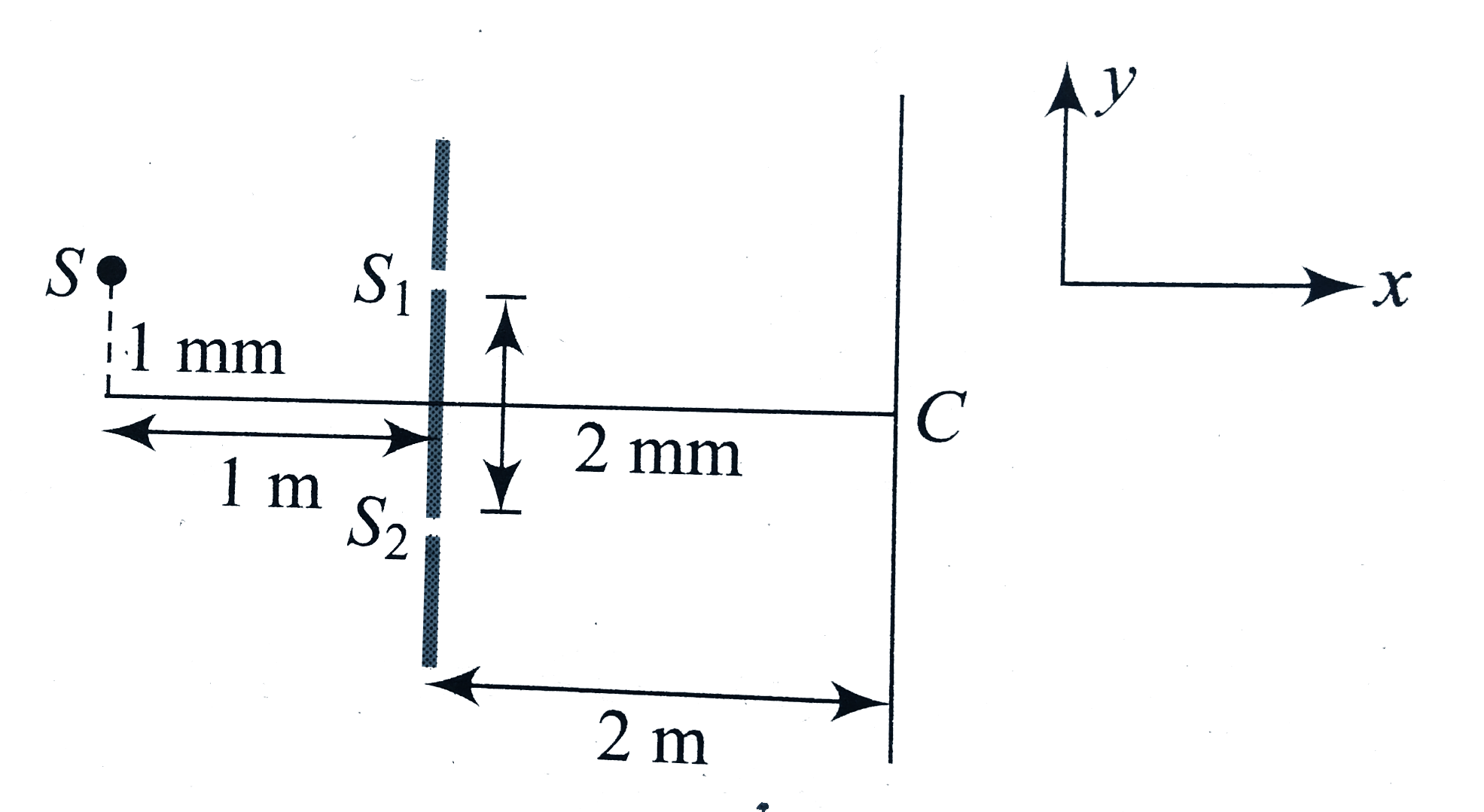
A coherent parallel beam of microwaves of wavelength λ=0.5mm falls on aYoung's double- slit apparatus. The separation between the slits is 1.0 mm. The intensity of microwaves is measured on a screen placed parallel to the plane of the slits at a distance of 1.0 m from it as shown in figure
If the incient beam falls normally on the double-slit apparatus, find the order of the interference minima on the screenView SolutionQuestion 209
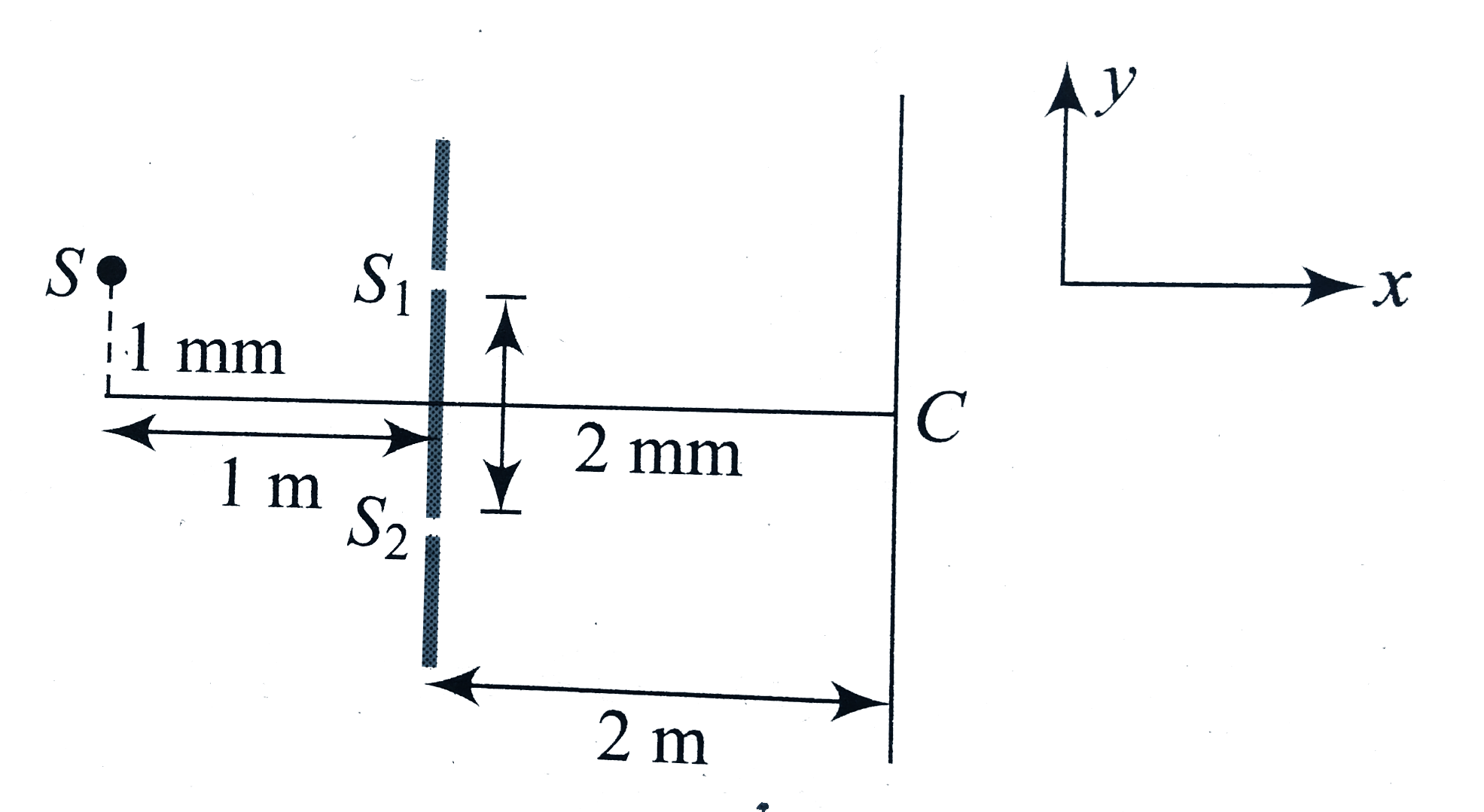
A coherent parallel beam of microwaves of wavelength λ=0.5mm falls on aYoung's double- slit apparatus. The separation between the slits is 1.0 mm. The intensity of microwaves is measured on a screen placed parallel to the plane of the slits at a distance of 1.0 m from it as shown in Fig. 2.42.
If the incident beam makes an angle or 30∘ with the x-axis (as in the dotted arrow shown in the figure), find the y-coordinates of the first minima on either side of the central maximum.
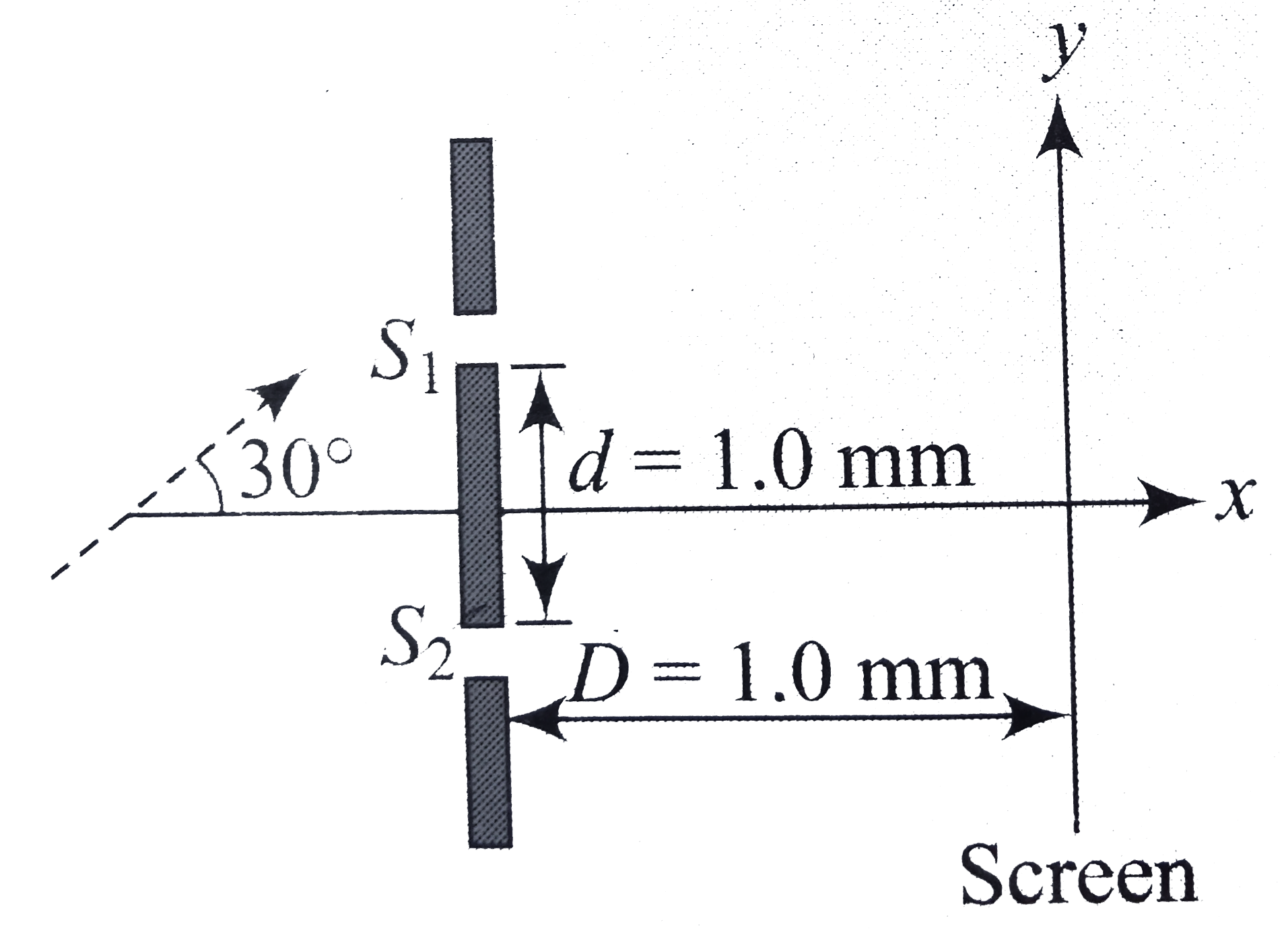 View Solution
View SolutionQuestion 210
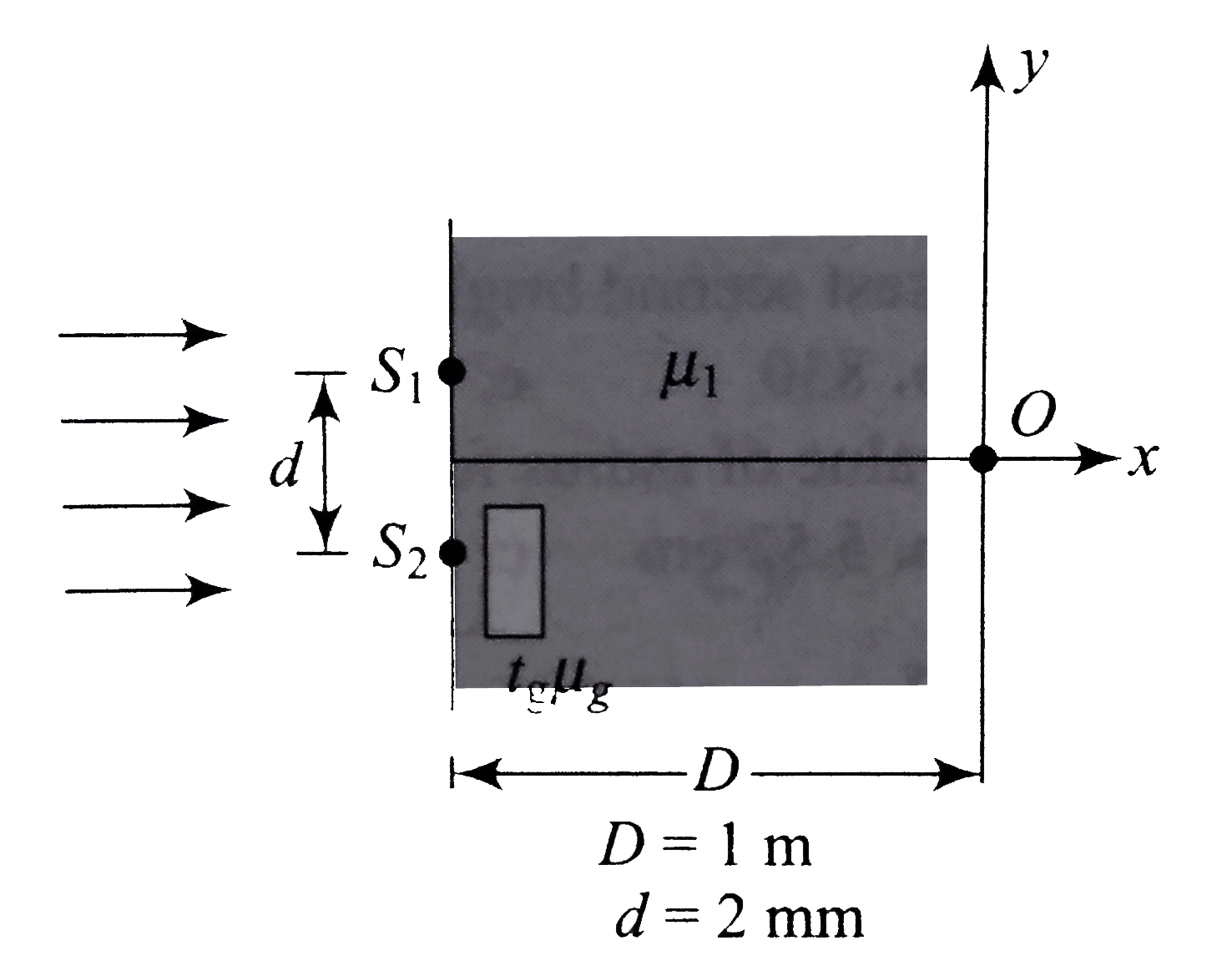
A YDSE is performed in a medium of refractive index 4/3, A light of 600 nm wavelength is falling on the slits having 0.45 nm separation . The lower slit S2 is covered b a thin glass plate of thickness 10.4 mm and refractive index 1.5. The interference pattern is observed on a screen placed 1.5 m from the slits as shown in figure. (All the wavelengths in this problem are for the given medium of refractive index 4/3, ignore absorption.)
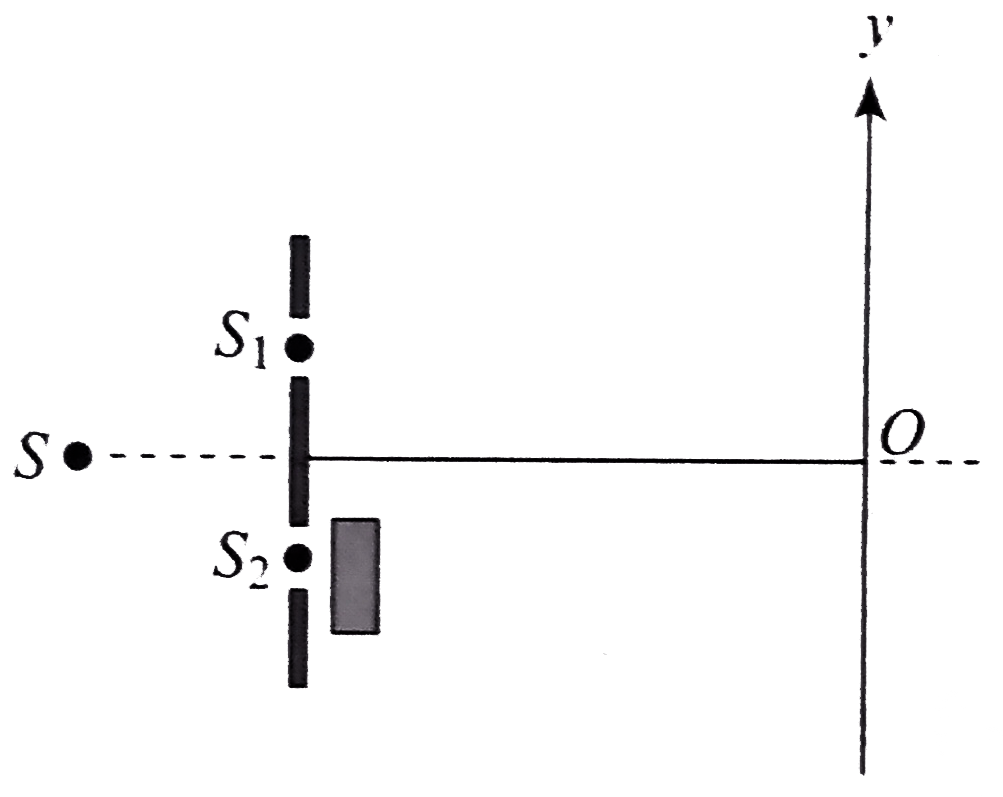
The location of the central maximum (bright fringe with zero path difference) on the y-axis will beView SolutionQuestion 211
A YDSE is performed in a medium of refractive index 4/3, A light of 600 nm wavelength is falling on the slits having 0.45 nm separation . The lower slit S2 is covered b a thin glass plate of thickness 10.4 mm and refractive index 1.5. The interference pattern is observed on a screen placed 1.5 m from the slits as shown in figure. (All the wavelengths in this problem are for the given medium of refractive index 4/3, ignore absorption.)
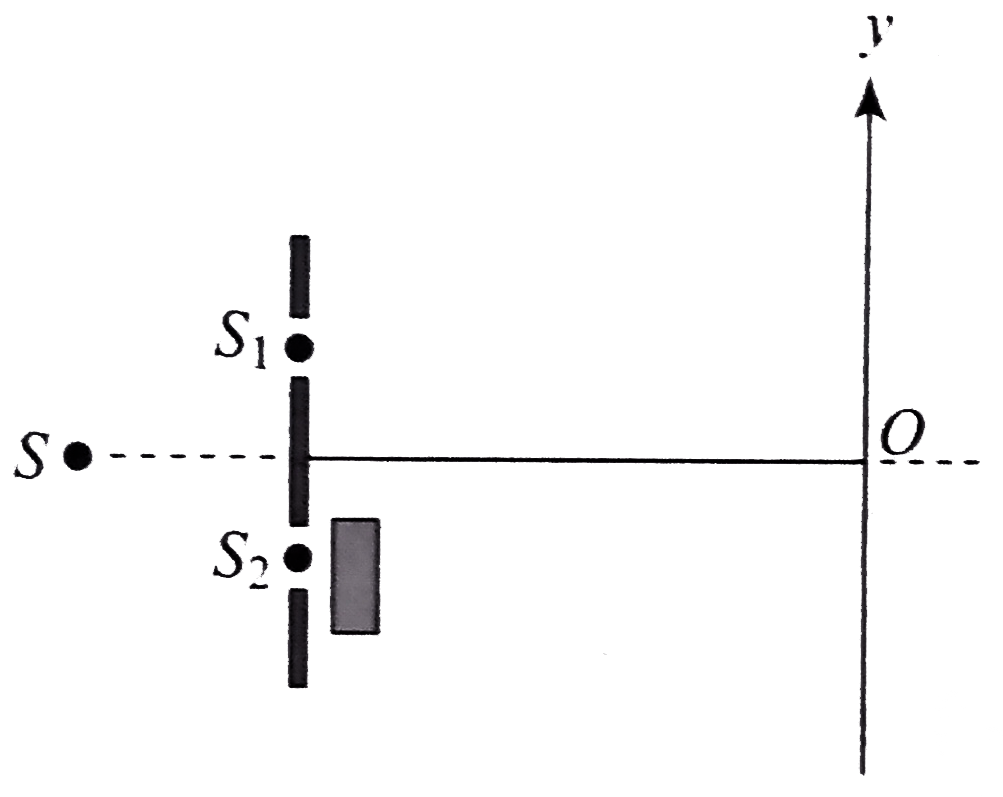
Find the light intensity at point O relative t maximum fringe intensity.View SolutionQuestion 212
A YDSE is performed in a medium of refractive index 4/3, A light of 600 nm wavelength is falling on the slits having 0.45 nm separation . The lower slit S2 is covered b a thin glass plate of thickness 10.4 mm and refractive index 1.5. The interference pattern is observed on a screen placed 1.5 m from the slits as shown in figure. (All the wavelengths in this problem are for the given medium of refractive index 4/3, ignore absorption.)
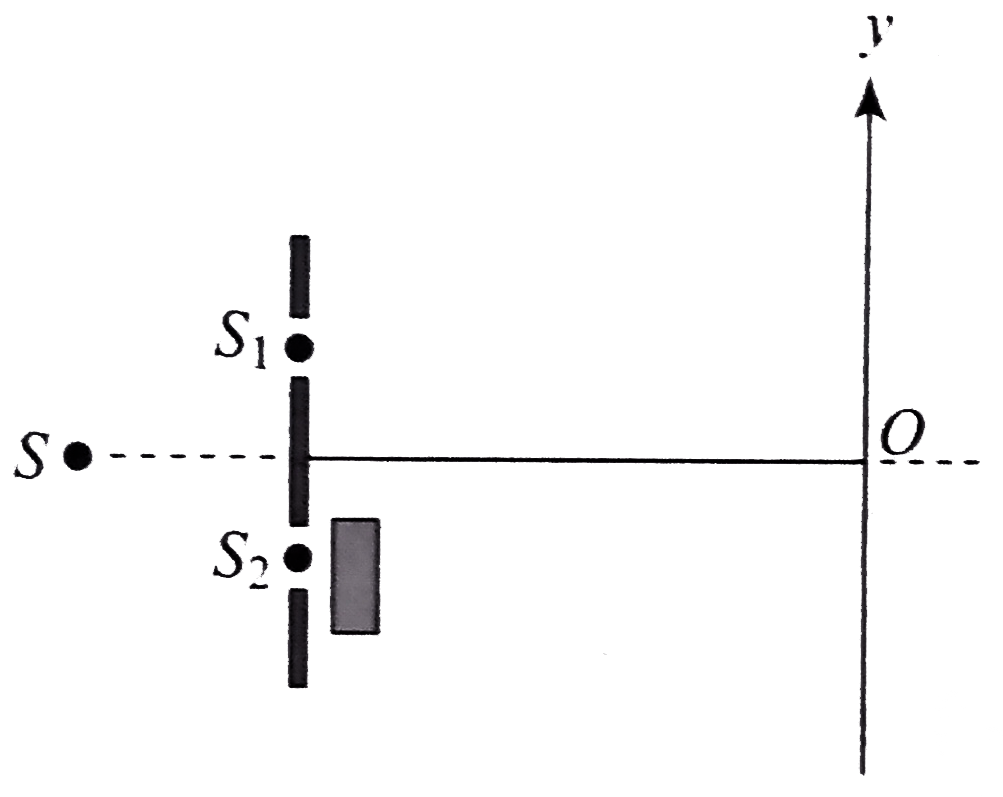
Now, if 600 nm, find the wavelength of the ligth that forms maximum exactly at point O.View SolutionQuestion 213
In a YDSE perfromed with light of wavelength 600Å, the screen is placed 1 m from the slits. Fringes formed on the screen are observed by a student sitting close to the slits. The student's eye can distinguish two neighboring fringes. If they subtend an angle more then 1 minute of ace, then
In order to have the clear visibility of the fringe, the maximum distance that can be maintained between the slits isView SolutionQuestion 214
In a YDSE perfromed with light of wavelength 600Å, the screen is placed 1 m from the slits. Fringes formed on the screen are observed by a student sitting close to the slits. The student's eye can distinguish two neighboring fringes. If they subtend an angle more then 1 minute of ace, then
Find the location of third bright fringe from center of the screen.View SolutionQuestion 215
In a YDSE perfromed with light of wavelength 600Å, the screen is placed 1 m from the slits. Fringes formed on the screen are observed by a student sitting close to the slits. The student's eye can distinguish two neighboring fringes. If they subtend an angle more then 1 minute of ace, then
Under similar conditions or arrangement, what will be the position of fifth dark fringe from the center of screen?View SolutionQuestion 216
In a modified YDSE, sources S is kept in front of slit S1. Find the phase difference at point O that is equidistant from slits S1 and S2 and point P that is in front of slit S1 in the following situations.
A liquid of refractive index μ is filled between the screen and slits.View SolutionQuestion 218
In a modified YDSE, the region between the screen and slits is immersed in a liquid whose refractive index varies with time as μ1=(5/2)−(T/4) until it reaches s steady state value of 5/4. A glass plate of thickness 36 μm and refractive index 3/2 is introduced in front of one of the slits.
Find the time when central maxima is at point O. located symmetrically on the x-asix.View SolutionQuestion 219
In a modified YDSE, the region between the screen and slits is immersed in a liquid whose refractive index varies with time as μ1=(5/2)−(T/4) until it reaches s steady state value of 5/4. A glass plate of thickness 36 μm and refractive index 3/2 is introduced in front of one of the slits.

What is the speed of the central maxima when it is atView SolutionQuestion 220
In a YDSE using monochromatic visible light, the distance between the plate of slits and the screen is 1.7 m. At point P on the screen which is directly in front of the upper slit, maximum path is observed. Now, the screen is moved 50 cm closer to the plane of slits. Point P now lies between third and fouth minima above the central maxima and the intensity at P in one-fourth of the maxima intensity on the screen.
Find the value of n.View SolutionQuestion 221
In a YDSE using monochromatic visible light, the distance between the plate of slits and the screen is 1.7 m. At point P on the screen which is directly in front of the upper slit, maximum path is observed. Now, the screen is moved 50 cm closer to the plane of slits. Point P now lies between third and fouth minima above the central maxima and the intensity at P in one-fourth of the maxima intensity on the screen. ltbr> Find the wavelength of light if the separation of slits is 2 mm.View SolutionQuestion 225
In the arrangement shown in figure, light of wavelength 6000Å is incident on slits S1 and S2 have been opened such that S3 is the position of first maximum above the central maximum and S4 is the closest position where intensity is same as that of the ligth used, below the central maximum Point O is equidistant from S1 and S2 and O' is equidistant from S3 and S4. Then intensity of inciden light is I0
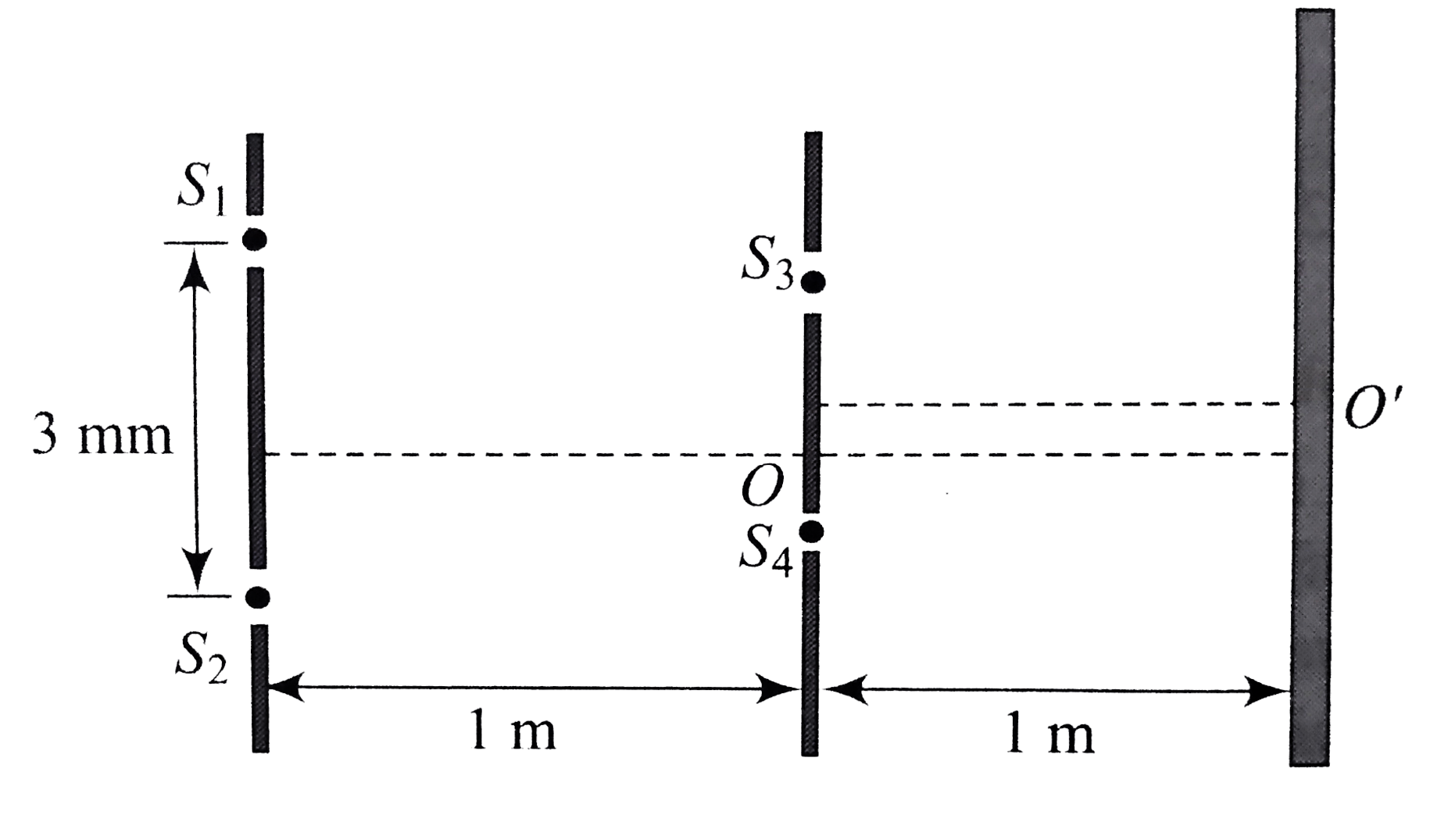
Find the intensity at O' (on the screen).View SolutionQuestion 226
In the arrangement shown in figure, light of wavelength 6000Å is incident on slits S1 and S2 have been opened such that S3 is the position of first maximum above the central maximum and S4 is the closest position where intensity is same as that of the ligth used, below the central maximum Point O is equidistant from S1 and S2 and O' is equidistant from S3 and S4. Then intensity of inciden light is I0
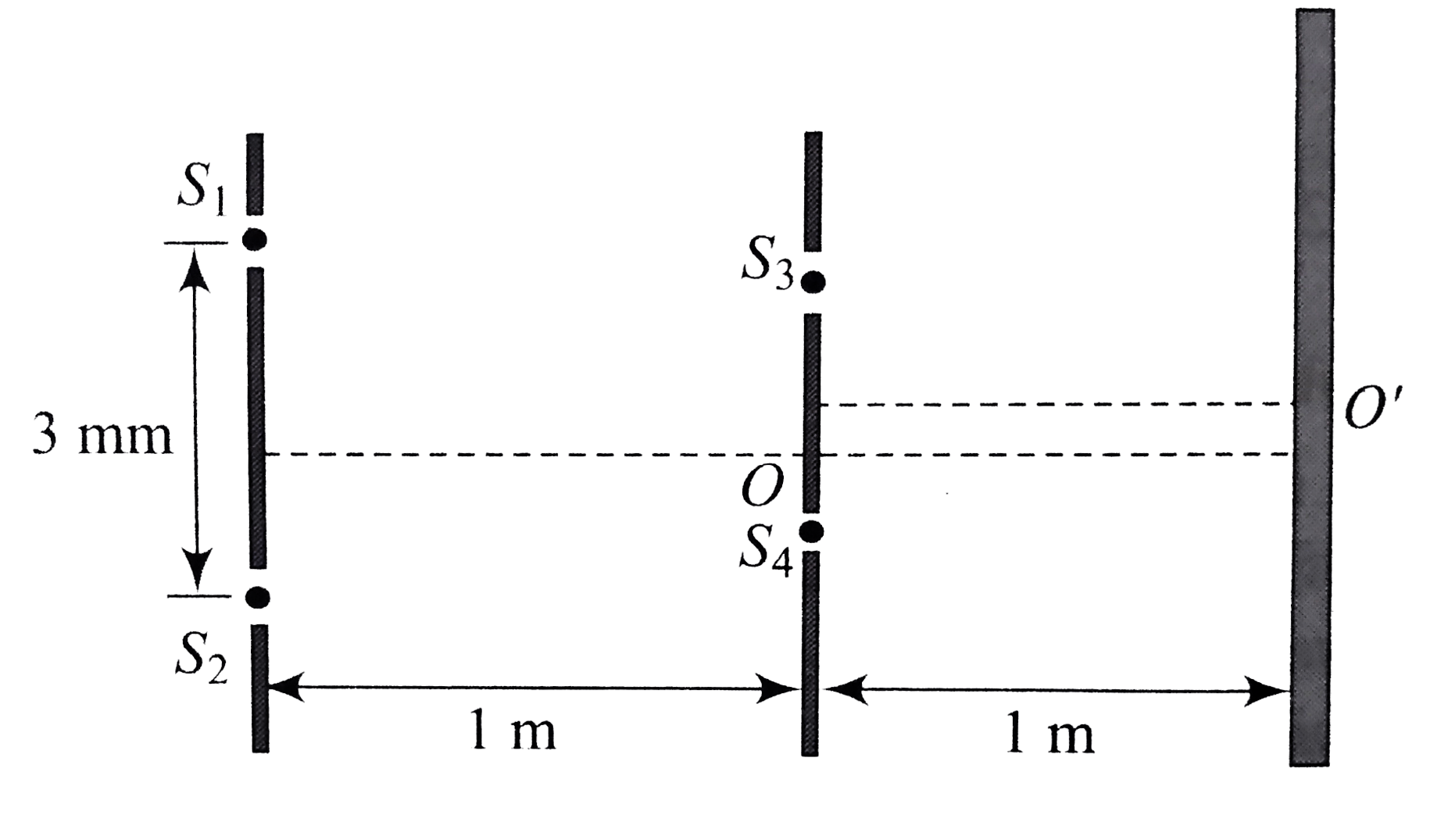
Find the intensity of the brightest fringe.View SolutionQuestion 227
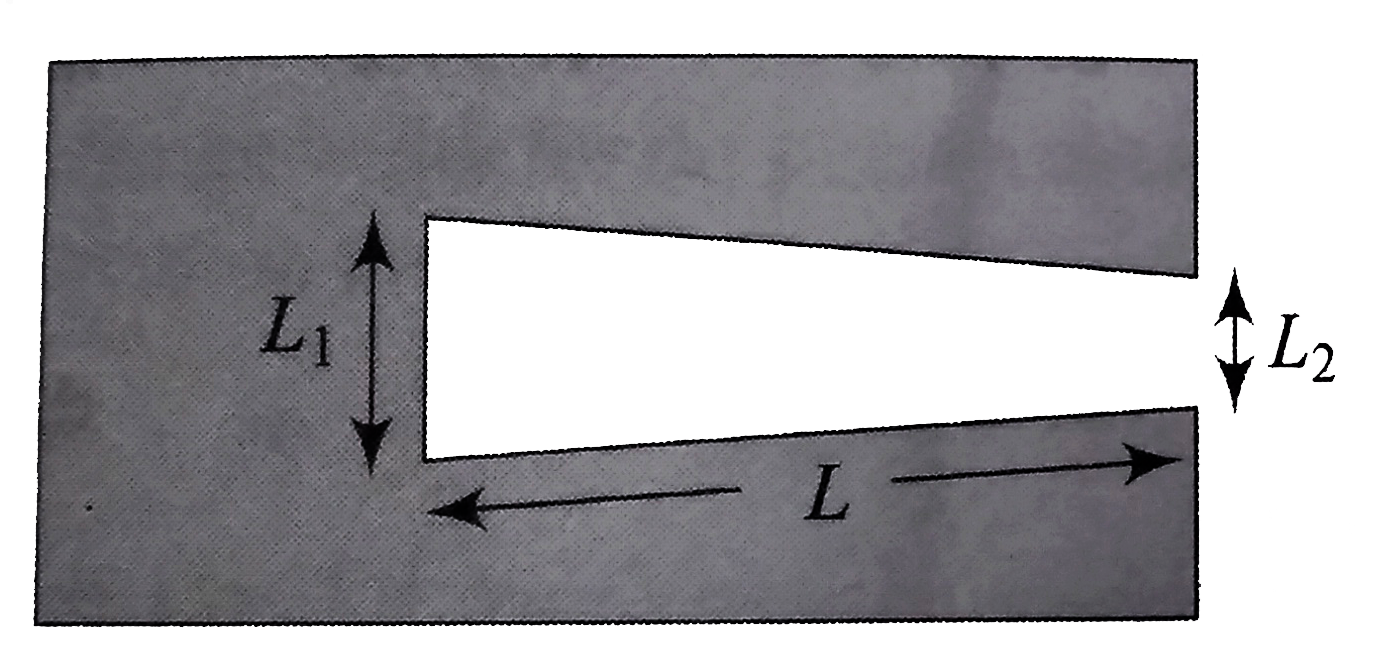
A lens of focal length f is cut along the diameter into two identical halevs. In this process, a layer of the lens t in thickness is lost, then the halves are put together to form a composite lens. In between the focal plane and the composite lens, a narrow slit is placed near the focal plane. The slit is emitting monochromatic λ. Behind the lens, a screen is located at a distance L front it.
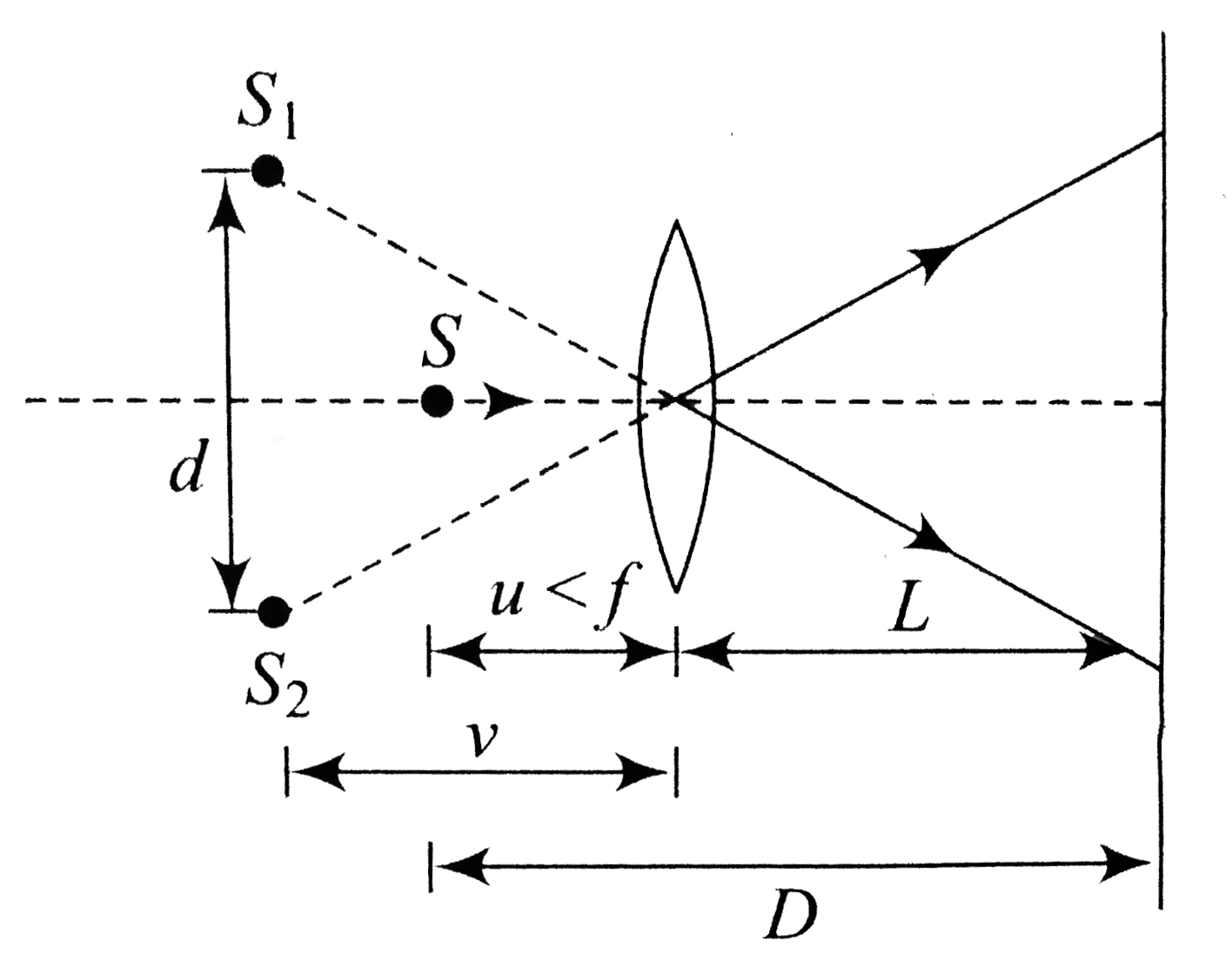
Find the fringe width for the pattern obtained under given arrangement on the screen.View SolutionQuestion 228
A lens of focal length f is cut along the diameter into two identical halevs. In this process, a layer of the lens t in thickness is lost, then the halves are put together to form a composite lens. In between the focal plane and the composite lens, a narrow slit is placed near the focal plane. The slit is emitting monochromatic λ. Behind the lens, a screen is located at a distance L front it.
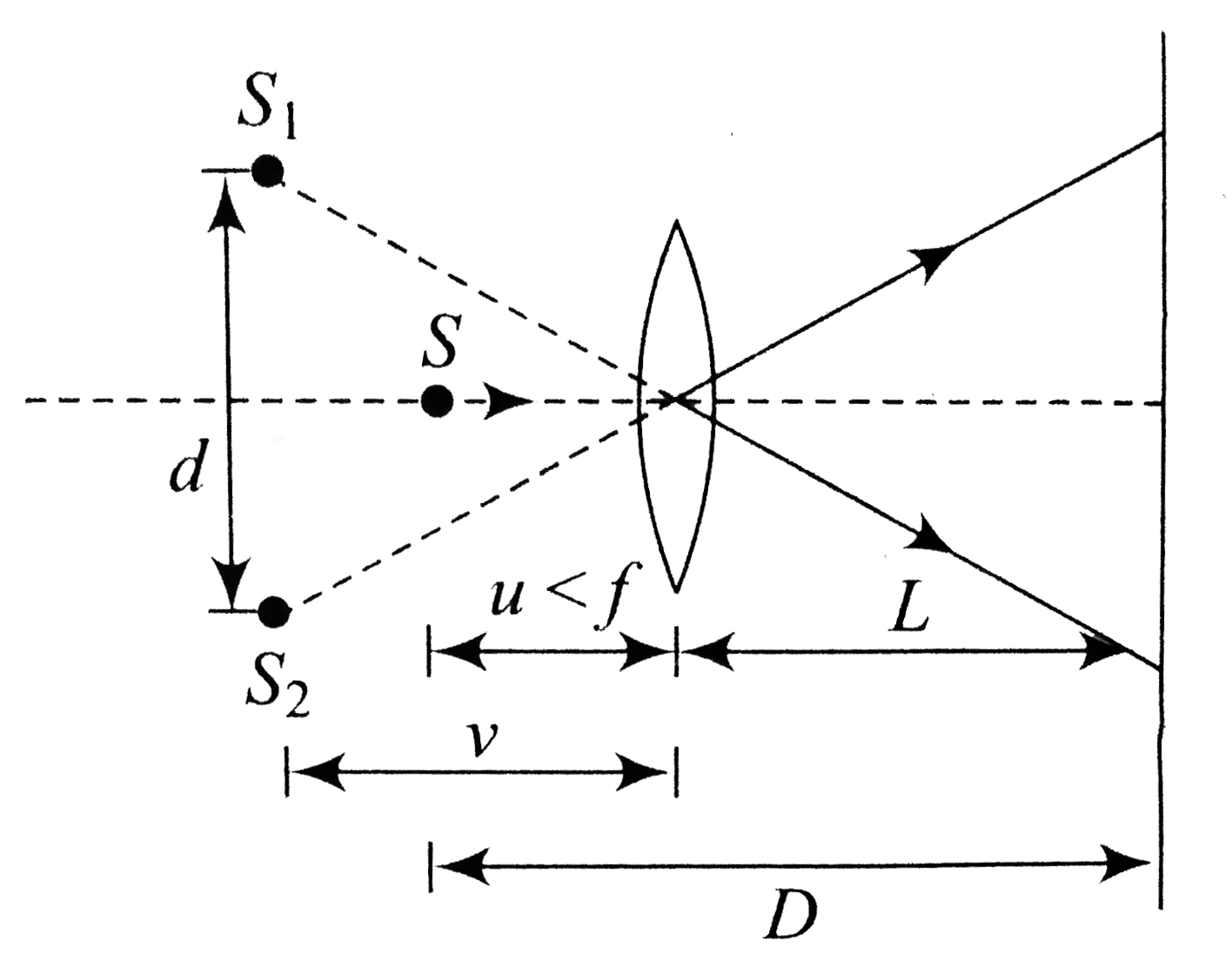
The expression for the number of visible maxima which are obtained through above said arrangement will turn out to beView SolutionQuestion 232
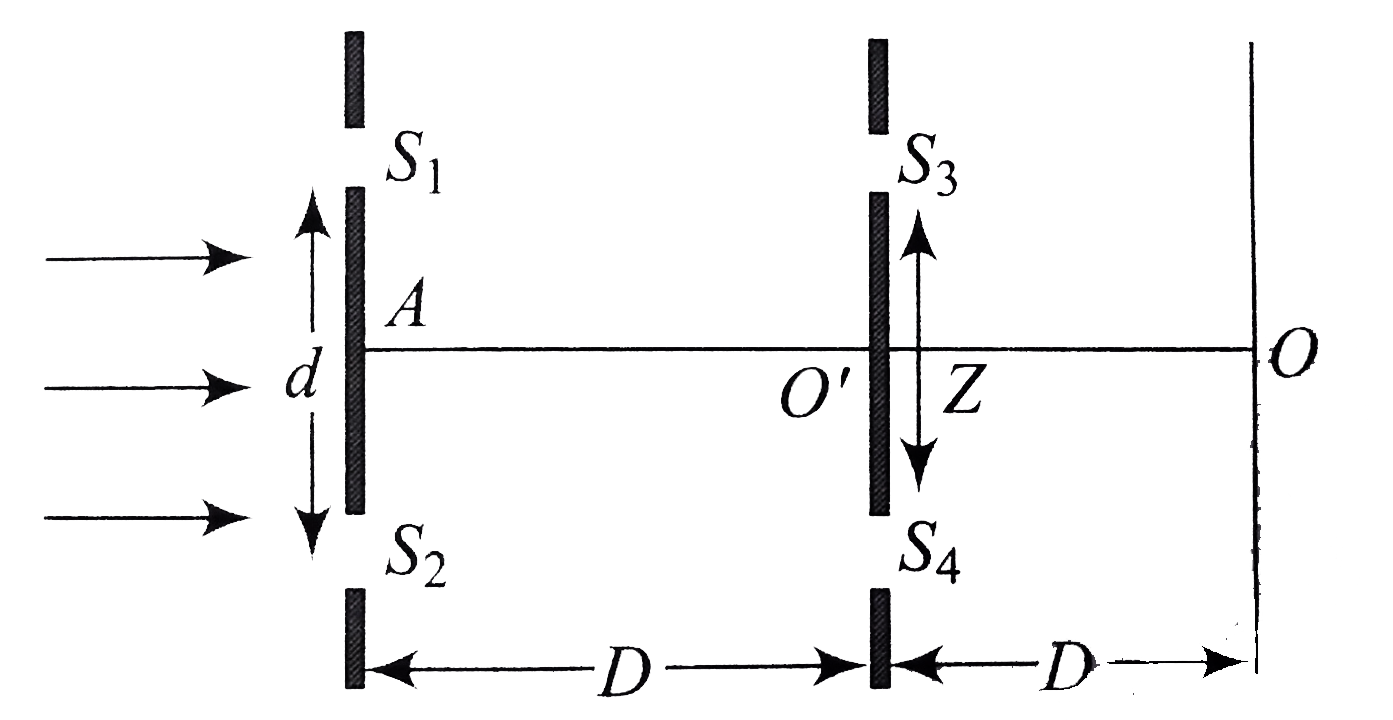
Consider the situation shown in fig. The two slits S1 and S2 placed symmetrically around the central line are illuminated by monochromatic light of wavelength λ. The separation between the slit is d. The ligth transmitted by the slits falls on a screen S0 placed at a distance D form the slits. The slit S3 is at the central line and the slit S4 is at a distance z from S3 Another screen Sc is placed a further distance D away from Sc Find the ratio of the maximum to minimum intensity observed on Sc
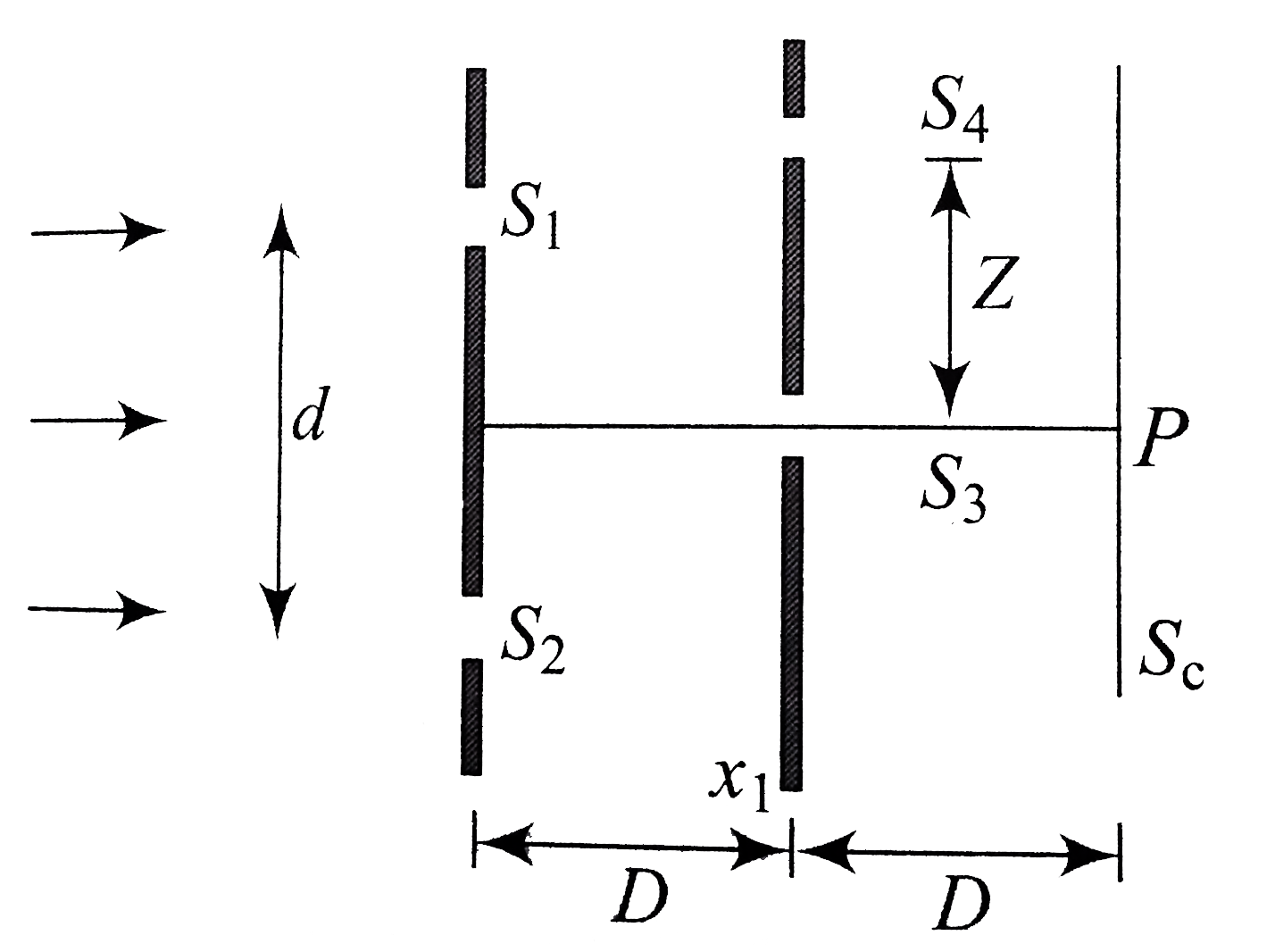
If z=λD2dView SolutionQuestion 233
Consider the situation shown in fig. The two slits S1 and S2 placed symmetrically around the central line are illuminated by monochromatic light of wavelength λ. The separation between the slit is d. The ligth transmitted by the slits falls on a screen S0 placed at a distance D form the slits. The slit S3 is at the central line and the slit S4 is at a distance z from S3 Another screen Sc is placed a further distance D away from Sc Find the ratio of the maximum to minimum intensity observed on Sc
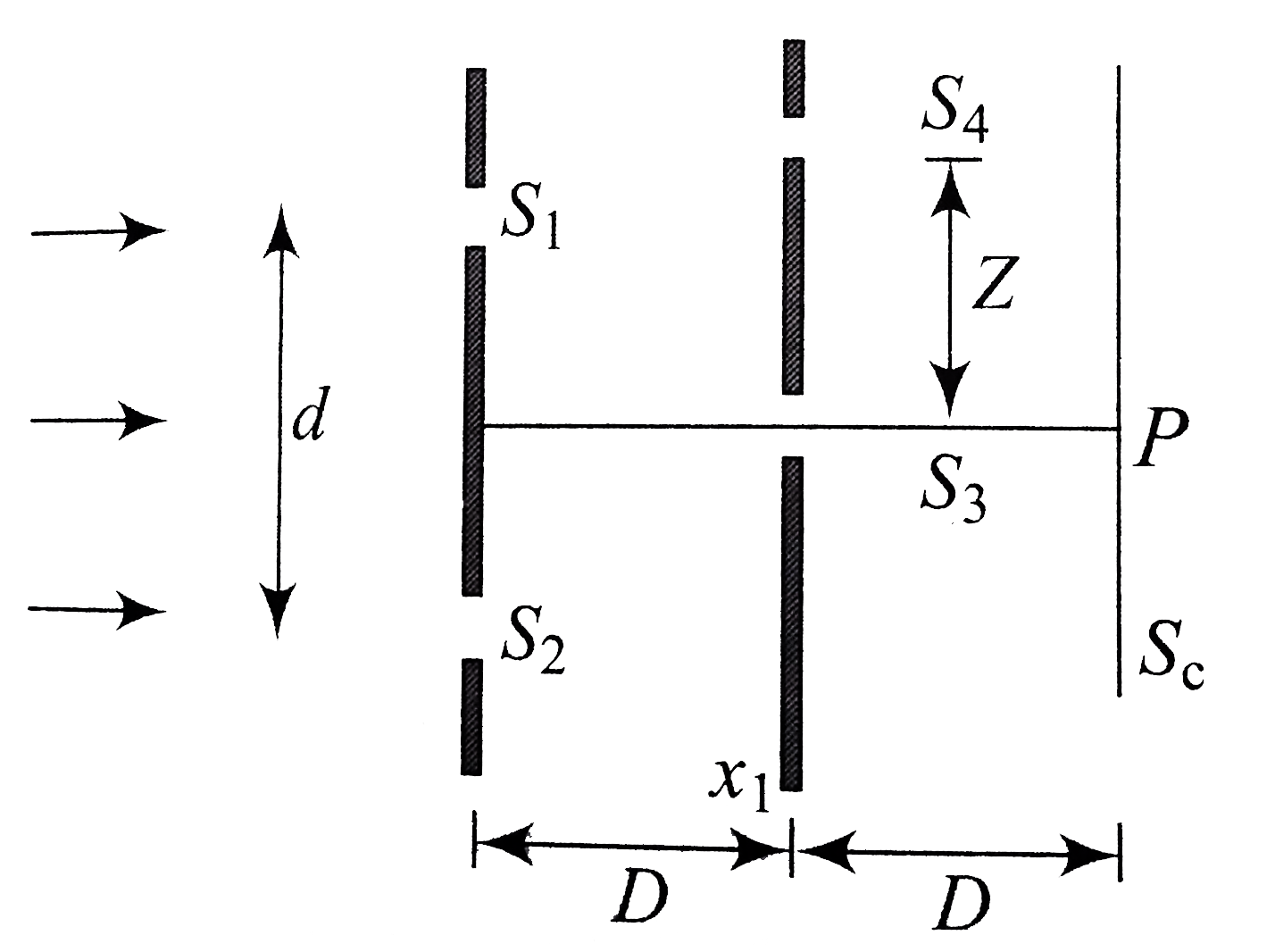
If z=λDdView SolutionQuestion 234
Consider the situation shown in fig. The two slits S1 and S2 placed symmetrically around the central line are illuminated by monochromatic light of wavelength λ. The separation between the slit is d. The ligth transmitted by the slits falls on a screen S0 placed at a distance D form the slits. The slit S3 is at the central line and the slit S4 is at a distance z from S3 Another screen Sc is placed a further distance D away from Sc Find the ratio of the maximum to minimum intensity observed on Sc
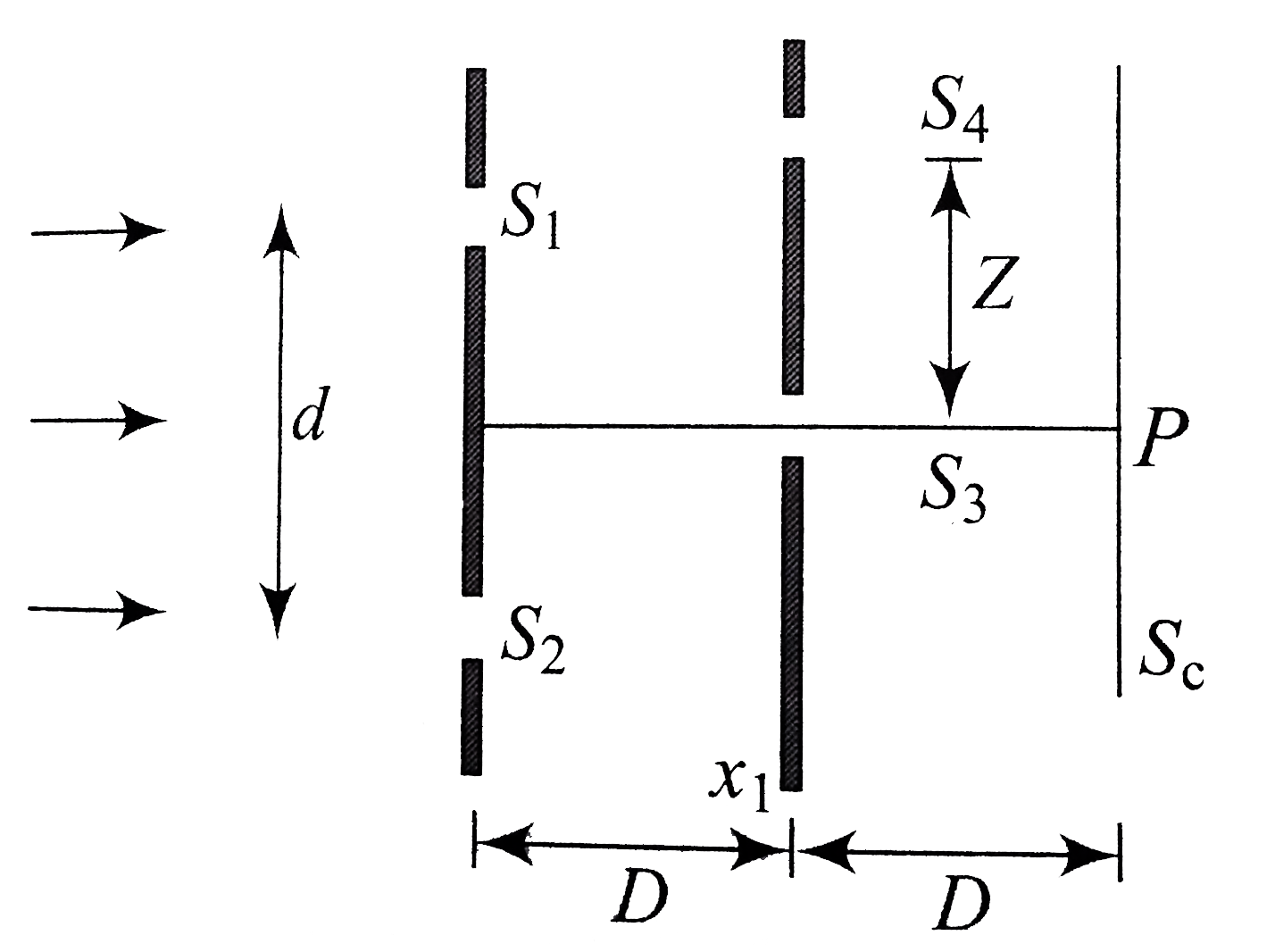
If z=λD4dView SolutionQuestion 238
Young's double-slit experiment setup with ligth of wavelength λ=6000Å, distance between two slit in 2 mm and distance between the plane of slits and the screen. Is 2 m. The slits are of equal intensity. When a sheet of glass of refractive index 1.5 (which permtis only a fraction η of the incident light to pass through) and thickness 8000Å is placed in front of the lower slit, it is observed that the intensity at a point P, 0.15 mm above the central maxima, does not change.
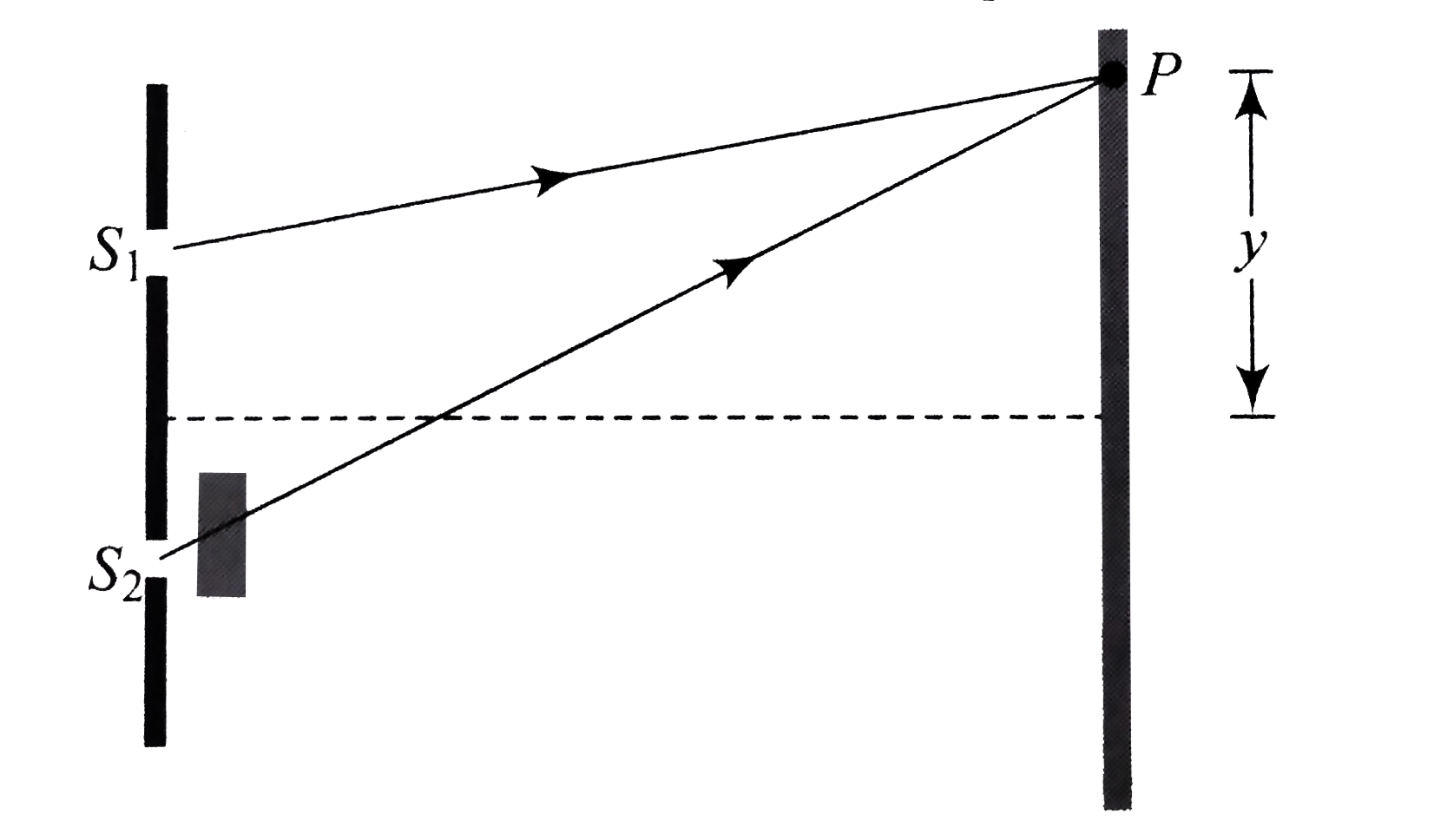
The phase difference at point P without inserting the slab isView SolutionQuestion 239
Young's double-slit experiment setup with ligth of wavelength λ=6000Å, distance between two slit in 2 mm and distance between the plane of slits and the screen. Is 2 m. The slits are of equal intensity. When a sheet of glass of refractive index 1.5 (which permtis only a fraction η of the incident light to pass through) and thickness 8000Å is placed in front of the lower slit, it is observed that the intensity at a point P, 0.15 mm above the central maxima, does not change.
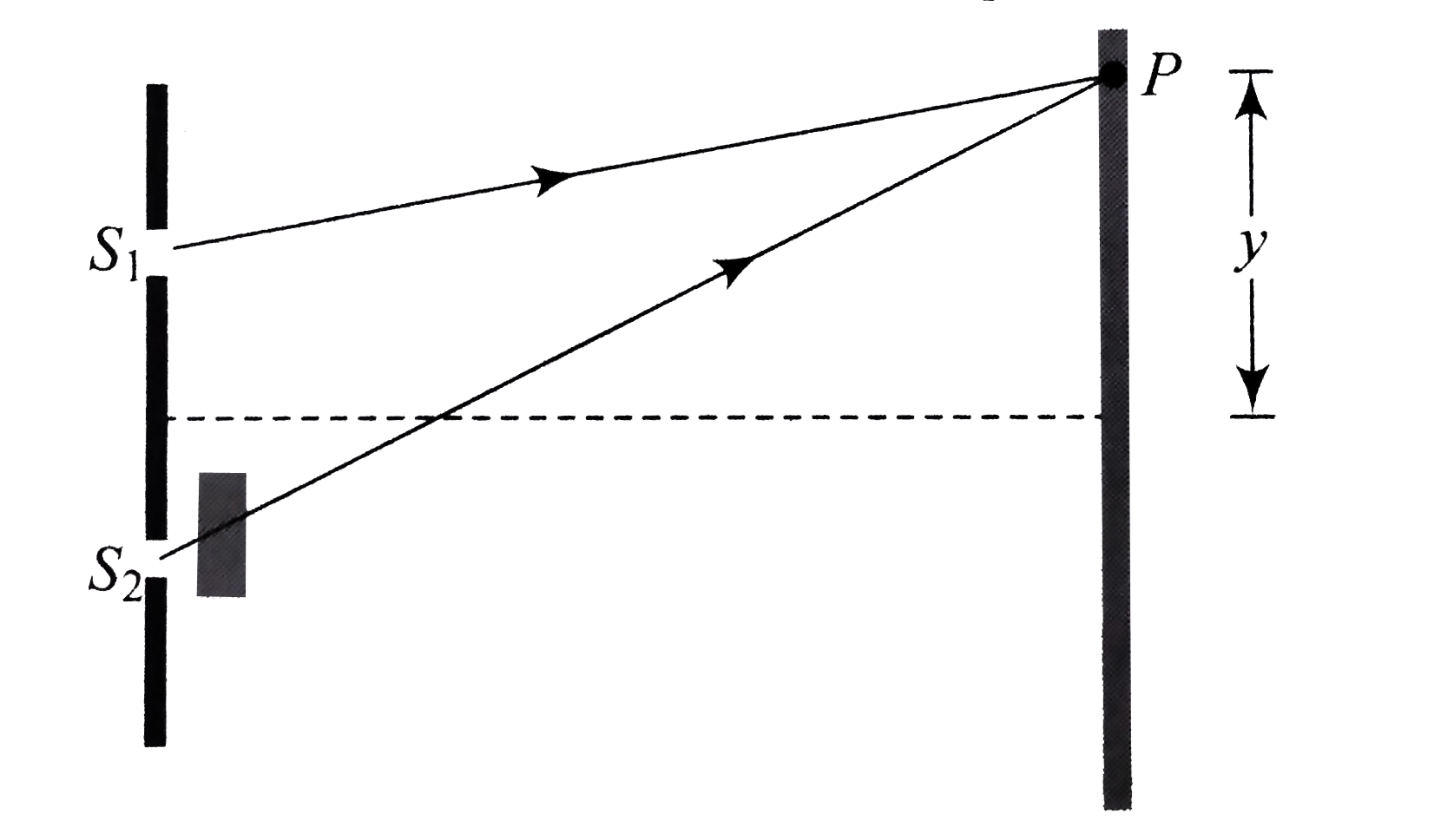
Intensity at point P isView SolutionQuestion 240
Young's double-slit experiment setup with ligth of wavelength λ=6000Å, distance between two slit in 2 mm and distance between the plane of slits and the screen. Is 2 m. The slits are of equal intensity. When a sheet of glass of refractive index 1.5 (which permtis only a fraction η of the incident light to pass through) and thickness 8000Å is placed in front of the lower slit, it is observed that the intensity at a point P, 0.15 mm above the central maxima, does not change.
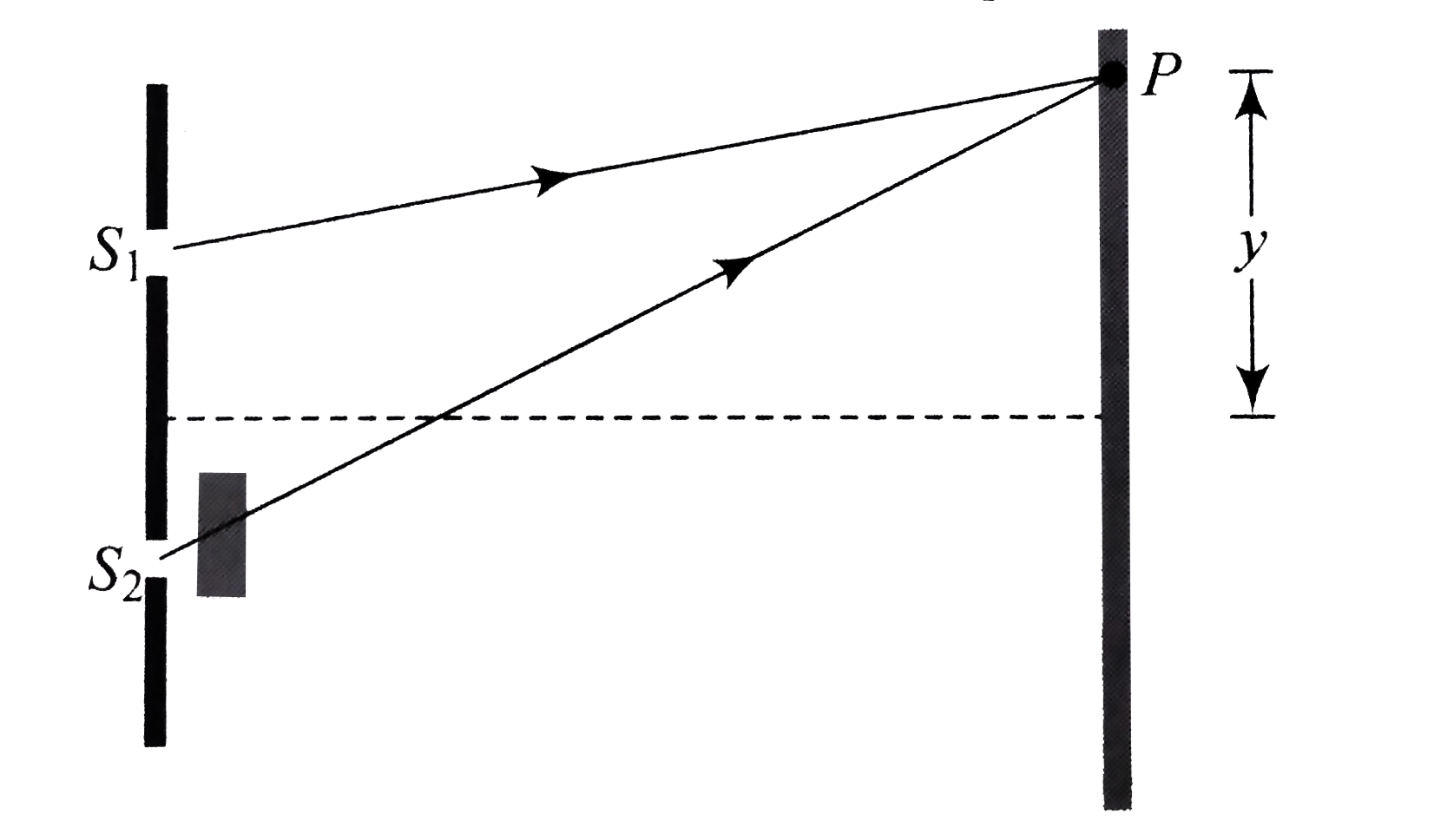
The value of η isView SolutionQuestion 241
An interference is observed due to two coherent sources S1 placed at origin and S2 placed at (0, 3l, 0). Here, λ is the wavelength of the sources. Both sources are having equal intensity I0. A detector D is moved along the positive x-axix.
Total number of black points observed by the observer on positive x-axis isView SolutionQuestion 242
An interference is observed due to two coherent sources S1 placed at origin and S2 placed at (0, 3l, 0). Here, λ is the wavelength of the sources. Both sources are having equal intensity I0. A detector D is moved along the positive x-axix.
Find x-coordinates on the x-axis (exchange x=0 and x=OO).View SolutionQuestion 246
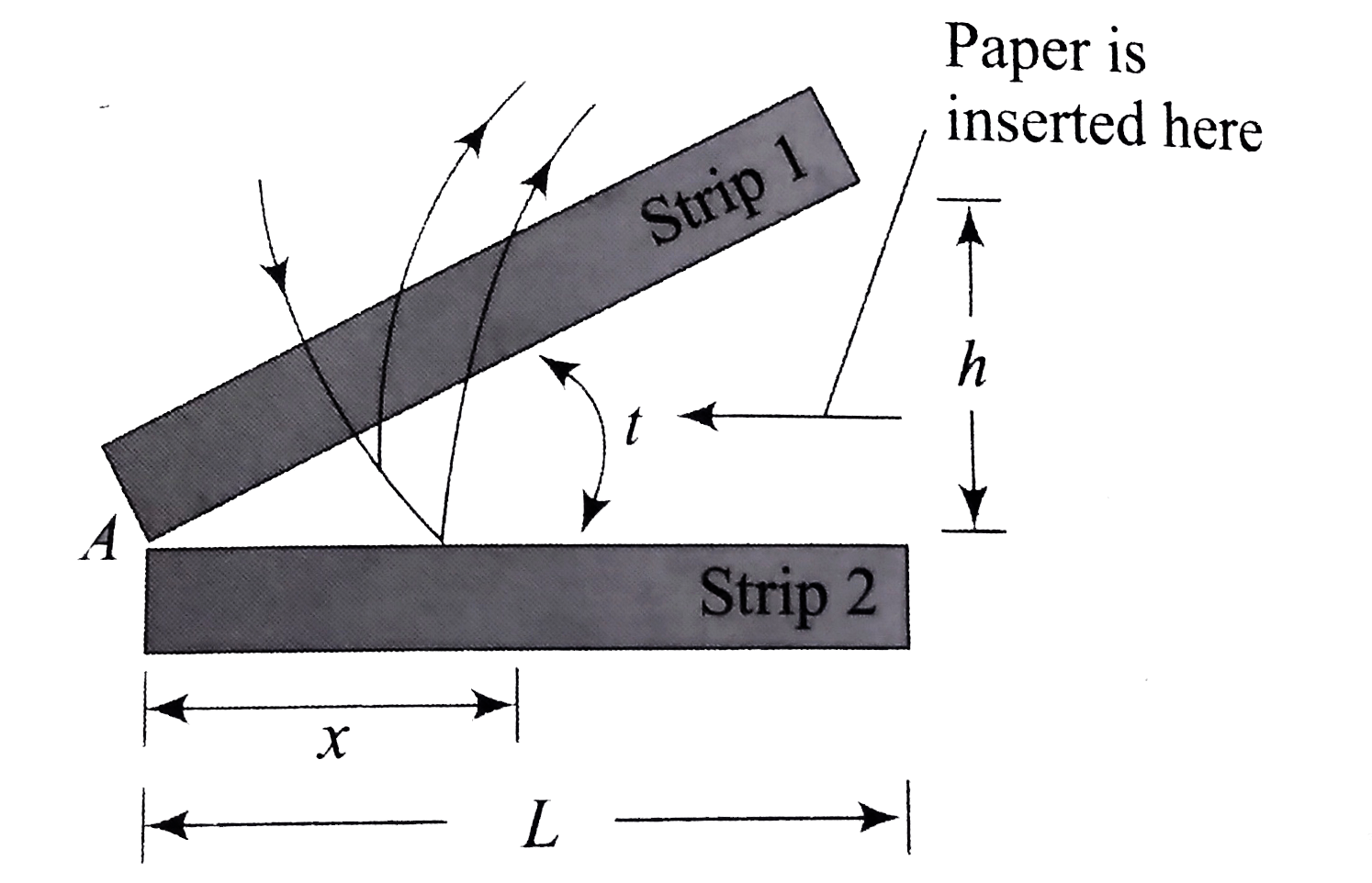
This interference film is used to measure the thickness of slides, paper, etc. The arrangement is as shown in fig. For the sake of clarity, the two strips are shown thick. Consider the wedge formed in between strips 1 and 2. If the interference pattern because of the two waves reflected from wedge surface is observed, then from the observed data we can compute thickness of paper, refractive index of the medium filled in wedge, number of bonds formed, etc.
Considre the strips to be thick as compared to wavelength of ligth and light is incident normally.
Neglect the effect due to reflection from top surface of strip 1 and bottom surface of strip 2. Take L=5cm and λair=40nm.
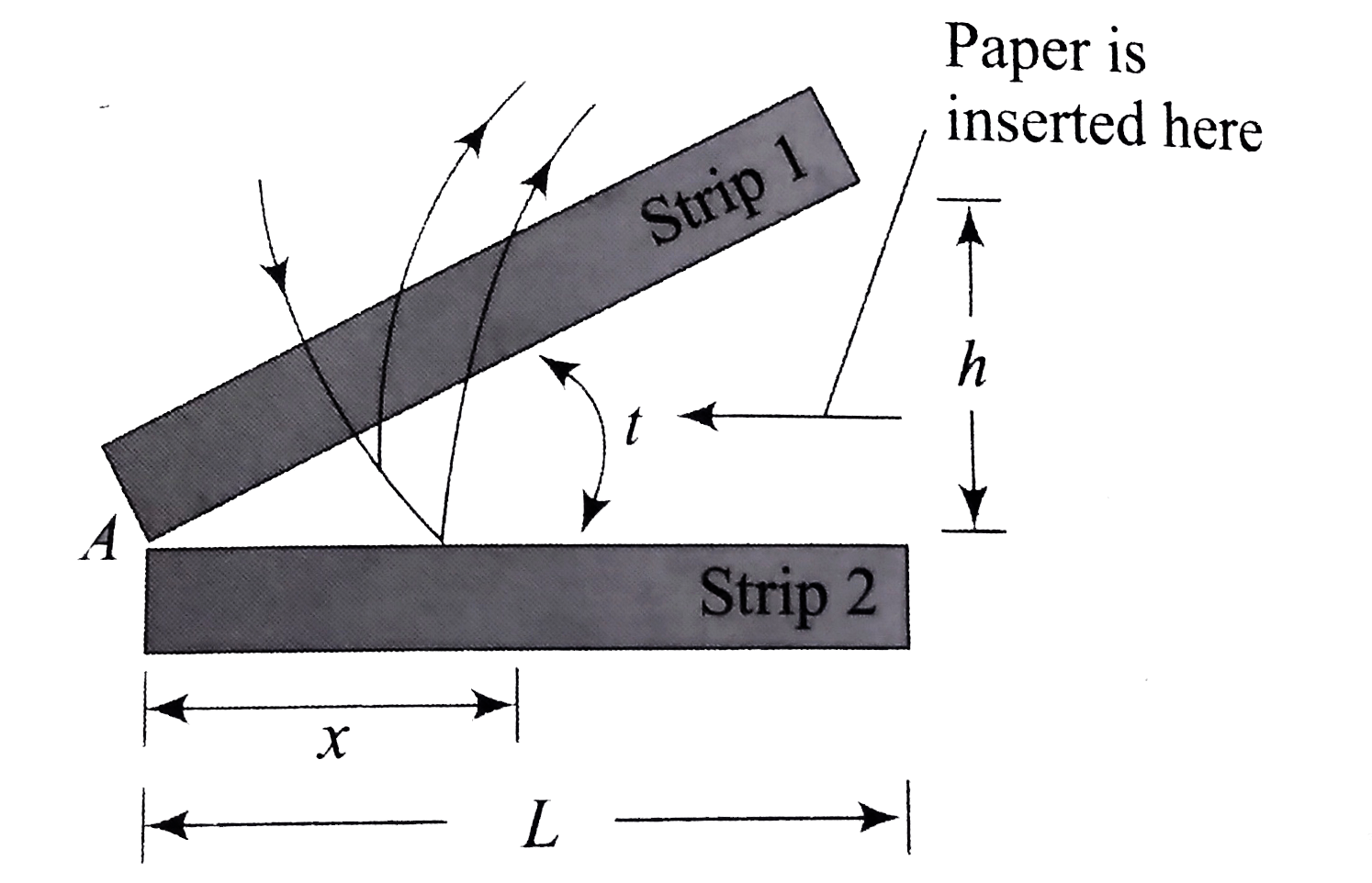
Consider an air wedge formed by two glass plates. having refractive index 1.5 by placing a piece of paper of thickness 20 mm. Determine the number of dark bands formed.View SolutionQuestion 247
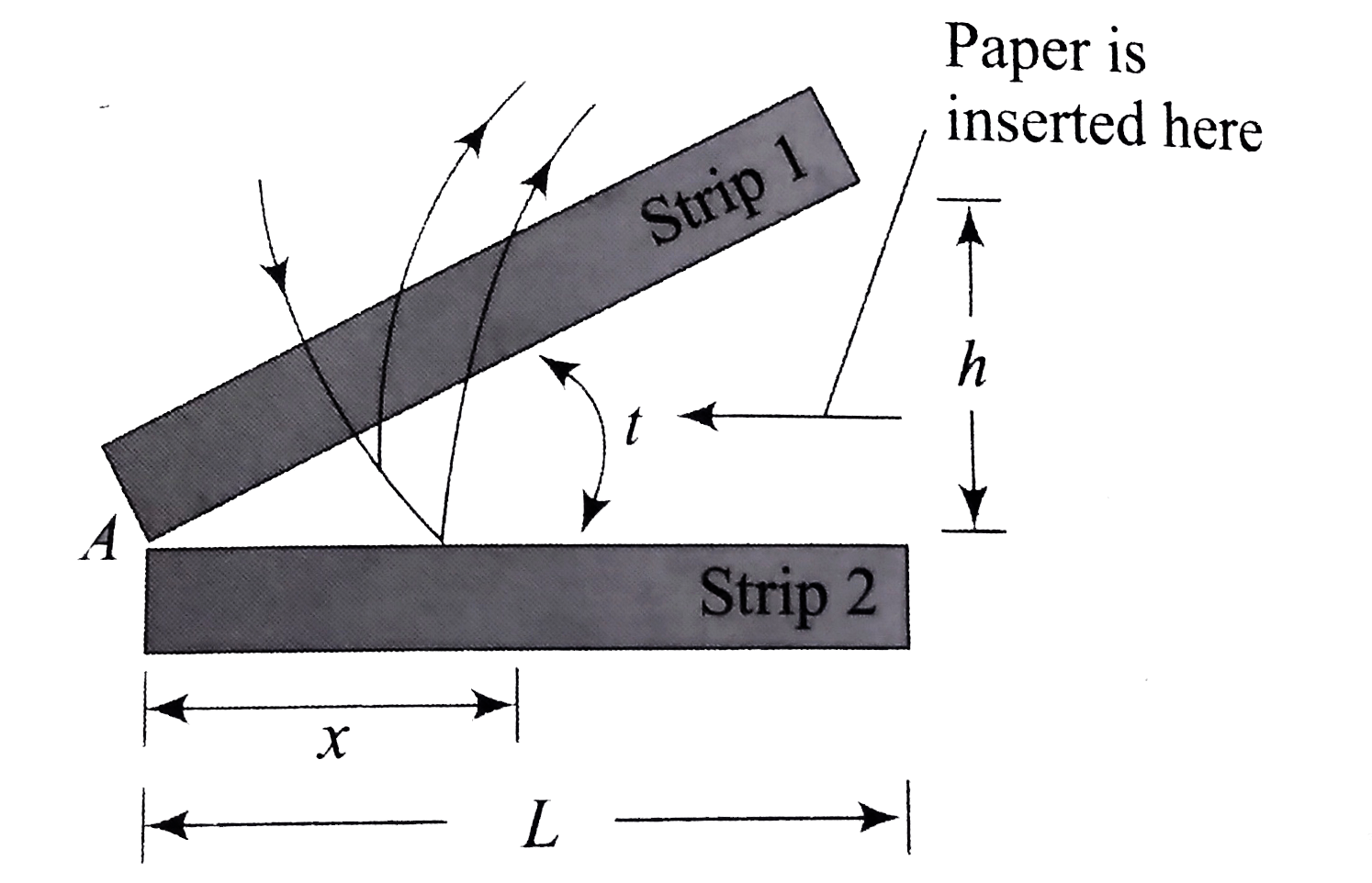
This interference film is used to measure the thickness of slides, paper, etc. The arrangement is as shown in fig. For the sake of clarity, the two strips are shown thick. Consider the wedge formed in between strips 1 and 2. If the interference pattern because of the two waves reflected from wedge surface is observed, then from the observed data we can compute thickness of paper, refractive index of the medium filled in wedge, number of bonds formed, etc.
Considre the strips to be thick as compared to wavelength of ligth and light is incident normally.
Neglect the effect due to reflection from top surface of strip 1 and bottom surface of strip 2. Take L=5cm and λair=40nm.
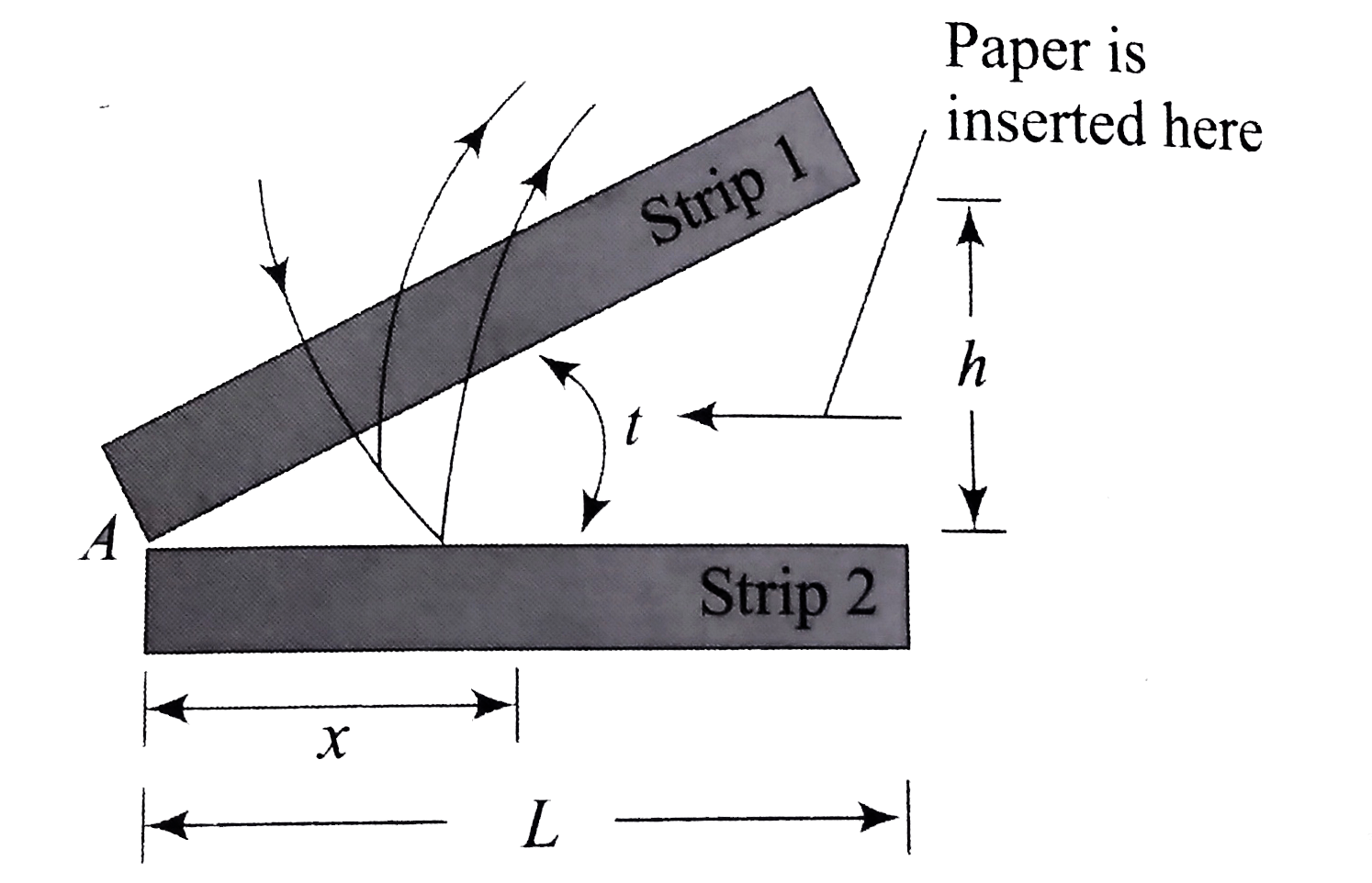
For strip 1 refractive index is 1.34 abd for strip 2 refractive index is 1.6 The wedge. is filled with a medium having refractive index 1.5. ThenView SolutionQuestion 248
This interference film is used to measure the thickness of slides, paper, etc. The arrangement is as shown in fig. For the sake of clarity, the two strips are shown thick. Consider the wedge formed in between strips 1 and 2. If the interference pattern because of the two waves reflected from wedge surface is observed, then from the observed data we can compute thickness of paper, refractive index of the medium filled in wedge, number of bonds formed, etc.
Considre the strips to be thick as compared to wavelength of ligth and light is incident normally.
Neglect the effect due to reflection from top surface of strip 1 and bottom surface of strip 2. Take L=5cm and λair=40nm.
In question 53, if air wedge has been filled with a medium having refractive index 1.3 then find the number of bright bands.View SolutionQuestion 249
This interference film is used to measure the thickness of slides, paper, etc. The arrangement is as shown in fig. For the sake of clarity, the two strips are shown thick. Consider the wedge formed in between strips 1 and 2. If the interference pattern because of the two waves reflected from wedge surface is observed, then from the observed data we can compute thickness of paper, refractive index of the medium filled in wedge, number of bonds formed, etc.
Considre the strips to be thick as compared to wavelength of ligth and light is incident normally.
Neglect the effect due to reflection from top surface of strip 1 and bottom surface of strip 2. Take L=5cm and λair=40nm.
For data in question53 determine the distance of point B from the 20th dark band. Counting of dark point has to start from the contact point.View SolutionQuestion 250
A block o plastic having a thin air cavity (whose thickenss is comparable to wavelength of light waves) is shown in fig. The thickness of air cavity (which can be considered as air wedge for interference pattern) is varying linearly from one end to other as shown.
A broad beam of monochromatic light is incident normally from the top of the plastic box. Some ligth lis reflected back above and below the cavity .The plastic layers than wavelength of incident light . An observer when looking down from top sees an interference pattern consisting of eight dark fringes and seven bright fringe along the wedge. Take wavelength of incident light in air as λ0 and refractive index of plastic as μ
Assume that the thickness of the ends of air cavity are such that formation of fringe takes place there.
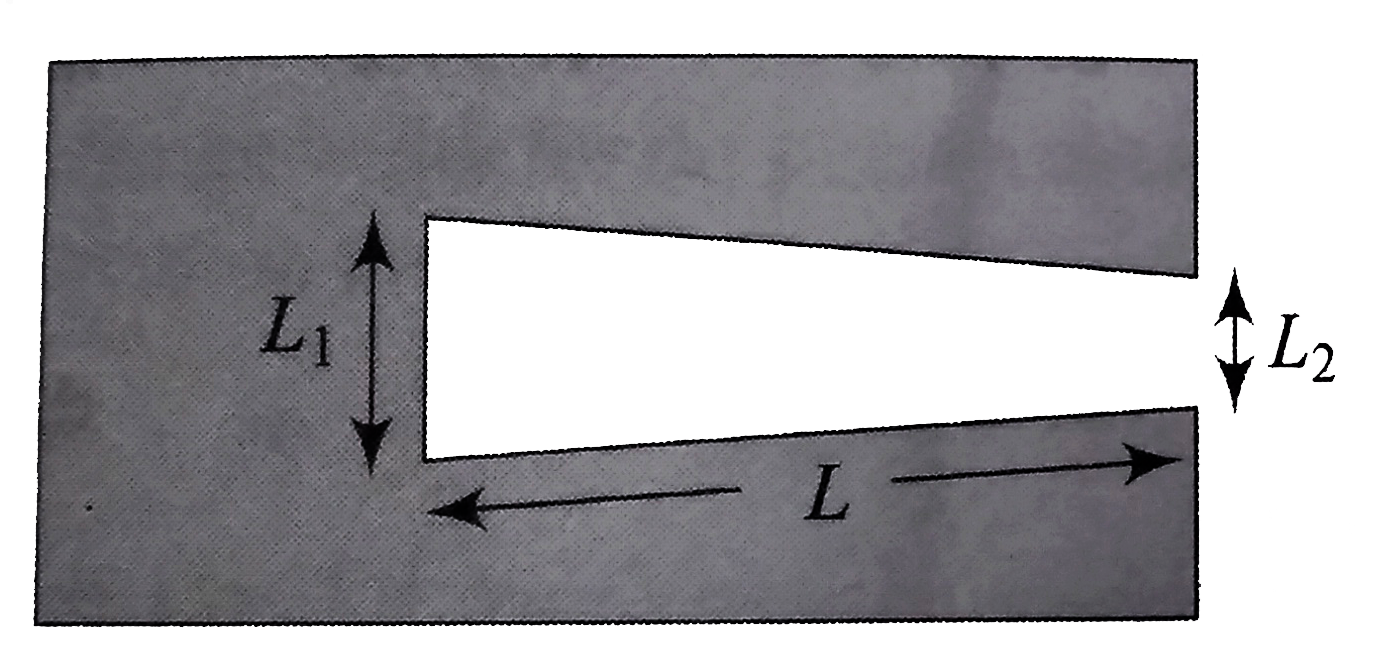
Determine of difference L1−L2=ΔL) in terms of λ0.View SolutionQuestion 251
A block o plastic having a thin air cavity (whose thickenss is comparable to wavelength of light waves) is shown in fig. The thickness of air cavity (which can be considered as air wedge for interference pattern) is varying linearly from one end to other as shown.
A broad beam of monochromatic light is incident normally from the top of the plastic box. Some ligth lis reflected back above and below the cavity .The plastic layers than wavelength of incident light . An observer when looking down from top sees an interference pattern consisting of eight dark fringes and seven bright fringe along the wedge. Take wavelength of incident light in air as λ0 and refractive index of plastic as μ
Assume that the thickness of the ends of air cavity are such that formation of fringe takes place there.
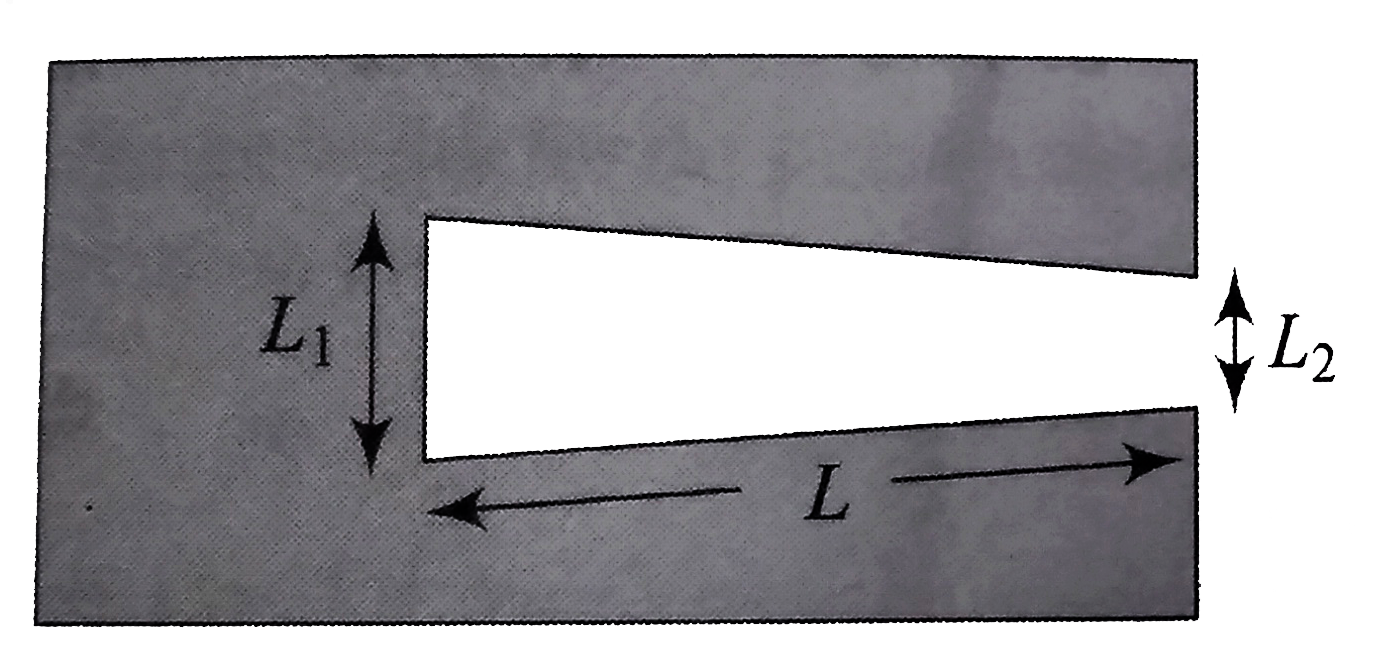
Determine the distance of 4th dark fringe from the left end of air cavity.View SolutionQuestion 252
A block o plastic having a thin air cavity (whose thickenss is comparable to wavelength of light waves) is shown in fig. The thickness of air cavity (which can be considered as air wedge for interference pattern) is varying linearly from one end to other as shown.
A broad beam of monochromatic light is incident normally from the top of the plastic box. Some ligth lis reflected back above and below the cavity .The plastic layers than wavelength of incident light . An observer when looking down from top sees an interference pattern consisting of eight dark fringes and seven bright fringe along the wedge. Take wavelength of incident light in air as λ0 and refractive index of plastic as μ
Assume that the thickness of the ends of air cavity are such that formation of fringe takes place there.
Determine the separation between 1st and 2nd dark fringes form the left end of air cavityView SolutionQuestion 253
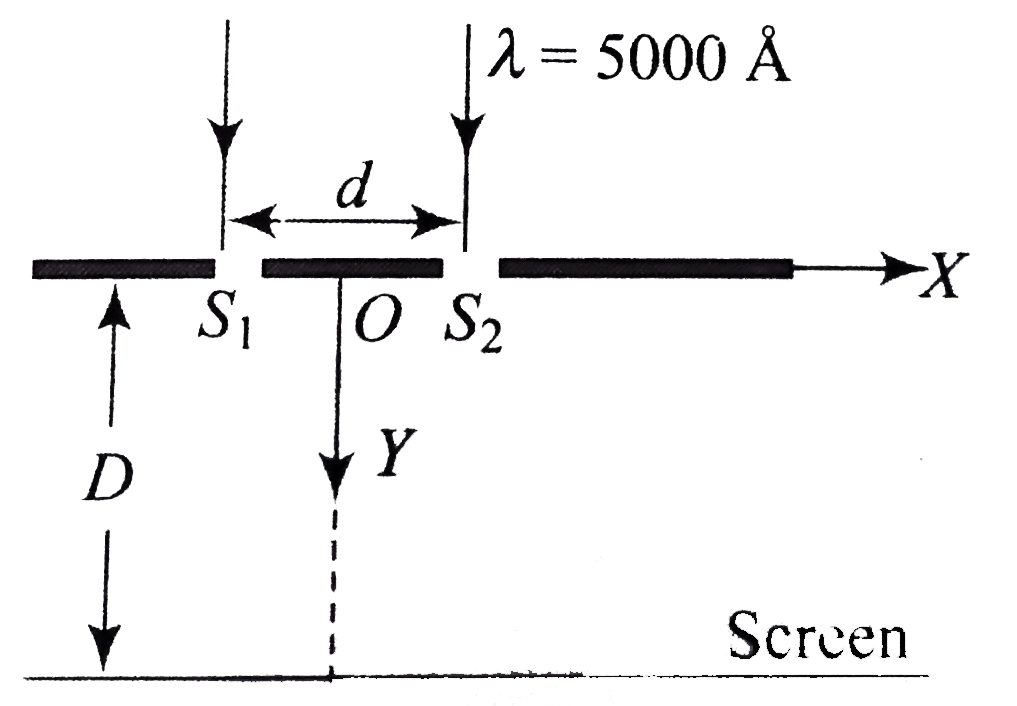
In fig., light of wavelength λ=5000Å is incident on the slits (in a horizontally fixed place).
Here, d=1mm and D=1m
Take origin at O and XY plane as shown in the figure. The screen is released from rest from the initial position as shown
The velocity of central maxima at t=5s isView SolutionQuestion 254
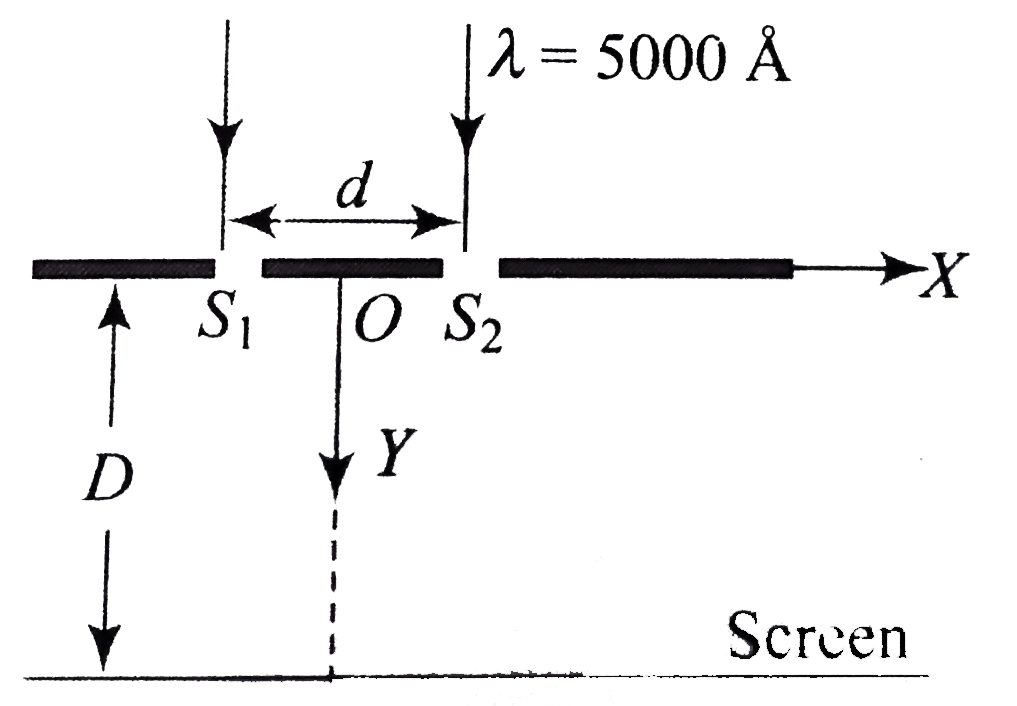
In fig., light of wavelength λ=5000Å is incident on the slits (in a horizontally fixed place).
Here, d=1mm and D=1m
Take origin at O and XY plane as shown in the figure. The screen is released from rest from the initial position as shown
Velocity of 2nd maixma w.r.t central maxima at t=2s isView SolutionQuestion 255
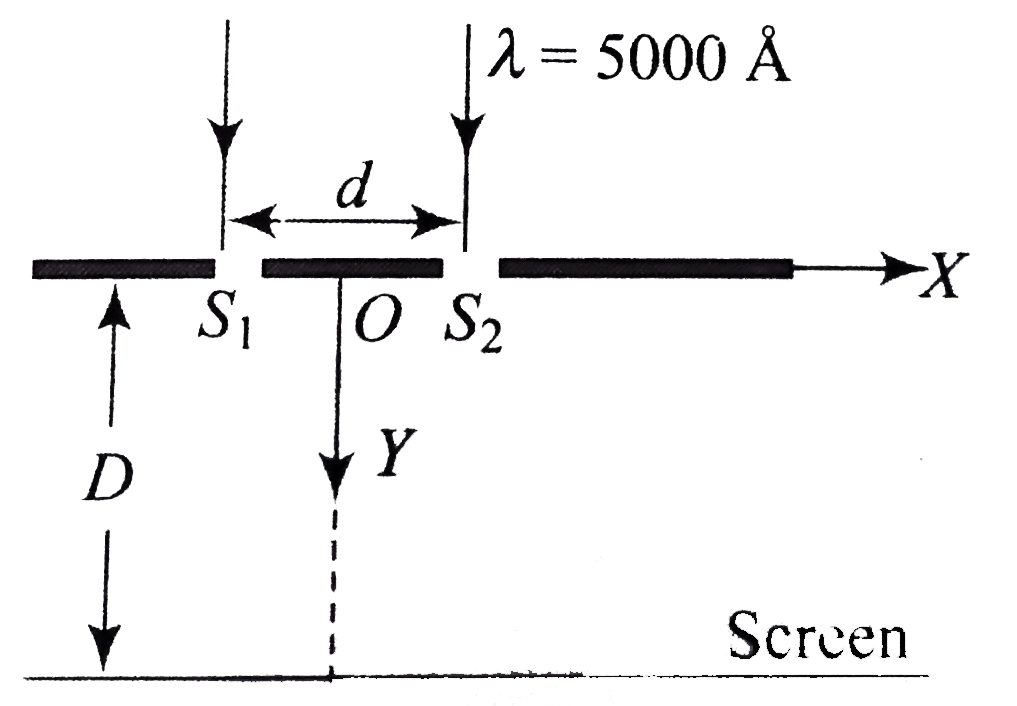
In fig., light of wavelength λ=5000Å is incident on the slits (in a horizontally fixed place).
Here, d=1mm and D=1m
Take origin at O and XY plane as shown in the figure. The screen is released from rest from the initial position as shown
Acceleration of 3rd maxima w.r.t. 3rd maxiam on other side of central maxima at t=3 s isView SolutionQuestion 256
When two coherent sources interact with each other, there will be production of alternate bright and dark fringes on the screen . Young's double-slit experiment domonstrates the idea of making two coherent sources. For better visibility, one has to choose proper amplitude for the sources. The phenomena is good enough to satisfy the conservation of energy principle. The pattern formed in YDSE is of uniform thickness and is nicely placed on a long distance screen.
Law of convervation of energy is satisfied becauseView SolutionQuestion 257
When two coherent sources interact with each other, there will be production of alternate bright and dark fringes on the screen . Young's double-slit experiment domonstrates the idea of making two coherent sources. For better visibility, one has to choose proper amplitude for the sources. The phenomena is good enough to satisfy the conservation of energy principle. The pattern formed in YDSE is of uniform thickness and is nicely placed on a long distance screen.
For better visibility of fringe pattern View SolutionQuestion 258
When two coherent sources interact with each other, there will be production of alternate bright and dark fringes on the screen . Young's double-slit experiment domonstrates the idea of making two coherent sources. For better visibility, one has to choose proper amplitude for the sources. The phenomena is good enough to satisfy the conservation of energy principle. The pattern formed in YDSE is of uniform thickness and is nicely placed on a long distance screen.
The best combination of independent sources to produce sustained pattern among the following is
Y1=asinωt Y2=acosωt
Y3=asin(ωt+π4) Y4=2asin(ωt+π)View SolutionQuestion 259
When a light wave passes from a rarer medium to a denser medium, there will be a phase change of π radians. This difference brings change in the conditions for constructive and destructive interference. This phenomena also reasons the fromation of interference pattern in thin films like, oily layer, soap film, etc., but has no reason on the shifting of fringes from the central portion outward. The shift is dependent on the refractive index of the material as per the relation, Δy=(μ−1)t
Thin film interference happens with View SolutionQuestion 260
When a light wave passes from a rarer medium to a denser medium, there will be a phase change of π radians. This difference brings change in the conditions for constructive and destructive interference. This phenomena also reasons the fromation of interference pattern in thin films like, oily layer, soap film, etc., but has no reason on the shifting of fringes from the central portion outward. The shift is dependent on the refractive index of the material as per the relation, Δy=(μ−1)t
On introducing a transparent slab (μ) the central fringe shifts to the point originally occupied by the fifth bright fringe. The thickness of the slab isView SolutionQuestion 261
When a light wave passes from a rarer medium to a denser medium, there will be a phase change of π radians. This difference brings change in the conditions for constructive and destructive interference. This phenomena also reasons the fromation of interference pattern in thin films like, oily layer, soap film, etc., but has no reason on the shifting of fringes from the central portion outward. The shift is dependent on the refractive index of the material as per the relation, Δy=(μ−1)t
The condition for constructive interference in Lloyd's single mirror experiment is the path difference which is equal toView SolutionQuestion 262
When light from two sources (say slits S1 and S2) interfere, they form alternate dark and bright fringes. Bright fringe is formed at all point where the path difference is an odd multiple of half wavelength. At the condition of equal amplitudes, A1=A2=a, the maximum intensity will be 4a2 and the visibility improves, The resultant intensity can also be indicated with phase factor as I=2a2cos2(ϕ/2).Using this passage, answer the following questions.
If the path difference between the slits S1 and S2 is λ2 the central fringe will have an intensity ofView SolutionQuestion 263
When light from two sources (say slits S1 and S2) interfere, they form alternate dark and bright fringes. Bright fringe is formed at all point where the path difference is an odd multiple of half wavelength. At the condition of equal amplitudes, A1=A2=a, the maximum intensity will be 4a2 and the visibility improves, The resultant intensity can also be indicated with phase factor as I=2a2cos2(ϕ/2).Using this passage, answer the following questions.
At point having a path difference of λ4, the intensityView SolutionQuestion 264
When light from two sources (say slits S1 and S2) interfere, they form alternate dark and bright fringes. Bright fringe is formed at all point where the path difference is an odd multiple of half wavelength. At the condition of equal amplitudes, A1=A2=a, the maximum intensity will be 4a2 and the visibility improves, The resultant intensity can also be indicated with phase factor as I=2a2cos2(ϕ/2).Using this passage, answer the following questions.
If the slits S1 and S2 are arranged as shown in Fig. the ratio of internsity of fringe at P and R is
 View Solution
View SolutionQuestion 265
Thin films, including soap bubbles and oil show patterns of alternative dark and bright regions resulting from interference among the reflected ligth waves. If two waves are in phase, their crests and troughs will coincide. The interference will be cosntructive and the amlitude of resultant wave will be greater then either of constituent waves. If the two wave are not of phase by half a wavelength (180∘), the crests of one wave will coincide width the troughs of the other wave. The interference will be destructive and the ampliutde of the resultant wave will be less than that of either consituent wave.
1. When incident light I, reaches the surface at point a, some of the ligth is reflected as ray Ra and some is refracted following the path ab to the back of the film.
2. At point b, some of the light is refracted out of the film and part is reflected back through the film along path bc. At point c, some of the light is reflected back into the film and part is reflected out of the film as ray Rc.
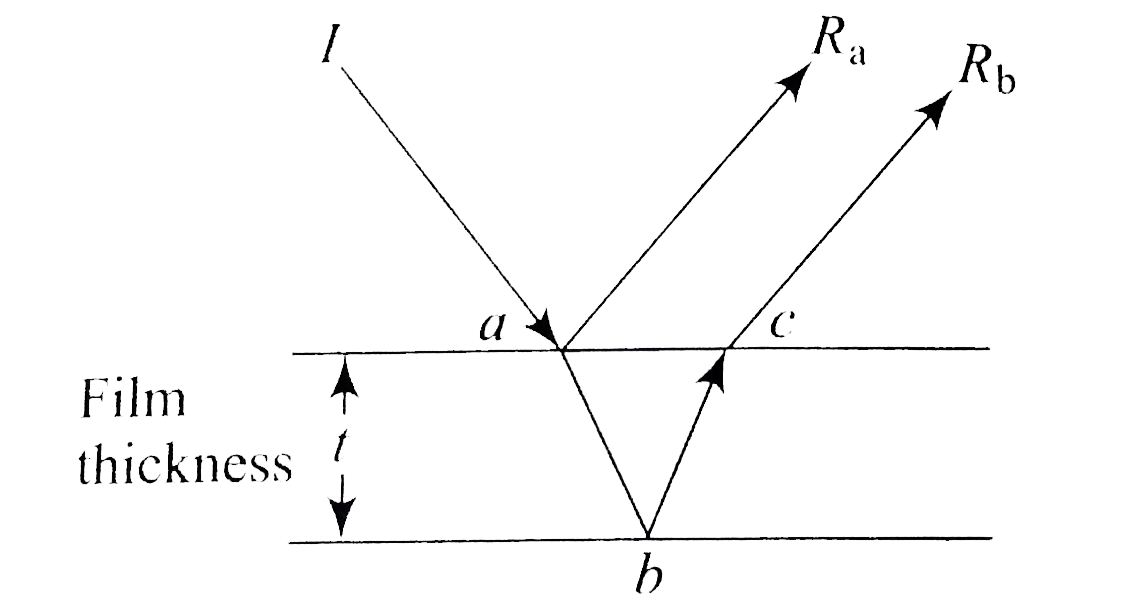
Ra and Rc are parallel. However, Rc has travelled the extra distance within the film fo abc. If the angle of incidence is small, then abc is approxmately twice the film's thickness .
If Ra and Rc are in phase, they will undergo constructive interference and the region ac will be bright. If Ra and Rc are out of phase, they will undergo destructive interference and the region ac will be dark.
I. Refraction at an interface never changes the phase of the wave.
II. For reflection at the interfere between two media 1 and 2, if n1>n2, the reflected wave will change phase. If n1<n2, the reflected wave will not undergo a phase change.
For reference, nair=1.00.

III. If the waves are in phase after reflection at all intensities, then the effects of path length in the film are:
Constrictive interference occurs when 2t=mλ/n,m=0,1,2,3,...
Destrcutive interference occurs when 2t=(m+12)λn,
m=0,1,2,3,...
If the waves are 180∘ out of the phase after reflection at all interference, then the effects of path length in the film ara:
Constructive interference occurs when
2t=(m+12)λn,m=0,1,2,3,...
Destructive interference occurs when
2t=mλn,m=0,1,2,3,...
72. A film with index of refraction 1.50 coats a glass lens with index of refraction 1.80. What is the minimum thickness of the thin film that will strongly reflect light with wavelength 600 nm?View SolutionQuestion 266
Thin films, including soap bubbles and oil show patterns of alternative dark and bright regions resulting from interference among the reflected ligth waves. If two waves are in phase, their crests and troughs will coincide. The interference will be cosntructive and the amlitude of resultant wave will be greater then either of constituent waves. If the two wave are not of phase by half a wavelength (180∘), the crests of one wave will coincide width the troughs of the other wave. The interference will be destructive and the ampliutde of the resultant wave will be less than that of either consituent wave.
1. When incident light I, reaches the surface at point a, some of the ligth is reflected as ray Ra and some is refracted following the path ab to the back of the film.
2. At point b, some of the light is refracted out of the film and part is reflected back through the film along path bc. At point c, some of the light is reflected back into the film and part is reflected out of the film as ray Rc.
Ra and Rc are parallel. However, Rc has travelled the extra distance within the film fo abc. If the angle of incidence is small, then abc is approxmately twice the film's thickness .
If Ra and Rc are in phase, they will undergo constructive interference and the region ac will be bright. If Ra and Rc are out of phase, they will undergo destructive interference and the region ac will be dark.
I. Refraction at an interface never changes the phase of the wave.
II. For reflection at the interfere between two media 1 and 2, if n1>n2, the reflected wave will change phase. If n1<n2, the reflected wave will not undergo a phase change.
For reference, nair=1.00.
III. If the waves are in phase after reflection at all intensities, then the effects of path length in the film are:
Constrictive interference occurs when 2t=mλ/n,m=0,1,2,3,...
Destrcutive interference occurs when 2t=(m+12)λn,
m=0,1,2,3,...
If the waves are 180∘ out of the phase after reflection at all interference, then the effects of path length in the film ara:
Constructive interference occurs when
2t=(m+12)λn,m=0,1,2,3,...
Destructive interference occurs when
2t=mλn,m=0,1,2,3,...
A thin film with index of refraction 1.33 coats a glass lens with index of refraction 1.50. Which of the following choices is the smallest film thickness that will not reflect light with wavelength 640 nm?View SolutionQuestion 267
Thin films, including soap bubbles and oil show patterns of alternative dark and bright regions resulting from interference among the reflected ligth waves. If two waves are in phase, their crests and troughs will coincide. The interference will be cosntructive and the amlitude of resultant wave will be greater then either of constituent waves. If the two wave are not of phase by half a wavelength (180∘), the crests of one wave will coincide width the troughs of the other wave. The interference will be destructive and the ampliutde of the resultant wave will be less than that of either consituent wave.
1. When incident light I, reaches the surface at point a, some of the ligth is reflected as ray Ra and some is refracted following the path ab to the back of the film.
2. At point b, some of the light is refracted out of the film and part is reflected back through the film along path bc. At point c, some of the light is reflected back into the film and part is reflected out of the film as ray Rc.
Ra and Rc are parallel. However, Rc has travelled the extra distance within the film fo abc. If the angle of incidence is small, then abc is approxmately twice the film's thickness .
If Ra and Rc are in phase, they will undergo constructive interference and the region ac will be bright. If Ra and Rc are out of phase, they will undergo destructive interference and the region ac will be dark.
I. Refraction at an interface never changes the phase of the wave.
II. For reflection at the interfere between two media 1 and 2, if n1>n2, the reflected wave will change phase. If n1<n2, the reflected wave will not undergo a phase change.
For reference, nair=1.00.
III. If the waves are in phase after reflection at all intensities, then the effects of path length in the film are:
Constrictive interference occurs when 2t=mλ/n,m=0,1,2,3,...
Destrcutive interference occurs when 2t=(m+12)λn,
m=0,1,2,3,...
If the waves are 180∘ out of the phase after reflection at all interference, then the effects of path length in the film ara:
Constructive interference occurs when
2t=(m+12)λn,m=0,1,2,3,...
Destructive interference occurs when
2t=mλn,m=0,1,2,3,...
A soap film of thickness t is surrounded by air and is illuminated at near normal incidence by noonochromatic light wavelength λ in the film. With respect to the wavelength of the monochromatic ligth in the film, what film thickness will produce maximum consturctive interferenceView SolutionQuestion 268
Thin films, including soap bubbles and oil show patterns of alternative dark and bright regions resulting from interference among the reflected ligth waves. If two waves are in phase, their crests and troughs will coincide. The interference will be cosntructive and the amlitude of resultant wave will be greater then either of constituent waves. If the two wave are not of phase by half a wavelength (180∘), the crests of one wave will coincide width the troughs of the other wave. The interference will be destructive and the ampliutde of the resultant wave will be less than that of either consituent wave.
1. When incident light I, reaches the surface at point a, some of the ligth is reflected as ray Ra and some is refracted following the path ab to the back of the film.
2. At point b, some of the light is refracted out of the film and part is reflected back through the film along path bc. At point c, some of the light is reflected back into the film and part is reflected out of the film as ray Rc.
Ra and Rc are parallel. However, Rc has travelled the extra distance within the film fo abc. If the angle of incidence is small, then abc is approxmately twice the film's thickness .
If Ra and Rc are in phase, they will undergo constructive interference and the region ac will be bright. If Ra and Rc are out of phase, they will undergo destructive interference and the region ac will be dark.
I. Refraction at an interface never changes the phase of the wave.
II. For reflection at the interfere between two media 1 and 2, if n1>n2, the reflected wave will change phase. If n1<n2, the reflected wave will not undergo a phase change.
For reference, nair=1.00.
III. If the waves are in phase after reflection at all intensities, then the effects of path length in the film are:
Constrictive interference occurs when 2t=mλ/n,m=0,1,2,3,...
Destrcutive interference occurs when 2t=(m+12)λn,
m=0,1,2,3,...
If the waves are 180∘ out of the phase after reflection at all interference, then the effects of path length in the film ara:
Constructive interference occurs when
2t=(m+12)λn,m=0,1,2,3,...
Destructive interference occurs when
2t=mλn,m=0,1,2,3,...
The average human eye sees colors with wavelength between 430 nm to 680 nm. For what visible wavelength (s) will a 350 nm thick (n=1.35) soap film produce maximum destructive interference?View SolutionQuestion 269
Thin films, including soap bubbles and oil show patterns of alternative dark and bright regions resulting from interference among the reflected ligth waves. If two waves are in phase, their crests and troughs will coincide. The interference will be cosntructive and the amlitude of resultant wave will be greater then either of constituent waves. If the two wave are not of phase by half a wavelength (180∘), the crests of one wave will coincide width the troughs of the other wave. The interference will be destructive and the ampliutde of the resultant wave will be less than that of either consituent wave.
1. When incident light I, reaches the surface at point a, some of the ligth is reflected as ray Ra and some is refracted following the path ab to the back of the film.
2. At point b, some of the light is refracted out of the film and part is reflected back through the film along path bc. At point c, some of the light is reflected back into the film and part is reflected out of the film as ray Rc.
Ra and Rc are parallel. However, Rc has travelled the extra distance within the film fo abc. If the angle of incidence is small, then abc is approxmately twice the film's thickness .
If Ra and Rc are in phase, they will undergo constructive interference and the region ac will be bright. If Ra and Rc are out of phase, they will undergo destructive interference and the region ac will be dark.
I. Refraction at an interface never changes the phase of the wave.
II. For reflection at the interfere between two media 1 and 2, if n1>n2, the reflected wave will change phase. If n1<n2, the reflected wave will not undergo a phase change.
For reference, nair=1.00.
III. If the waves are in phase after reflection at all intensities, then the effects of path length in the film are:
Constrictive interference occurs when 2t=mλ/n,m=0,1,2,3,...
Destrcutive interference occurs when 2t=(m+12)λn,
m=0,1,2,3,...
If the waves are 180∘ out of the phase after reflection at all interference, then the effects of path length in the film ara:
Constructive interference occurs when
2t=(m+12)λn,m=0,1,2,3,...
Destructive interference occurs when
2t=mλn,m=0,1,2,3,...
A 600 nm light is perpendicularly incident on a soap film suspended air. The film is 1.00 μm thick with n=.37. Which statement most accurately describes the interference of ligth reflected by the two surfaces of the film?View SolutionQuestion 270
Thin films, including soap bubbles and oil show patterns of alternative dark and bright regions resulting from interference among the reflected ligth waves. If two waves are in phase, their crests and troughs will coincide. The interference will be cosntructive and the amlitude of resultant wave will be greater then either of constituent waves. If the two wave are not of phase by half a wavelength (180∘), the crests of one wave will coincide width the troughs of the other wave. The interference will be destructive and the ampliutde of the resultant wave will be less than that of either consituent wave.
1. When incident light I, reaches the surface at point a, some of the ligth is reflected as ray Ra and some is refracted following the path ab to the back of the film.
2. At point b, some of the light is refracted out of the film and part is reflected back through the film along path bc. At point c, some of the light is reflected back into the film and part is reflected out of the film as ray Rc.
Ra and Rc are parallel. However, Rc has travelled the extra distance within the film fo abc. If the angle of incidence is small, then abc is approxmately twice the film's thickness .
If Ra and Rc are in phase, they will undergo constructive interference and the region ac will be bright. If Ra and Rc are out of phase, they will undergo destructive interference and the region ac will be dark.
I. Refraction at an interface never changes the phase of the wave.
II. For reflection at the interfere between two media 1 and 2, if n1>n2, the reflected wave will change phase. If n1<n2, the reflected wave will not undergo a phase change.
For reference, nair=1.00.
III. If the waves are in phase after reflection at all intensities, then the effects of path length in the film are:
Constrictive interference occurs when 2t=mλ/n,m=0,1,2,3,...
Destrcutive interference occurs when 2t=(m+12)λn,
m=0,1,2,3,...
If the waves are 180∘ out of the phase after reflection at all interference, then the effects of path length in the film ara:
Constructive interference occurs when
2t=(m+12)λn,m=0,1,2,3,...
Destructive interference occurs when
2t=mλn,m=0,1,2,3,...
A thin of liquid polymer, n=1.25, coats a slab of pyrex, n=1.50. White light is incident perpendicularly on the film. In the reflection, full destructive interference occurs for λ=600nm and full constructive interference occurs for λ=700nm What is the thickness of the polymer film?View SolutionQuestion 271
In YDSE set up (see fig.), the light sources executes SHM between P and Q according to the equation x=Asinωt, S being the mean position. Assume d→0 and A<<L. ω is small enough to neglect Doppler effect. If the sources were stationary at S, intenstiy at O would be I0
Read the paragraph carefully and answer the following questions.
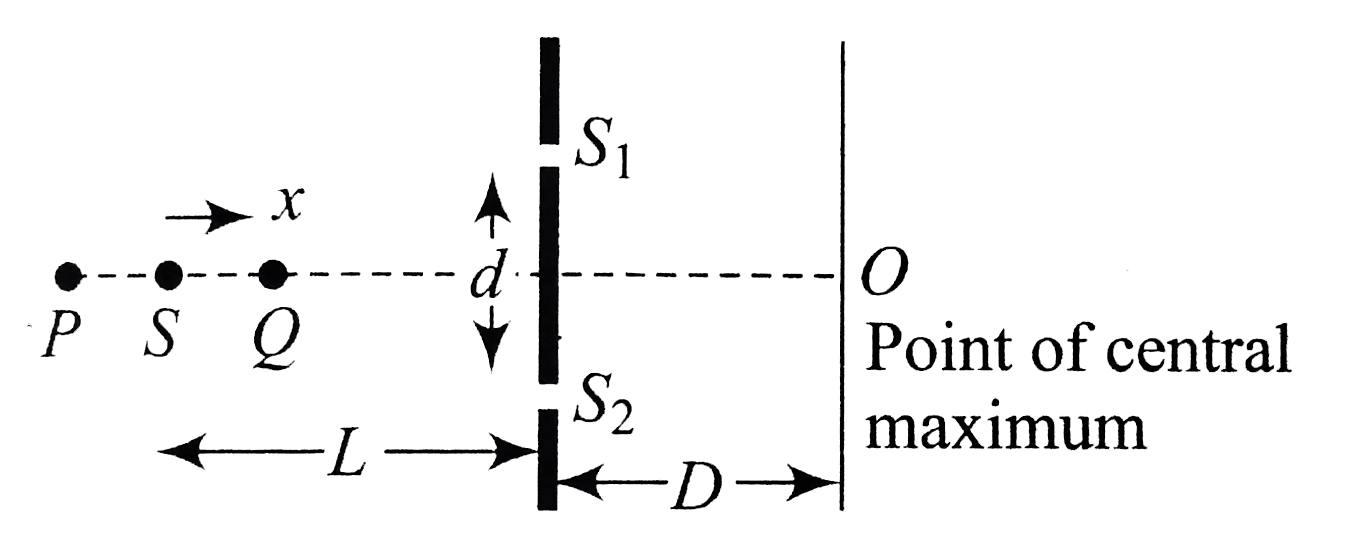
The fractional change in intensity of the central maximum as function of time isView SolutionQuestion 272
In YDSE set up (see fig.), the light sources executes SHM between P and Q according to the equation x=Asinωt, S being the mean position. Assume d→0 and A<<L. ω is small enough to neglect Doppler effect. If the sources were stationary at S, intenstiy at O would be I0
Read the paragraph carefully and answer the following questions.
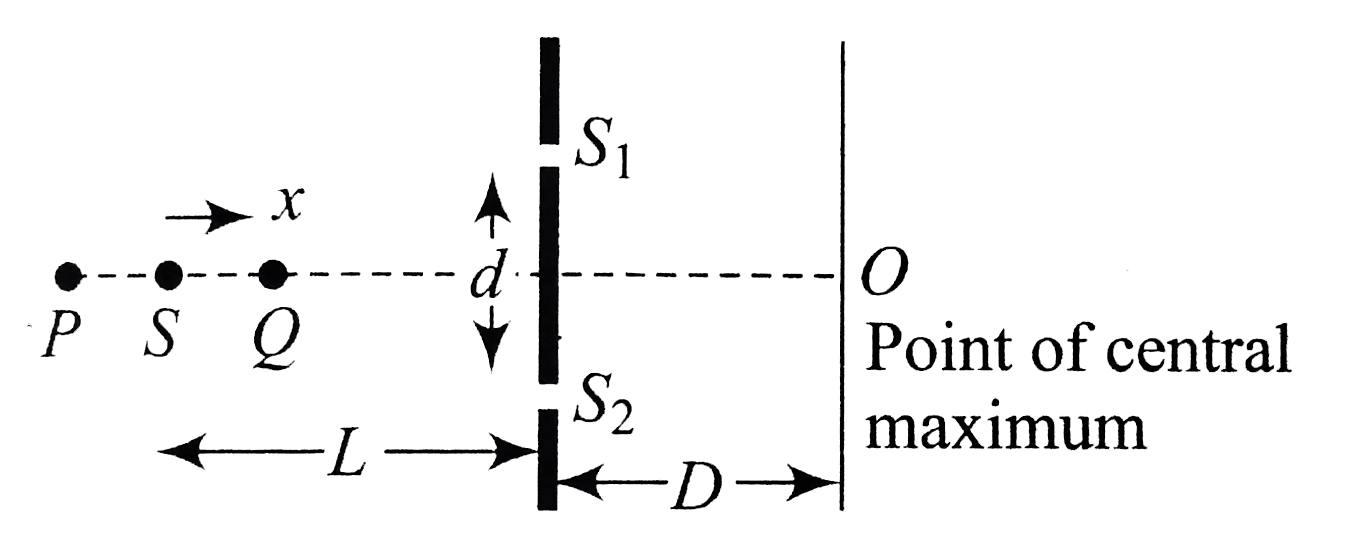
When the source comes toward the point Q,View SolutionQuestion 273
In YDSE set up (see fig.), the light sources executes SHM between P and Q according to the equation x=Asinωt, S being the mean position. Assume d→0 and A<<L. ω is small enough to neglect Doppler effect. If the sources were stationary at S, intenstiy at O would be I0
Read the paragraph carefully and answer the following questions.
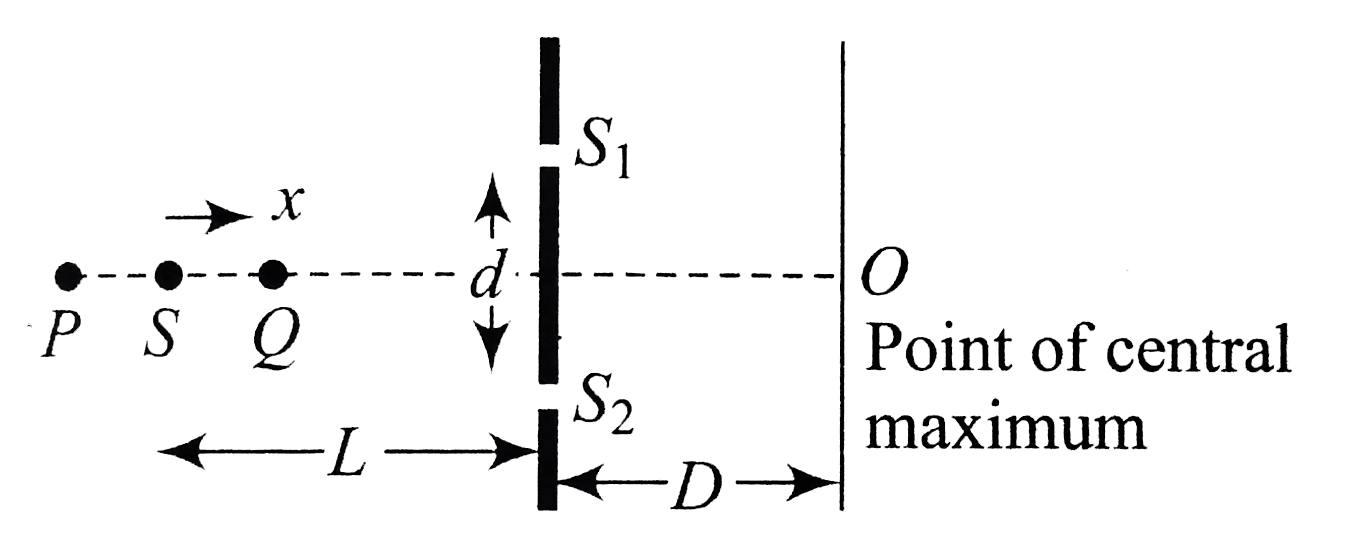
The fringe width β can be expressed asView SolutionQuestion 276
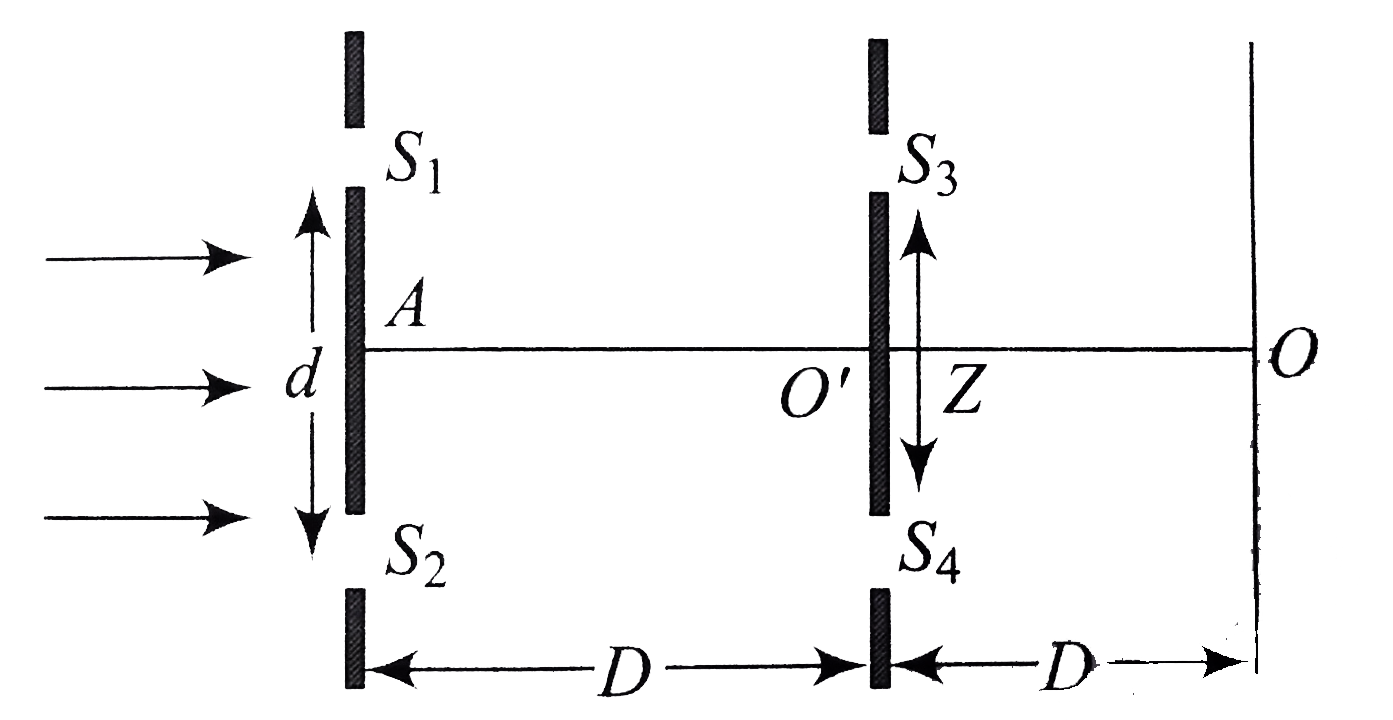
In the arrangement shown in Fig., slits S1 and S4are having a variable separation Z. Point O on the screen is at the common perpendicular bisector of S1S2 and S3S4.
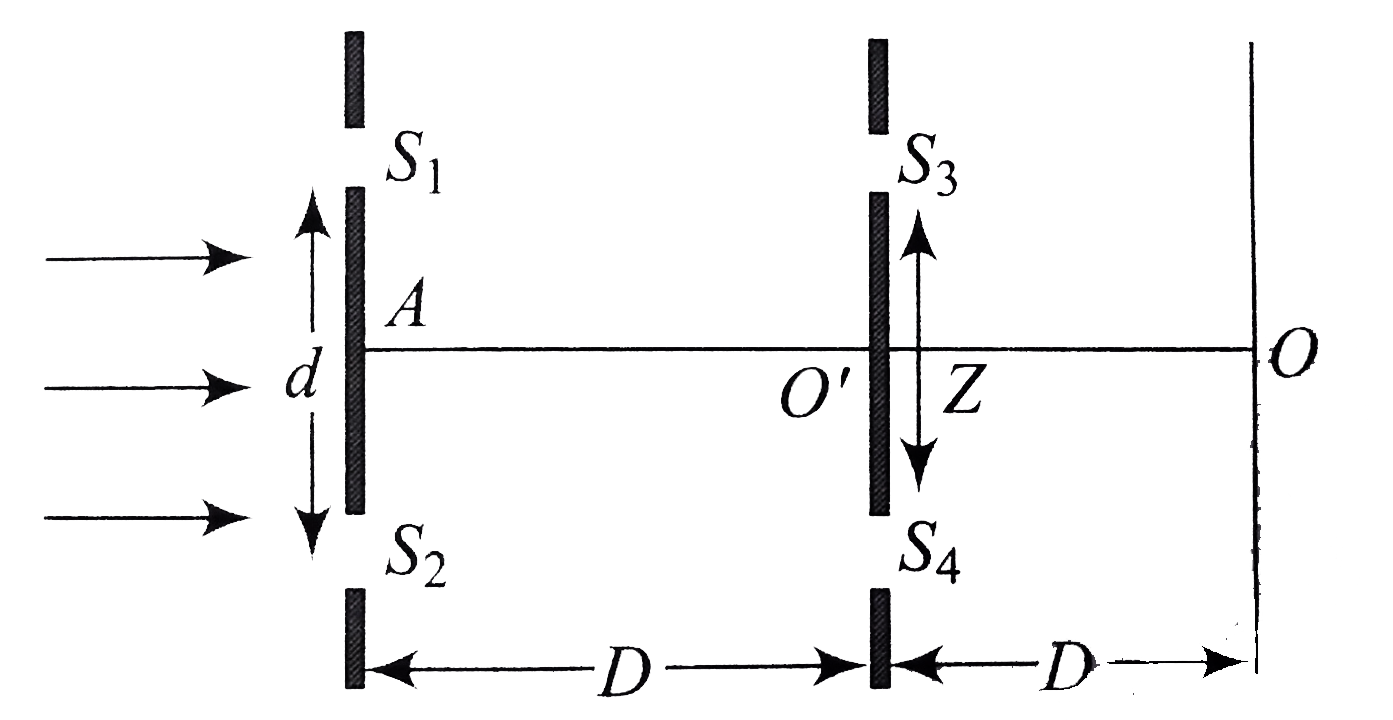
If a hole is made at O' on AO' O and the slit S4 is closed, then the ratio of the maximum to minimum observed on screen at O , if O'S3 is equal to λD4d, isView SolutionQuestion 278
A monochromatic light of λ=500Å is incident on two indentical slits separated by a distance of 5×10−4 m. The interference pattern is seen on a screen placed at a distance of 1 m from the plane of slits. A thin glass plate of thickness 1.5×10−6 m and refractive index μ=1.5 is placed between one of the slits and the screen . Find the intensity at the center of the screen.View SolutionQuestion 279
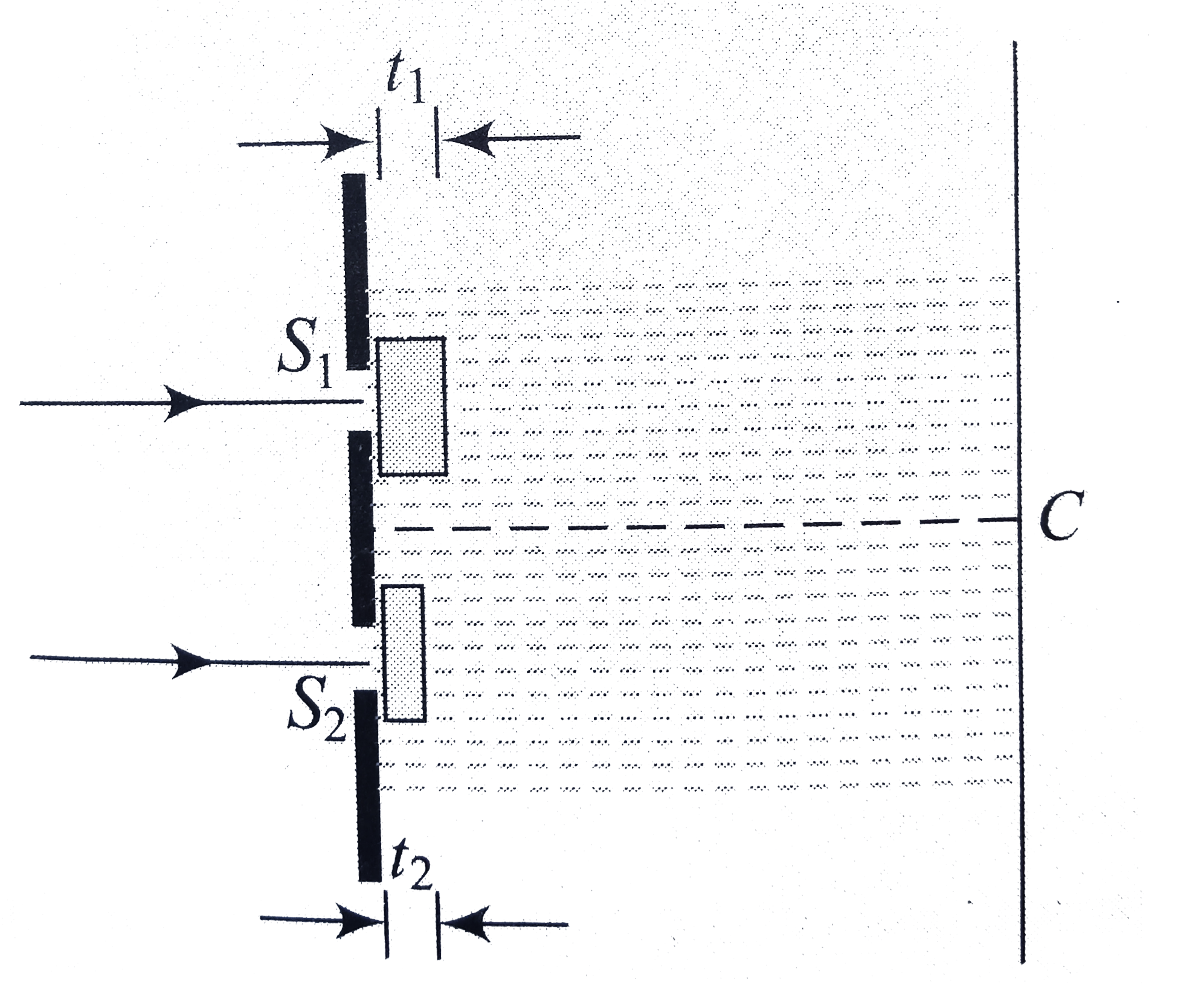
A screen is at distance D=80 cm form a diaphragm having two narrow slits S1 and S2 which are d=2 mm apart.
Slit S1 is covered by a transparent sheet of thickness
t1=2.5μm slit S2 is covered by another sheet of thikness
t2=1.25μm as shown if Fig. 2.52.
Both sheets are made of same material having refractive index μ=1.40
Water is filled in the space between diaphragm and screen. Amondichromatic light beam of wavelength λ=5000Å is incident normally on the diaphragm.
Assuming intensity of beam to be uniform, calculate ratio of intensity of C to maximum intensity of interference pattern obtained on the screen (μw=4/3)
 View Solution
View SolutionQuestion 280
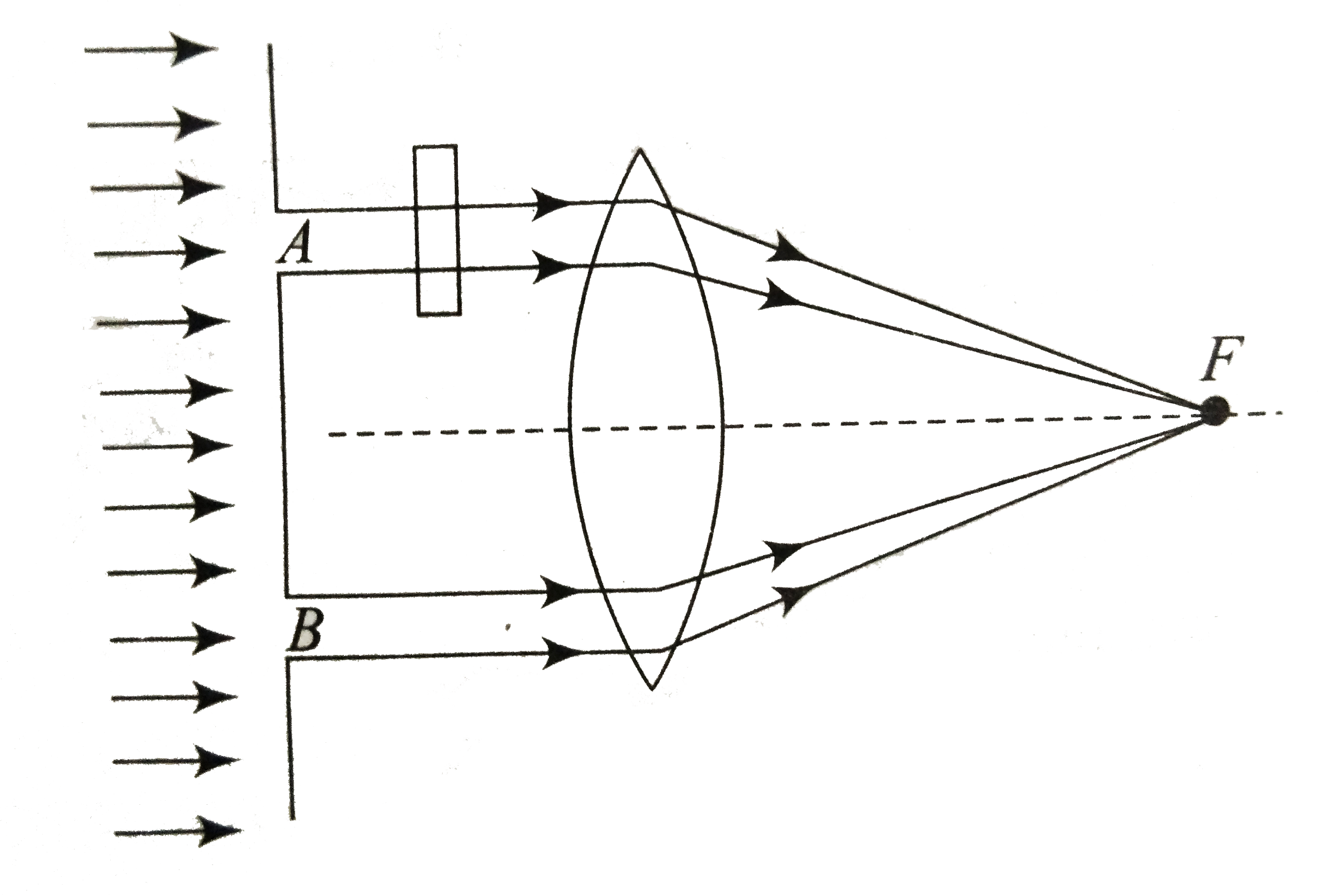
In a modified Young's double-slit experiment, a monochromatic uniform and parallel beam of light of wavelength 6000Å and intensity (10/π) W m−2 is incident normally on two circular apertures A and B of radii 0.001 m and 0.002 m, respectively. A perfectly transparent film of thickness 2000Å and refractive index 1.5 for the wavelength of 6000Å is placed in front of aperture A (see the figure). Calculate the power (in mW) received at the focal spot F of the lens. Then lens is symmetrically placed with respect to the aperture. Assume that 10% of the power received by each aperture goes in the original direction and is brought to the focal spot.
 View Solution
View SolutionQuestion 281
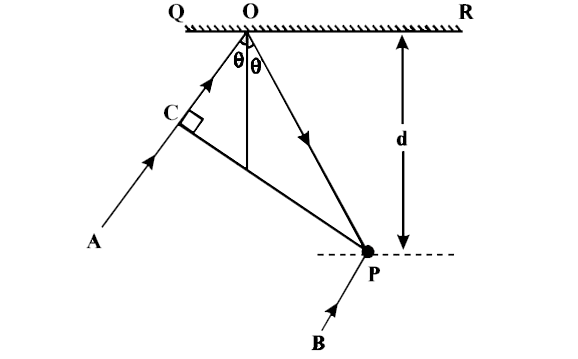
A narrow monochromatic beam of light of intensity 1 is incident on a glass plate as shown in figure Another identical glass plate is kept close to the first one and parallel to it. Each glass plate reflects 25% of the light incident on it and transmits intensities in the interference pattern formed by two beams obtained after one reflection at each plate.
 View Solution
View SolutionQuestion 282
A glass of refractive index 1.5 is coated with a thin layer of thickness t and refractive index 1.8 . Light of wavelength λ travelling in air is incident normally on the layer. It is partly reflected at the upper and the lower surfaces of the layer. It is partly reflected at the upper and the lower surfaces of the layer ant the two reflected rays interface . If λ=648nm, obtain the least value of t(in10−8m) which the rays interface constructively.View SolutionQuestion 287
In Young's double-slit experiment, the two slits act as coherent sources of equal amplitude A and of wavelength λ. In another experiment with the same set-up the two slits are sources of equal amplitude A and wavelength λ, but are incoherent. The ratio of the intensity of light at the midpoint of the screen in the first case to that in the second case is....View SolutionQuestion 291
In an interference arrangement similar to Young's double-slit experiment, the slits S_1 and S_2 are illuminated with coherent microwave sources, each of frequency 10^6 Hz. The sources are synchronized to have zero phase difference. The slits are separated by a distance d=150.0 m. The intensity I (theta) is measured as a function of theta, where theta is defined as shown. If I_0 is the maximum intensity, then I (theta) for 0lethetale90degree is given by
 View Solution
View SolutionQuestion 296
In the ideal double-slit experiment, when a glass-plate(refractive index 1.5) of thickness t is introduced in the path of one of the interfering beams (wave-length λ), the intensity at the position where the central maximum occurred previously remains unchanged. The minimum thickness of the glass-plate isView SolutionQuestion 304
White light is used to illuminate the two slits in a Young's double slit experiment. The separation between the slits is b and the screen is at a distance d(gtb) from the slits. At a point on the screen directly in front of one of the slits, certain wavelength are missing. Some of these missing wavelength areView SolutionQuestion 305
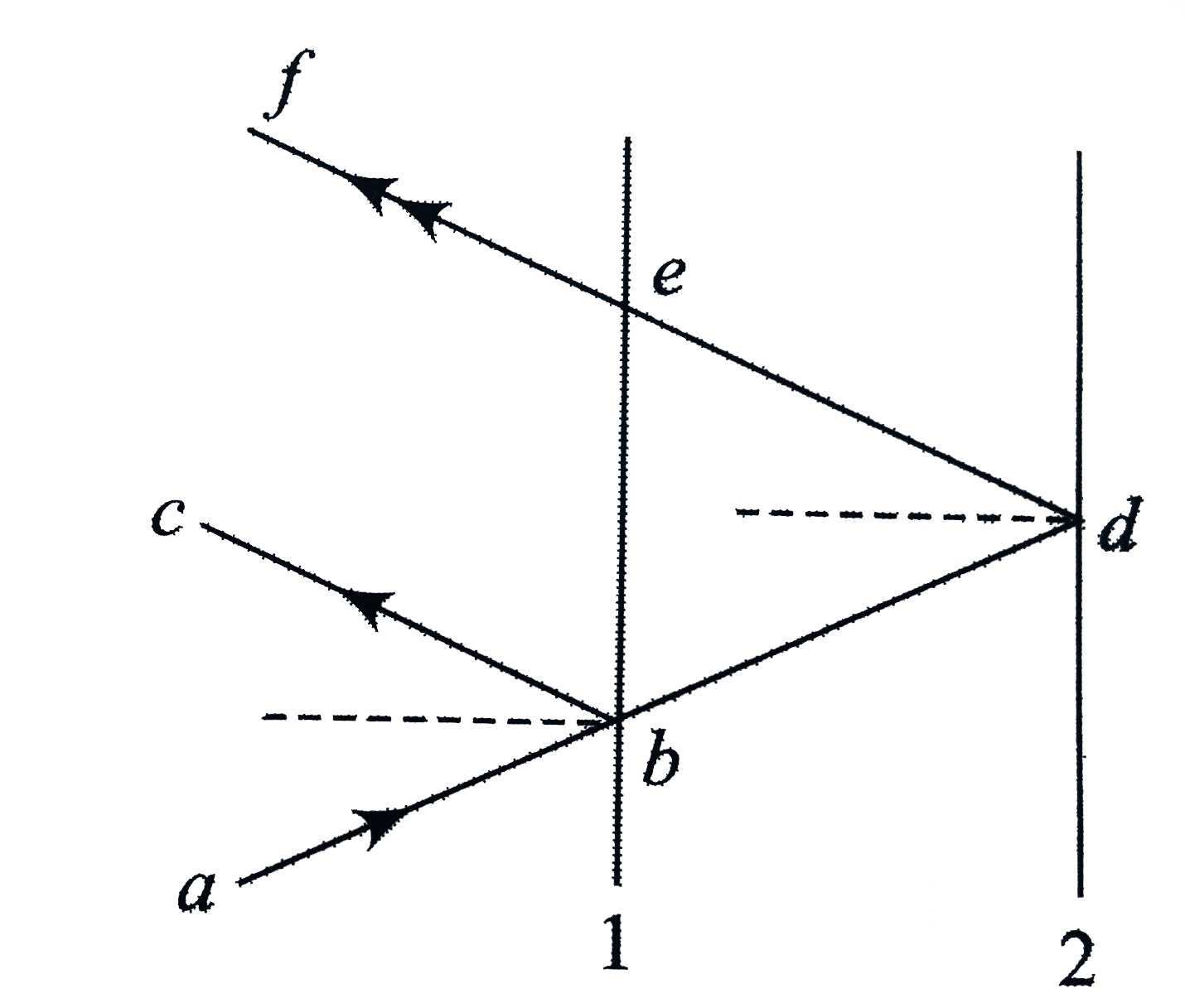
Fig. shows a surface XY separating two transparent media, medium 1 and medium 2. Lines ab and cd represent wavefronts of a light wave travelling in medium 1 and incident on XY. Line ef and gh represent wavefront of the light wave in medium 2 after rafraciton.
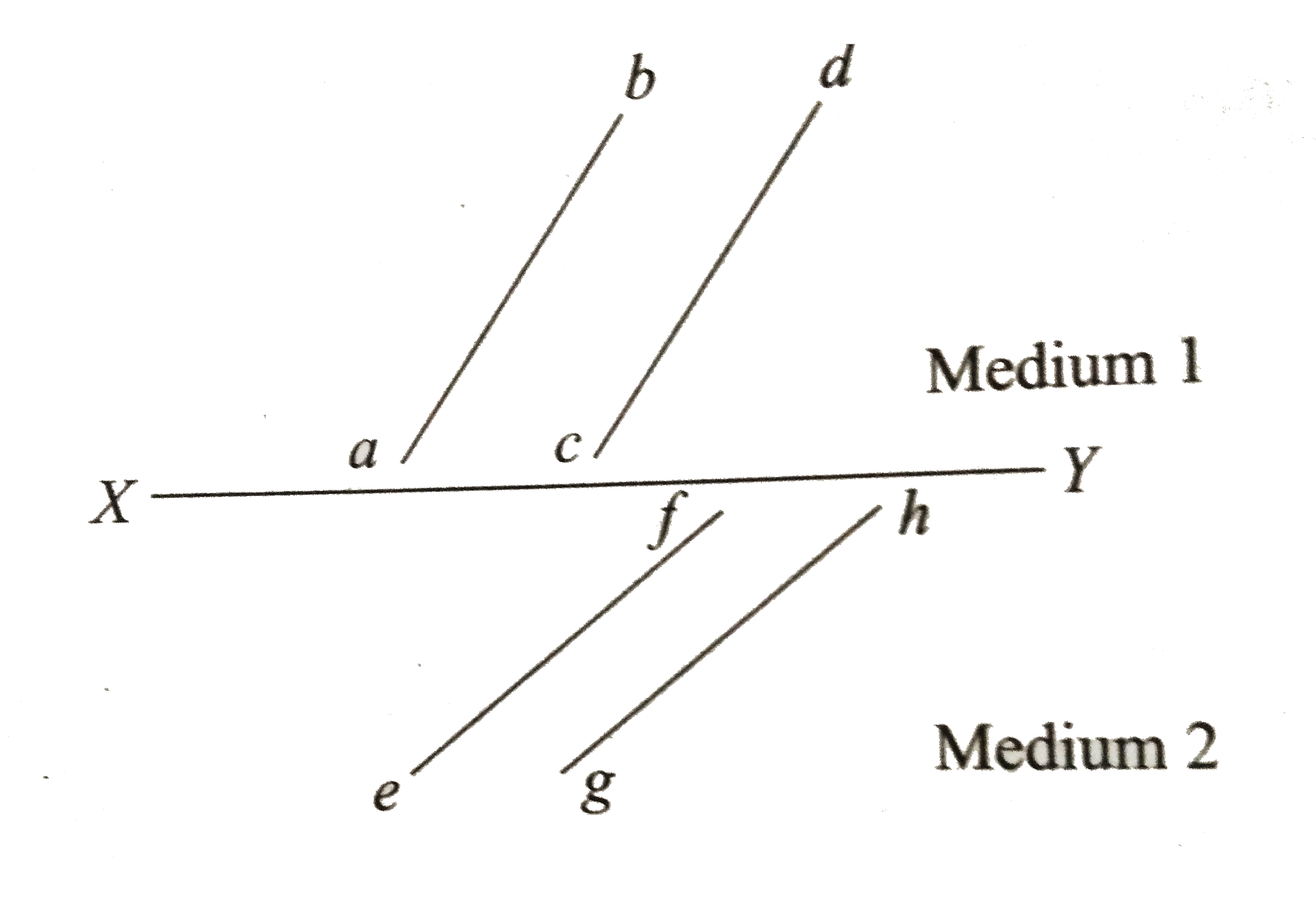
Light travel as aView SolutionQuestion 306
Fig. shows a surface XY separating two transparent media, medium 1 and medium 2. Lines ab and cd represent wavefronts of a light wave travelling in medium 1 and incident on XY. Line ef and gh represent wavefront of the light wave in medium 2 after rafraciton.

The phase of the ligth wave at c, d, e, and f are ϕc, phi_(d), ϕe and ϕf, respectively. It is given that ϕc≠ϕf. ThenView SolutionQuestion 307
Fig. shows a surface XY separating two transparent media, medium 1 and medium 2. Lines ab and cd represent wavefronts of a light wave travelling in medium 1 and incident on XY. Line ef and gh represent wavefront of the light wave in medium 2 after rafraciton.
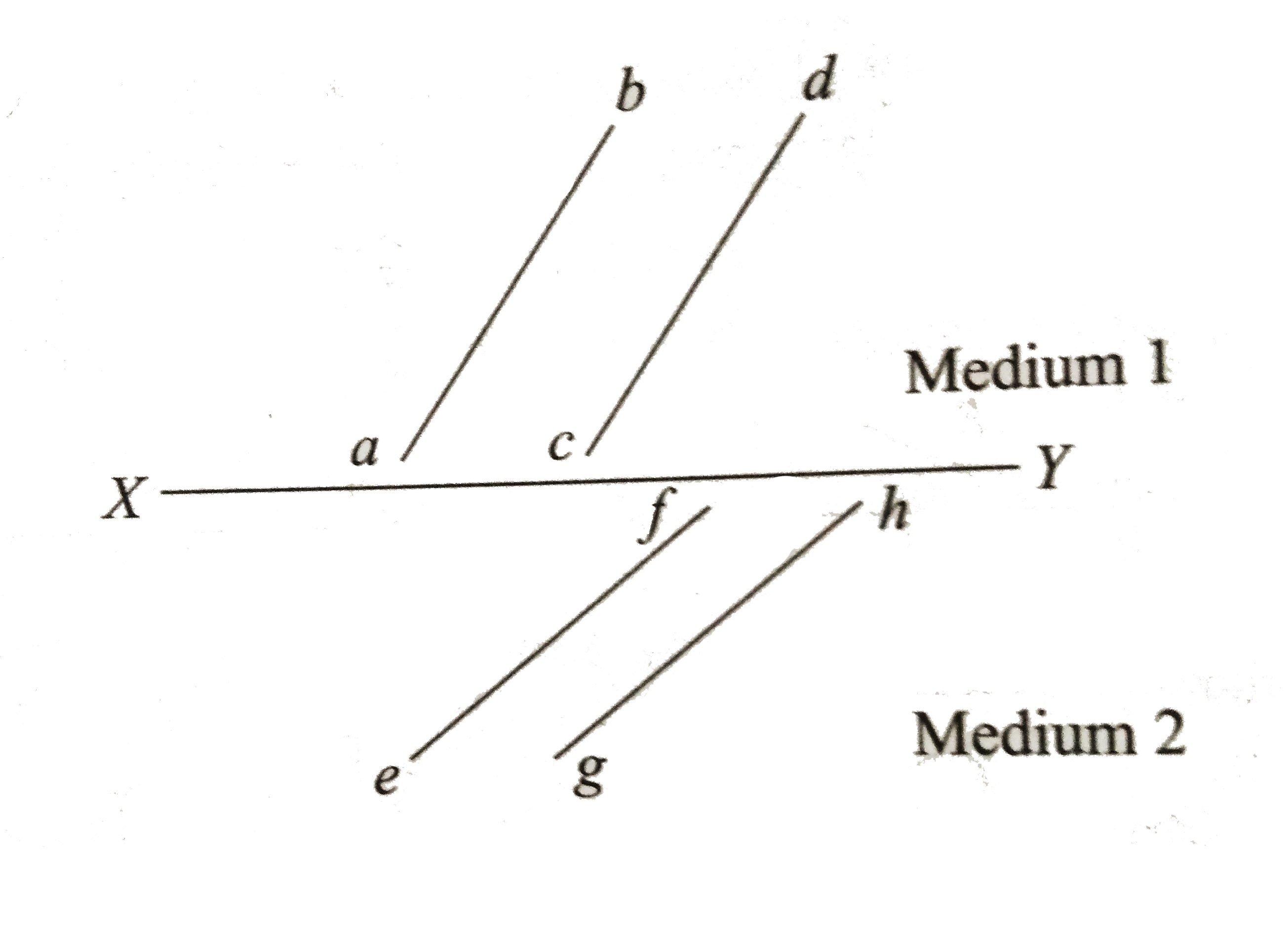 Speed of light isView Solution
Speed of light isView SolutionQuestion 308
Most materials have the refractive index, n>1. So, when a light ray from air enters a naturally occuring material, then by Snell's law, sinθ1sinθ2=n1n2, it is understood that the refracted ray bends towards the normal. But it never emerges on the same side of the normal as the incident ray. According to electromagnetism, the refractive index of the medium is given by the relation, n=(c/v)=±√εr,μr, where c is the speed of the electromagnetic waves in vacuum, v its speed in the medium, εr and μr are negative, one must choose the negative root of n. Such negative refractive index materials can now be artifically prepared and are called meta-materials. They exhibit significantly different optical behaviour, without violating any physical laws. Since n is negative, it results in a change in the direction of propagation of the refracted light. However, similar to normal materials, the frequency of light remains unchanged upon refraction even in meta-materials.
Answer the following questions :
Choose the correct statement. View SolutionQuestion 309
Most materials have the refractive index, n>1. So, when a light ray from air enters a naturally occuring material, then by Snell's law, sinθ1sinθ2=n1n2, it is understood that the refracted ray bends towards the normal. But it never emerges on the same side of the normal as the incident ray. According to electromagnetism, the refractive index of the medium is given by the relation, n=(c/v)=±√εr,μr, where c is the speed of the electromagnetic waves in vacuum, v its speed in the medium, εr and μr are negative, one must choose the negative root of n. Such negative refractive index materials can now be artifically prepared and are called meta-materials. They exhibit significantly different optical behaviour, without violating any physical laws. Since n is negative, it results in a change in the direction of propagation of the refracted light. However, similar to normal materials, the frequency of light remains unchanged upon refraction even in meta-materials.
Answer the following questions :
For light incident from air on a meta-material, the appropriate ray diagram is View Solution
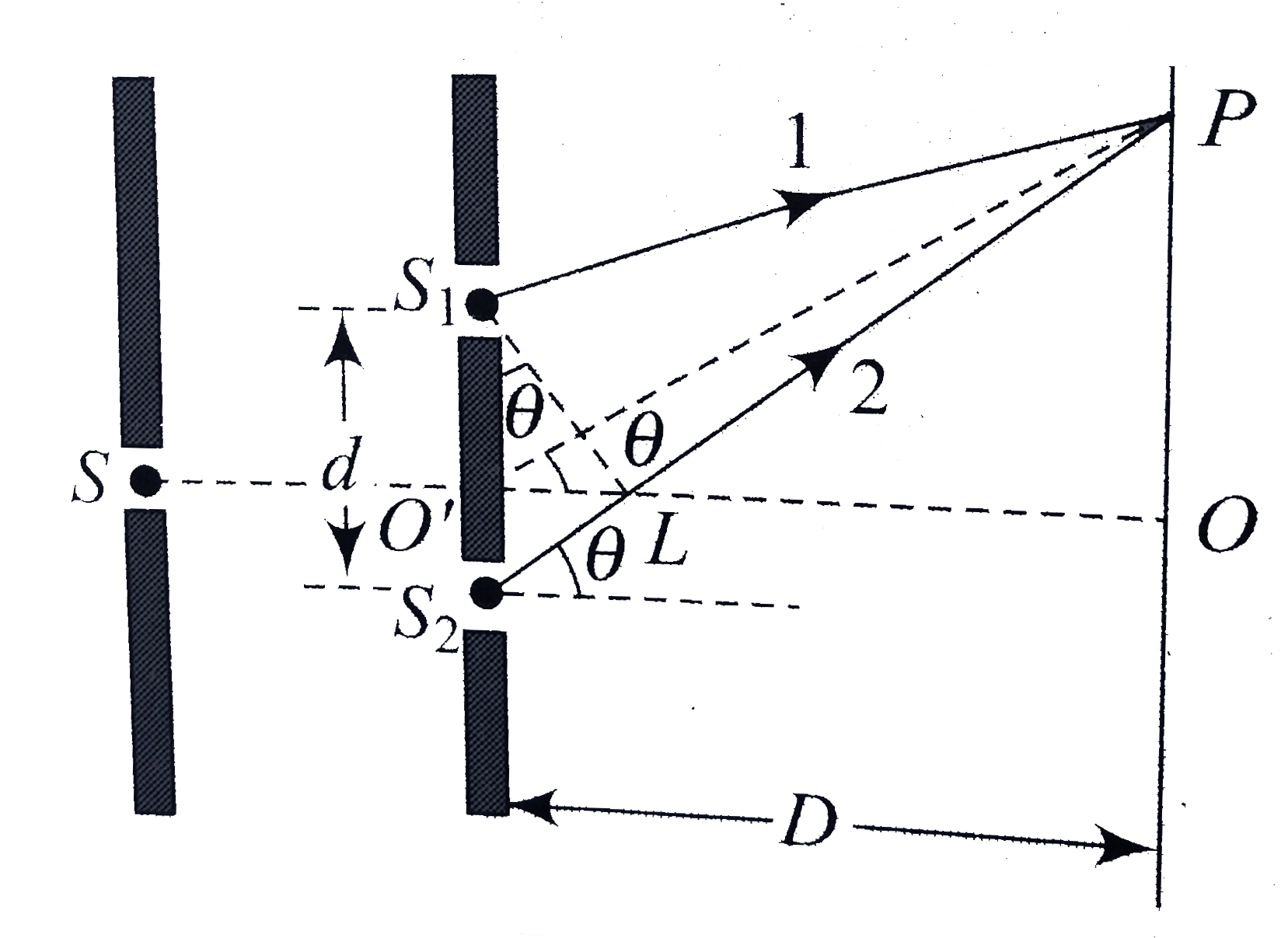 .
.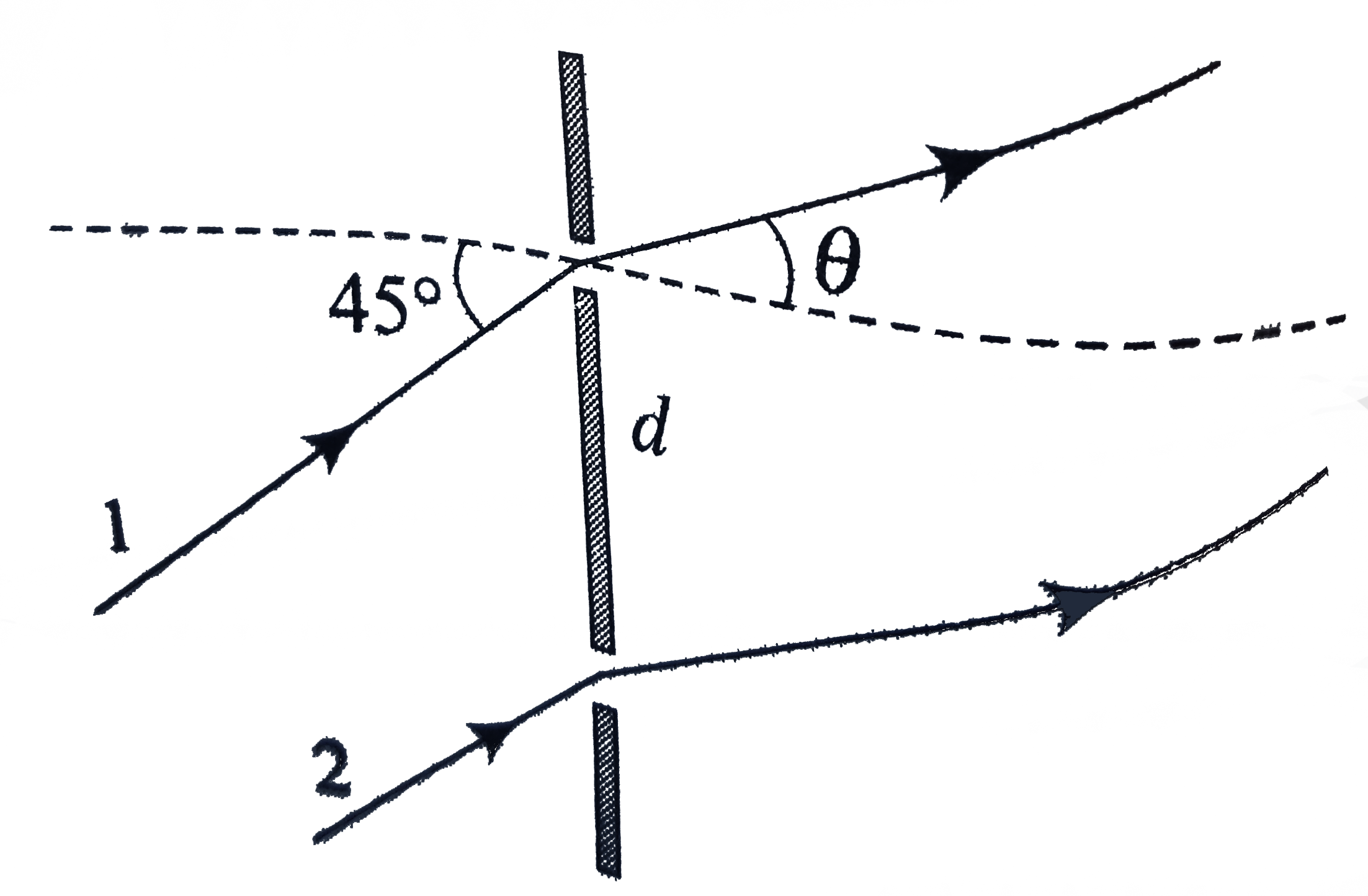

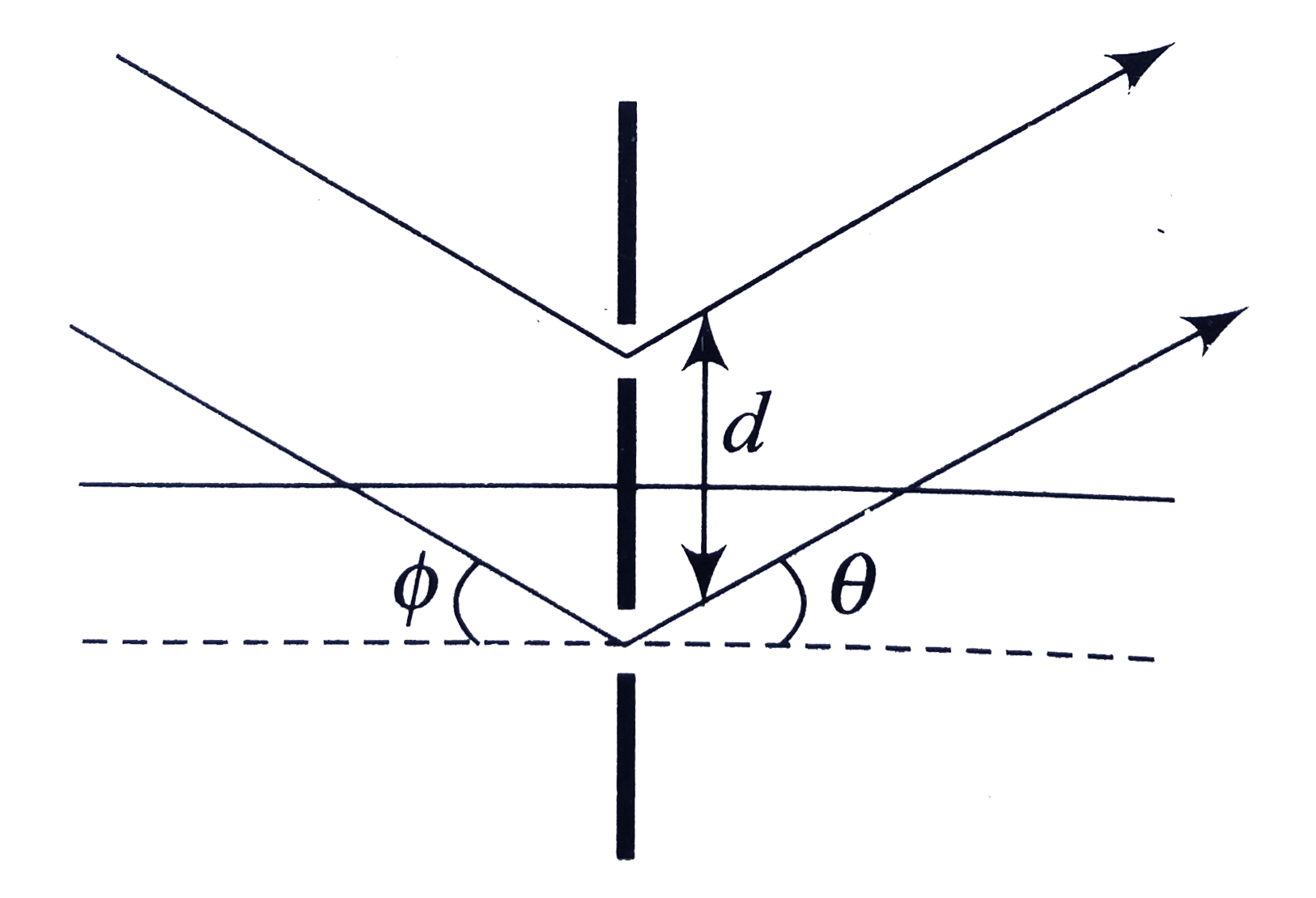
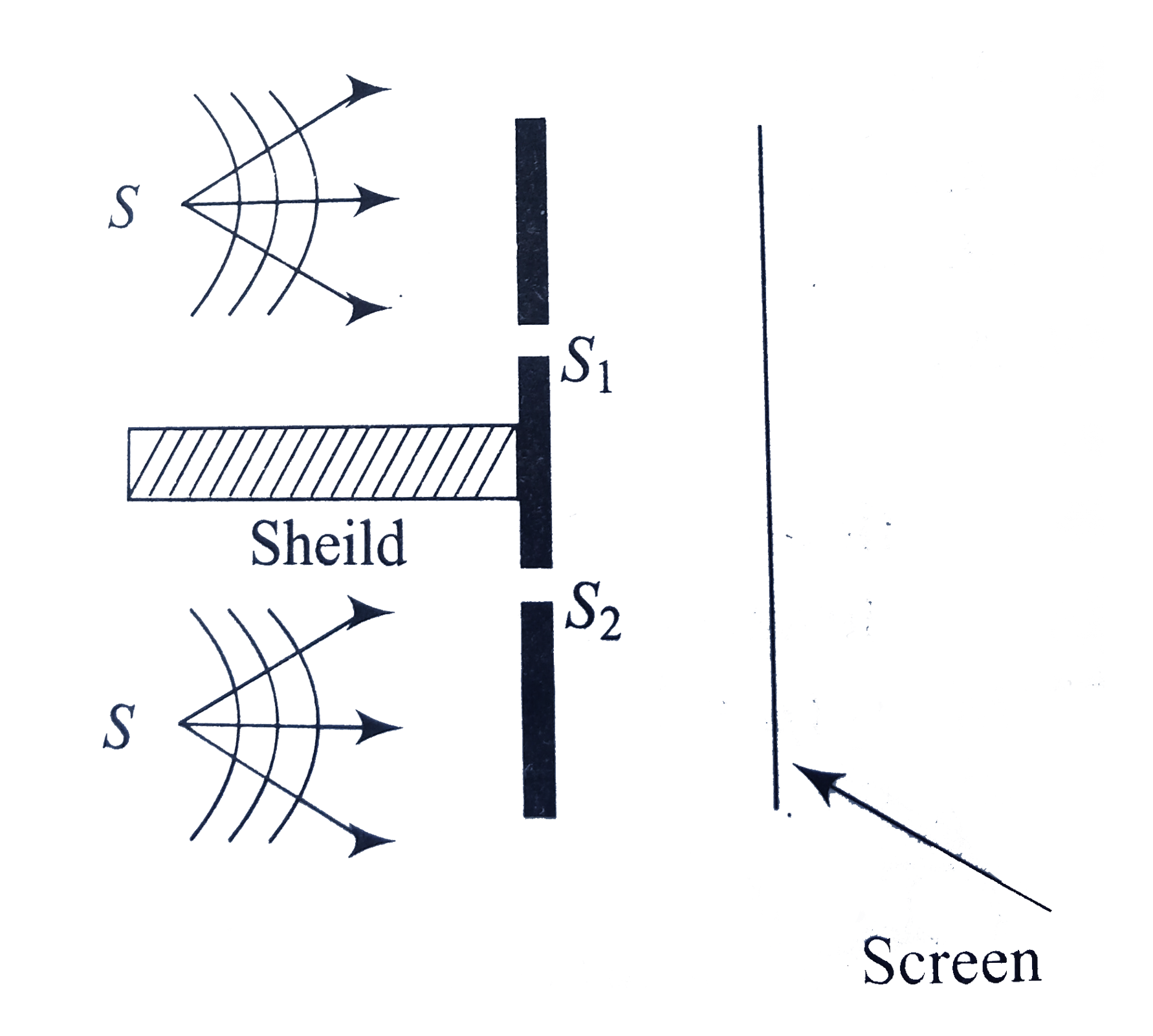
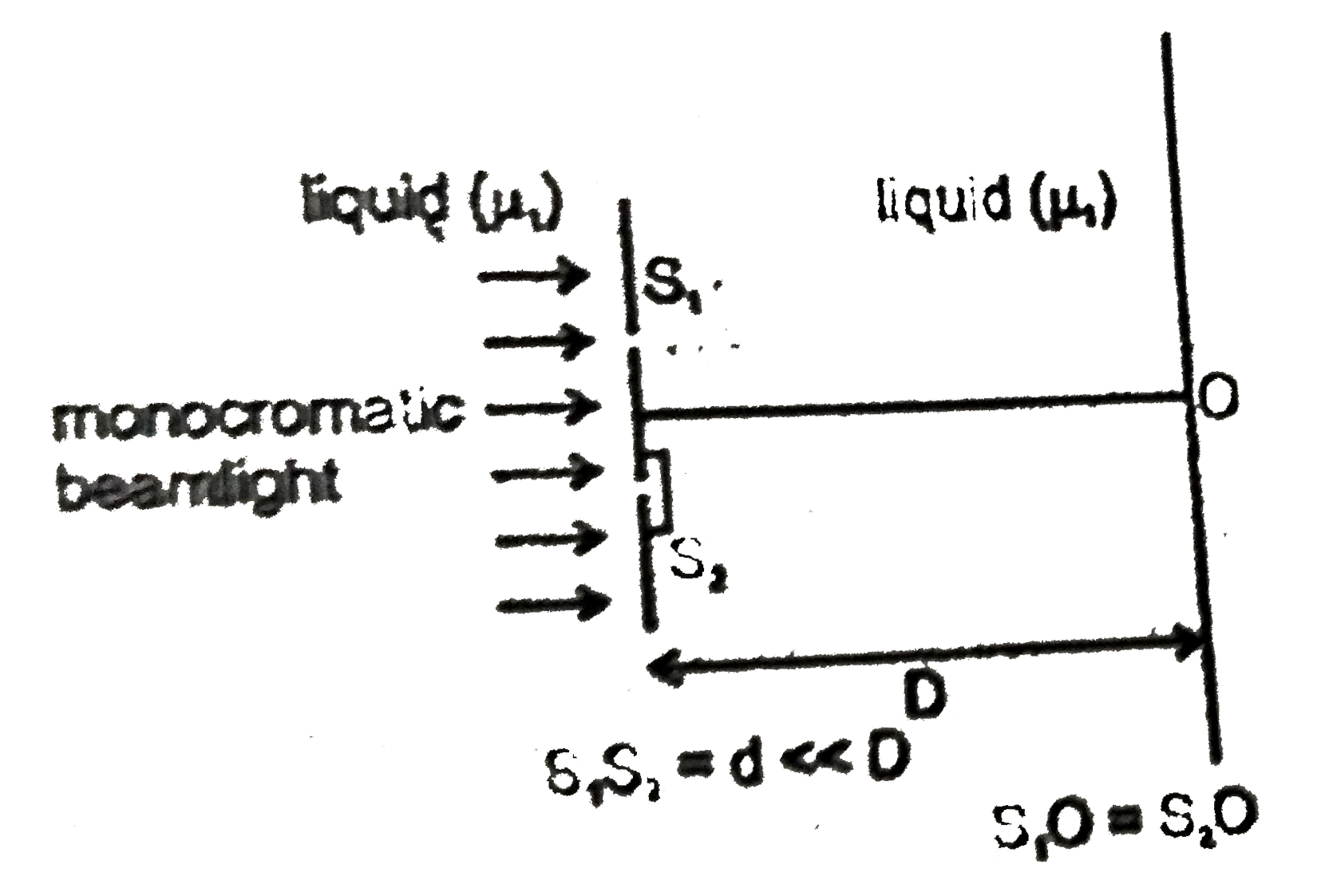

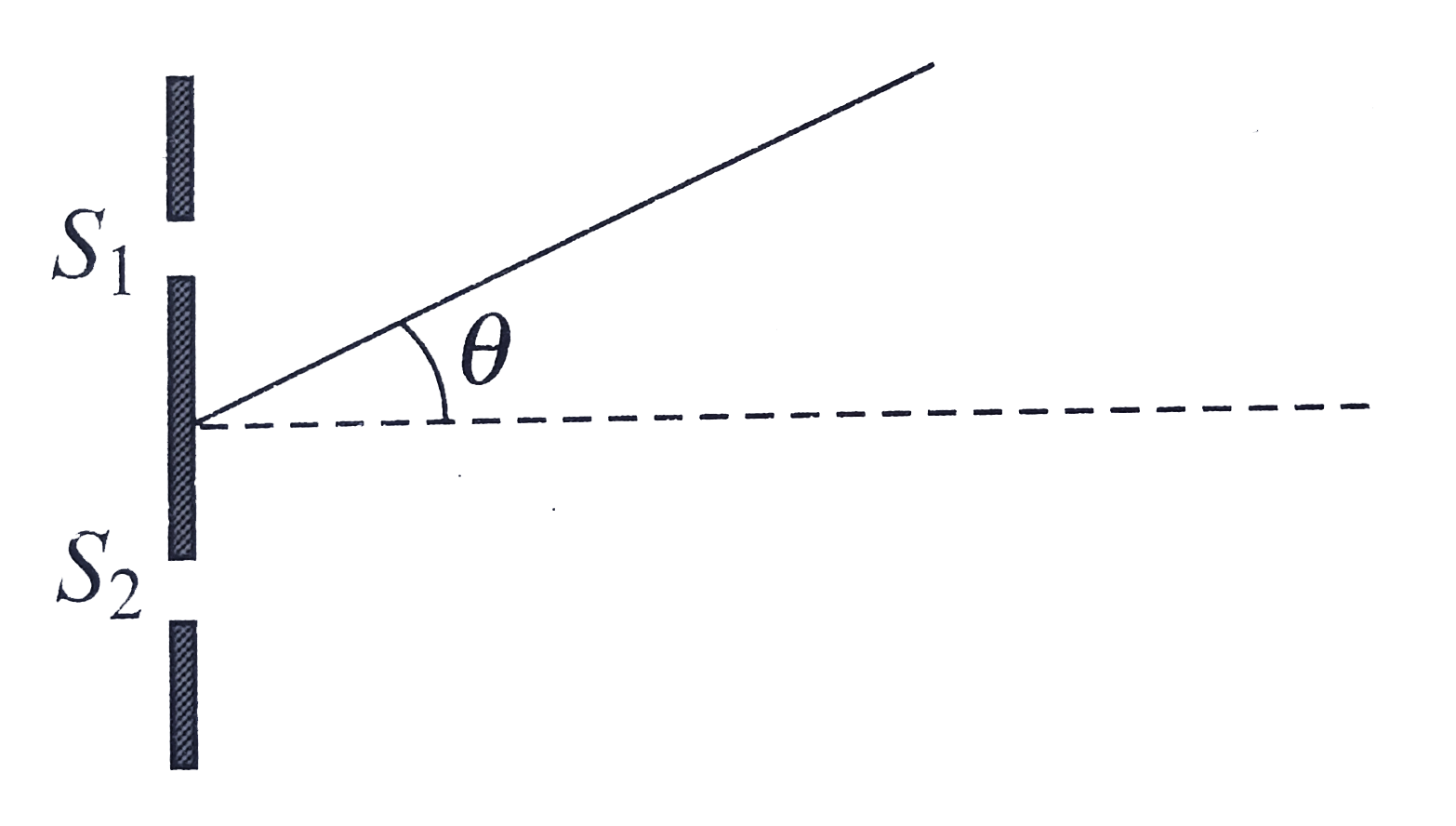

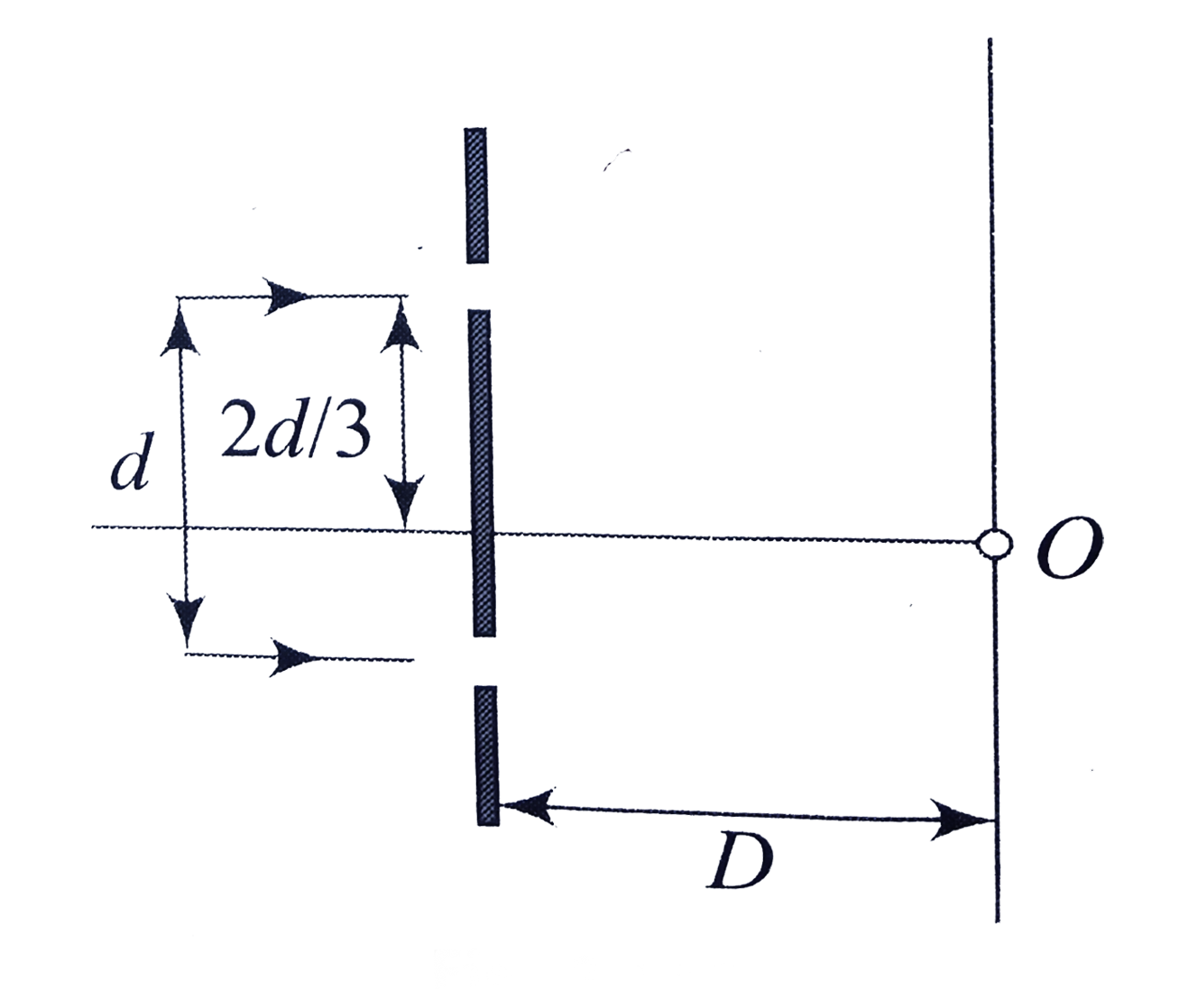

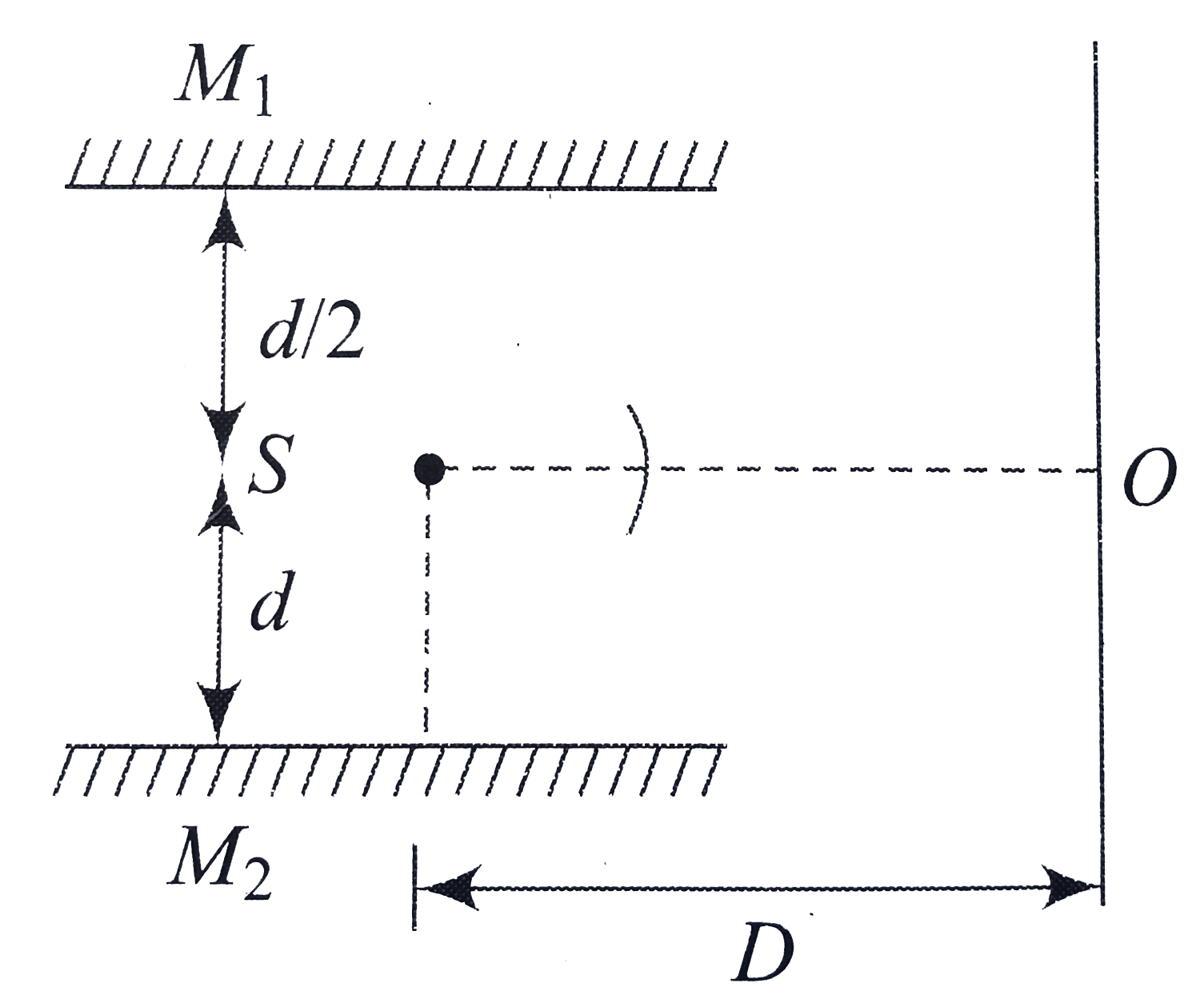
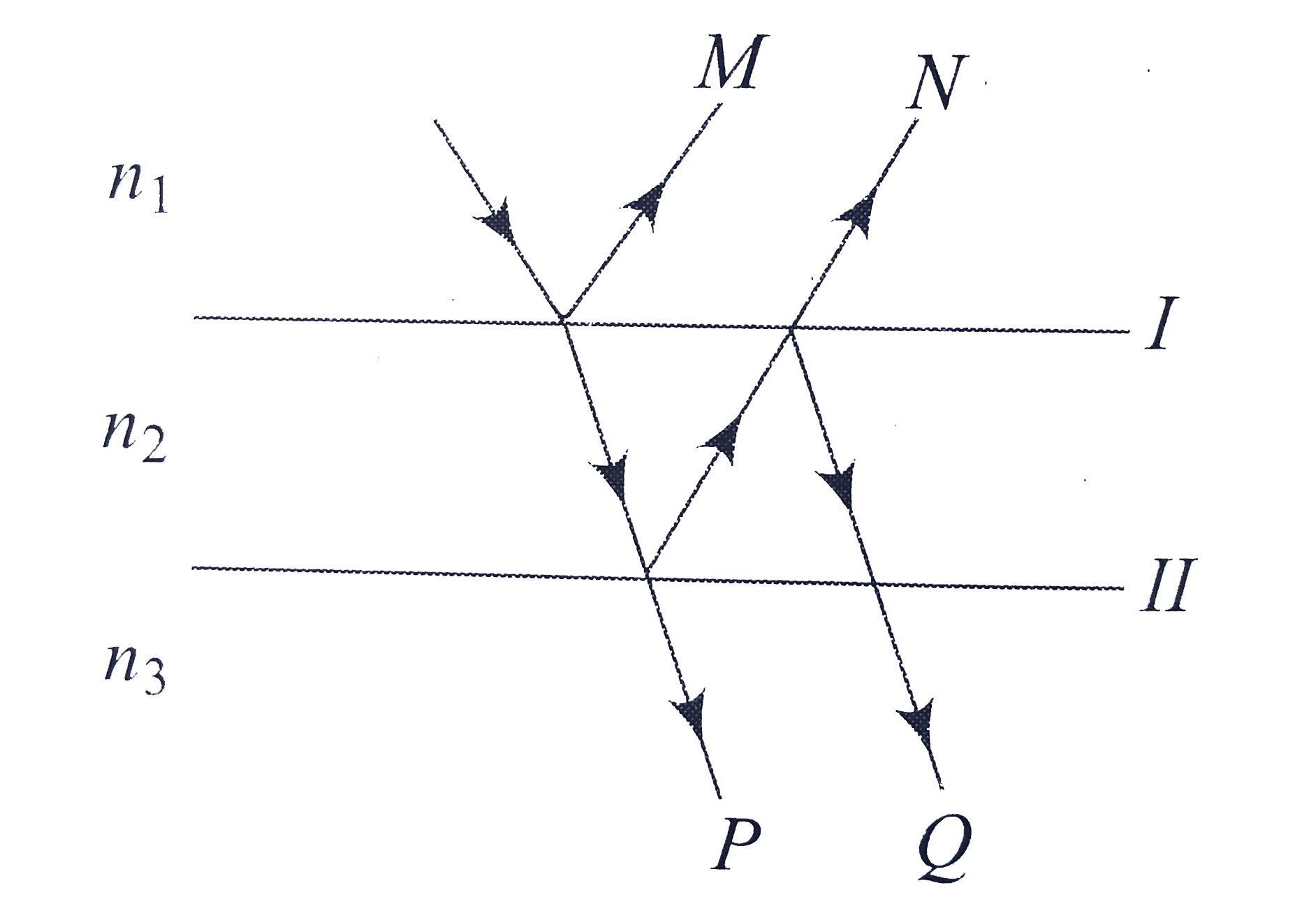
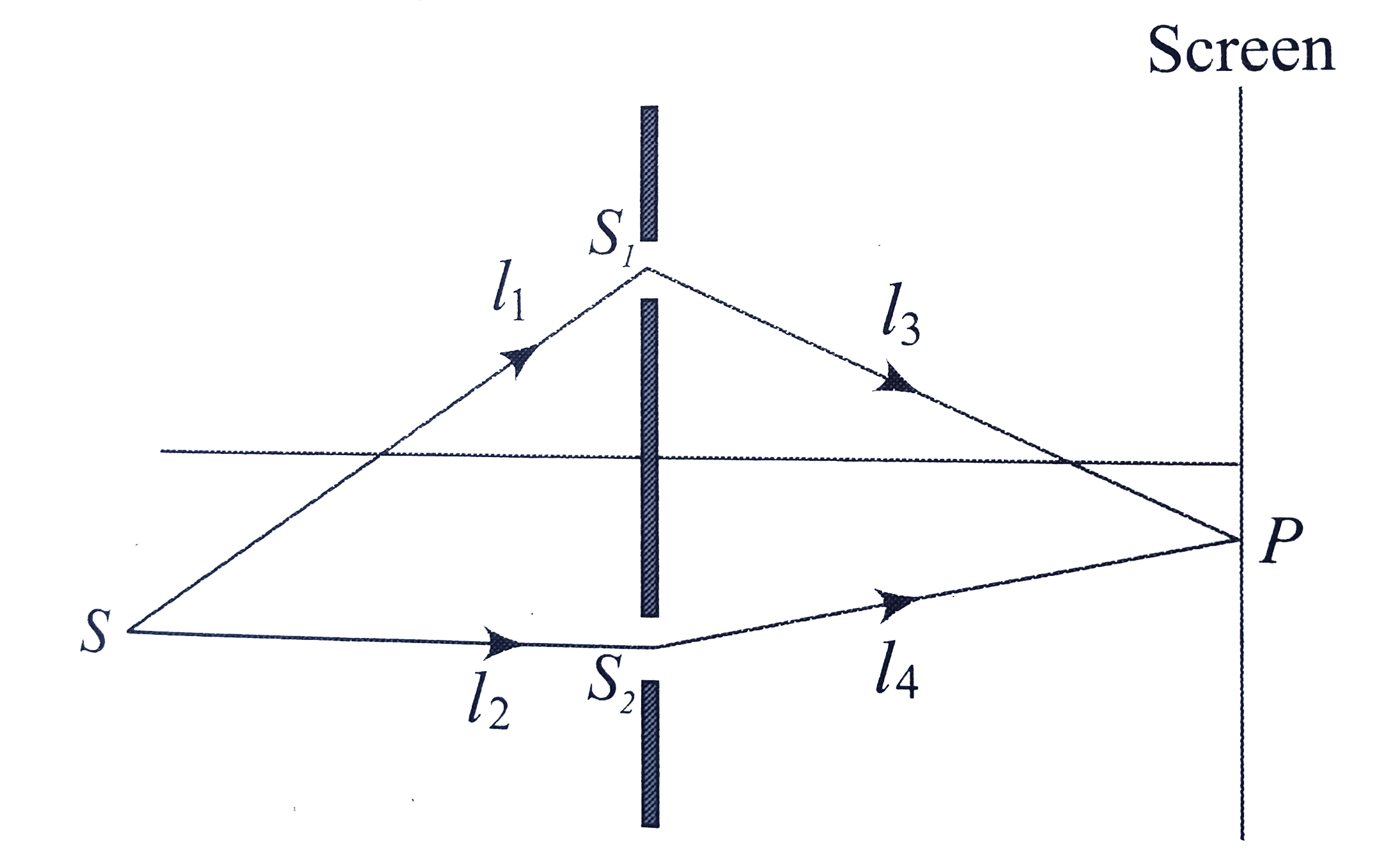
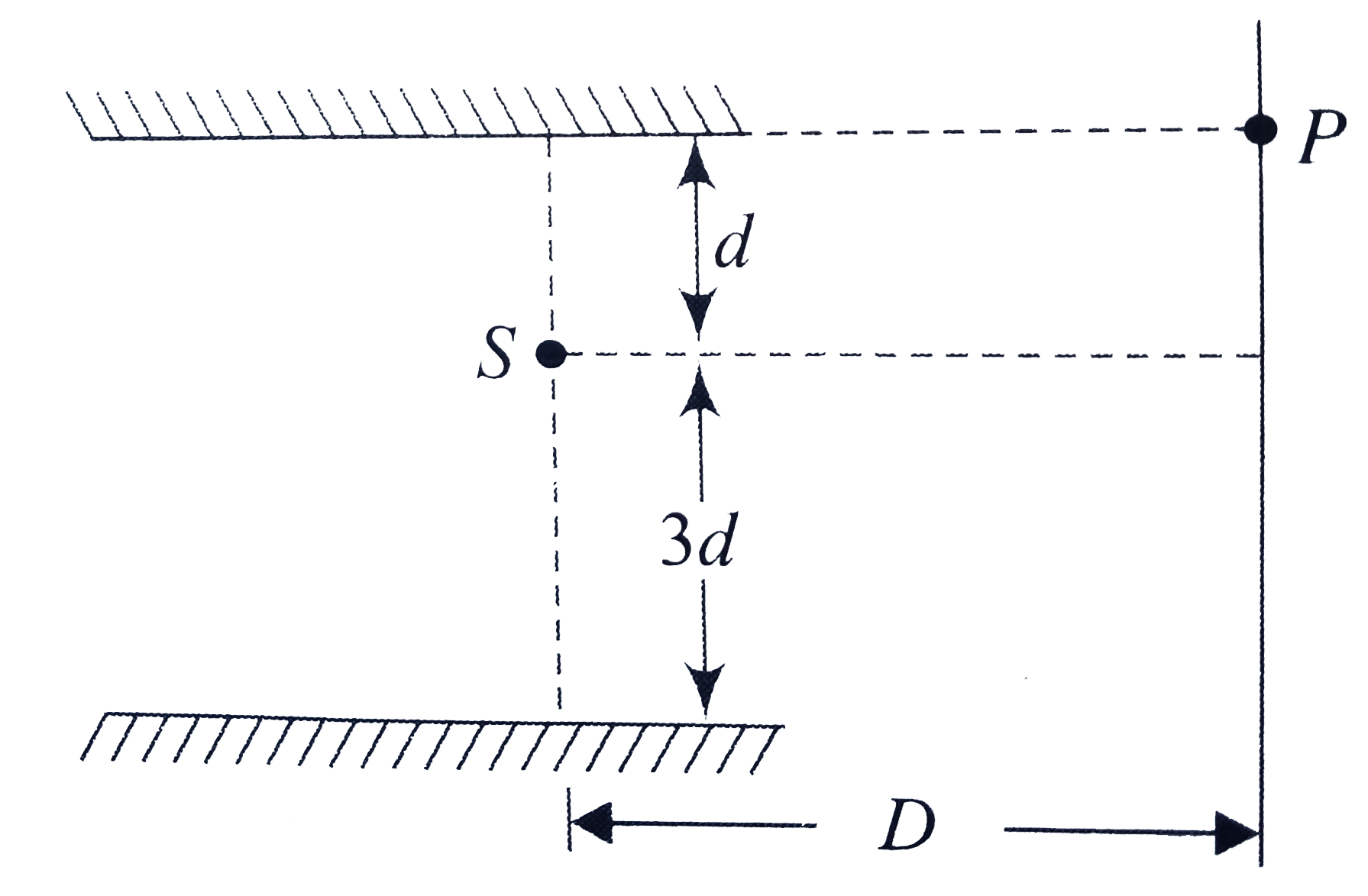
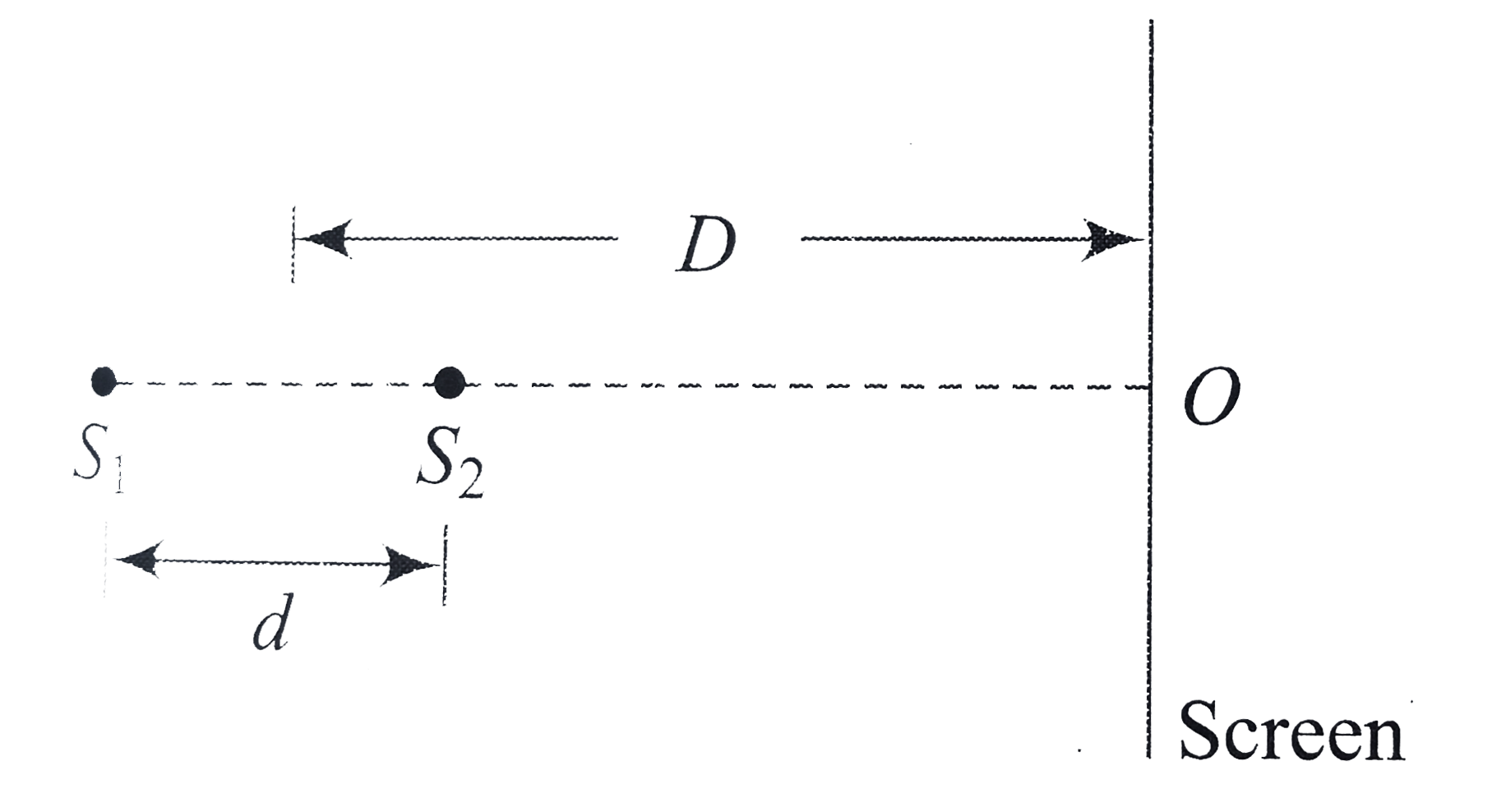

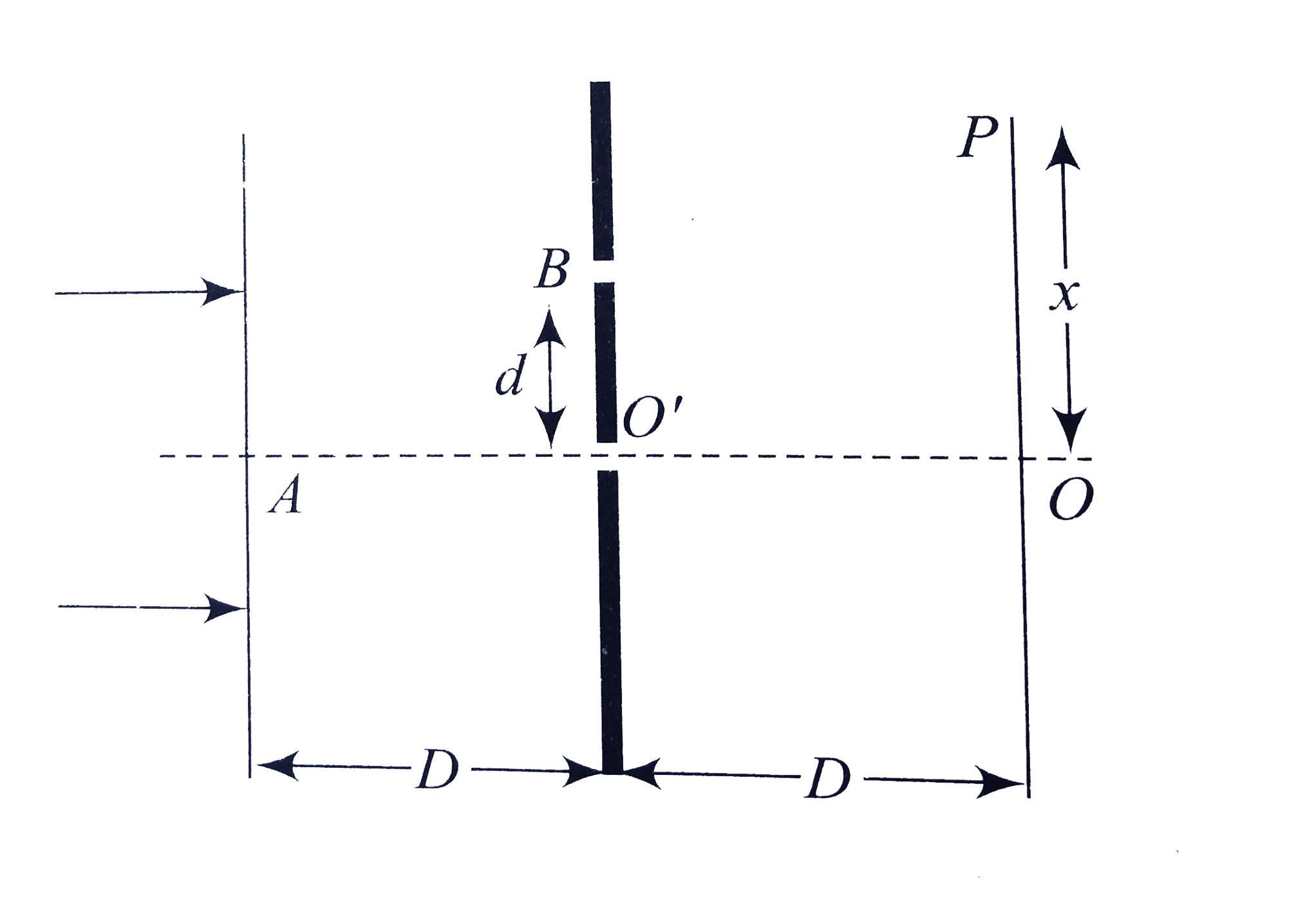
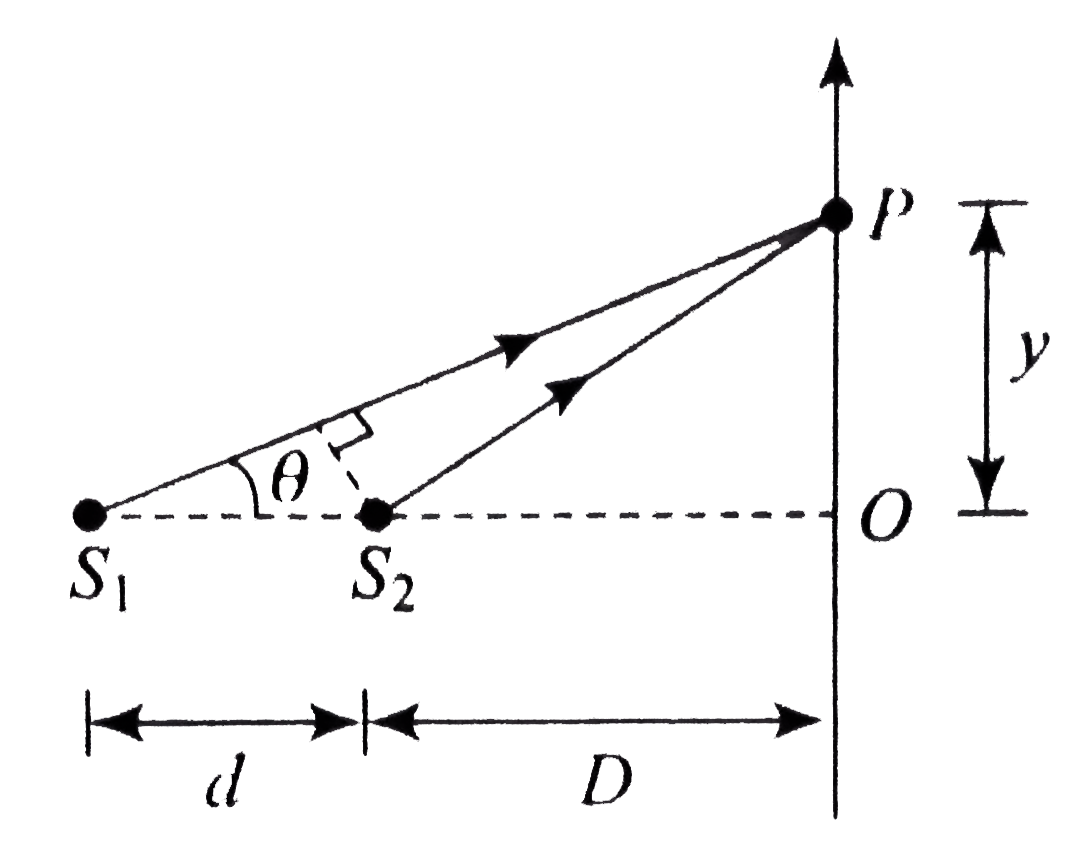
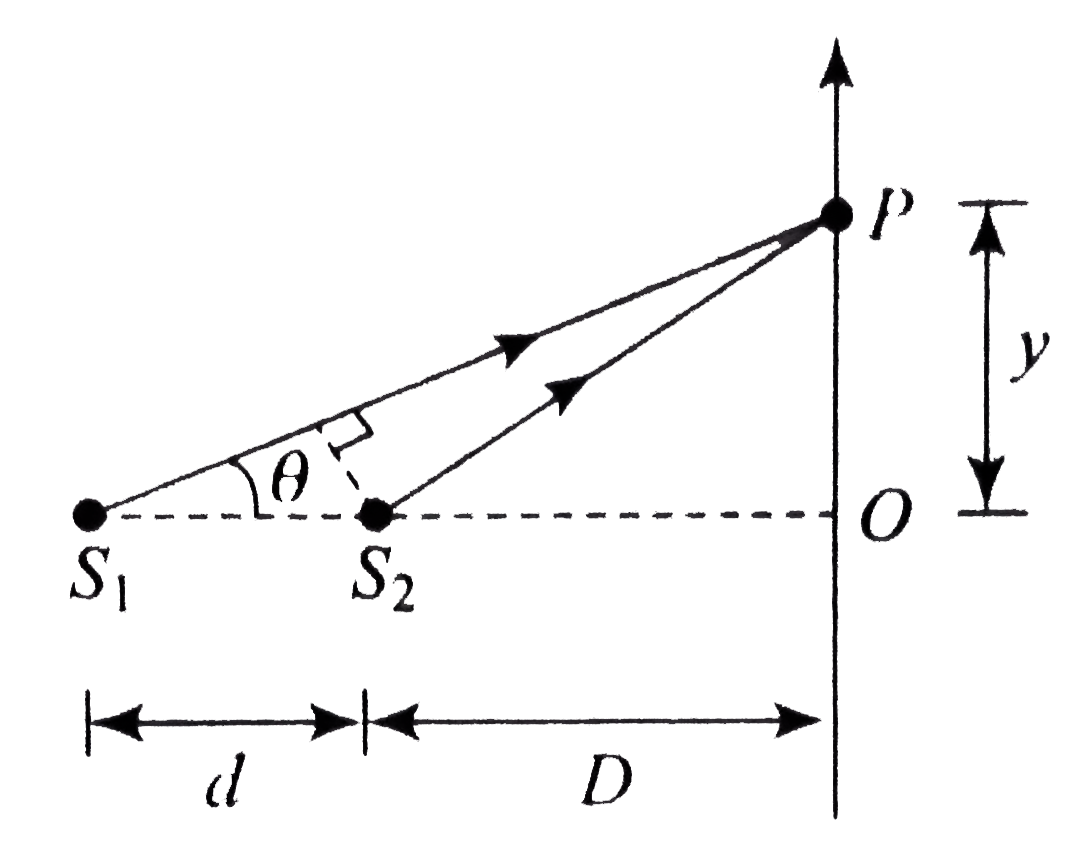
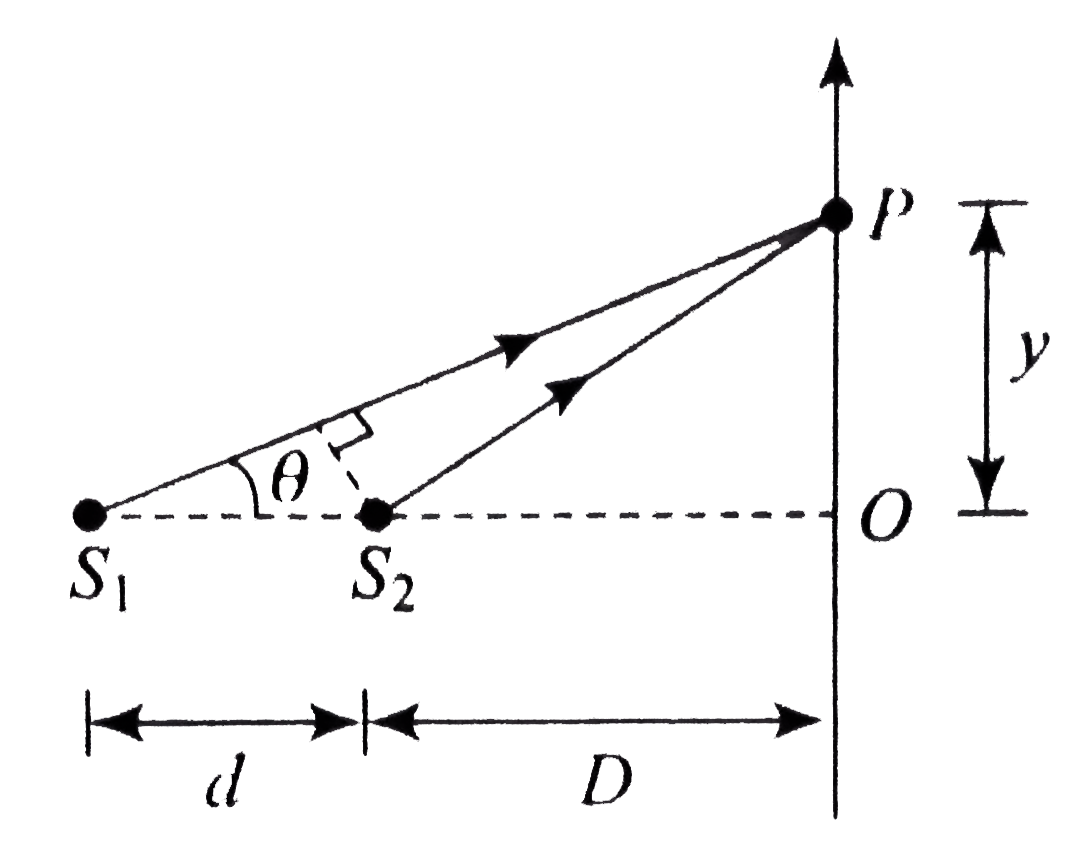


 Find the fringe width.
Find the fringe width.


_E01_080_Q01.png)
_E01_081_Q01.png)
_E01_082_Q01.png)