Complete Solutions to EXERCISE of chapter ELECTROMAGNETIC INDUCTION of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | ELECTROMAGNETIC INDUCTION |
| HC VERMA | EXERCISE |
| Number Of Questions | 98 |
| Academic Year | 2023-24 |
Calculate the dimensions of (a) `int vecE .dvecl, (b)vBl and (dPhi_0)/(dt)`. The symbols have their usual meanings.
View SolutionThe flux of magnetic field through a closed conducting loop changes with time according to the equation, `Phi = at^2 + bt+ c. (a) Write the SI units of a,b and c. (b) if the magnitudes of a,b and c are 0.20, 0.40 and 0.60 respectely, find the induced emf at t = 2 s.
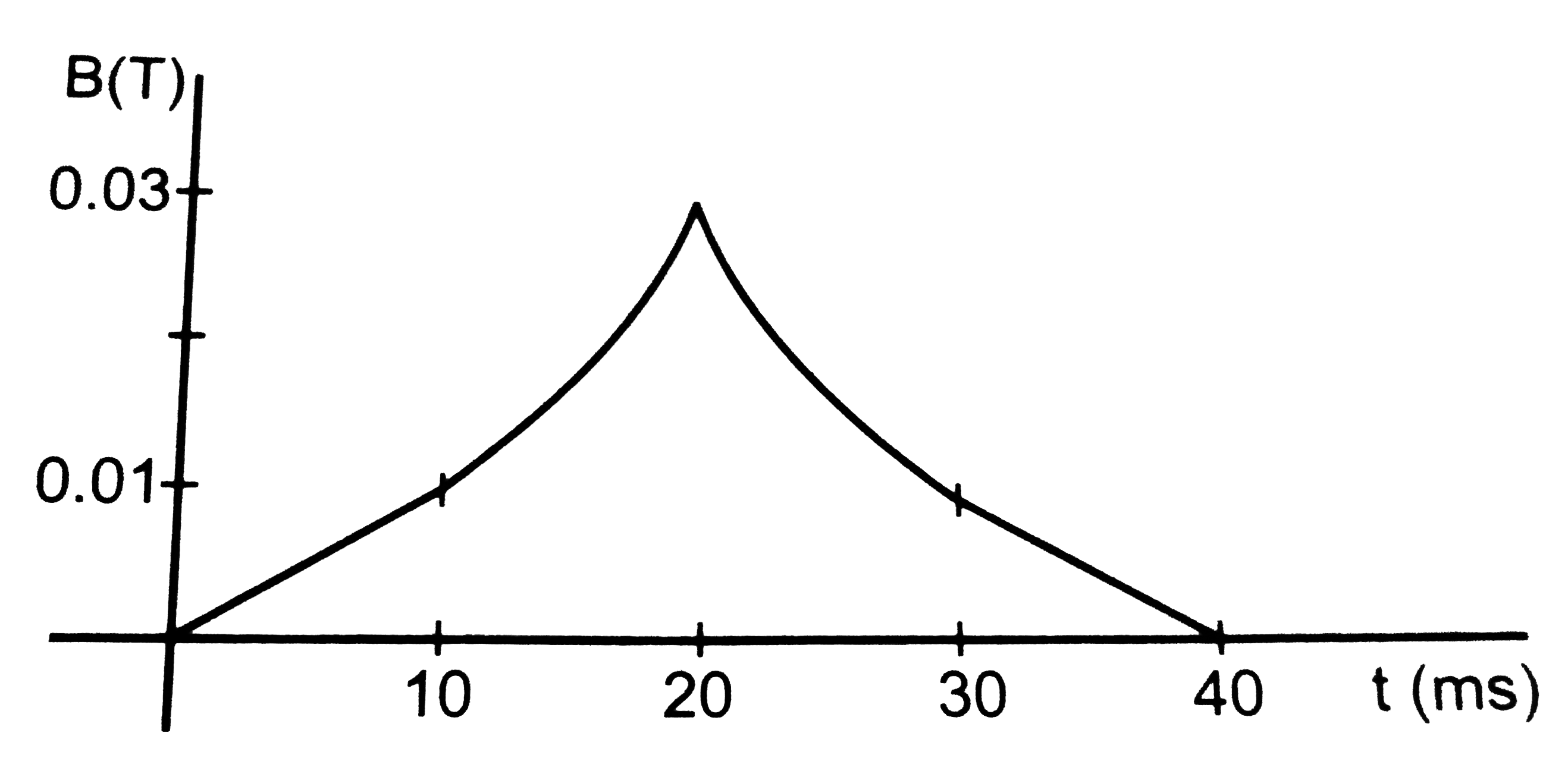
View Solution(a) the magnetic field in a region varies as shown in . Calculate the average induced emf in a conducting loop of area `2.0X10^(-3) m^2 placed perpendicular to the field in each of the10ms intervals shown. (b) In which intervals is the emf not constant? Neglect behaviour near the ends of 10 ms intervals.
View SolutionA conducintg circular loop having a redius of 5.0cm, is placed perpendicular to a magnetic field of 0.50 T. It is removed from the field in 0.50 s. Find the average emf produced In the loop during this time.
View SolutionA coducting circular loop of area `1mm^2` is placed coplanarly with a long, straight wire at a distance of 20 cm from if. The straight wire carries an electric current which changes from 10A to zero is 0.1 s. Find the average emf induced in the loop in 0.1 s.
View SolutionA square- shaped copper coil has edges of length 50cm and contains 50 turns. It is placed perpendicular to 1.0 T magnetic field. It is removed form the magnetic field in 0.25 s and restored in its joriginal place in the next 0.25 s. Find the magnitude of the average emf induced in the loop during (a) its removal, (b) its restoration and (c) its motion.
View SolutionSuppose the resistance of the coil in the previous problem is `25Omega`. Assume that the coil moves with uniform velocity during its removal and restoration. Find the thermal energy developed in the coil during (a) its removal, (b) its restoration and (c) its motion.
View SolutionA conducting loop of area `5.0 cm^2` is placed in a magnetic field which varies sinusoidally with time as `B = B_0 sin omegat` where `B_0` = 0.20 T and `omega = 300 s^(-1)`. The normal to the coil makes an angle of `60^@` with the field. Find (a) the maximum emf induced in the coil, (b) the emf induced at `t = (pi/900)s` and (c) the emf induced at ` t = (pi/600)s`.
View SolutionShows a conducting square loop placed parallel to the pole faces of a ring magnet. The pole faces have an area of 1cm^2 each and the field between the poles is 0.10 T. The wires making the loop are all outside the magnetic field. If the magnet is removed in 1.0 s, what is the average emf induced in the loop?

View SolutionA conducting square loop having edges of length2.0 cm is rotated through `180^@` about a diagonal in 0.20s. A magnetic field B exists in the region which si perpendicular to the loop in its initial position. If the average induced emf during the rotation is 20 mV, find the magnitude of the magnetic field.
View SolutionA conducting loop of face area A and resistance R is plaed perpendicular to a magnetic field B. The loop is withdrawn completely from the field. Find the charge which flows through any cross section of the wire in the process. Note that it is independent of the shape of the loop as well as the way it is withdrawn.
View SolutionA long slenoid of radius 2 cm has 100 turns/cm and carries a current of 5A. A coil of radus 1 cm having 100 turns and a total resistance of 20 Omega is placed inside the solenoid coxially. The coil is conneted to a galvanometer. If the current in the solenoid is reversed in direction, find the charge flown through the galvanometer.
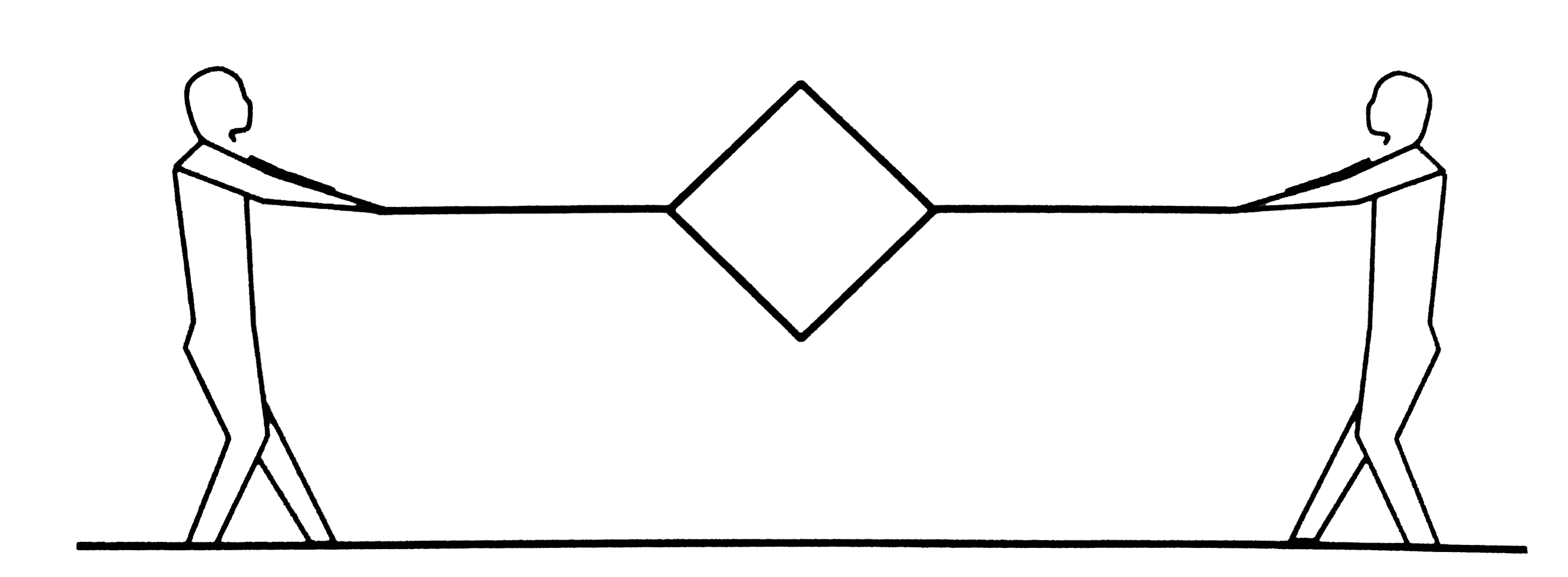
View SolutionShown a metallic square frame of edga a in a vertical plane. A uniform magnetic field B exists in the space in a directon perpendicular to the plane of the figure. Two boys pull the opposite corners of the square ot deform it into a rhombus. They start pulling the corners t t=0 and sisplace the corners at a uniform speed u. (a) find the induced emf in the frame at the instant when the angles at these corners reduce to `60^@`. (b) find the induced current in the frame at this instatnt if the total resistance of the frame is R. (c) Find the total charge which flows through a side of the frame by the time the square is deformed into a straight line.
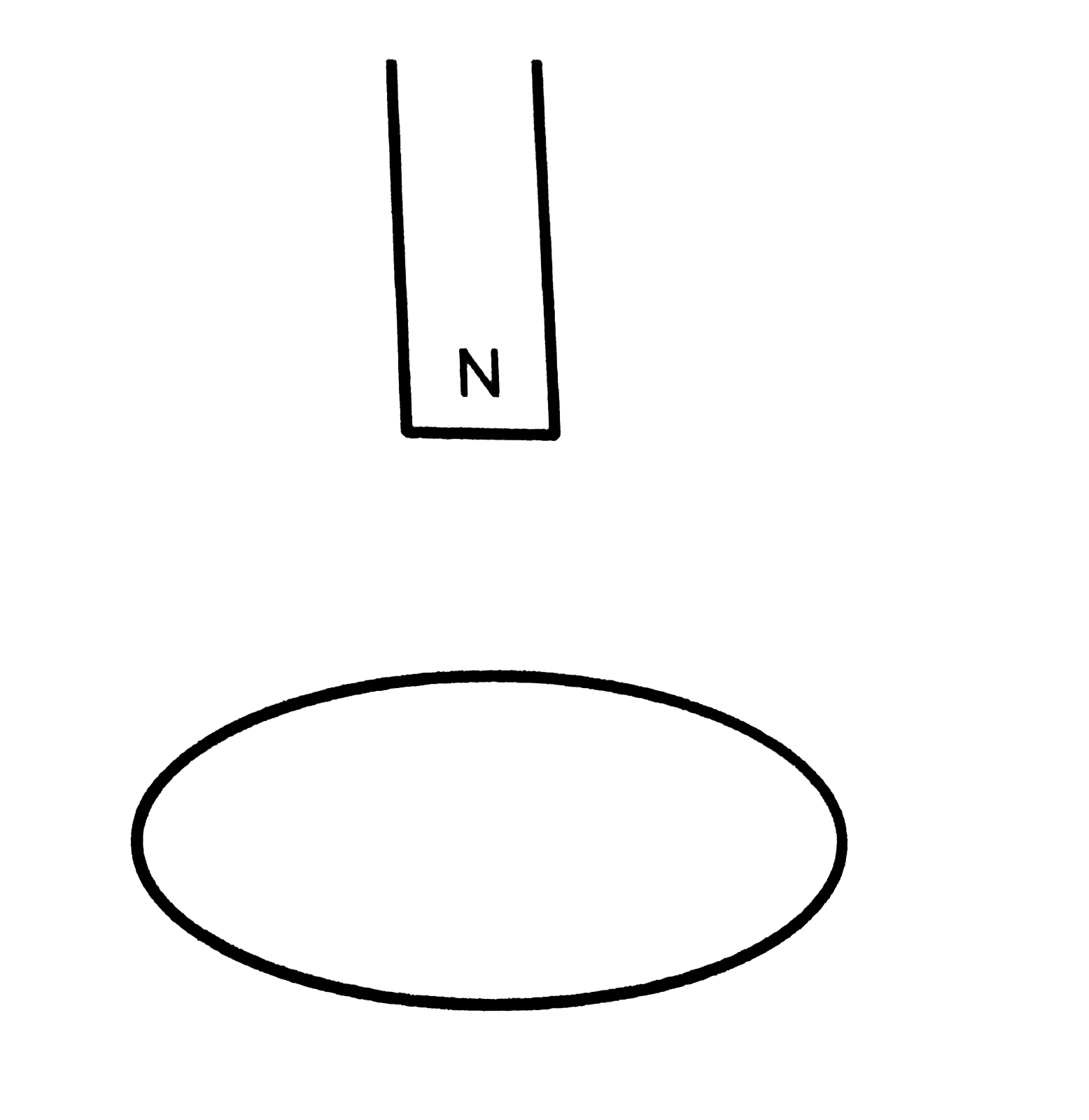
View SolutionThe north pole of a magnet is brought down along the axis of a horizontal circular coil . As a result, the flux through the coil changes from 0.35 weber to 0.85 weber in an interval of half a second. Find the average emf induced during this period. Is the induced current clockwise or anticlockwise as you look into the coil from the side of the magnet?
View SolutionA wire loop confined in a plane is rotated in its open plane with some angular velocity. A uniform magnetic field exists in the region. Find the emf induced in the loop.
View SolutionShown a square loop of side 5 cm beign moved towards right at a constant speed of 1 cm/s. the front edge enters the 20 cm wide magnetic field at ` t=0`. Find the emf induced in the loop at `(a)t =2s, (b) t =10s, (c ) t= 22s, (d)t =30s`.

View SolutionFind the total heat produced in the loop of the previous problem during the interval 0 to 30 s if the resistance of the loop is `4.5 mOmega.
View SolutionA unifrom magnetic field B exists in a cylindrical region of radius 10 cm as shown in. A uniform wire of length 80 cm and resistance 4.0 Omega is bent into a square frame and is placed with one side along a diameter of the cylindrical region. If the magnetic field increases at a constant rate of 0.010 T/s, find the current induced in the frame.
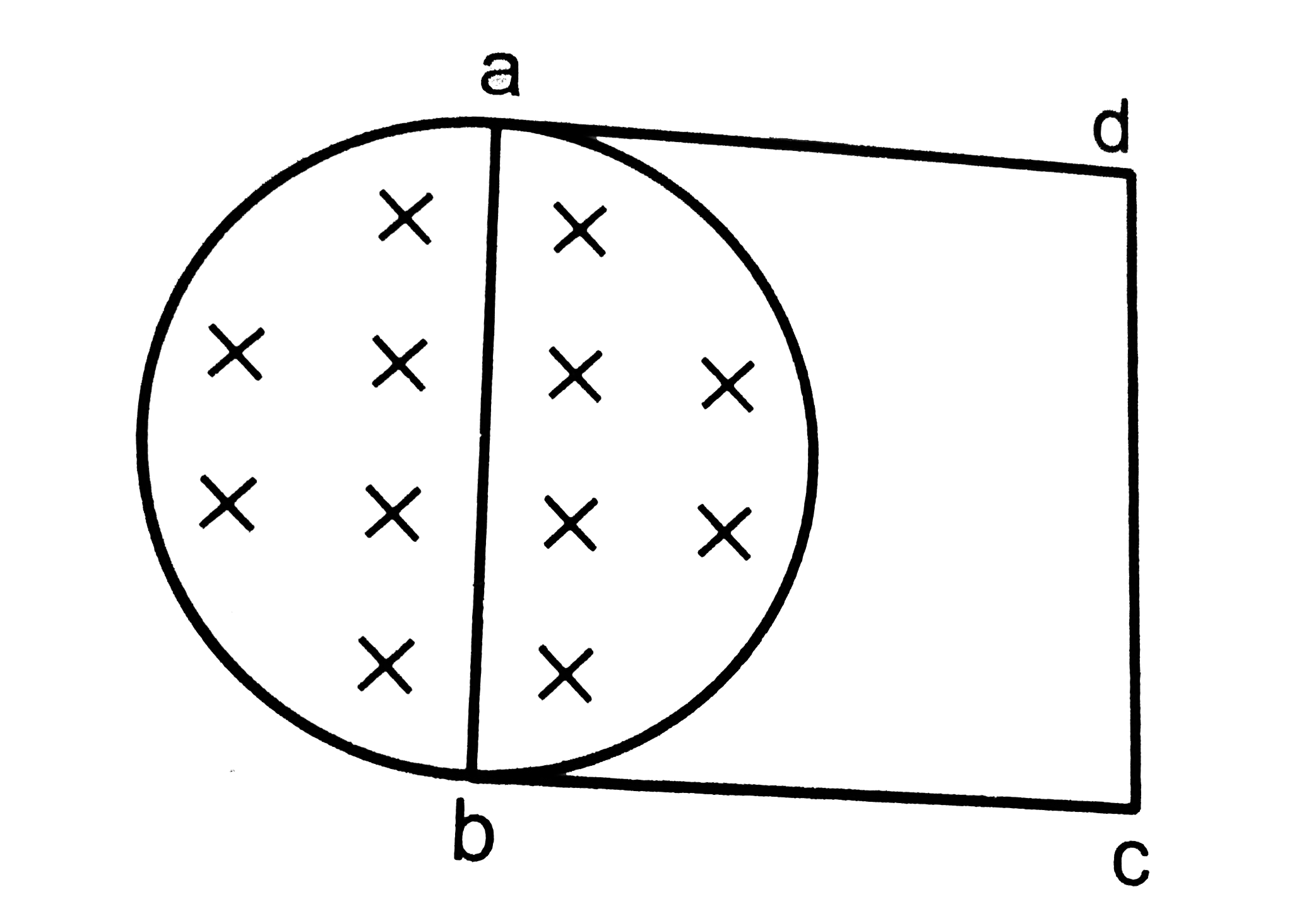
View SolutionThe magnetic field in the cylindrical region shown in increases at a constant rate of 20.0 mT/s. Each side of the square loop abcd and defa has a length of 1.00 cm and a resistance of `4.00 Omega`. Find the current (magnitude and sense) in the wire ad if (a) the switch `S_1` is closed but `S_2` is open, (b) `S_1` is open but `S_2` is closed, (c) both `S_1` and `S_2` are open and (d) both `S_1` and `S_2` are closed.

View SolutionShown a circular coil of N turns and radius a, connected to a battery of emf `epsilon` through a rheostat. The rheostat has a total length L and resistance R. The resistance of the coil is r. A small circular loop of radius a' and resistance r' is placed coaxially with the coil. The centre of the loop is at a distance x from the centre of hte coil. In the beginning, the sliding contact of the rehostat is at the left end and then on wards it is moved towards right at a constant speed v. Find the emf induced int he small circular loop at the instant (a) the contact begins to slide and (b) it has slid thrugh half the length of the rheostat.

View SolutionA circular coil of radius 2.00 cm has 50 turns. A uniform magnetic field B= 0.200 T exists in the space is a direction parallel to the axis of the loop. The coil is now rotated about a diameter through an angle of `60.0^@`. The operation takes 0.100 s. (a) find the average emf induced in the coil. (b) if the coil is a closed one(with the two ends joined together) and has a resistance of `4.00 Omega`. calculate the net charge crossing a cross- section of the wire of the coil.
View SolutionA closed coil having 100 turns is rotated in a uniform magnetic field `B = 4.0 X 10^(-4)` T about a diameter which is perpendicular to the field. The angular velocity of rotation is 300 revolutions per minute. The area of the coil is `25 cm^2` and its resistance is `4.0 Omega.` Find (a) the average emf developed in the half a turn form a position where the coil is perpendicular to the magnetic field, (b) the average emf in a full turn and (c) the net charge displaced in part (a).
View SolutionA coil of radius 10 cm and resistance 40 Omega has 1000 turns. It is placed with its plane vertical and its axis parallel to the magnetic meridian. The coil is connected to a galvanometer and is rotated about the vertical diameter through an angle of `180^@`. Find the charge which flows through the galvanometer if the horizontal component of the earth's magnetic field is `B_H = 3.0X 10^(-5) T`.
View SolutionA circular coil of one turn of radiius 5.0 cm is rotated about a diameter with a constatn angular speed of 80 revolutions per minute. A uniform magnetic field B =0.010 T exists in a directon perpendicular to the axis of rotation. Find (a) the maximum emf induced, (b) the average emf induced in the coil over a long period and (c ) the average of the squares of emf induced over a long period.
View Solutionsuppose the ends of the coil in the previous problem are connected to a resistane of `100 Omega`. Neglecting the resistance of the coil, find the heat produced in the circuit in one minute.
View Solutionshows a circular wheel of radius 10.0 cm whose upper half, shown dark in the figure, is made of iron and the lower half of wood. The two junctions are joinded by an iron rod. A uniform magnetic field B of magnitdue 2.00X 10^(-4) T exists in the space above the central line as suggested by the figure. The wheel is set into pure rolling on the horizontal surface. The wheel is set into pure rolling on the horizontal surface. If it takes 2.00 seconds for the iron part to come down and the wooden part to go up, find the average emf induced during this period.

View SolutionA 20 cm long conducting rod is set into pure translation with a uniform velocity of `10cm s ^(-1) perpendicular to its length. A uniform magnetic field of magnitude 0.10 T exists in a direction perpendicular to the plane of motion. Find emf developed in the rod.
View SolutionA metallic metre sitck moves with a velocity of ` 2 ms ^(-1) in a direction perpendicular to its length and perpendicular to a uniform magnetic field of magnitude 0.2 T. Find the emf indcued between the ends of the stick.
View SolutionA 10 m wide spaceraft moves through the interstellar space at a speed `3X10^7 ms^(-1)`. A magnetic field `B= 3 X 10^(-10) T` exists in the space in a direction perpendicular to the plane of motion. Treating the spacecraft as a conductor, calculate the emf induced across its width.
View SolutionThe two rails of a railway track, insulataed form each other and from the ground, are connected to a millivoltmeter. What will be the reading of the millivoltmeter when a train travels on the track at a speed of `180km h^(-1)? The vertical component of earth's magnetic field is 0.2 X 10^(-4) and the rails are separted by 1m.
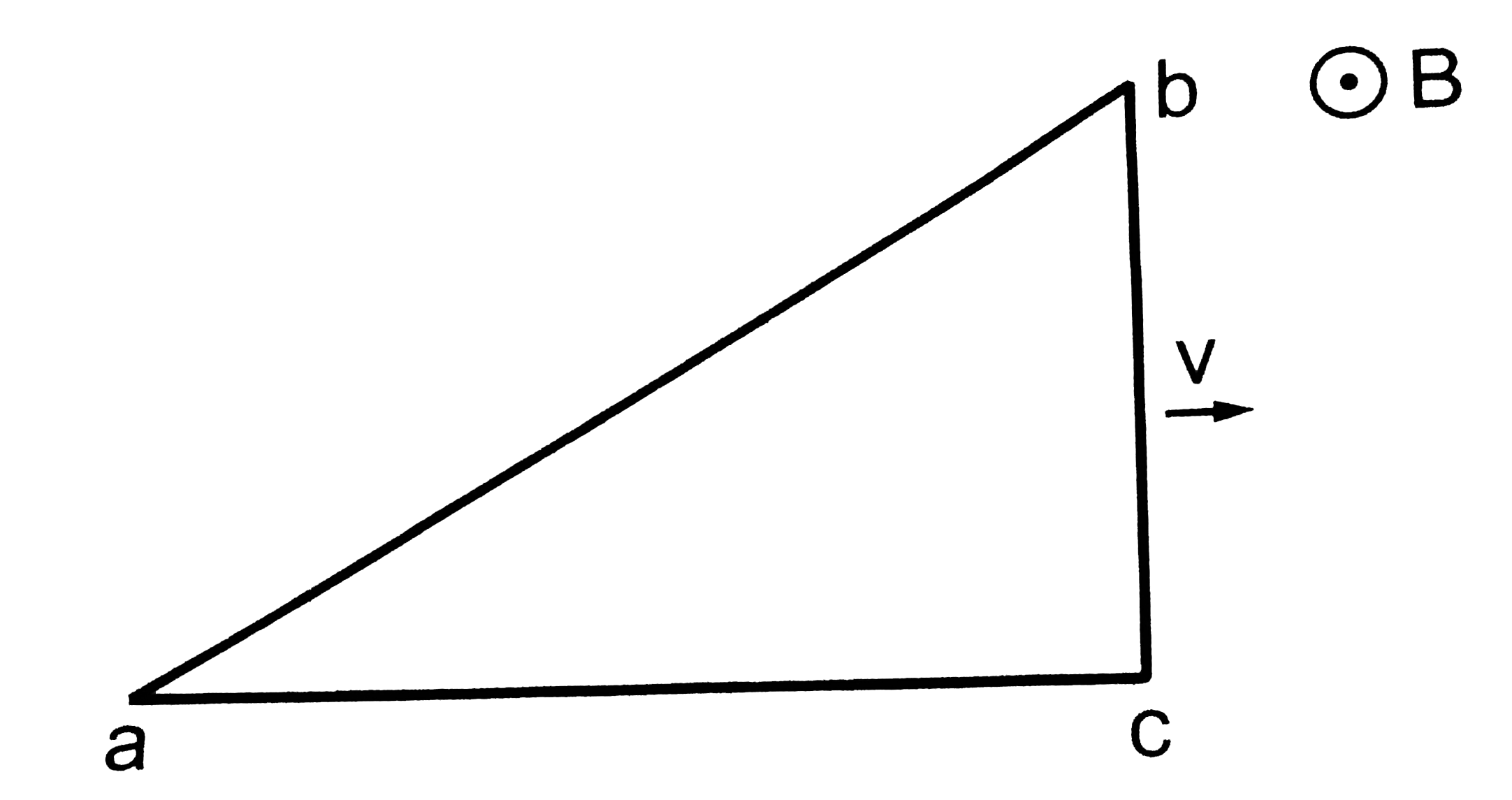
View SolutionA right angled triangle abc, made from a metallic wire, moves at a uniform speed v in its plane as shown in . A uniform magnetic field B exists in the perpendicular direction. Find the emf induced (a) in the loop abc, (b) in the segment bc, (c ) in the segment ac and (d) in the segment ab.
View SolutionA copper wire bent in the shape of a semicircle of radius r translates in its plane with a constant velocity v. A uniform magnetic field B exists in the direction perpendicular to the plane of the wire. Find the emf induced between the ends of the wire if (a) the velocity is perpendicular ot the diameter joining free ends, (b) the velocity is parallel to this diameter.
View SolutionA wire of length 10cm translates in a direction making an angle of `60^@` with its length. The plane of motion is perpendicular ot a uniform magnetic field of 1.0 T that exists in the space. Find the emf induced between the ends of the rod if the speed of translation of `20 cm s^(-1).
View SolutionA circular copper ring of radius r translates in its plane with a constant velocity v. A uniform magnetic field B exists in the space in a direction perpendicular of the plane o the ring. Consider different pairs of diametrically opposite points on the ring. (a) Between which pair of points is the emf? (b) Between which pair of points is the emf minimum? What is the value of this minimum emf?
View SolutionShows a wire sliding on two parallel, conducting rails placed at a separaton l. A magnetic feld B exists in a direction perpendicular to the plane of the rails. What force is necessary to keep the wire moving at a constatn velocity `v` ?
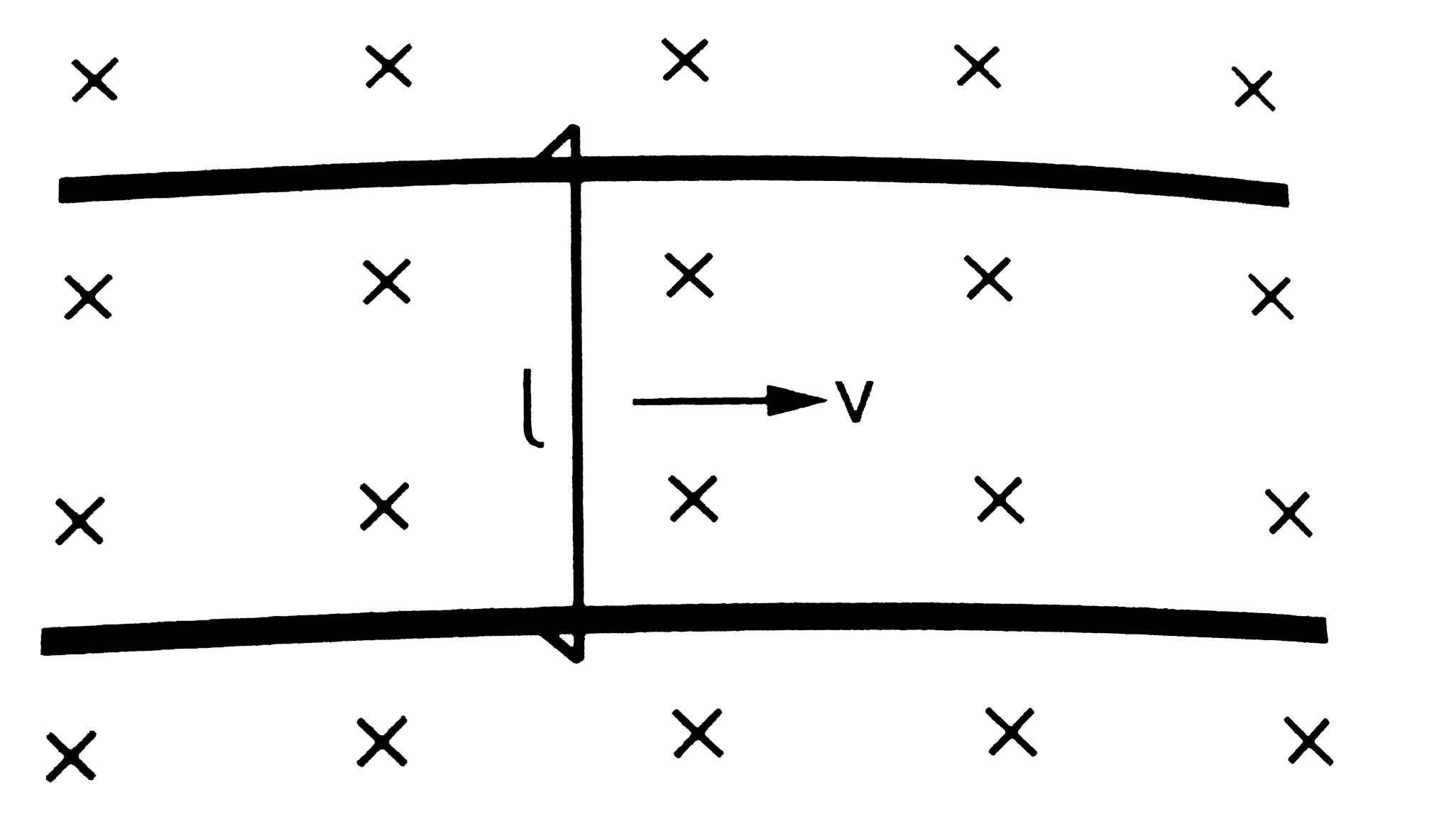
View Solutionshows a long U shaped wire of widt l placed in a perpendicular magnetic field B. A wire of length l is slid on the U shaped wire with a constant velocity v towards right. The resistance of all the wires is r per unit length. At t =0, the sliding wire is close to the left edge of the U shaped wire. Draw an equivalent circuit diagram, showing the induced emf as a battery. Calculate the current in the circuit.
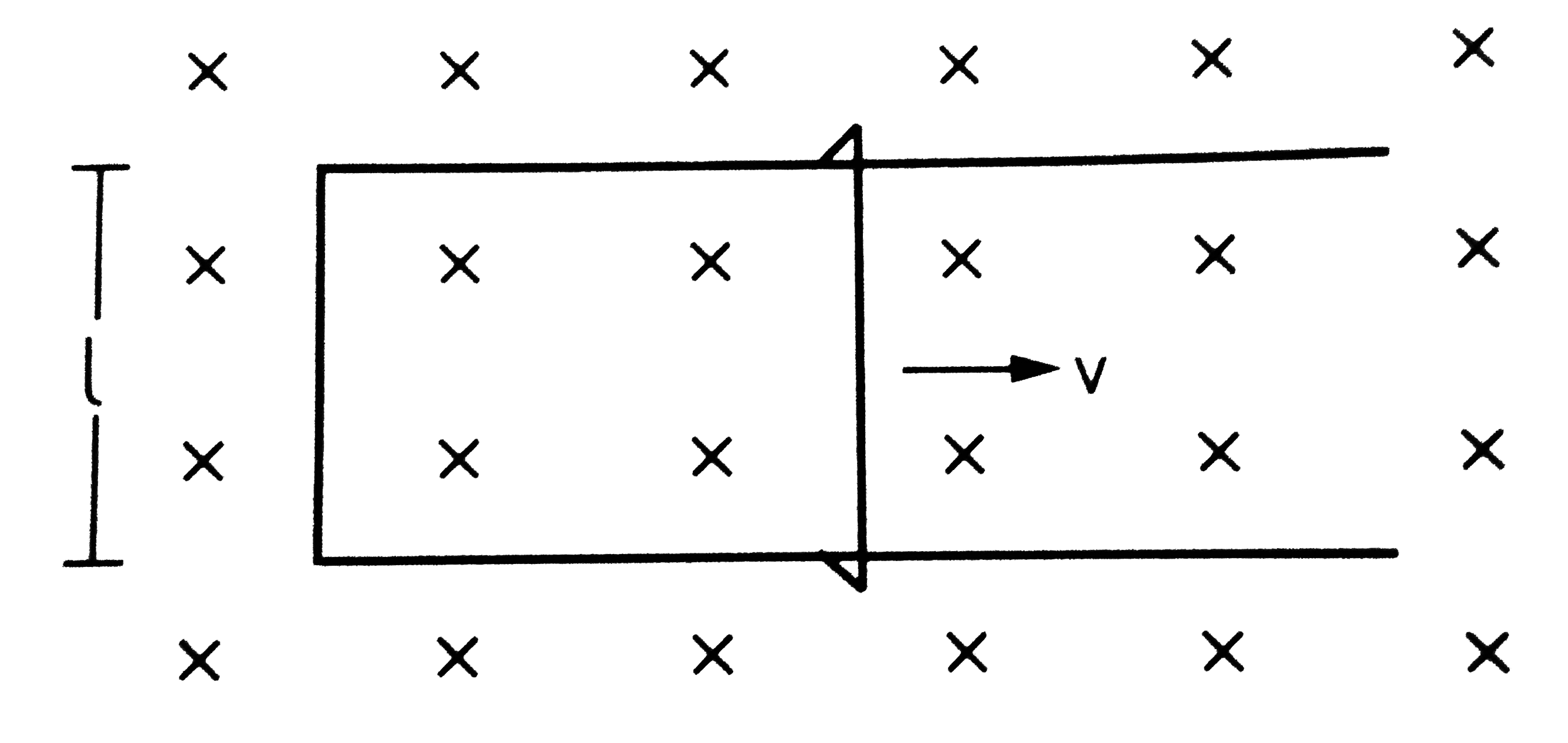
View SolutionConsider the situation of the previous problem. (a) Calculate the force needed to keep the sliding wire moving with a constant velocity v. (b) If the force needed just after t = 0 is `F_0`, find the time at which the force needed will be `F_0` /2.
View SolutionConsider the situation shown in . The wire PQ has mass m, resistance r and can slide on the smooth, horizontal parallel rails separted by a distance l. The resistance of the rails is negligible. A uniform magnetic field B exists in the rectangualr region and a resistance R connects the rails outsided the field region At t= 0, the wire PQ is puched towards right with a speed `v_0`. find (a) the current in the loop at an instant when the speed of the wire PQ is v, (b) the acceleration of the wire at this instatn, (c ) the velocity v as a function of x and (d) the maximum distance the wire will move.

View SolutionA rectanguar frame of wire abcd has dimensions 32cm X 8.0 cm and a total resistance of 2.0 `Omega`. It is pulled out of a magentic field B = 0.020 T by applying a force of `3.2 X 10^(-5)` N . It is found that the frame moves with constant speed. Find (a) this constant speed, (b) the emf induced in the loop, (c) the potential difference between the points c and d.

View SolutionShows a metallic wire of resistance `0.20 Omega` sliding on a horizontal, U shaped metallic rail The separation between the parallel arms is 20 cm. jAn electric current of `2.0 mu A` passes through the wire when it is slid at a rate of `20cm s ^(-1)`. If the horizontal component of the earth's magnetic field is `3.0 X 10^(-5)` T, calculate the dip at the place.
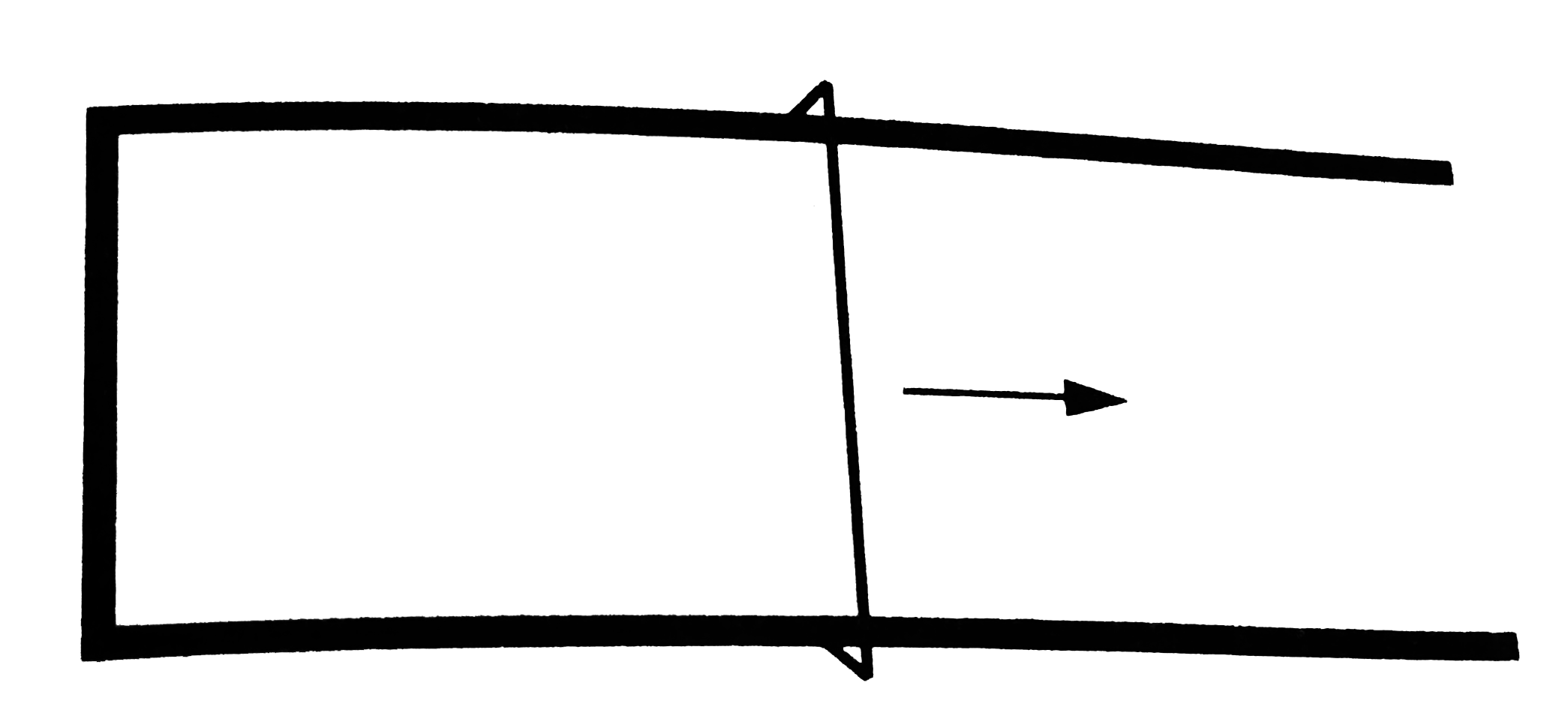
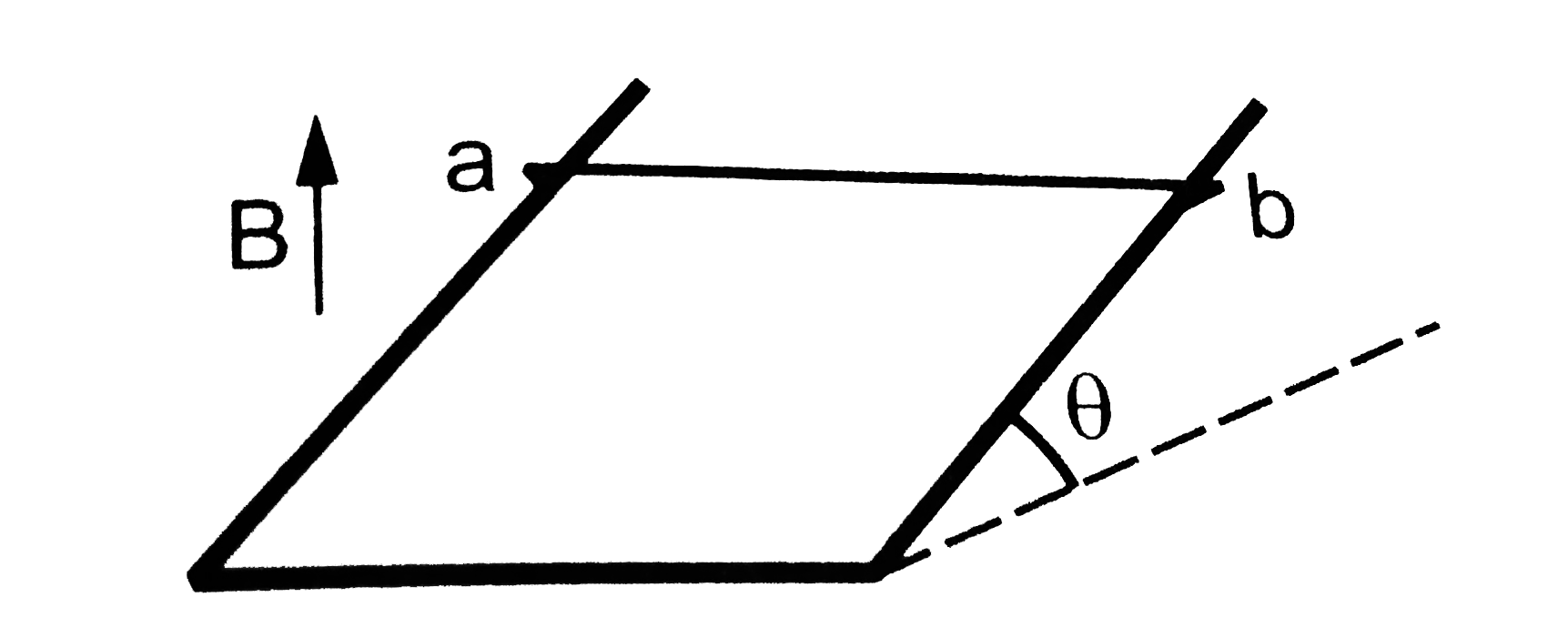
View SolutionA wire ab of length l, mass m and resistance R slided on a smooth, thick pair of metallic rails joined at the bottom as shown in . The plane of the the rails makes an angle `theta` with the horizontal. A vertical magnetic field B exists in the ragion. if the wire slides on the rails at a constant speed v, show that `B = sqrt(mg R sin theta)/(vl^2 cos^theta)`.
View SolutionConsider the situation shown in figure. The wires P1Q1 and P2Q2 are made to slide on the rails with the same speed 5cm/sec . Find the electric current in the 19Ω resistor if (a) both the wires move towards right and (b) if P 1Q1 moves towards left but P2Q2 moves towards right.
View SolutionSuppose the `19 Omega` resistor of the previous problem is disconnected. Find the current through `P_2 Q_2` in the two situations (a) and (b) of that problem.
View SolutionConsider the situation shown in . The wire PQ has a negligible resistance and is made to slide on the three rails with a constant speed of `5 cm s^(-1)` . Find the current in the `10 Omega` resistor when the switch S is thrown to (a) the middle rail (b) the bottom rail.

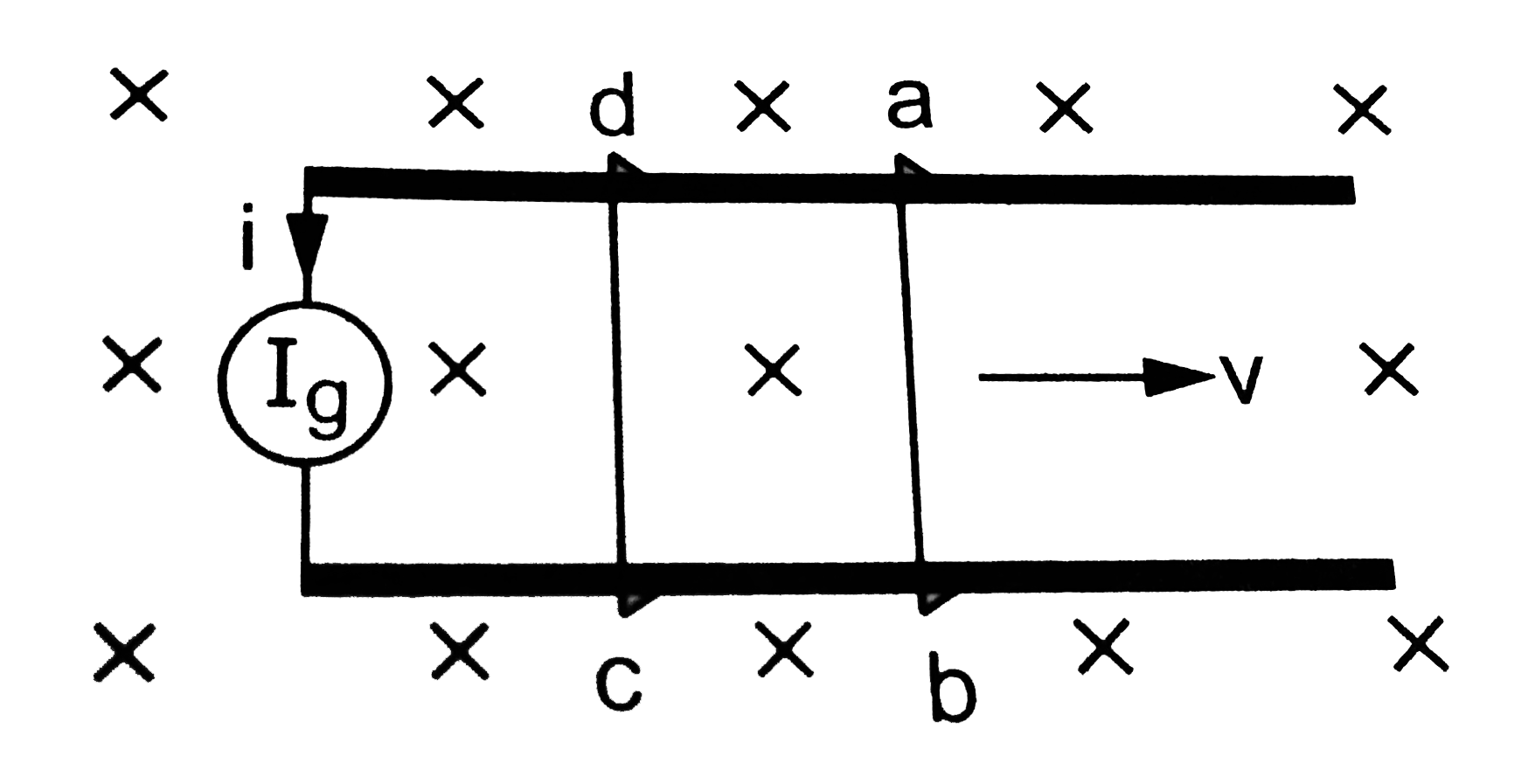
View SolutionThe current generator `I _g`, shown in . Sends a constant current I throught the curcuti. The wire cd is fixed and ab is made ot slide on the smooth, thick rails with a constant velocity v towards right. Each of these wire has resistance r. Find the current through the wire cd.
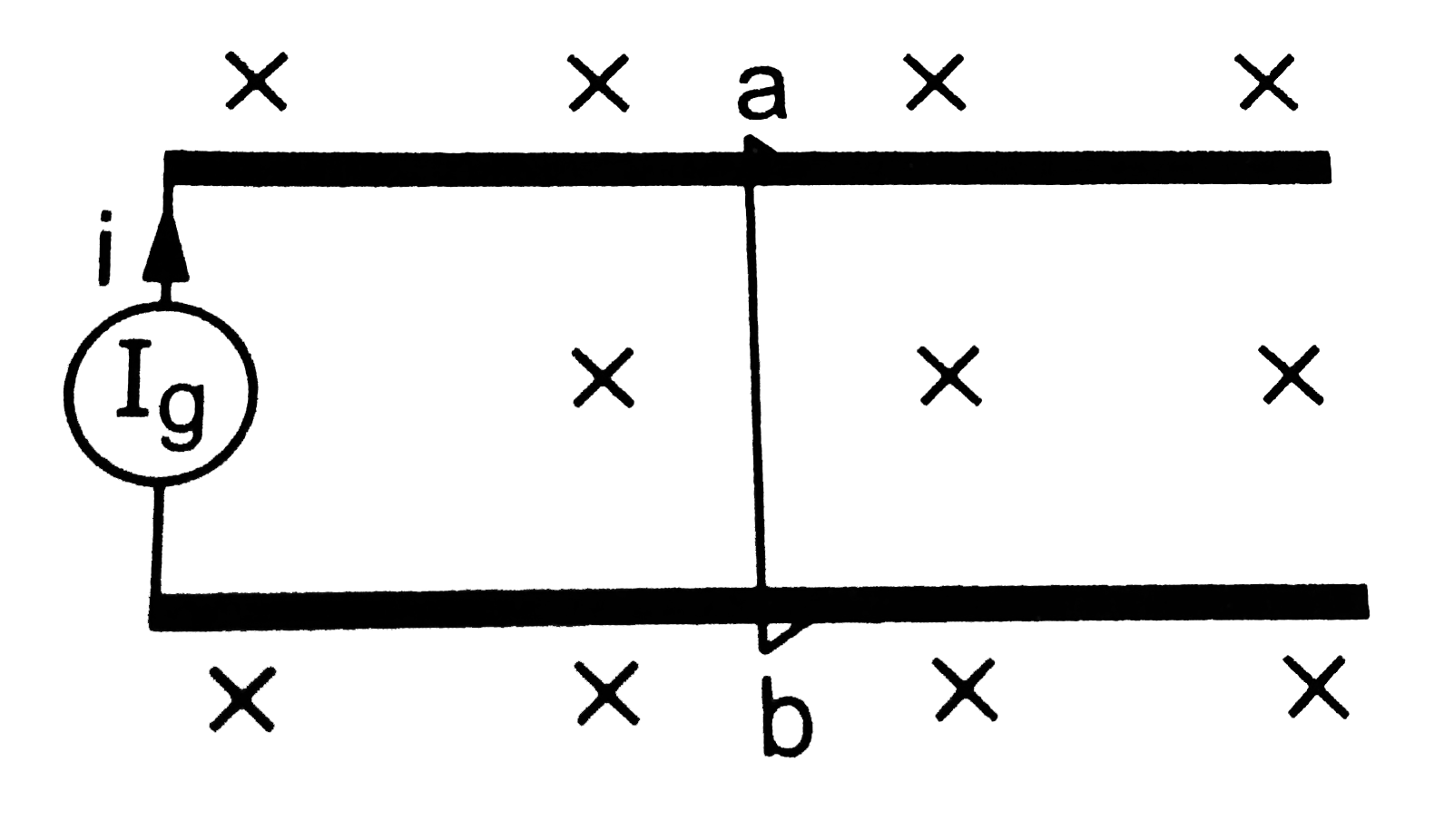
View SolutionThe current generator `i_g`, shown in , sends a constant current I through the circuit. The wire ab has a length l and mass m and can slide on the smooth, horizontal rails connected to `l_g`. The entire system lies in a vertical magnetic field B. Find velocity of the wire as a function of time.
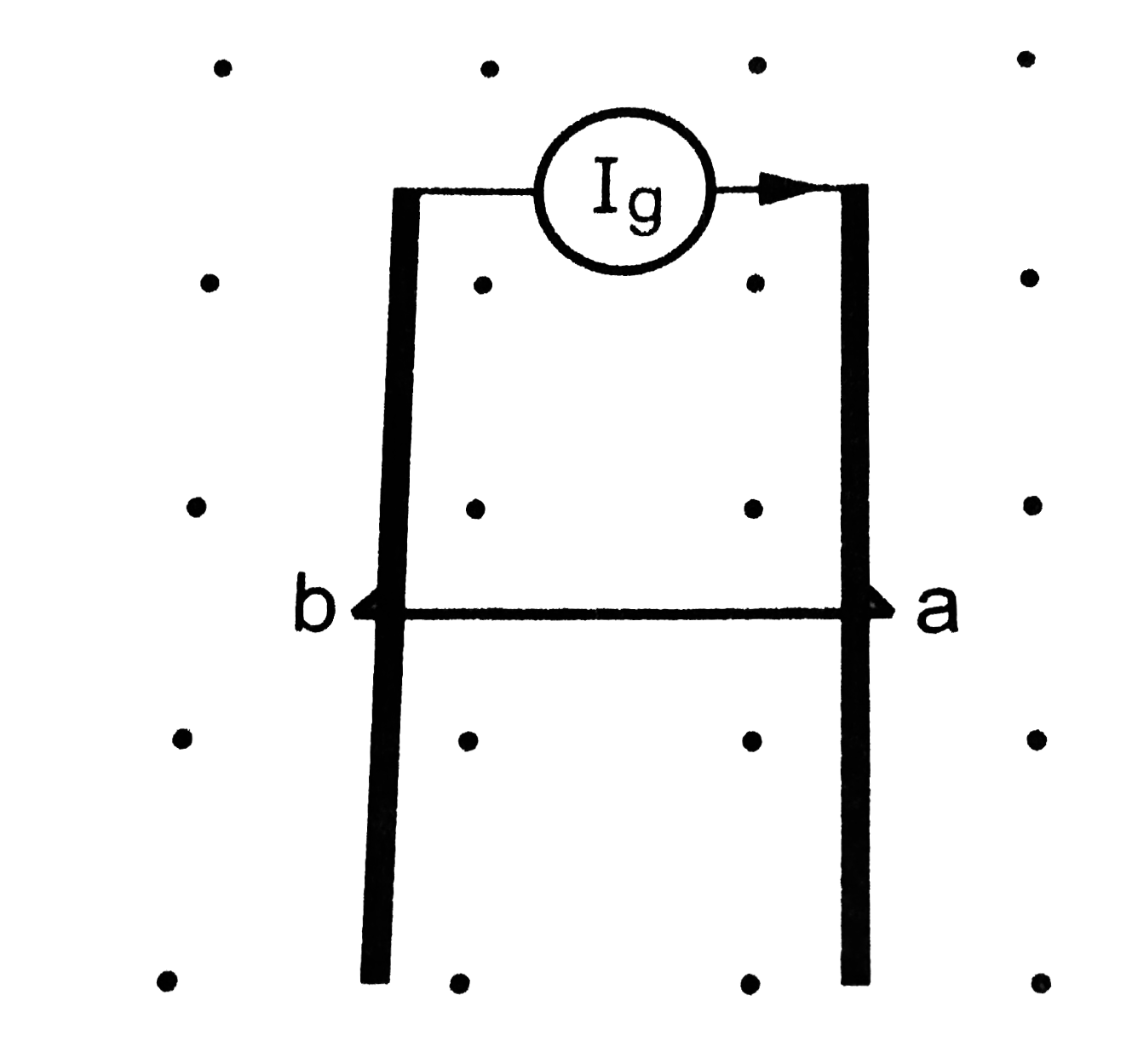
View SolutionThe system containing the rails and the wire of the previous problem is kept vertically in a uniform horizontal magnetic field B that is perpendicular to the plane of the rails. It is found that the wire stays in equilibrium. If the wire ab is replaced by another of double its mass, how long will it take in falling through a distance equal ot its length?
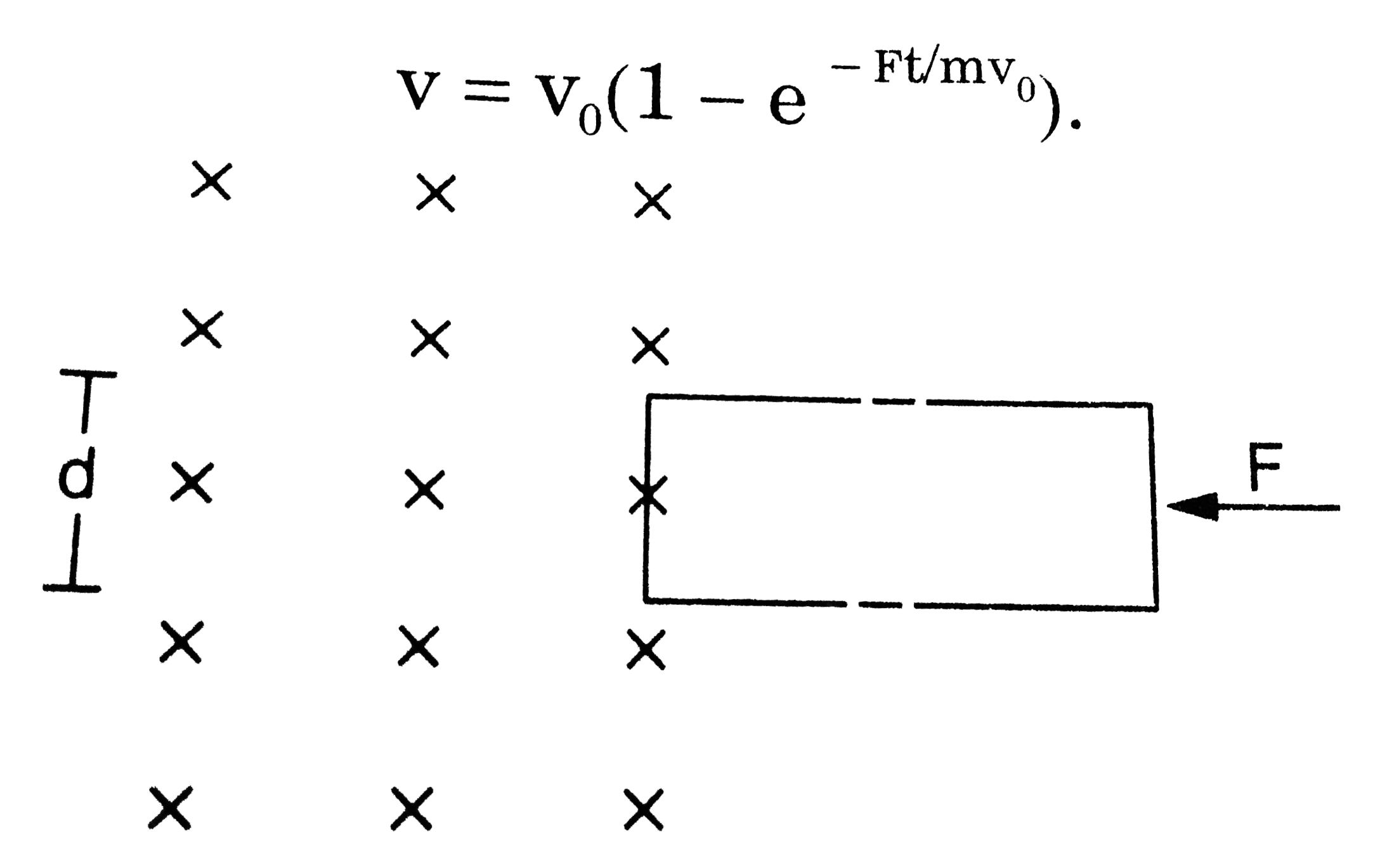
View SolutionThe rectangualr wire- frame, shown in has a width d, mass m, resistance R and a large length. A uniform magnetic field B exists to the left of the frame. A constant force F starts pushing the frame into the magnetic field at t =0. (a) Find the acceleration of the frame when its speed has increased to v. (b) Show that after some time the frame will move with a constant velocity till the whole frame enters into the magnetic field. find this velocity `v_0`. (c) show that the velocity at tiem t is given by ` v= v_0(1 - e^(-Ft/mv_0)`.
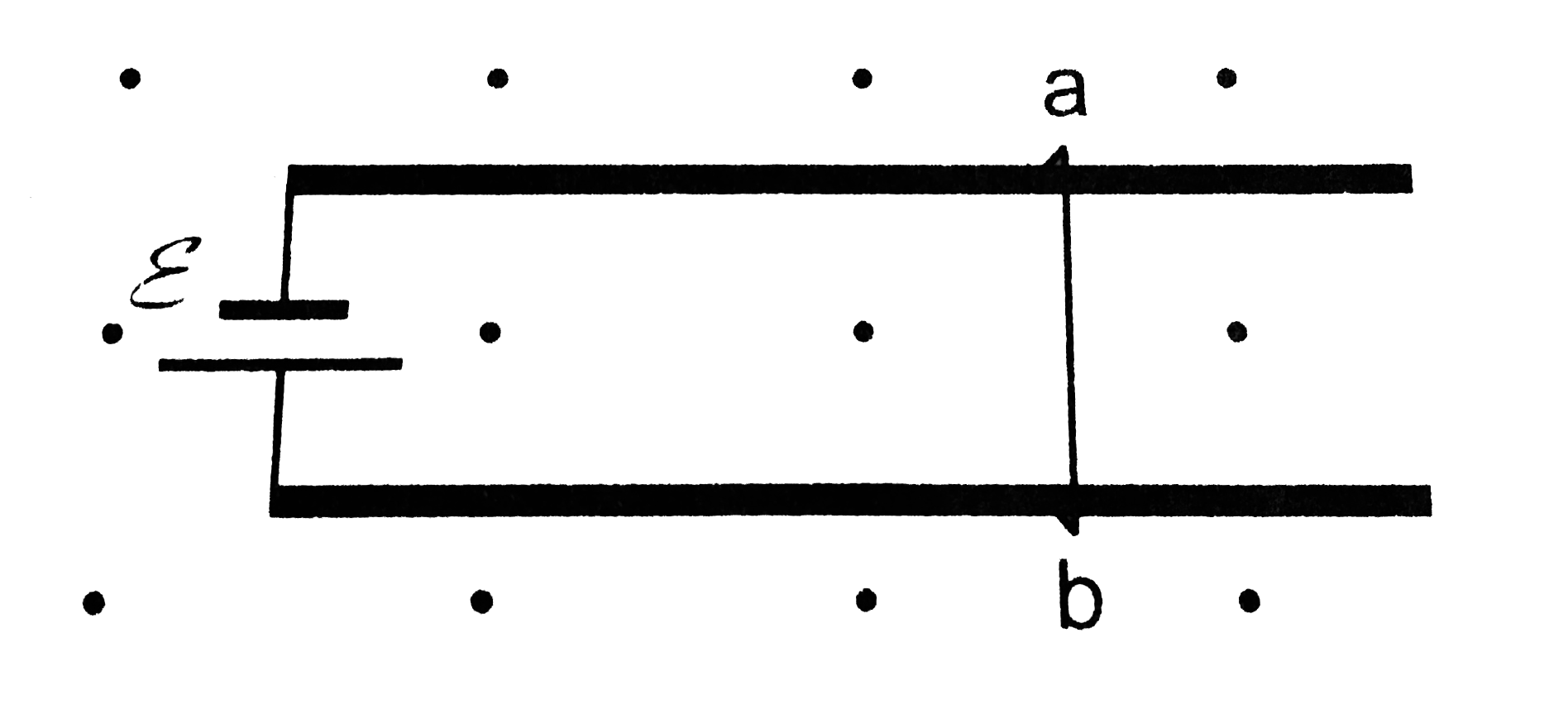
View SolutionShows a smooth pair of thick metallic rails connected across a battery of emf `epsilon` having a negligible internal resistance. A wire ab of length l and resistance r can slide smoothly on the rails. The entire system lien in a horizontal plane and is immersed in a uniform vertical magnetic field B. At an instant t, the wire is given a small velocity v towards right. (a) find the current in it at this instant. What is the direction of the current? (b) What is the force acting on the wire at this instant? (c) Show that after some time the wire ab will slide with a constant velocity. Find this velocity.
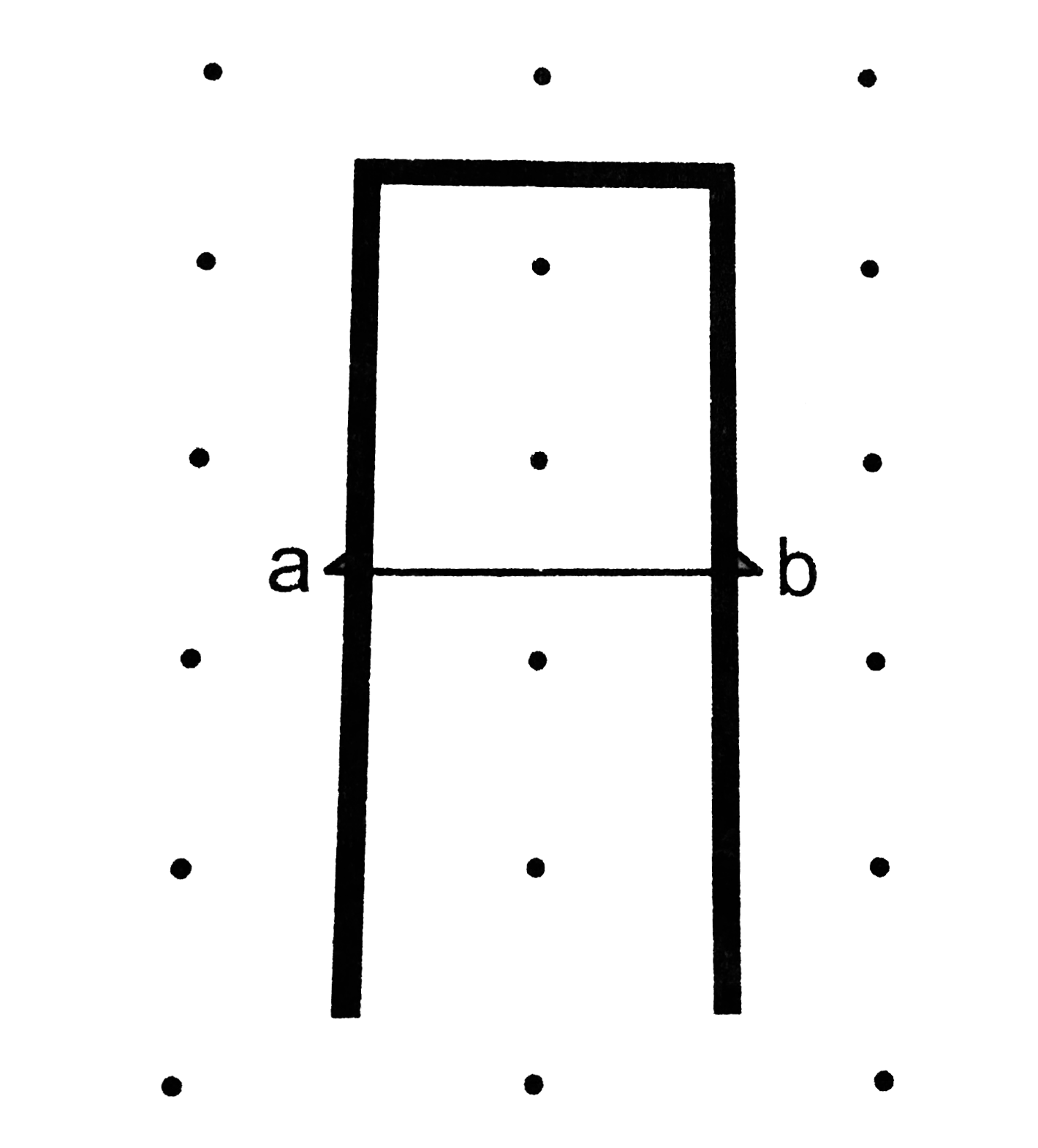
View SolutionA conducting wire ab of length l, resistance r and mass m starts sliding at t = 0 down a smooth, vertical, thick pair of connected rails as shown in . A uniform magnetic field B exists in the space in a diraction perpendicular to the plane of the rails. (a) Write the induced emf in the loop at an instant t when the speed of the wire is v. (b) what would be the magnitude and direction of the induced current in the wire? (c) Find the downward acceleration of the wire at this instant. (d) After sufficient time, the wire starts moving with a constant velocity. Find this velocity `v_m. (e) Find the velocity of the wire as a function of time. (f) Find the displacement of the wire as a functong of time. (g) Show that the rate of heat developed inte wire is equal to the rate at which the gravitational potential energy is decreased after steady state is reached.
View SolutionA bicycle is resting on its stand in the east - west direction and the rear wheel is rotated at an angular speed of 100 revolutions per minute. If the length of each spoke is 30.0 cm and the horizontal comonet of the earth's magnetic field is `2.0 X 10^(-5) T`, find the emf induced between the axis and the outer end of a spoke. Neglect centripetal force acting on the free electrons of the spoke.
View SolutionA conducting disc of radius r rotaes with a small but constant angular velocity `omega` about its axis. A uniform magnetic field B exists parallel to the axis of rotation. Find the motional emf between the centre and the periphery of the disc.
View Solutionshows a conducting disc rotating about its axis in a perpendicular magnetic field B. A resistor of resistance R is connected between the centre and the rim. Calculate the current in the resistor. Does it enter the disc or leave it at the centre? The radius of hte disc is 5.0 cm , angular speed `omega = 10 rad/s, B = 0.40 T` and `R = 10 Omega`.

View SolutionThe magnetic field inn a region is given by `vec B = veck (B_0)/(L) y where L is a fixed length. A conducting rod of length L lies along the Y - axis between the origin and the point (0,L, 0). If the rod moves with a velocity `v= v_0 veci, find the emf induced between the ends of the rod.
View Solutionshows a straight, long wire carrying a current I and a rod of length l coplanar with the wire and perpendicular to it. The rod moves with a constant velocity v in a direction parallel to the wire. The distance of the wire from the centre of the rod is x. Find the motional emf induced in the rod.
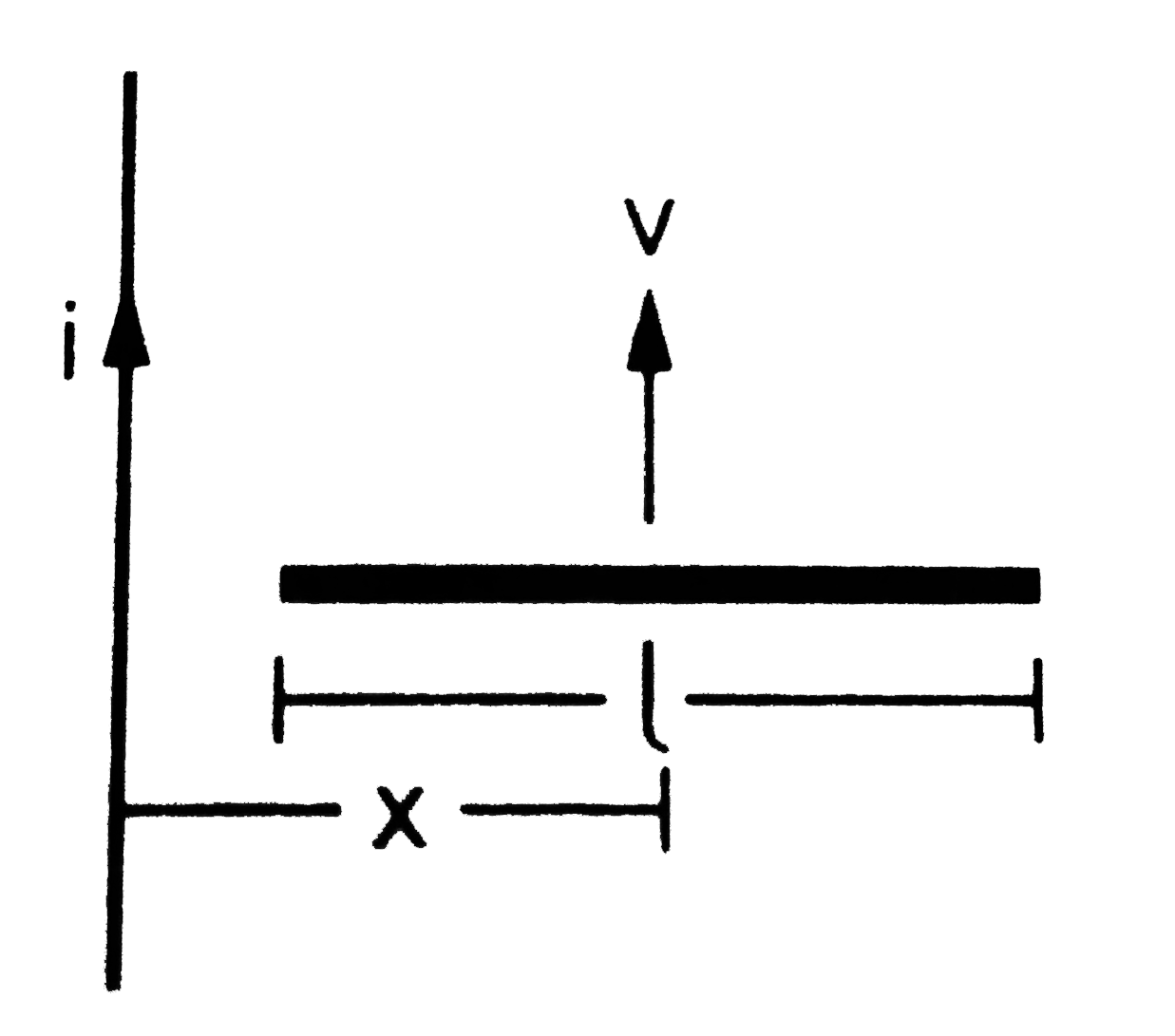
View SolutionConsider a situation similar ot that of the previous problem except that the ends of the rod slide on a pair of thick metallic rails laid parallel to the wire. At one end the rails are connected by resistor of resistance R. (a) what force is needed ot keep the rod sliding at a constant speed v? (b) in this situation what is the current in the resistance R? (c) Find the rate of heat developed in the resistor. (d) find the power delivered by the external agent exerting the force on the rod.
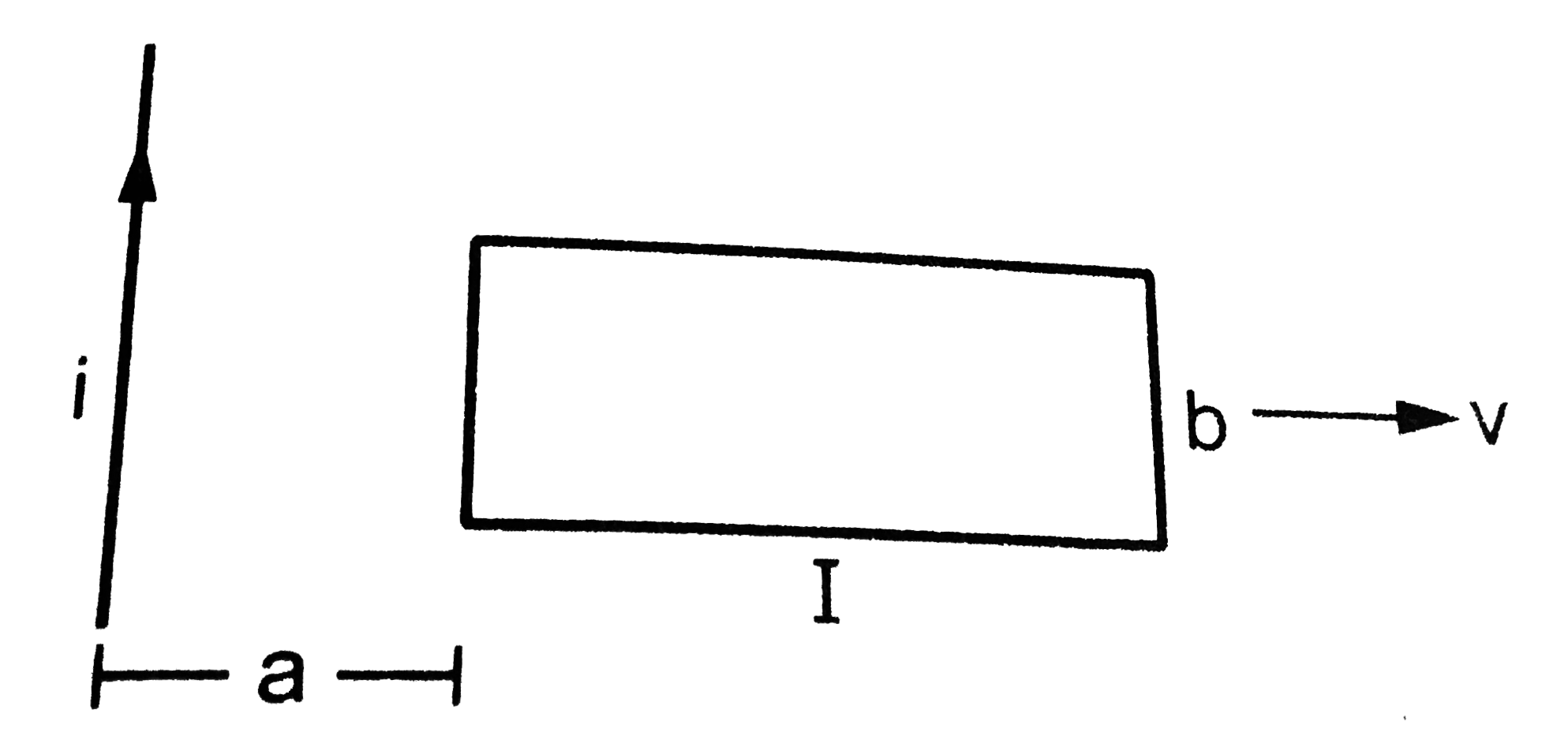
View SolutionShows a square frame of wire having a total resistance r placed coplanarly with a long, straight wire. The wire carries a current I given by `i= i_0 sin omega t`. Find(a) the flux of the magnetic field through the square frame, (b) the emf induced in the frame and (c) the heat developd in the frame in the time interval 0 to `(20pi)/(omega)`.
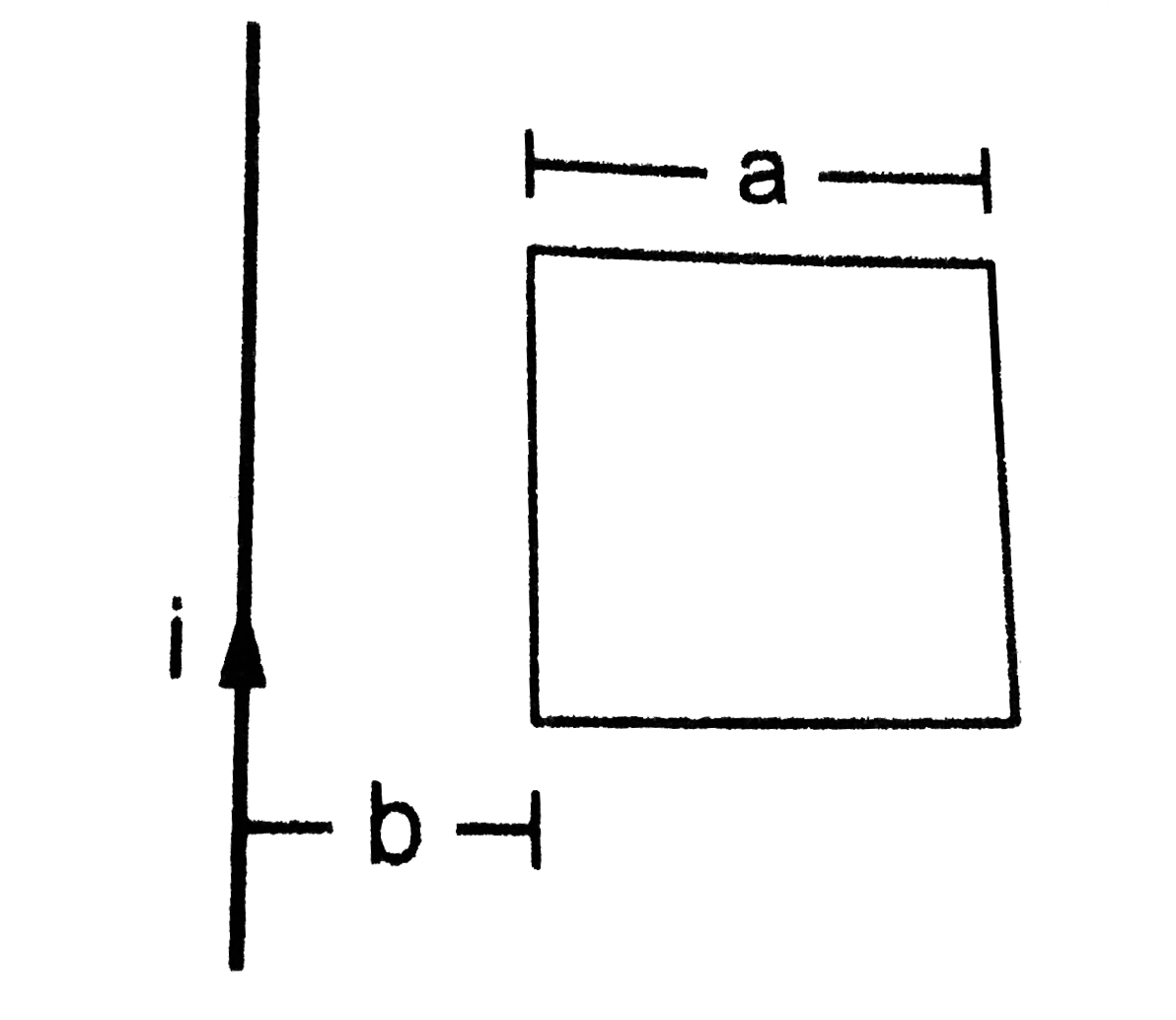
View SolutionA rectangular metallic loop of length l and width b is placed coplanerly with a long wire carrying a current I . The loop is moved perpendicular to the wire with a speed v in the plane containing the wire and the loop. Calculate the emf induced in the loop when the rear end of hte loop is at a distance a from the wire. Solve by unsing Faraday's law for the flux thrugh the loop and also by replacing different sagments with equivalent batteries.
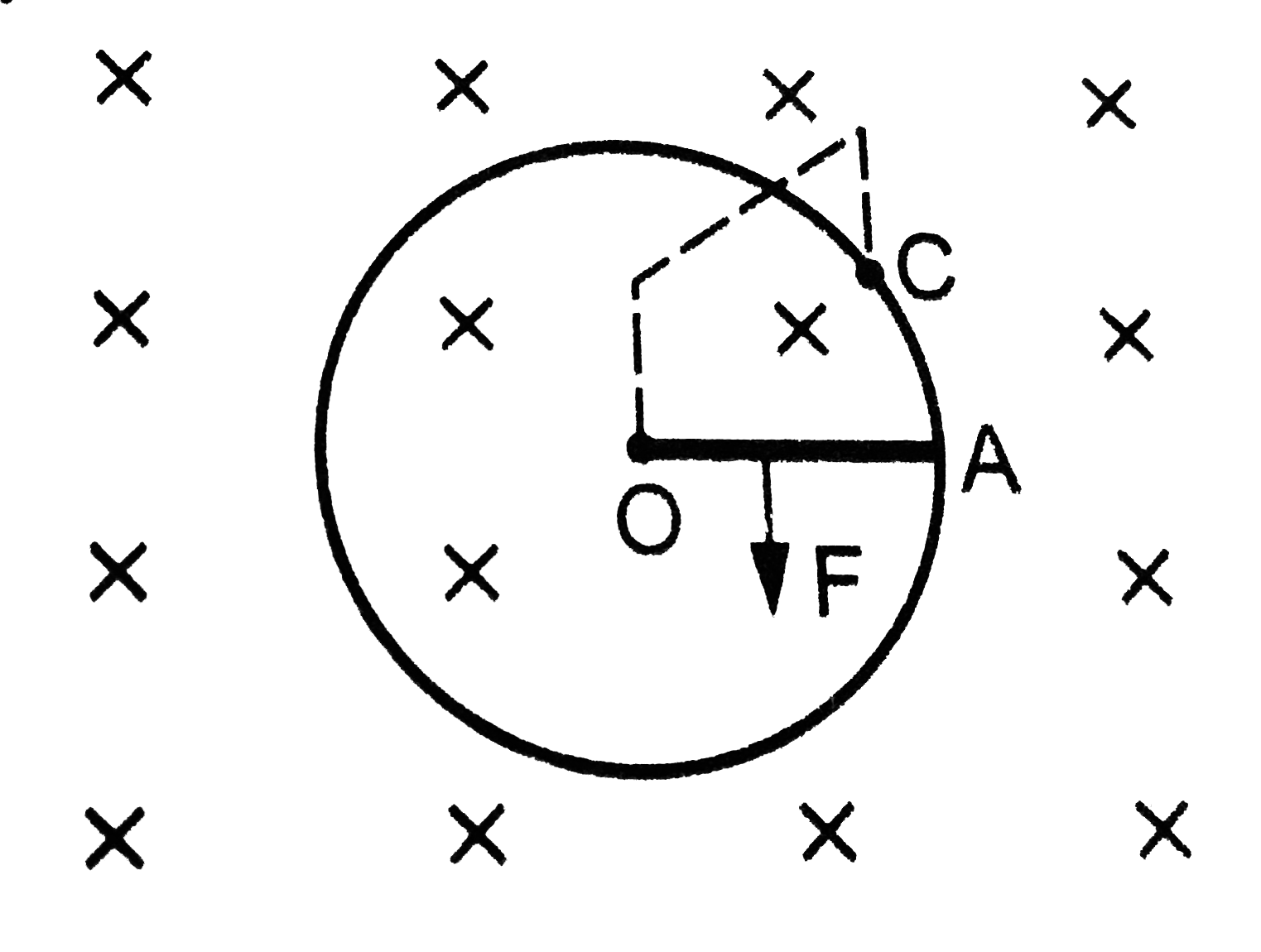
View SolutionShows a conducting circular loop of radius a placed in a uniform, perpendicular magnetic field B. A thick metal rod OA is pivoted at the centre O. The other end of the rod touches the loop at A. The centre O and a fixed point C on the loop are connected by a wire OC of resistance R. A force is applied at the middle point of the rod OA perpendicularly, so that the rod rotates clockwise at a uniform angular velocity `omega`. Find the force.
View SolutionConsider the situation shown in the figure of the previous problem. Suppose the wire connecting O and C has zero resistance but circular loop has a resistance R uniformly distributed along its length. The rod OA is made to rotate with a uniform angular speed `omega` as shown in the figure. Find the current in the rod when `ltAOC = 90^@`.
View SolutionConsider a variation of the previous problem. Suppose the circular loop lies in a vertical plane. The rod has a mass m. The rod and the loop have negligible resistances but the wire connecting O and C has a resistance R. The rod is made to rotate with a uniform angular velocity `omega` in the clockwise direction by applying a force at the midpoint of OA in a dirction perpendicular ot it. find the magnitude of this force when the rod makes an angle `theta` with the vertical.
View SolutionShows a situation similar to the previous problem. All parameters are the same except that a battery of emf `epsilon` and a variable resistance R are connected wires. Let `theta` be the angle made by the rod from the horizontal position (shown in the figurer), measuredin the clockwise direction. During the part of the motion `Oltthetaltpi/4` the only forces acting on the rod are gravity and the forces exerted by the magnetic field and the pivot. However, during the part of the motion, the resistance R is varied in such a way that the rod continues to rotate with a constant angular velocity `omega`. Find the value of R in terms of the given quantities.

View SolutionA wire of mass m and length l can slide freely on a pair of smooth, vertical rails. A magnetic field B exists in the region in the direction perpendicular to the plane of the rails. The rails are connected at the top end by a capacitor of capacitance C. Find the acceleration of the wire neglecting any electric resistance.
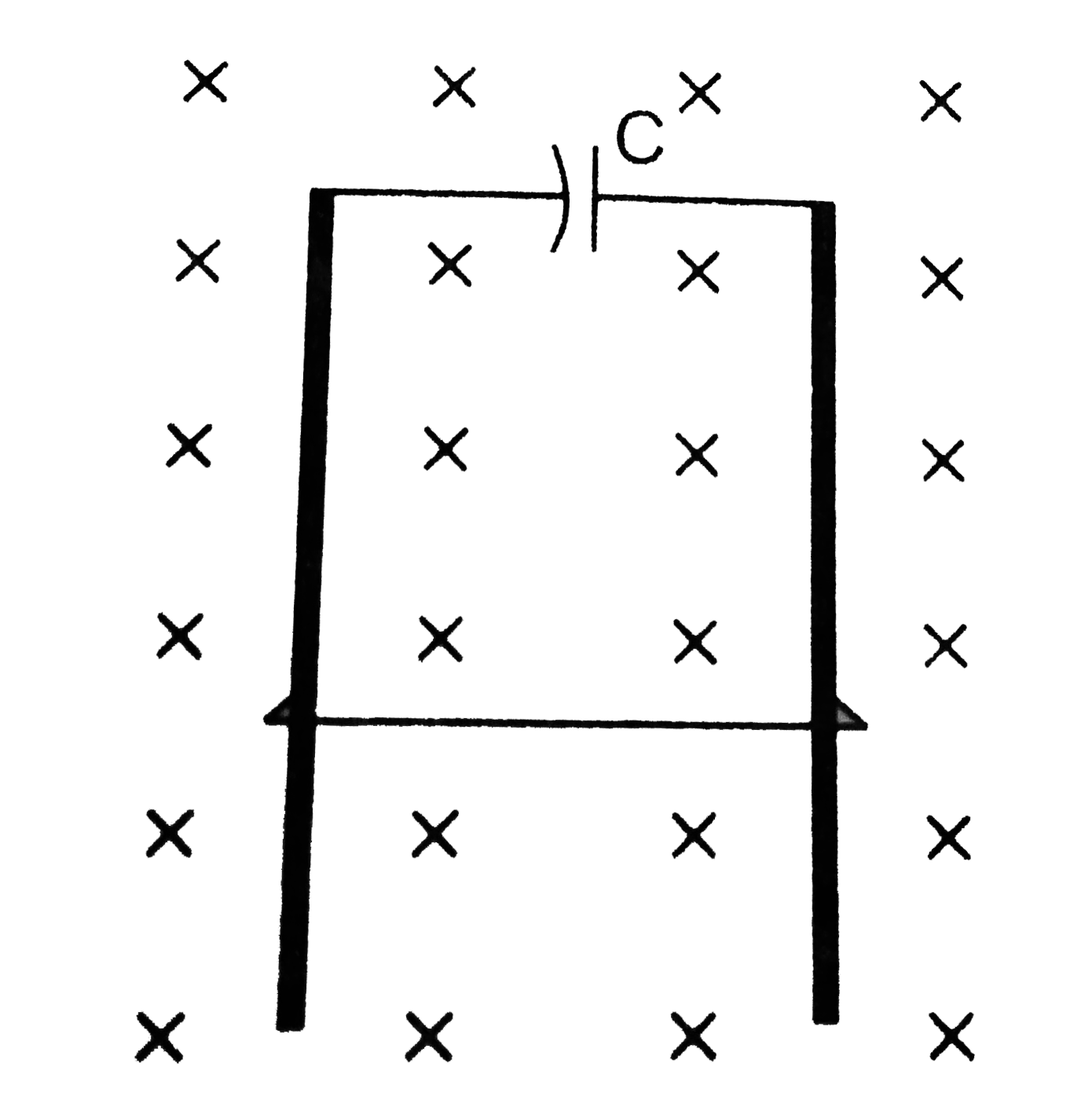
View SolutionA uniform magnetic field B exists in a cylindrical region, shown dotted in. The magnetic field increases at a constant rate `(dB)/(dt)` Consider a circle of radius r coaxial with the cylindrical region. (a) find the magnitude of the electric field E at a point on the circumference of the circle. (b) Consider a point P on the side of hte square circumscribing the circle. Show that the component of the induced electric field at P along ba is the same as the magnitude found in part(a).

View SolutionThe current in an ideal, long solenoid is varied at a uniform rate of `0.01 As^(-1). The solenoid has 2000 turns/m and its radius 6.0 cm.
(a) Consider a circle of radius 1.0 cm inside the solenoid with its axis coinciding with the axis of the solenoid. write the change in the magnetic flux through this circle in 2.0 seconds.
(b) find the electric field induced at a point on the circumference of the circle.
(c) find the electric field induced at a point outside the solenoid at a distance 8.0cm from its axis.
View SolutionAn average emf of 20 V is induced in an inductor when the current in it is changed from `2.5 A` in one direction to the same valute in the opposite direction in 0.1 s. Find the self inductance of the inductor.
View SolutionA magnetic flux of `8X10^(-4)` weber is linked with each turn of a 200 - turn coil when thereis an electric current of 4 A in it. Calculate the self inductance of the coil.
View SolutionThe current in a solenoid of 240 turns, having a length of 12 cm and a radius of 2 cm, changes at a rate of `0.8 A s^(-)`. Find the emf induced in it.
View SolutionFind the value of `t/tau` for which the current in an LR circuit builds up to (a) 90%, (b) 99% and ( c) 99.9% of the steady-state value.
View SolutionAn inductor coil carries a steady state current of 2.0 A when connected across an ideal battery of emf 4.0 V. if its inductance is 1.0 H, find the time constant of the circuit.
View SolutionA coil having inductance 2.0 H and resistance `20 Omega` is connected to a battery of emf 4.0 V. Find (a) the current a the instant 0.20 s after the connection Is made and (b) the magnetic energy at this instant.
View SolutionA coil of resistance `40 Omega` is connected across a 4.0 V battery. 0.10 s after the battery is connected, the current in the coil is 63 mA. Find the inductance of the coil.
View SolutionAn inductor of inducance 5.0 H, having a negligible resistance, is connected in series with a `100 Omega` resistor and a battery of emf 2.0 V. Find the potential difference across the resistor 20 ms after the circuit is switched on.
View SolutionThe time constant of an LR circuit is 40 ms. The circuit is connected at t= 0 and the steady state current is found ot be 2.0 A. Find the current at (a) t= 10 ms (b) t =20ms, (c ) t =100ms and (d) t = 1 s.
View SolutionAn LR circuit has L = 1.0 H `R =20 Omega`. It is connected across an emf of `2.0 V` at t=0. Find `di/dt` at (a) t =100ms, (b) t =200 ms and (c ) t = 1.0 s.
View SolutionWhat are the values of the self induced emf in the circuit of the previous problem at the times indicated therein?
View SolutionAn inductor-coil of inductance 20 mH having resistance `10 Omega` is joined to an ideal battery of emf 5.0 V. Find the rate of change of the induced emf at t=0, (b) t = 10 ms and (c ) t = 1.0s.
View SolutionAn LR circuit constains an inductor of 500 mH, a resistor of `25.O Omega` and an emf of 5.00 V in series. Find the potential difference across the resistor at t =(a) 20.0 ms, (b) 100 ms and ( c) 1.00 s.
View SolutionAn inductor coil of resistance `10 Omega` and inductance 120 mH is connected across a battery of emf 6 V and internal resistance `2 Omega`. Find the charge which flows through the inductor in (a) 10 ms, (b) 20ms and (c ) 100ms after the connections are made.
View SolutionAn inductor coil of inductance 17 mH is constructed from a copper wire of length 100 m and cross - sectional are `1mm^2`. Calculate the time constant of the circuit if this inductor is joined across an ideal battery. The resistivity of copper =`1.7 X 10^(-8) Omega m.
View SolutionAn LR circuit having a time constant of 50 ms is connected with an ideal battery of emf `epsilon` . Find the time taken for the magnetic energy stored in the circuti to change from one fourth of the steady state value to half of the steady stae value.
View SolutionA coil having an inductance `L` and a resistance `R` is connected to a battery of `emf epsilon`. Find the time elapsed before (a) the current reaches half its maximum value, (b) the power dissipated in heat reaches half its maximum value and © the magnetic field energy stored in the circuit reaches half its maximum value.
View SolutionA solenoid having inductance 4.0 H and resistance 10 Ω is connected to a 4.0 V battery at t = 0. Find (a) the time constant, (b) the time elapsed before the current reached 0.63 of its steady state value, (c ) the power delivered by the battery at this instant and (d) the power dissipated in Joule heating at this instant.
View SolutionThe magnetic field at a point inside a 2.0 mH inductor coil becomes 0.80 of its maximum value in `20 mu s ` when the inductor is joined to a battery. Find the resistance of the circuit.
View SolutionAn LR circuit with emf `epsilon` is connected at t = 0. (a) find the charge Q which flows through the battery during O to t. (b) Calculate the work done by the battery during this period. (d) find the magnetic field energy stored in the circuit at time t. (e) Verify that the results in the three parts above are consistent with enegy conservation.
View Solutionan inductor of inductance 2.00 H is joined in series with a resistor of resistance `200 Omega` and a battery of emf 2.00 V. At t = 10 ms, find (a) th current in the circuit, (b) the power delivered by the batter, (c ) the power dissipated in heating the resistor and (d) the rate at which energy is being stored in magnetic field.
View SolutionTwo coil A and B have inductances 1.0 H and 2.0 H respectively. The resistance of each coil is ` 10 Omega`. Each coil is connected to an ideal battery of emf `2.0 V at t = 0 Let i_A` and i_B be the current in the two circuit at time t. Find the raito `i_A/ I_B` at (a) t=100ms, (b) t= 200 ms and (c ) t = 1 s.
View SolutionThe current in a discharging LR circuit without the battery drops from 2.0 A to 1.0A in 0.10 s. (a) find the time constant of the circuit. (b) if the inductance of the circuit is 4.0 H, what is its resistance?
View SolutionA constant current exists in an inductor coil connected to a battery. The coil is short circuited and the battery is removed. Show that the charge flown through the coil after the short circuiting is the same as that which flows in one time constant before the short circuiting.
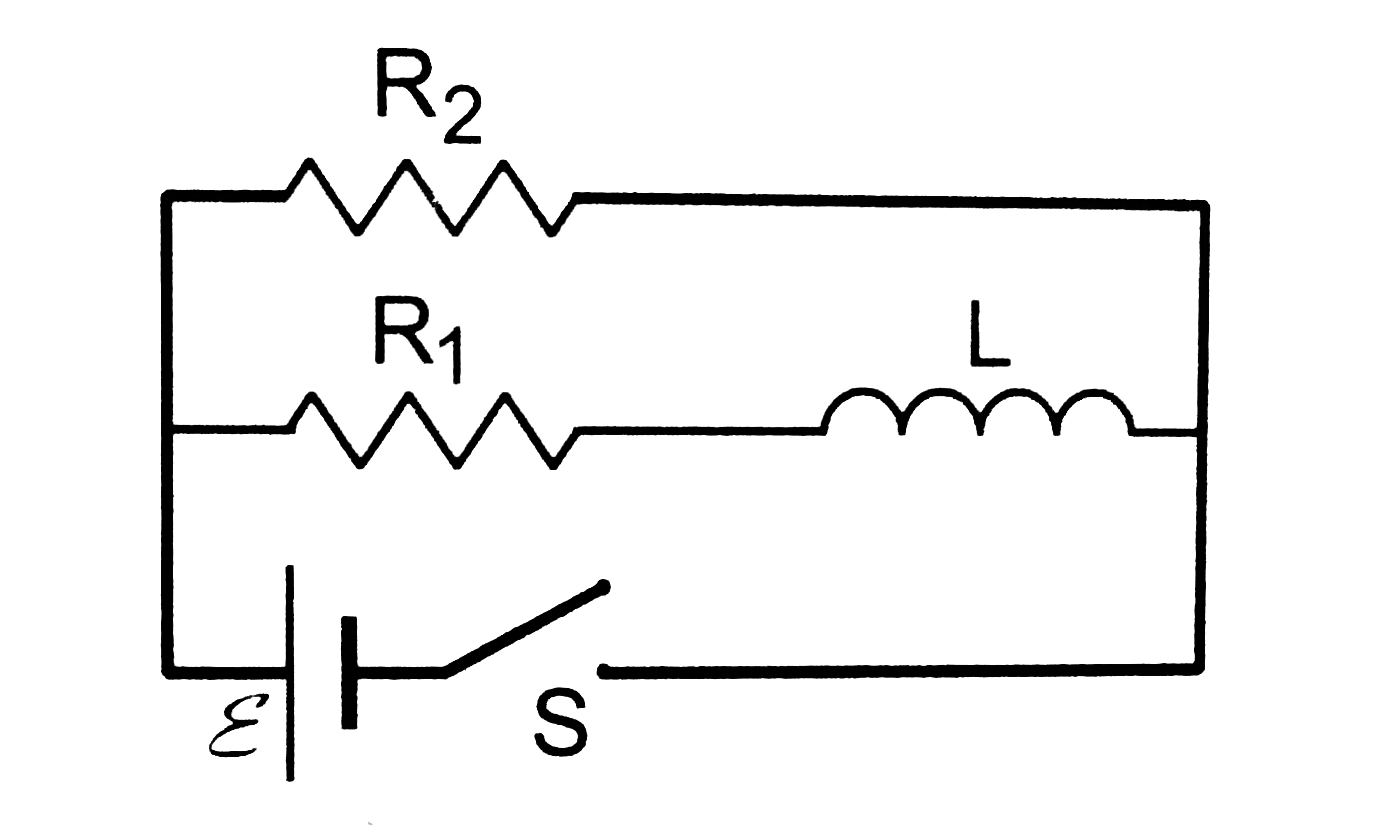
View SolutionConsider the circuit shown in figure.(a) find the current through the battery a long time after the switch S is closed. (b) Suppose the switch is again opened at t = 0. What is the time constant of the discharging circuit? (c ) Find t current through the inductor after one time constant.
View SolutionA current of 1.0 A is established in a tightly wound solenoid of radius 2 cm having 1000 turns/metre. Find the magnetic energy stored in each metre of the solenoid.
View SolutionConsider a small cube of volume `1 mm ^3` at the centre of a circular loop of radius 10 cm carrying a current of 4 A. Find the magnetic energy stored inside the cube.
View SolutionA long wire carries a current of 4.00 A. Find the energy stored in the magnetic field inside a volume of `1.0 mm^3` at a distance of 10.0 cm from the wire.
View SolutionThe mutual inductance between two coils is `2.5 H`. If the current in one coil is changed at the rate of `1 As^(-1)`, what will be the `emf` induced in the other coil?
View SolutionFind the mutual inductance between the straight wire and the square loop of figure.
View SolutionFind the mutual inductance between the circular coil and the loop shown in figure.
View SolutionA solenoid of length 20cm, area of cross- section `4.0 cm^2` and having 4000 turns is placed inside another solenoid of 2000 turns having a cross - sectional area `8.0cm ^2` and length `10 cm`. Find the mutual inductance between the solenoids.
View SolutionThe current in a long solenoid of radius R and having n turns per unit length is given by `i= i_0 sin omega t`. A coil having N turns is wound around it near the centre. Find (a) the induced emf in the coil and (b) the mutual inductance between the solenoid and the coil.
View Solution
EXERCISE questions and complete solutions for chapter ELECTROMAGNETIC INDUCTION of HC VERMA PHYSICS (ENGLISH) of Class 12
